- 1.04 MB
- 2022-04-22 11:28:04 发布
- 1、本文档共5页,可阅读全部内容。
- 2、本文档内容版权归属内容提供方,所产生的收益全部归内容提供方所有。如果您对本文有版权争议,可选择认领,认领后既往收益都归您。
- 3、本文档由用户上传,本站不保证质量和数量令人满意,可能有诸多瑕疵,付费之前,请仔细先通过免费阅读内容等途径辨别内容交易风险。如存在严重挂羊头卖狗肉之情形,可联系本站下载客服投诉处理。
- 文档侵权举报电话:19940600175。
'课后答案网您最真诚的朋友www.hackshp.cn网团队竭诚为学生服务,免费提供各门课后答案,不用积分,甚至不用注册,旨在为广大学生提供自主学习的平台!课后答案网:www.hackshp.cn视频教程网:www.efanjy.comPPT课件网:www.ppthouse.com课后答案网www.hackshp.cn
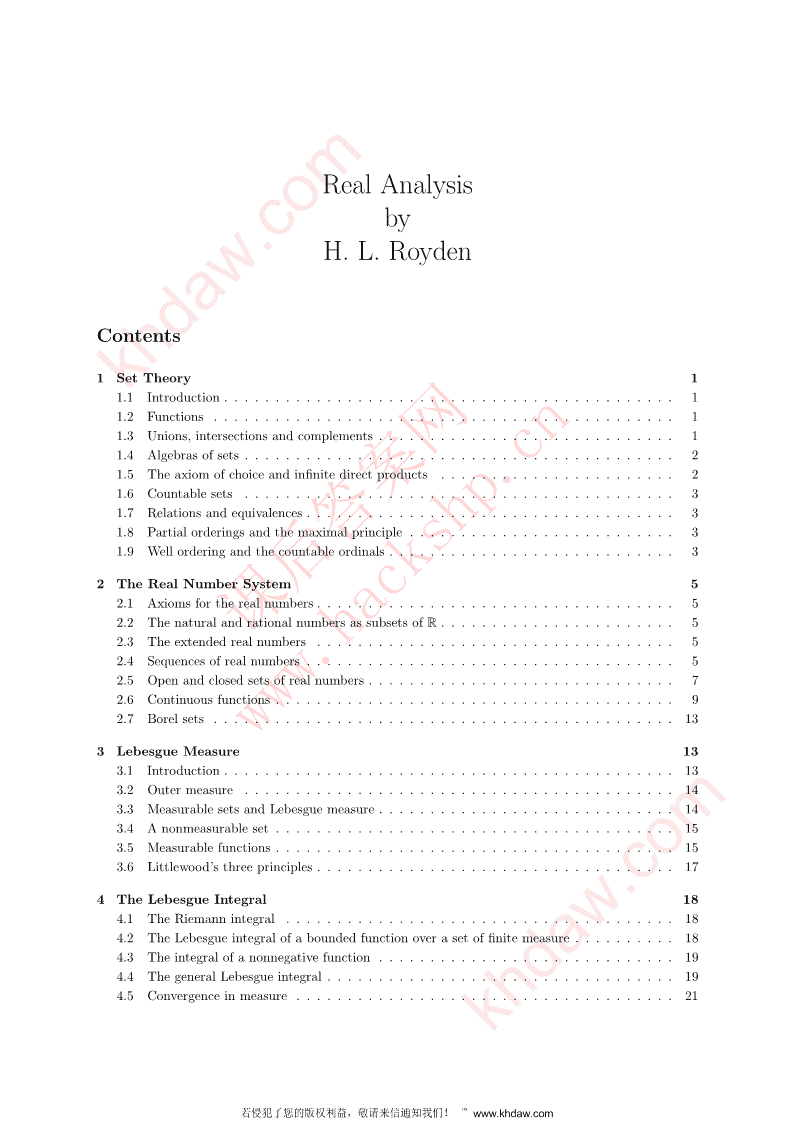
RealAnalysisbyH.L.RoydenContents1SetTheorykhdaw.com11.1Introduction............................................11.2Functions.............................................11.3Unions,intersectionsandcomplements.............................11.4Algebrasofsets..........................................21.5Theaxiomofchoiceandin
nitedirectproducts.......................21.6Countablesets..........................................31.7Relationsandequivalences....................................31.8Partialorderingsandthemaximalprinciple..........................31.9Wellorderingandthecountableordinals............................32TheRealNumberSystem52.1Axiomsfortherealnumbers...................................52.2Thenaturalandrationalnumbersassubsetsof课后答案网R.......................52.3Theextendedrealnumbers...................................52.4Sequencesofrealnumbers....................................52.5Openandclosedsetsofrealnumbers..............................72.6Continuousfunctions.......................................92.7Borelsets.............................................www.hackshp.cn133LebesgueMeasure133.1Introduction............................................133.2Outermeasure..........................................143.3MeasurablesetsandLebesguemeasure.............................143.4Anonmeasurableset.......................................153.5Measurablefunctions.......................................153.6Littlewood"sthreeprinciples...................................174TheLebesgueIntegral184.1TheRiemannintegral......................................184.2TheLebesgueintegralofaboundedfunctionoverasetof
nitemeasure..........184.3Theintegralofanonnegativefunction.............................194.4ThegeneralLebesgueintegral..................................194.5Convergenceinmeasure.....................................khdaw.com21若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
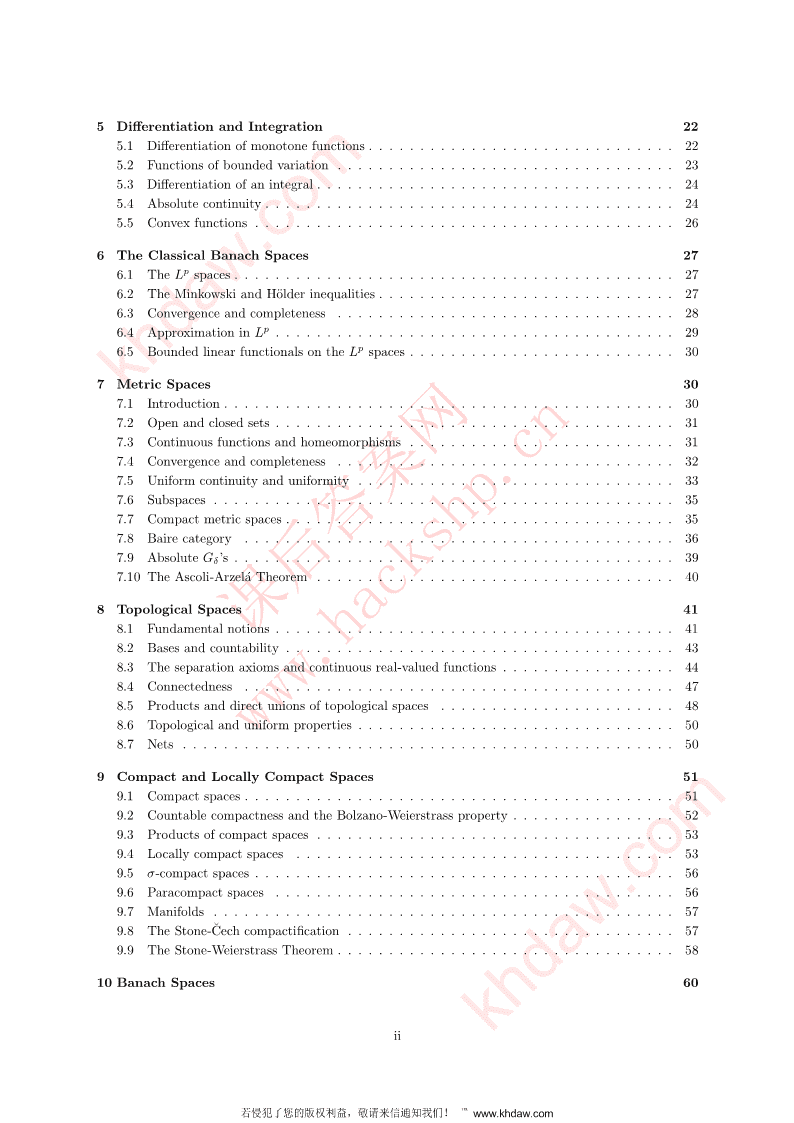
5Di
erentiationandIntegration225.1Di
erentiationofmonotonefunctions..............................225.2Functionsofboundedvariation.................................235.3Di
erentiationofanintegral...................................245.4Absolutecontinuity........................................245.5Convexfunctions.........................................266TheClassicalBanachSpaces276.1TheLpspaces...........................................276.2TheMinkowskiandH•olderinequalities.............................276.3Convergenceandcompleteness.................................286.4ApproximationinLp.......................................296.5BoundedlinearfunctionalsontheLpspaces..........................307MetricSpaceskhdaw.com307.1Introduction............................................307.2Openandclosedsets.......................................317.3Continuousfunctionsandhomeomorphisms..........................317.4Convergenceandcompleteness.................................327.5Uniformcontinuityanduniformity...............................337.6Subspaces.............................................357.7Compactmetricspaces......................................357.8Bairecategory..........................................367.9AbsoluteG"s...........................................397.10TheAscoli-ArzelaTheorem...................................408TopologicalSpaces418.1Fundamentalnotions.......................................课后答案网418.2Basesandcountability......................................438.3Theseparationaxiomsandcontinuousreal-valuedfunctions.................448.4Connectedness..........................................478.5Productsanddirectunionsoftopologicalspaces.......................488.6Topologicalanduniformproperties...............................www.hackshp.cn508.7Nets................................................509CompactandLocallyCompactSpaces519.1Compactspaces..........................................519.2CountablecompactnessandtheBolzano-Weierstrassproperty................529.3Productsofcompactspaces...................................539.4Locallycompactspaces.....................................539.5-compactspaces.........................................569.6Paracompactspaces.......................................569.7Manifolds.............................................579.8TheStone-Cechcompacti
cation................................579.9TheStone-WeierstrassTheorem.................................5810BanachSpaces60iikhdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
10.1Introduction............................................6010.2Linearoperators.........................................6110.3LinearfunctionalsandtheHahn-BanachTheorem......................6210.4TheClosedGraphTheorem...................................6310.5Topologicalvectorspaces....................................6410.6Weaktopologies.........................................6610.7Convexity.............................................6910.8Hilbertspace...........................................7111MeasureandIntegration7311.1Measurespaces..........................................7311.2Measurablefunctions.......................................7611.3Integration............................................7711.4Generalconvergencetheorems..................................khdaw.com7911.5Signedmeasures.........................................7911.6TheRadon-NikodymTheorem.................................8011.7TheLpspaces...........................................8212MeasureandOuterMeasure8312.1Outermeasureandmeasurability................................8312.2Theextensiontheorem......................................8412.3TheLebesgue-Stieltjesintegral.................................8512.4Productmeasures.........................................8612.5Integraloperators.........................................8912.6Innermeasure...........................................8912.7Extensionbysetsofmeasurezero................................9112.8Caratheodoryoutermeasure...................................课后答案网9112.9Hausdor
measures........................................9113MeasureandTopology9213.1BairesetsandBorelsets.....................................92www.hackshp.cniiikhdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
1SetTheory1.1Introduction1.Iffx:x6=xg6=;,thenthereexistsxsuchthatx6=x.Contradiction.2.;fgreen-eyedlionsg.3.X(YZ)=fhx;hy;ziig,(XY)Z=fhhx;yi;zig;hx;hy;zii$hhx;yi;zi$hx;y;zi.4.SupposeP(1)istrueandP(n))P(n+1)foralln.Supposethatfn2N:P(n)isfalseg6=;.Thenithasasmallestelementm.Inparticular,m>1andP(m)isfalse.ButP(1))P(2)))P(m).Contradiction.5.GivenanonemptysubsetSofnaturalnumbers,letP(n)bethepropositionthatifthereexistsm2Swithmn,thenShasasmallestelement.P(1)istruesince1willthenbethesmallestelementofS.SupposethatP(n)istrueandthatthereexistsm2Swithmn+1.Ifmn,thenShasasmallestelementbytheinductionhypothesis.Ifm=n+1,theneithermisthesmallestelementofSorthereexistsm02Swithm0x,thenS[fxg=X.Otherwise,fx2X:x>xghasaleastelementx0and000S[fxg=fx:x0sojxyj=xy=(�x)(�y)=jxjjyj.Ifx0andy<0,thenxy0sojxyj=�xy=x(�y)=jxjjyj.Similarlywhenx<0andy0.5b.Ifx;y0,thenx+y0sojx+yj=x+y=jxj+jyj.Ifx;y<0,thenx+y<0sojx+yj=�x�y=jxj+j课后答案网yj.Ifx0,y<0andx+y0,thenjx+yj=x+yx�y=jxj+jyj.Ifx0,y<0andx+y<0,thenjx+yj=�x�yx�y=jxj+jyj.Similarlywhenx<0andy0.5c.Ifx0,thenx�xsojxj=x=x_(�x).Similarlyforx<0.5d.Ifxy,thenx�y0sox_y=x=1(x+y+x�y)=1(x+y+jx�yj).Similarlyforyx.225e.Suppose�yxy.Ifwww.hackshp.cnx0,thenjxj=xy.Ifx<0,thenjxj=�xysince�yx.2.2ThenaturalandrationalnumbersassubsetsofRNoproblems2.3Theextendedrealnumbers6.IfE=;,thensupE=�1andinfE=1sosupE0,thereexistN1andN2suchthatjxn�l1j<"fornN1andjxn�l2j<"fornN2.Thenjl1�l2j<2"fornmax(N1;N2).Since"isarbitrary,jl1�l2j=0andl1=l2.Thecaseswhere1or�1isalimitareclear.8.Supposethereisasubsequencehxithatconvergestol2R.Thengiven">0,thereexistsN0suchnjthatjx�lj<"forjN0.GivenN,choosejN0suchthatnN.Thenjx�lj<"andlisanjjnjclusterpointofhxni.Conversely,supposel2Risaclusterpointofhxkhdaw.comni.Given">0,thereexistsn115若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
suchthatjxn1�lj<".Supposexn1;:::;xnkhavebeenchosen.Choosenk+11+max(xn1;:::;xnk)suchthatjxnk+1�lj<".Thenthesubsequencehxnjiconvergestol.Thecaseswherelis1or�1aresimilarlydealtwith.9a.Letl=limxn2R.Given">0,thereexistsn1suchthatxkl�".Thusjxk1�lj<".Supposen1;:::;njandxk1;:::;xkjhavebeenchosen.Choosenj+1>max(k1;:::;kj).Thenxkl�".Thusjxkj+1�lj<".Thenthesubsequencehxkjiconvergestolandlisaclusterpointofhxni.Iflis1or�1,itfollowsfromthede
nitionsthatlisaclusterpointofhxni.Ifl0isaclusterpointofhxiandl0>l,wemayassumethatl2R.Byde
nition,thereexistsNsuchnthatsupx<(l+l0)=2.Ifl02R,thensinceitisaclusterpoint,thereexistskNsuchthatkNkjx�l0j<(l0�l)=2sox>(l+l0)=2.Contradiction.Byde
nitionagain,thereexistsN0suchthatkksup0,thereexistsNsuchthatsupxx�".Thusjxk�xj<"forkmax(N;N)andhxniconvergestox.Ifx=1,thenlimxn=1soforanythereexistsNsuchthatinfkNxk>.i.e.xk>forkN.Thuslimxn=1.Similarlywhenx=�1.Thestatementdoesnotholdwhenthewordextended"isremoved.Thesequenceh1;1;1;2;1;3;1;4;:::ihasexactlyonerealnumber1thatisaclusterpointbutitdoesnotconverge.11a.Supposehxniconvergestol2R.Given">0,thereexistsNsuchthatjxn�lj<"=2fornN.Nowifm;nN,jxn�xmjjxn�lj+jxm�lj<".ThushxniisaCauchysequence.11b.LethxnibeaCauchysequence.ThenthereexistsNsuchthatjjxnj�jxmjjjxn�xmj<1form;nN.Inparticular,jjxnj�jxNjj<1fornN.Thusthesequencehjxnjiisboundedabovebymax(jx1j;:::;jxN�1j;jxNj+1)sothesequencehxniisbounded.11c.SupposehxniisaCauchysequencewithasubsequencehxnkithatconvergestol.Given">0,thereexistsNsuchthatj课后答案网xn�xmj<"=2form;nNandjxnk�lj<"=2fornkN.NowchooseksuchthatnkN.Thenjxn�ljjxn�xnkj+jxnk�lj<"fornN.Thushxniconvergestol.11d.IfhxniisaCauchysequence,thenitisboundedbypart(b)soithasasubsequencethatconvergestoarealnumberlbyQ9b.Bypart(c),hxniconvergestol.Theconverseholdsbypart(a).12.Ifx=limxn,theneverysubsequenceofhxnialsoconvergestox.Conversely,supposeeverysubsequenceofhxnihasinturnasubsequencethatconvergestox.Ifx2Randhxnidoesnotconvergetox,thenthereexists">0suchthatforallwww.hackshp.cnN,thereexistsnNwithjxn�xj".Thisgivesrisetoasubsequencehxnkiwithjxnk�xj"forallk.Thissubsequencewillnothaveafurthersubsequencethatconvergestox.Ifx=1andlimxn6=1,thenthereexistssuchthatforallN,thereexistsnNwithxn<.Thisgivesrisetoasubsequencehxnkiwithxnk<forallk.Thissubsequencewillnothaveafurthersubsequencewithlimit1.Similarlywhenx=�1.13.Supposel=limxn.Given",thereexistsnsuchthatsupknxkl�"sothereexistsknsuchthatxk>l�".Conversely,supposeconditions(i)and(ii)hold.By(ii),forany"andn,supknxkl�".Thussupknxklforalln.Furthermoreby(i),ifl0>l,thenthereexistsnsuchthatx.Thusthereexistsknsuchthatxk>.Conversely,supposethatgivenandn,thereexistsknsuchthatxk>.Letxn1=x1andletnk+1>nkbechosensuchthatxnk+1>xnk.Thenlimxnk=1solimxn=1.15.Forallm0andsupknxkyksupknxksupknyk.Now,takinglimits,wegetlimxnynlimxnlimyn.18.Sinceeachxv0,thesequencePhsniismonotoneincreasing.Ifthesequencehsniisbounded,1thenlimPsn=supsn2Rsosupsn=v=1xv.Ifthesequencehsniisunbounded,thenlimsn=1so11=v=1xv.PnP119.Foreachn,lettn=v=1jxvj.Sincev=1jxvj<1,thesequencehtniisaCauchysequencesogiven",thereexistsNsuchthatjtn�tmj<"forn>mN.Thenjsn�smj=jxm+1++xnjjxm+1j++jxnj=jtn�tmj<"forn>mN.ThusthesequencehsniisaCauchysequencesoitconvergesandhxnihasasum.khdaw.comP1PnPn20.x1+v=1(xv+1�xv)=x1+limnv=1(xv+1P�xv)=limn[x1+v=1(xv+1�xv)]=limnxn+1=1limnxn.Thusx=limnxnifandonlyifx=x1+v=1(xv+1�xv).P21a.Supposex2Ex<1.Foreachn,letEn=fx2E:x1=ng.TheneachEnisa
nitesubsetofE.Otherwise,ifEn0isanin
nitesetforsomePn0,thenlettingFkbeasubsetofEn0withSkn0elementsforeachk2N,sFkk.Thenx2ExsFkkforeachk.Contradiction.NowE=EnsoEiscountable.21b.Clearly,fx1;:::;xng2Fforalln.ThussupsnsupF2FsF.Ontheotherhand,givenFP2F,thereexistsnsuchthatPFfx1;:::;xngsosFsnandsupF2FsFsupsn.Hence1x2Ex=supF2FsF=supsn=n=1xn.22.Givenx2R,leta1bethelargestintegersuchthat0a1nalsosatis
esx=1b.nmnmn=1nPnkConversely,ifPhaniisasequenceofintegerswith0an0,thereisarationalnumberrwithx0,thereisanirrationalnumberswithx0suchthat(x�;x+)X.ThusthesetS=fy:[x;y)Xgisnonempty.Suppose[x;y)6Xforsomey>x.ThenSisboundedabove.Letb=supS.Thenb2SandbisapointofclosureofXsob2XsinceXisclosed.ButsinceXisopen,thereexists0>0suchthat(b�0;b+0)X.Then[x;b+0)=[x;b)[[b;b+0)X.Contradiction.Thus[x;y)Xforally>x.Similarly,(z;x]Xforallz0,chooseNsuchthat1=N<".Thenjyn�xj<1=N<"fornNsohyniisasequenceinEwithx=limyn.Conversely,supposethereisasequencehyniinEwithx=limyn.Thenforany>0,thereexistsNsuchthatjx�ynj<fornN.Inparticular,jx�yNj<.ThusxisapointofclosureofE.28.LetxbeapointofclosureofE0.Given>0,thereexistsy2E0suchthatjx�yj<.Ify=x,thenx2E0andwearedone.Supposey6=x.Wemayassumey>x.Let0=min(y�x;x+�y).Thenx=2(y�0;y+0)and(y�0;y+0)(x�;x+).Sincey2E0,thereexistsz2Enfygsuchthatz2(y�0;y+0).Inparticular,z2Enfxgandjz�xj<.Thusx2E0.HenceE0isclosed.29.ClearlyEEandE0EsoE[E0E.Conversely,letx2Eandsupposex=2E.Thengiven>0,thereexistsy2Esuchthatjy�xj<.Sincex=2E,y2Enfxg.Thusx2E0andEE[E0.30.LetEbeanisolatedsetofrealnumbers.Foranyx2E,thereexistsx>0suchthatjy�xjxforally2Enfxg.WemaytakeeachxtoberationalandletIx=fy:jy�xj<xg.ThenfIx:x2Egisacountablecollectionofopenintervals,eachIxcontainsonlyoneelementofE,namelyx,andSEkhdaw.comx2EIx.IfEisuncountable,thenIx0willcontaintwoelementsofEforsomex0.Contradiction.ThusEiscountable.31.Letx2R.Given>0,thereexistsr2Qsuchthatx0suchthatfy:jy�xj<gAifandonlyifeverypointofAisaninteriorpointofA.ThusAisopenifandonlyifA=A.34b.Supposex2A.Thenthereexists>0suchthat(x�;x+)A.Inparticular,x=2Acandx=2(Ac)0.Thusx2(Ac)c.Conversely,supposex2(Ac)c.Thenx2AandxisnotanaccumulationpointofAc.Thusthereexists课后答案网>0suchthatjy�xjforally2Acnfxg=Ac.Hence(x�;x+)Asox2A.35.LetCbeacollectionofclosedsetsofrealnumberssuchthatevery
nitesubcollectionofChasTnonemptyintersectionandsupposeoneofthesetsF2Cisbounded.SupposeF=;.ThenSF2CFc=RF.BytheHeine-BorelTheorem,thereisa
nitesubcollectionfF;:::;FgCsuchF2CSnTnT1nthatFFc.ThenFF=;.Contradiction.HenceF6=;.i=1iwww.hackshp.cni=1iF2C36.LethFnibeasequenceofnonemptyclosedsetsofrealnumberswithFn+1Fn.Thenforany
niteTksubcollectionfFn1;:::;FTnkgwithn1<0,chooseNsuchthat1=3N<.NowlethbibethesequencennwithbN+1=jaN+1�2jandbn=anforn6=N+1.Letybethenumberwithternaryexpansionhbni.Theny2Cnfxgandjx�yj=2=3N+1<1=3N<.Thusx2C0.HenceC=C0.2.6Continuousfunctions40.SinceFisclosed,Fcisopenanditistheunionofacountablecollectionofdisjointopenintervals.Takegtobelinearoneachoftheseopenintervalsandtakeg(x)=f(x)forx2F.Thengisde
nedandcontinuousonRandg(x)=f(x)forallx2F.41.SupposefiscontinuousonE.LetObeanopensetandletx2f�1[O].Thenf(x)2Osothereexists"x>0suchthat(f(x)�"x;f(x)+"x)O.Sincefiscontinuous,thereexistsx>0suchthatjf(y)�f(x)j<"wheny2Eandjy�xj<.Hence(x�;x+)Ef�1[O].LetSxxxxU=khdaw.com(x�;x+).ThenUisopenandf�1[O]=EU.Conversely,supposethatforx2f�1[O]xxeachopensetO,thereisanopensetUsuchthatf�1[O]=EU.Letx2Eandlet">0.ThenO=(f(x)�";f(x)+")isopensothereisanopensetUsuchthatf�1[O]=EU.Nowx2UandUisopensothereexists>0suchthat(x�;x+)U.ThusE(x�;x+)f�1[O].i.e.foranyy2Ewithjy�xj<,jf(y)�f(x)j<".ThusfiscontinuousonE.42.SupposehfniisasequenceofcontinuousfunctionsonEandthathfniconvergesuniformlytofonE.Given">0,thereexistsNsuchthatforallx2EandnN,jfn(x)�f(x)j<"=3.Also,thereexists>0suchthatjfN(y)�fN(x)j<"=3ify2Eandjy�xj<.Nowify2Eandjy�xj<,thenjf(y)�f(x)jjf(y)�fN(y)j+jfN(y)�fN(x)j+jfN(x)�f(x)j<".ThusfiscontinuousonE.*43.fisdiscontinuousatthenonzerorationals:Givenanonzerorationalq,let"=jf(q)�qj>0.Ifq>0,givenany>0,pickanirrationalx2(q;q+).Thenjf(x)�f(q)j=x�f(q)>q�f(q)=".Ifq<0,givenany>0,pickanirrationalx2(q�;q).Thenjf(x)�f(q)j=f(q)�x>f(q)�q=".fiscontinuousat0:Given">0,let=".Thenwhen课后答案网jxj<,jf(x)jjxj<".fiscontinuousateachirrational:Letxbeirrational.FirstweshowthatforanyM,thereexists>0suchthatqMforanyrationalp=q2(x�;x+).Otherwise,thereexistsMsuchthatforanyn,thereexistsarationalpn=qn2(x�1=n;x+1=n)withqn0,chooseMsuchthatM2>max(jx+1j;jx�1j)=6".Thenchoose>0suchthat0andx0,choose>0suchthatjf(x)�f(y)j<"=2andjg(x)�g(y)j<"=2wheneverjx�yj<.Thenj(f+g)(x)�(f+g)(y)j=jf(x)�f(y)+g(x)�g(y)jjf(x)�f(y)j+jg(x)�g(y)j<"wheneverjx�yj<.Thusf+giscontinuousatx.Nowchoose0>0suchthatjg(x)�g(y)j<"=2jf(x)jandjf(x)�f(y)j<"=2max(jg(x)�"=2jf(x)jj;jg(x)+"=2jf(x)jj)wheneverjx�yj<0.Thenj(fg)(x)�(fg)(y)j=jf(x)g(x)�f(x)g(y)+f(x)g(y)�f(y)g(y)jjf(x)jjg(x)�g(y)j+jf(x)�f(y)jjg(y)j<"wheneverjx�yj<0.Thusfgiscontinuousatx.44b.Letfandgbecontinuousfunctions.Given">0,thereexists>0suchthatjf(a)�f(b)j<"wheneverja�bj<.Therealsoexists0>0suchthatjg(x)�g(y)j<wheneverjx�yj<0.Thusj(fg)(x)�(fg)(y)j=jf(g(x))�f(g(y))j<"whenjx�yj<0.Thusfgiscontinuousatx.9khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
44c.Letfandgbecontinuousfunctions.Given">0,choose>0suchthatjf(x)�f(y)j<"=2andjg(x)�g(y)j<"=2wheneverjx�yj<.Nowj(f_g)(x)�(f_g)(y)jjf(x)�f(y)j+jg(x)�g(y)j<".Thusf_giscontinuousatx.Furthermore,f^g=f+g�(f_g)sof^giscontinuousatx.44d.Letfbeacontinuousfunction.Thenjfj=(f_0)�(f^0)sofiscontinuous.45.Letfbeacontinuousreal-valuedfunctionon[a;b]andsupposethatf(a)f(b).LetS=fx2[a;b]:f(x)g.ThenS6=;sincea2SandSisbounded.Letc=supS.Thenc2[a;b].Iff(c)<
,thereexists>0suchthat
,thereexists0>0suchthat0
andx=2Sforallsuchx.Contradiction.Hencef(c)=.46.Letfbeacontinuousfunctionon[a;b].Supposefisstrictlymonotone.Wemayassumefisstrictlymonotoneincreasing.Thenfisone-to-one.Also,bytheIntermediateValueTheorem,fmaps[a;b]onto[f(a);f(b)].Letg=f�1:[f(a);f(b)]![a;b].Theng(f(x))=xforallx2[a;b].Lety2[f(a);f(b)].Theny=f(x)forsomex2[a;b].Given">0,choose>0suchthat0suchthatjf(x)�f(y)j<"=2wheneverx;y2[a;b]andjx�yj<.ChooseNsuchthat(b�a)=N<andletxi=a+i(b�a)=Nfori=1;:::;N.Nowde
ne"on[a;b]tobelinearoneach[xi;xi+1]with"(xi)=f(xi)foreachisothat"ispolygonalon[a;b].Letx2[a;b].Thenx2[xi;xi+1]forsomei.Wemayassumef(xi)f(xi+1).Then"(xi)"(x)"(xi+1)andj"(x)�f(x)jj"(x)�"(xi)j+j"(xi)�f(x)jj"(xi+1)�"(xi)j+jf(xi)�f(x)j<".48.Supposex2[0;1]isoftheformq=3n0withq=1or2.Thenxhastwoternaryexpansionshaiandha0iwherea=q,a=0forn6=n,a0=q�1,a0=0fornnP0.Ifq=1,thenfromthe
rstexpansionwegetN=n0,bN=1andbn=0fornnsoNb=2n=11=2n=1=2n0.Ifq=2,thenfromthe
rstexpansionwegetN=1,0n=1nn=n0+1PNnP1nn0bn0=1andbn=0forn6=n0son=1bn=2=Pn=1bn=2=1=2.FromthesecondexpansionwegetN=n,b=1andb=0forn0,chooseMsuchthat1=2<".Nowchoose>0suchthat<1=3M+2.Thenwhenjx�yj<,jf(x)�f(y)j<".Hencefismonotoneandcontinuouson[0;1].EachintervalcontainedinthecomplementoftheCantorternarysetconsistsofelementswithternaryexpansionscontaining1.Furthermore,foranyx;yinthesameintervalandhavingternaryexpansionshaiandha0irespectively,thesmallestnsuchthata=1isalsothesmallestsuchthata0=1.Hencefnnnnisconstantoneachsuchinterval.Foranyy2[0;1],lethbnibeitsbinaryexpansion.Takex2[0;1]withternaryexpansionhanisuchthatan=2bnforalln.ThenxisintheCantorternarysetandf(x)=y.ThusfmapstheCantorternarysetonto[0;1].49a.Supposelimf(x)A.Given">0,thereexists>0suchthatsupf(x)0some>0suchthatf(x)A+"forallxwith00suchthatf(x)A+1=nforallxwith0000and>0,supf(x)>A�"sothereexistsxsuchthatx!y00and>0,thereexistsxsuchthat00,supf(x)A�1=nforallnsosupf(x)A.Hence0000,if1<2,theninff(x)inff(x)supf(x)00sosupinff(x)infsupf(x).i.e.>0000000,thereexistsx!yx!y1>0suchthatsupf(x)0suchthatinff(x)>L�".i.e.f(x)>L�"whenever00suchthatjf(x)�Lj<"wheneverx!y00,x!ythereexistsNsuchthat00,infsupf(xn)supf(xn)NnNnNsupf(x)soinfsupf课后答案网(xn)infsupf(x).i.e.limf(xn)limf(x)=A.0000suchthatf(x)A�1=n.Thus00,x!ythereexists>0suchthatjf(x)�lj<"whenever00suchthatforeachnthereexistsxnwith00,thereexists>0suchthatx!y�f(x)�f(y)+"whenever00,thereexists>0suchthat�f(x)�f(y)+"wheneverjx�yj<ifandonlyifgiven">0,thereexists>0suchthatf(y)f(x)+"wheneverjx�yj<.50b.Supposefisbothupperandlowersemicontinuousaty.Thenlimf(x)f(y)limf(x).Thusx!yx!ylimf(x)existsandequalsf(y)sofiscontinuousaty.Conversely,iffiscontinuousaty,thenlimf(x)x!yx!yexistsandequalsf(y).Thuslimf(x)=limf(x)=f(y)sofisbothupperandlowersemicontinuousx!yx!y11khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
aty.Theresultforintervalsfollowsfromtheresultforpoints.50c.Letfbeareal-valuedfunction.Supposefislowersemicontinuouson[a;b].For2R,considerthesetS=fx2[a;b]:f(x)g.LetybeapointofclosureofS.Thenthereisasequencehxniwithxn2Sandy=limxn.Thusf(y)limf(xn)soy2S.HenceSisclosedsofx2[a;b]:f(x)>gisopen(in[a;b]).Conversely,supposefx2[a;b]:f(x)>gisopen(in[a;b])foreach2R.Lety2[a;b].Given">0,thesetfx2[a;b]:f(x)>f(y)�"gisopensothereexists>0suchthatf(x)>f(y)�"wheneverjx�yj<.i.e.f(y)0,thereexists>0suchthatf(y)f(x)+"=2andg(y)g(x)+"=2wheneverjx�yj<.Thus(f_g)(y)(f_g)(x)+"and(f+g)(y)(f+g)(x)+"wheneverjx�yj<.Bypart(a),f_gandf+garelowersemicontinuous.50e.Lethfnibeasequenceoflowersemicontinuousfunctionsandde
nef(x)=supnfn(x).Given">0,f(y)�"=20suchthatfn(y)fn(x)+"=2wheneverjx�yj<.Thusf(y)fn(x)+"f(x)+"wheneverjx�yj<.Hencefislowersemicontinuous.50f.khdaw.comLet":[a;b]!Rbeastepfunction.Suppose"islowersemicontinuous.Letciandci+1bethevaluesassumedby"in(xi�1;xi)and(xi;xi+1)respectively.Foreachn,thereexists>0suchthat"(xi)"(x)+1=nwheneverjx�xij<.Inparticular,"(xi)ci+1=nand"(xi)ci+1+1=nforeachn.Thus"(xi)min(ci;ci+1).Conversely,suppose"(xi)min(ci;ci+1)foreachi.Let">0andlety2[a;b].Ify=xiforsomei,let=min(xi�xi�1;xi+1�xi).Thenf(y)=f(xi)f(x)+"wheneverjx�yj<.Ify2(xi;xi+1)forsomei,let=min(y�xi;xi+1�y).Thenf(y)cgwithc2Zformanopencoveringof[a;b]sobytheHeine-BorelTheorem,thereisa
nitesubcovering.Thusthereexistsc2Zsuchthatf(x)>cforallx2[a;b].Nowforeachn,letx(n)=a+k(b�a)=2nandkletI(n)=(x(n);x(n))fork=0;1;2;:::;2n.De
ne"(x)=inff(x)ifx2I(n)and"(x(n))=kk�1knx2I(n)knkk(n)(n)(n)(n)(n)min(ck;ck+1;f(xk))whereck=infx2I(n)f(x)andck+1=infx2I(n)f(x).Theneach"nisalower课后答案网kk+1semicontinuousstepfunctionon[a;b]bypart(f)andf"n+1"nforeachn.Lety2[a;b].Given">0,thereexists>0suchthatf(y)f(x)+"wheneverjx�yj<.ChooseNsuchthat1=2N<.(n)(n)FornN,ify2Ikforsomek,then"n(y)=infx2I(n)f(x)f(y)�"sinceIk(y�;y+).k(n)(n)(n)Thusf(y)�"n(y)".Ify=xkforsomek,then"n(y)=f(y)�"sinceIk[Ik+1(y�;y+).Thusf(y)�"n(y)".Hencef(y)=lim"n(y).*50h.Letfbeafunctionde
nedon[www.hackshp.cna;b].Supposethereisamonotoneincreasingsequencehniofcontinuousfunctionson[a;b]suchthatforeachx2[a;b]wehavef(x)=limn(x).Theneachnislowersemicontinuousbypart(b)andf(x)=supnn(x).Bypart(e),fislowersemicontinuous.Conversely,supposethatfislowersemicontinuous.Bypart(g),thereisamonotoneincreasingsequenceh"nioflowersemicontinuousstepfunctionson[a;b]withf(x)=lim"n(x)foreachx2[a;b].Foreachn,de
nenbylinearising"nataneighbourhoodofeachpartitionpointsuchthatnn+1and0"n(x)�n(x)<"=2foreachx2[a;b].Thenhniisamonotoneincreasingsequenceofcontinuousfunctionson[a;b]andf(x)=limn(x)foreachx2[a;b].(*)Morerigorousproof:De
nenbyn(x)=infff(t)+njt�xj:t2[a;b]g.Thenn(x)infff(t)+njt�yj+njy�xj:t2[a;b]g=n(y)+njy�xj.Thusnis(uniformly)continuouson[a;b].Alsonn+1fforalln.Inparticular,f(x)isanupperboundforfn(x):n2Ng.Nowif
0suchthatf(y)f(y)+njy�xjwheneverjy�xj<.Ontheotherhand,whenjy�xj,wehavef(y)+nf(y)+njy�xjforsucientlylargen.Thusn(x)forsucientlylargen.Hencef(x)=supn(x)=limn(x).50i.Letfbealowersemicontinuousfunctionon[a;b].Thesetsfx2[a;b]:f(x)>cgwithc2Zformanopencoveringof[a;b]sobytheHeine-BorelTheorem,thereisa
nitesubcovering.Thusthereexistsc2Zsuchthatf(x)>cforallx2[a;b].Hencefisboundedfrombelow.Letm=infx2[a;b]f(x),which12khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
is
nitesincefisboundedfrombelow.Supposef(x)>mforallx2[a;b].Foreachx2[a;b],thereexistsx>0suchthatf(x)f(y)+m�f(x)fory2Ix=(x�x;x+x).TheopenintervalsfIx:x2[a;b]gformanopencoveringof[a;b]sobytheHeine-BorelTheorem,thereisa
nitesubcoveringfIx1;:::;Ixng.Letc=min(f(x1);:::;f(xn)).Eachy2[a;b]belongstosomeIxksof(y)2f(xk)�m2c�m.Thus2c�misalowerboundforfon[a;b]but2c�m>m.Contradiction.Hencethereexistsx02[a;b]suchthatm=f(x0).51a.Letx2[a;b].Theninff(y)f(x)supf(y)forany>0.Hencewehaveg(x)=jy�xj<jy�xj<supinff(y)f(x)infsupf(y)=h(x).Supposeg(x)=f(x).Thengiven">0,thereexists>0jy�xj<>0jy�xj<>0suchthatf(x)�"=g(x)�"0gandgiven">0,thereexists>0suchthatf(x)�"f(y)wheneverjx�yj<jx�yj<.Thusf(x)�"inff(y)sof(x)=supinff(y)=g(x).Byasimilarargument,jx�yj<>0jx�yj<f(x)=h(x)ifandonlyiffisuppersemicontinuousatx.Thusfiscontinuousatxifandonlyiffisbothuppersemicontinuousandlowersemicontinuousatkhdaw.comxifandonlyiff(x)=h(x)andg(x)=f(x)ifandonlyifg(x)=h(x).51b.Let2R.Supposeg(x)>.Thenthereexists>0suchthatf(y)>wheneverjx�yj<.Hencefx:g(x)>gisopenin[a;b]andgislowersemicontinuous.Supposeh(x)<.Thenthereexists>0suchthatf(y)<wheneverjx�yj<.Hencefx:h(x)<gisopenin[a;b]andhisuppersemicontinuous.51c.Let"bealowersemicontinuousfunctionsuchthat"(x)f(x)forallx2[a;b].Suppose"(x)>g(x)forsomex2[a;b].Thenthereexists>0suchthat"(x)"(y)+"(x)�g(x)wheneverjx�yj<.i.e.g(x)"(y)wheneverjx�yj<.Inparticular,g(x)"(x).Contradiction.Hence"(x)g(x)forallx2[a;b].2.7Borelsets52.LetfbealowersemicontinuousfunctiononR.Thenfx:f(x)>
gisopen.fx:f(x)Tg=fx:f(x)>
�1=ngsoitisaGset.fx:f(x)g=fx:f(x)>
gcisclosed.nfx:f(x)<
g=fx:f(x)gcsoitisanFset.fx:f(x)=g=fx:f(x)gfx:f(x)gis课后答案网theintersectionofaGsetwithaclosedsetsoitisaGset.53.Letfbeareal-valuedfunctionde
nedonR.LetSbethesetofpointsatwhichfiscontinuous.Iffiscontinuousatx,thenforeachn,thereexistsn;x>0suchthatjf(x)�f(y)j<1=nwheneverSTjx�yj<n;x.ConsiderGn=x2S(x�n;x=2;x+n;x=2)andG=nGn.Thenx2Gforeveryx2S.Conversely,supposex02Gforsomex02=S.Thereexists">0suchthatforevery>0,thereexistsywithjy�x0j<andwww.hackshp.cnjf(x0)�f(y)j".ChooseNsuchthat1S=N<"=2.Thereexistsywithjy�x0j<N;x=2andjf(y)�f(x0)j".Ontheotherhand,x02GN=x2S(x�N;x=2;x+N;x=2)sox02(x�N;x=2;x+N;x=2)forsomex2S.Thusjf(x)�f(x0)j<1=N<"=2.Also,jy�xjjy�x0j+jx0�xj<N;xsojf(y)�f(x)j<1=N<"=2.Thusjf(y)�f(x0)jjf(y)�f(x)j+jf(x)�f(x0)j<".Contradiction.HenceG=SandSisaGset.54.LethfnibeasequenceofcontinuousfunctionsonRandletCbethesetofpointswherethesequenceconverges.Ifx2C,thenforanym,thereexistsnsuchthatjfk(xT)�Sfn(x)j1=mforallkn.ConsiderTSFn;m=fx:jfk(x)�fn(x)j1=mforkng.NowCSmnFn;m.Conversely,ifx2mnFn;m,thengiven">0,chooseMsuchthat1=M<".Nowx2TnFn;MSsothereexistsNsuchthatjfk(x)�fN(x)j1=M<"forkN.Thushfn(x)iconvergessomnFn;mC.Bycontinuityofeachfk,eachFn;misclosed.HenceCisanFset.3LebesgueMeasure3.1Introduction1.LetAandBbetwosetsinMwithAB.ThenmAmA+mkhdaw.com(BnA)=mB.13若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
Sn2.LethEnibeasequenceofsetsinMS.LetFS1=E1andletSFn+1=En+1Snk=1PEk.ThenPFmFn=;form6=n,FnEnforeachnandEn=Fn.Thusm(En)=m(Fn)=mFnmEn.3.SupposethereisasetAinMwithmA<1.ThenmA=m(A[;)=mA+m;som;=0.4.Clearlynistranslationinvariantandde
nedforallsetsofrealnumbers.LethEkibeasequenceofdisjointsetsofrealnumbers.WemayassumeSSPEk6=;forallksincen;=0.IfsomeEkisanin
niteset,thensoisSEk.Thusn(Ek)=1=nEk.IfallEk"sare
nitesetsandSPfEk:k2Ngisa
niteset,thenEkisa
nitesetandsincetheEk"saredisjoint,n(ESk)=nEk.Ontheotherhand,ifallSEk"sare
nitesetsandfEk:k2NPgisanin
niteset,thenEkisacountablyin
nitesetson(Ek)=1andsincenEk1forallk,nEk=1.3.2Outermeasure5.LetAbethesetofrationalnumbersbetween0and1.AlsoletfIngbea
nitecollectionofopenSSPPintervalscoveringA.Then1=m([0;1])=mAm(I)=m(I)mI=l(I)=Pnnnnl(In).6.GivenanysetAandany">0,thereisacountablecollectionfIngofopenintervalscoveringkhdaw.comPSAsuchthatPl(InP)mA+".LetO=In.ThenOisanopensetsuchthatAOandmOmI=l(I)mA+".Nowforeachn,thereisanopensetOsuchthatAOandnnTnnmOmA+1=n.LetG=O.ThenGisaGsetsuchthatAGandmG=mA.nn7.LetEbeasetofrealnumbersandlety2R.IffIngisacountablecollectionofopenintervalssuchSSPPthatEI,thenE+y(I+y)som(E+y)l(I+y)=l(I).Thusm(E+y)mE.nnnnConversely,byasimilarargument,mEm(E+y).Hencem(E+y)=mE.8.SupposemA=0.Thenm(A[B)mA+mB=mB.Conversely,sinceBA[B,mBm(A[B).Hencem(A[B)=mB.3.3MeasurablesetsandLebesguemeasure9.LetEbeameasurableset,letAbeanysetandlety2R.ThenmA=m(A�y)=m((A�y)E)+m((A�y)Ec)=m(((A�y)E)+y)+m(((A�y)Ec)+y)=m(A(E+y))+m(A(Ec+y))=m(A(E+y))+m(A(E+y)c).ThusE+yisameasurableset.10.SupposeE1andE2aremeasurable.Then课后答案网m(E1[E2)+m(E1E2)=mE1+m(E2nE1)+m(E1E2)=mE1+mE2.T11.Foreachn,letEn=(n;1).ThenEn+1Enforeachn,En=;andmEn=1foreachn.S112.SLet1hEiibeasequenceofdisjointmeasurablesetsandletP1Abeanyset.Thenm(AS1i=1Ei)=m((AE))m(AE)bycountablesubadditivity.Conversely,m(AE)i=1SniPni=1iS1P1i=1im(AE)=m(AE)forallnbyLemma9.Thusm(AE)m(AE).i=1Sii=1Pii=1ii=1iHencem(A1E)=1m(AE).i=1iwww.hackshp.cni=1i13a.SupposemE<1.(i))(ii).SupposeEismeasurable.Given">0,thereisacountablecollectionfIngofopenintervalsSPSsuchthatEIandl(I)0,thereisanopensetOsuchthatEOandm(OnE)<"=2.OistheunionPSofacountablecollectionofdisjointopenintervalsfIngsol(In)=m(In)0,thereisa
niteunionUofopenintervalssuchthatm(UE)<"=3.AlsothereisanopensetOsuchthatEnUOandmOm(EnU)+"=3.ThenEU[Oandm((U[O)nE)=m((UnE)[(OnE))m((On(EnU))[(EnU)[(UnE))<".13b.(i))(ii).SupposeEismeasurable.ThecasewheremE<1wasproveninpart(a).SupposemE=1.Foreachn,letE=[�n;n]E.Bypart(a),foreachn,thereexistsanopensetOEnSSSnnsuchthatm(OnE)<"=2n.LetO=O.ThenEOandm(OnE)=m(OnE)Snnnnnm((OnE))<".nn14khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
(ii))(iv).Foreachn,thereexistsanopensetOsuchthatEOandm(OnE)<1=n.LetTnnnG=O.ThenEGandm(GnE)m(OnE)<1=nforalln.Thusm(GnE)=0.nn(iv))(i).ThereexistsaGsetGsuchthatEGandm(GnE)=0.NowGandGnEaremeasurablesetssoE=Gn(GnE)isameasurableset.13c.(i))(iii).SupposeEismeasurable.ThenEcismeasurable.Bypart(b),given">0,thereisanopensetOsuchthatEcOandm(OnEc)<".i.e.m(OE)<".LetF=Oc.ThenFisclosed,FEandm(EnF)=m(EnOc)=m(EO)<".S(iii))(v).Foreachn,thereisaclosedsetFsuchthatFEandm(EnF)<1=n.LetF=F.nnnnThenFEandm(EnF)m(EnF)<1=nforalln.Thusm(EnF)=0.n(v))(i).ThereexistsanFsetFsuchthatFEandm(EnF)=0.NowFandEnFaremeasurablesetssoE=F[(EnF)isameasurableset.14a.Foreachn,letEbetheunionoftheintervalsremovedinthenthstep.ThenmE=2n�1=3nsoSPnSnm(En)=mEn=1.ThusmC=m([0;1])�m(En)=0.14b.EachstepofremovingopenintervalsresultsinaclosedsetandFistheintersectionofalltheseclosedsetssoFisclosed.Letx2[0;1]nFc.Notethatremovingintervalsinthenthstepresultsin2ndisjointintervals,eachoflengthlessthan1khdaw.com=2n.Given>0,chooseNsuchthat1=2N<2.Then(x�;x+)mustintersectoneoftheintervalsremovedinstepN.i.e.thereexistsy2(x�;x+)Fc.PThusFcisdensein[0;1].Finally,mF=m([0;1])�2n�1=3n=1�.3.4Anonmeasurableset15.LetEbeameasurablesetwithEP.Foreachi,letEiP=E+ri.SinceSEiPiforeachi,hEiiisadisjointsequenceofmeasurablesetswithPPmEi=mE.ThusmEi=m(Ei)m([0;1))=1.SincemEi=mE,wemusthavemE=0.16.LetAbeanysetwithmA>0.IfA[0;1),lethri1beanenumerationofQ[�1;1).ForSii=0eachi,letPi=P+ri.Then[0;1)Pisinceforanyx2[0;1),thereexistsy2Psuchthatxandydi
erbysomerationalri.Also,PiPj=;ifi6=jsinceifpi+ri=pj+rj,thenpipjandsincePcontainsexactlyoneelementfromeachequivalenceclass,pi=pjandri=rj.NowletEi=APiforeachi.IfeachEPiismeasurable,thenSmEi=m(APi)=m((A�ri)P)=0foreachibyQ15.Ontheotherhand,mEm(E)m(A[0;1))=mA>0.HenceEisnonmeasurableforiii0somei0andEi0AS.Similarly,if课后答案网A[n;n+1)wherePn2Z,thenthereisanonmeasurablesetEA.Ingeneral,A=A[n;n+1)and00n2Zn2Zforsomen2ZandthereisanonmeasurablesetEA[n;n+1)A.17a.Lethri1beanenumerationofQ[�1;1)andletP=P+rforeachi.ThenhPiisadisjointii=0SPiPiiSsequenceofsetswithm(P)m[�1;2)=3andmP=mP=1.Thusm(P)0=m(E).ii3.5Measurablefunctions18.LetEbethenonmeasurablesetde
nedinSection3.4.Letfbede
nedon[0;1]withf(x)=x+1ifx2Eandf(x)=�xifx=2E.Thenftakeseachvalueatmostoncesofx:f(x)=ghasatmostoneelementforeach2Randeachofthesesetsismeasurable.However,fx:f(x)>0g=E,whichisnonmeasurable.19.LetDbeadensesetofrealnumbersandletfbeanextendedreal-valuedfunctiononRsuchthatfx:f(x)>
gismeasurableforeach2D.Let2R.Foreachn,thereexistsn2DsuchthatSS
<
n<
+1=n.Nowfx:f(x)>
g=fx:f(x)+1=ng=fx:f(x)>
ngsofx:f(x)>
gismeasurableandfismeasurable.Pn1Pn2Pn1Pn220.Let"1=i=1i Aiandlet"2=i=1i Bi.Then"1+"2=i=1i Ai+i=1i Biisa15khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
Psimplefunction.Also"1"2=i;jij Ai Bjisasimplefunction. AB(x)=1ifandonlyifx2Aandx2Bifandonlyif A(x)=1= B(x).Thus AB= A B.If A[B(x)=1,thenx2A[B.Ifx2AB,then A(x)+ B(x)+ A B(x)=1+1�1=1.Ifx=2AB,thenx2AnBorx2BnAso A(x)+ B(x)=1and A B(x)=0.If A[B(x)=0,thenx=2A[Bso A(x)= B(x)= A B(x)=0.Hence A[B= A+ B+ A B.If Ac(x)=1,thenx=2Aso A(x)=0.If Ac(x)=0,thenx2Aso A(x)=1.Hence Ac=1� A.21a.LetDandEbemeasurablesetsandfafunctionwithdomainD[E.Supposefismeasurable.SinceDandEaremeasurablesubsetsofD[E,fjDandfjEaremeasurable.Conversely,supposefjDandfjEaremeasurable.Thenforany2R,fx:f(x)>
g=fx2D:fjD(x)>
g[fx2E:fjE(x)>
g.Eachsetontherightismeasurablesofx:f(x)>
gismeasurableandfismeasurable.21b.LetfbeafunctionwithmeasurabledomainD.Letgbede
nedbyg(x)=f(x)ifx2Dandg(x)=0ifx=2D.Supposefismeasurable.If0,thenfx:g(x)>
g=fx:f(x)>
g,whichismeasurable.If
<0,thenfx:g(x)>
g=fx:f(x)>
g[Dc,whichismeasurable.Hencegismeasurable.Conversely,supposegismeasurable.Thenf=gjDandsinceDismeasurable,fismeasurable.22a.khdaw.comLetfbeanextendedreal-valuedfunctionwithmeasurabledomainDandletD1=fx:f(x)=1g;D2=fx:f(x)=�1g.Supposefismeasurable.ThenD1andD2aremeasurablebyProposition18.NowDn(D1[D2)isameasurablesubsetofDsotherestrictionofftoDn(D1[D2)ismeasurable.Conversely,supposeD1andD2aremeasurableandtherestrictionofftoDn(D1[D2)ismeasurable.For2R,fx:f(x)>
g=D1[fx:fjDn(D1[D2)(x)>
g,whichismeasurable.Hencefismeasurable.22b.Letfandgbemeasurableextendedreal-valuedfunctionsde
nedonD.D1=ffg=1g=ff=1;g>0g[ff=�1;g<0g[ff>0;g=1g[ff<0;g=�1g,whichismeasurable.D2=ffg=�1g=ff=1;g<0g[ff=�1;g>0g[ff>0;g=�1g[ff<0;g=1g,whichismeasurable.Leth=fgjDn(D1[D2)andlet2R.If0,thenfx:h(x)>
g=fx:fjDnfx:f(x)=1g(x)gjDnfx:g(x)=1g(x)>
g,whichismeasurable.If
<0,thenfx:h(x)>
g=fx:f(x)=0g[fx:g(x)=0g[fx:fjDnff=1g(x)gjDnfg=1g(x)>
g,whichismeasurable.Hencefgismeasurable.22c.Letfandgbemeasurableextendedreal-valuedfunctionsde
nedonDanda
xednumber.De
nef+gtobewheneveritisoftheform1�1or�1+1.D1=ff+g=1g=ff2R;g=1g[ff=g=1g[ff=1;g2Rg,whichismeasurable.D2=ff+g=�1g=ff2R;g=�1g[ff=g=�1g[ff=�1;g2课后答案网Rg,whichismeasurable.Leth=(f+g)jDn(D1[D2)andlet2R.If,thenfx:h(x)>
g=fx:fjDnff=1g(x)+gjDnfg=1g(x)>
g,whichismeasurable.If
<
,thenfx:h(x)>
g=ff=1;g=�1g[ff=�1;g=1g[fx:fjDnff=1g(x)+gjDnfg=1g(x)>
g,whichismeasurable.Hencef+gismeasurable.22d.Letfandgbemeasurableextendedreal-valuedfunctionsthatare
nitea.e.ThenthesetsD1;D2;fx:h(x)>
gcanbewrittenasunionsofsetsasinpart(c),possiblywithanadditionalsetofmeasurezero.Thusthesesetsaremeasurableandwww.hackshp.cnf+gismeasurable.23a.Letfbeameasurablefunctionon[a;b]thattakesthevalues1onlyonasetofmeasurezeroandlet">0.Foreachn,letEn=fx2[a;b]:jf(x)j>nTg.EachEnismeasurableandEn+1Enforeachn.Also,mE1b�a<1.ThuslimmEn=m(En)=0.ThusthereexistsMsuchthatmEM<"=3.i.e.jfjMexceptonasetofmeasurelessthan"=3.23b.Letfbeameasurablefunctionon[a;b].Let">0andMbegiven.ChooseNsuchthatM=N<".Foreachk2f�N;�N+1;:::;N�1g,letEk=fx2[a;b]:kM=Nf(x)<(k+1)M=Ng.EachEkPN�1ismeasurable.De
ne"by"=k=�N(kM=N) Ek.Then"isasimplefunction.Ifx2[a;b]suchthatjf(x)j0begiven.Let"=i=1ai Ai.Foreachi,thereNiisa
niteunionPUi=k=1Ii;kof(disjoint)openintervalssuchthatm(UiAi)<"=3n.De
negbynSg=i=1ai Uni�1U.Thengisastepfunctionon[a;b].Ifg(x)6="(x),theneitherg(x)=ai6="(x),im=1morg(x)=0and"(x)=aiS.Inthe
rstcase,x2UinSAi.Inthesecondcase,x2AinUi.Thusnnfx2[a;b]:"(x)6=g(x)gi=1((UinAi)[(AinUi))=i=1(UiAi)soithasmeasurelessthan"=3.Ifm"M,wemaytakegsothatmgMsincebothgandkhdaw.com"takevaluesinfa1;:::;ang.16若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
23d.Letgbeastepfunctionon[a;b]andlet">0begiven.Letx0;:::;xnbethepartitionpointscorrespondingtog.Letd=minfxi�xi�1:i=1;:::;ng.Foreachi,letIibeanopenintervaloflengthlessthanmin("=3(n+1);d=2)centredatSxi.De
nehbylinearisinggineachIi.Thenhiscontinuousnandfx2[a;b]:g(x)6=h(x)gi=0Ii,whichhasmeasurelessthan"=3.IfmgM,wemaytakehsothatmhMbyconstruction.24.LetfbemeasurableandBaBorelset.LetCbethecollectionofsetsEsuchthatf�1[E]ismeasurable.SupposeE2C.Thenf�1[Ec]=(f�1[E])c,whichismeasurable,soEc2C.SupposehEiSSSiisasequenceofsetsinC.Thenf�1[E]=f�1[E],whichismeasurable,soE2C.ThusCisiiia-algebra.Nowforanya;b2Rwithaagandfx:f(x)
g=(gf)�1[(
;1)]=f�1[g�1[(
;1)]],whichismeasurablebyQ24.Hencegfismeasurable.26.Propositions18and19andTheorem20followfromargumentssimilartothoseintheoriginalproofsandthefactthatthecollectionofBorelsetsisakhdaw.com-algebra.IffisaBorelmeasurablefunction,thenforany2R,thesetfx:f(x)>
gisaBorelsetsoitisLebesguemeasurable.ThusfisLebesguemeasurable.IffisBorelmeasurableandBisaBorelset,thenconsiderthecollectionCofsetsEsuchthatf�1[E]isaBorelset.ByasimilarargumenttothatinQ24,Cisa-algebracontainingalltheopenintervals.ThusCcontainsalltheBorelsets.Hencef�1[B]isaBorelset.IffandgareBorelmeasurable,thenfor2R,fx:(fg)(x)>
g=(fg)�1[(
;1)]=g�1[f�1[(
;1)]],whichisaBorelset.ThusfgisBorelmeasurable.IffisBorelmeasurableandgisLebesguemeasurable,thenforany2R,f�1[(
;1)]isaBorelsetandg�1[f�1[(
;1)]]isLebesguemeasurablebyQ24.ThusfgisLebesguemeasurable.27.CallafunctionA-measurableifforeach2Rthesetfx:f(x)>
gisinA.Propositions18and19andTheorem20stillhold.AnA-measurablefunctionneednotbeLebesguemeasurable.Forexample,letAbethe-algebrageneratedbythenonmeasurablesetPde
nedinSection4.Then PisA-measurablebutnotLebesguemeasurable.ThereexistsaLebesguemeasurablefunctiongandaLebesguemeasurablesetAsuchthatg�1[A]isnonmeasurable(seeQ28).IffandgareLebesguemeasurable,fgmaynotbeLebesguemeasurable.Forexample,takegandAtobeLebesguemeasurablewithg�1[A]nonmeasurable.Letf= sothatfisLebesguemeasurable.Thenfx:(fg)(x)>1=2g=g�1[A],课后答案网Awhichisnonmeasurable.Thisisalsoacounterexampleforthelaststatement.28a.Letfbede
nedbyf(x)=f1(x)+xforx2[0;1].ByQ2.48,f1iscontinuousandmonotoneon[0;1]sofiscontinuousandstrictlymonotoneon[0;1]andfmaps[0;1]onto[0;2].ByQ2.46,fhasacontinuousinversesoitisahomeomorphismof[0;1]onto[0;2].28b.ByQ2.48,f1isconstantoneachintervalcontainedinthecomplementoftheCantorset.Thusfmapseachoftheseintervalsontoanintervalofthesamelength.Thuswww.hackshp.cnm(f[[0;1]nC])=m([0;1]nC)=1andsincefisabijectionof[0;1]onto[0;2],mF=m(f[C])=m([0;2])�1=1.28c.Letg=f�1:[0;2]![0;1].Thengismeasurable.SincemF=1>0,thereisanonmeasurablesetEF.LetA=f�1[E].ThenACsoithasoutermeasurezeroandismeasurablebutg�1[A]=Esoitisnonmeasurable.28d.Thefunctiong=f�1iscontinuousandthefunctionh= ismeasurable,whereAisasde
nedAinpart(c).Howeverthesetfx:(hg)(x)>1=2g=g�1[A]isnonmeasurable.Thushgisnotmeasurable.28e.ThesetAinpart(c)ismeasurablebutbyQ24,itisnotaBorelsetsinceg�1[A]isnonmeasurable.3.6Littlewood"sthreeprinciples29.LetE=Randletfn= [n;1)foreachn.Thenfn(x)!0foreachx2E.ForanymeasurablesetAEwithmA<1andanyintegerN,pickxNsuchthatx=2A.ThenjfN(x)�0j1.30.Egoro
"sTheorem:Lethfnibeasequenceofmeasurablefunctionsthatconvergestoareal-valuedfunctionfa.e.onameasurablesetEof
nitemeasure.Let>0begiven.Foreachn,thereexistsAEwithmA<=2nandthereexistsNsuchthatforallx=2AandkN,jf(x)�f(x)j<1=n.nnnkhdaw.comnnk17若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
SPLetA=A.ThenAEandmA<=2n=.Choosensuchthat1=n<.Ifx=2Aandn00kNn0,wehavejfk(x)�f(x)j<1=n0<.ThusfnconvergestofuniformlyonEnA.31.Lusin"sTheorem:Letfbeameasurablereal-valuedfunctionon[a;b]andlet>0begiven.Foreachn,thereisacontinuousfunctionhon[a;b]suchthatmfx:jh(x)�f(x)j=2n+2g<=2n+2.nnSLetE=fx:jh(x)�f(x)j=2n+2g.Thenjh(x)�f(x)j<=2n+2forx2[a;b]nE.LetE=E.nnnnnThenmE<=4andhhniisasequenceofcontinuous,thusmeasurable,functionsthatconvergestofon[a;b]nE.ByEgoro
"sTheorem,thereisasubsetA[a;b]nEsuchthatmA<=4andhnconvergesuniformlytofon[a;b]n(E[A).Thusfiscontinuouson[a;b]n(E[A)withm(E[A)<=2.NowthereisanopensetOsuchthatO(E[A)andm(On(E[A))<=2.Thenfiscontinuouson[a;b]nO,whichisclosed,andmO<.ByQ2.40,thereisafunction"thatiscontinuouson(�1;1)suchthatf="on[a;b]nO.Inparticular,"iscontinuouson[a;b]andmfx2[a;b]:f(x)6="(x)g=mO<.Iffisde
nedon(�1;1),let0=min(=2n+3;1=2).Thenforeachn,thereisacontinuousfunction"on[n+0;n+1�0]suchthatmfx2[n+0;n+1�0]:f(x)6="(x)g<=2n+2.Similarlyfornn[�n�1+0;�n�0].Lineariseineachinterval[n�0;n+0].Similarlyforintervals[�n�0;�n+0].PThenwehaveacontinuousfunction"de
nedon(�1;1)withmfx:f(x)6="(x)g<4=2n+2=.*32.Fort2[0;1)with1=2i+1t<1=2i,de
nef:[0;1)!Rbyf(x)=1ifx2P=P+rttiiandxkhdaw.com=2i+1t�1,andf(x)=0otherwise.Foreacht,thereisatmostonexsuchthatf(x)=1ttsoeachfismeasurable.Foreachx,x2Pforsomei(x).Lett(x)=(x+1)=2i(x)+1.Thenti(x)1=2i(x)+1t(x)<1=2i(x)andf(x)=1.Thisistheonlytsuchthatf(x)=1.Thusforeachx,t(x)tft(x)!0ast!0.Notethatanymeasurablesubsetof[0;1)withpositivemeasureintersectsin
nitelymanyofthesetsPi.ThusforanymeasurablesetA[0;1)withmA<1=2,m([0;1)nA)1=2sothereexistsx2[0;1)nAwithi(x)arbitrarilylargeandsowitht(x)arbitrarilysmall.i.e.thereexistanx2[0;1)nAandarbitrarilysmalltsuchthatft(x)1=2.(*)AnymeasurablesetA[0;1)withpositivemeasureintersectsin
nitelymanyofthesetsPi:SnSupposeAintersectsonly
nitelymanyofthesetsPi.i.e.Ai=1Pqi,wherePqi=P+qi.Chooser12Q[�1;1]suchthatr16=qi�qjforalli;j.Supposer1;:::;rnhavebeenchosen.Choosern+1suchthatrn+16=qi�qj+rkforalli;jandkn.NowthemeasurablesetsSA+riParedisjointbythede
nitionnnofPandtheconstructionofthesequenceShrii.Thenm(i=1(A+ri))=i=1m(A+ri)=nmAforneachn.Sincei=1(A+ri)[�1;2],nmA3forallnandmA=0.4TheLebesgueIntegral课后答案网4.1TheRiemannintegral1a.Letfbede
nedbyf(x)=0ifxisirrationalandf(x)=1ifxisrational.Foranysubdivisiona=0<10jy�xj<Sincefisbounded,hisuppersemicontinuousbyQ2.51bandhisbounded.Forany2R,thesetfx:h(x)<
gisopen.Thushismeasurable.Let"beastepfunctionon[a;b]suchthat"f.RbRbRbThen"hexceptata
nitenumberofpoints(thepartitionpoints).ThusRaf=inf"fa"ah.Conversely,thereexistsamonotonedecreasingsequenceh"niofstepfunctionssuchthath(x)=lim"n(x)RbRbRbRbRbforeachx2[a;b].Thush=lim"nRf.HenceRf=h.aaaaa18khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
2b.Letfbeaboundedfunctionon[a;b]andletEbethesetofdiscontinuitiesoff.LetgbetheRbRblowerenvelopeoff.Byasimilarargumentasinpart(a),Rf=g.SupposeEhasmeasurezero.aaRbRbRbRbTheng=ha.e.soRf=g=h=RfsofisRiemannintegrable.Conversely,supposefisaRaRaRaRbbbbRiemannintegrable.Theng=h.Thusjg�hj=0sog=ha.e.sincejg�hj(1=n)mfx:aaaajg(x)�h(x)j>1=ngforalln.Hencefiscontinuousa.e.andmE=0.4.3Theintegralofanonnegativefunction3.Letfbeanonnegativemeasurablefunctionandsupposeinff=0.LetE=fx:f(x)>0g.ThenSRE=EnwhereEn=fx:f(x)1=ng.Nowf(1=n)mEnforeachnsomEn=0foreachnandmE=0.Thusf=0a.e.4a.Letfbeanonnegativemeasurablefunction.Forn=1;2;:::,letE=f�1[(i�1)2�n;i2�n)wheren;iPn2ni=1;:::;n2nandletE=f�1[n;1).De
ne"=(i�1)2�n +n .n;0ni=1(En;i[�n;n])(En;0[�n;n])Theneach"nisanonnegativesimplefunctionvanishingoutsideasetof
nitemeasureand"n"n+1foreachn.Furthermore,forsucientlylargen,f(x)�"(x)2�nifx2[�n;n]andf(x)0,thereexistsNsuchthatF(x)�F(x�1=n)<"andF(x+1=n)�F(x)<"whenevernN.Choose<1=N.ThenjF(y)�F(x)j<"wheneverjx�yj<.HenceFiscontinuous.课后答案网6.LethfnibeasequenceofnonnegativemeasurablefunctionsconvergingtoRRRRfandsupposefnfforeachn.ByFatou"sLemma,RRflimfn.Ontheotherhand,flimfnsinceffnforeachn.Hencef=limfn.7a.Foreachn,letRfn= [n;n+1).ThenRhfniisasequenceofnonnegativemeasurablefunctionswithlimfn=0.Now0=0<1=limwww.hackshp.cnfnandwehavestrictinequalityinFatou"sLemma.7b.Foreachn,letfnR= [n;1).ThenhfnRiisadecreasingsequenceofnonnegativemeasurablefunctionswithlimfn=0.Now0=0<1=limfnsotheMonotoneConvergenceTheoremdoesnothold.8.Lethfnibeasequenceofnonnegativemeasurablefunctions.Foreachn,lethRn=infknRfk.TheneachRhnisanonnegativemeasurablefunctionwithRhnfn.ByFatou"sLemma,limfn=limhnlimhnlimfn.9.LetRhfniRbeasequenceofnonnegativemeasurablefunctionssuchthatfn!fa.e.andsupposethatfn!f<1.ThenforanymeasurablesetE,hRfn EiisasequenceofnonnegativemeasurableRfunctionswithfn E!f Ea.e.ByFatou"sLemma,EflimEfn.Nowf Eisintegrablesincef Ef.Also,fRnisintegrableforsucientlylargeRnRsofRn EisintegrableforsucientlylargeRRRRn.ByFatou"sLemma,RR(f�f E)limR(fn�fn RE).i.e.f�Eflimfn�limEfn=f�limEfn.ThuslimEfnEfandwehaveEfn!Ef.4.4ThegeneralLebesgueintegral10a.IffisintegrableoverE,thensoaref+andf�.Thusjfj=f++f�isintegrableoverEandRRRRRRRRjfj=jf+�f�jjf+j+jf�j=f++f�=jfj.Conversely,ifjfjisintegrableEEEEEEEE19khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
RRRRoverE,thenf+jfj<1andf�jfj<1sof+andf�areintegrableoverEandfisEEEEintegrableoverE.R110b.f(x)=sinx=xisnotLebesgueintegrableon[0;1]althoughRf(x)==2(bycontour0Rbintegrationforexample).Ingeneral,supposefisLebesgueintegrableandtheRiemannintegralRfaexistswithimproperlowerlimita.Ifais
nite,letfn=f [a+1=n;b].Thenfn!fon[a;b]andjfnjjfjRbRbRRbsoRaf=limRa+1=nf=limfn=af.Ifa=�1,letgn=f [�n;b].Thengn!fon[a;b]RbRbRRbandjgnjjfjsoRaf=limR�nf=limgn=af.ThecaseswheretheRiemannintegralhasimproperupperlimitaresimilar.Pn11.Let"=i=1ai Aibeasimplefunctionwithcanonicalrepresentation.LetPPS+=fi:ai0gandletS�=fi:a<0g.Then"+=a and"�=�a .Clearly"+and"�iRPi2S+iAiRPi2S�iAiRRRaresimplefunctions.Then"+=amAand"�=�amAso"="+�"�=Pi2S+iii2S�iini=1aimAi.12.LetgbeanintegrablefunctiononasetEandsupposethathfniisasequenceofmeasurablefunctionswithRjfnjRga.e.onRE.Thenhfn+RgiisasequenceofnonnegativemeasurablefunctionsonRRRRE.Thuskhdaw.comlimfn+glim(fn+g)lim(fn+g)limfn+RgRsolimfnRlimfn.Also,hg�RfniisasequenceofnonnegativemeasurablefunctionsonRRRRE.ThusgR+limR(�fn)lim(g�fn)Rlim(g�fn)Rg+limR(�fnR)solim(�fn)lim(�fn).i.e.limfnlimfn.Hencewehavelimfnlimfnlimfnlimfn.13.Lethbeanintegrablefunctionandhfniasequenceofmeasurablefunctionswithfn�RhandRlimfn=f.ForeachRn,fnR+hisanonnegativemeasurablefunction.SinceRRRRRhisintegrable,RRfn=R(fn+h)R�h.Similarly,f=(f+h)�h.Nowf+hlim(fn+h)limfn+hsoflimfn.14a.Lethgnibeasequenceofintegrablefunctionswhichconvergesa.e.toanintegrablefunctiongandlethfniRbeasequenceofmeasurablefunctionssuchthatRjfnjgnandhfniconvergestofa.e.Supposeg=limgn.Sincejfnjgn,jfjg.Thusjfn�fjjfnj+jfjgRn+gandhgn+g�jfn�fjiisasequenceofnonnegativemeasurablefunctions.ByFatou"sLemma,RRRRRRlim(gn+g�jfn�fRj)lim(gn+g�jRfn�fj).i.e.2gR2g+lim(�jfn�fj)=2g�limjfn�fj.Hencelimjfn�fj0limjfn�fjandwehavejfn�fj!0.14b.RLethfnibeasequenceofintegrablefunctionssuchthatRRRRfn!fa.e.withRRfintegrable.Ifjfn�fRj!0,thenRjfn课后答案网j�jfjjjfnj�jfjjjfn�fj!0.Thusjfnj!jfRj.Conversely,supposejfnj!jfj.Bypart(a),withjfnjinplaceofgnandjfjinplaceofg,wehavejfn�fj!0.15a.LetfbeintegrableoverEandlet">0begiven.ByQ4,thereisasimplefunctionf+suchRRRRthatf+�"=2<.Also,thereisasimplefunction0f�suchthatf��"=2<0.LetEERRRERE"=�0.Then"isasimplefunctionandjf�"j=jf+��f�+0jjf+�j+jf��0j=RREEEE(f+�)+(f��0)<".EER15b.Letfn=f [�n;n].Thenwww.hackshp.cnRfn!fandjfnjjfj.ByLebesgue"sDominatedConvergenceTheorem,REjf�f [�n;n]j!0.i.e.E[�n;n]cjfj!0.ThusthereexistsRNsuchthatE[�N;N]cjfj<"=3.Bypart(a),thereisasimplefunction"suchthatjf�"j<"=3.ByProposition3.22,thereEisastepfunctionon[�N;N]suchthatj"�j<"=12NMexceptonasetofmeasurelessthan"=12NM,whereMmax(j"j;jj)+1.WemayregardasafunctiononRtakingthevalue0RNRRRoutside[�N;N].Then�Nj"�j<"=3soEjf�j=E[�N;N]jf�j+E[�N;N]cjf�jRRRRRNRE[�N;N]jf�"j+E[�N;N]j"�j+E[�N;N]cjf�jEjf�"j+�Nj"�j+E[�N;N]cjfj<".R15c.Bypart(b),thereisastepfunctionsuchthatjf�j<"=2.Supposeisde
nedon[a;b].EWemayregardasafunctiononRtakingthevalue0outside[a;b].Bylinearisingateachpartitionpoint,wegetacontinuousfunctiongvanishingoutsidea
niteintervalsuchthat=gexceptonasetRRRofmeasurelessthan"=4M,whereMjj.Thenjf�gjjf�j+j�gj<".EEE16.Riemann-LebesgueTheorem:Supposefisintegrableon(�1;1).ByQ15,given">0,RRRthereisastepfunctionsuchthatjf�j<"=2.Nowjf(x)cosnxdxjjf(x)cosnxjdxRRRj(f(x)�(x))cosnxjdx+j(x)cosnxjdx<"=2+j(x)cosnxjdx.Integratingj(x)cosnxjoverReachintervalonwhichisconstant,weseethatj(x)cosnxjdx!0asn!1.ThusthereRRexistsNsuchthatj(x)cosnxjdx<"=2fornNsojf(x)cosnxdxj<"fornN.i.e.20khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
Rlimn!1f(x)cosnxdx=0.17a.Letfbeintegrableover(�1;1).Thenf+andf�arenonnegativeintegrablefunctions.Thereexistsanincreasingsequenceh"iofnonnegativesimplefunctionssuchthatf+=lim".NowsinceRnRRnR E(x)dx=mE=m(E�t)= E(x+t)dxforanymeasurablesetRE,wehaveR"n(x)dx="(x+t)dxforalln.BytheMonotoneConvergenceTheorem,f+(x)dx=f+(x+t)dx.SimilarlynRRforf�.Thusf(x)dx=f(x+t)dx.17b.LetgbeaboundedmeasurablefunctionandletMbesuchthatjgjM.Sincefisintegrable,givenR">0,thereisacontinuousfunctionhvanishingoutsidea
niteinterval[a;b]suchthatjf�hj<"=4M.RRRNowjg(x)[f(x)�f(x+t)]jjg(x)[h(x)�h(x+t)]j+jg(x)[(f�h)(x)�(f�h)(x+t)]j.Nowhisuniformlycontinuouson[a;b]sothereexists>0suchthatjh(x)�h(x+t)j<"=2M(b�a)wheneverjtj0,thereexistsNsuchthatmfx:jfn(x)�f(x)j"g<"fornN.Foranysubsequencehfnki,chooseMsuchthatnkNforkM.Thenmfx:jfnk(x)�f(x)j"g<"forkM.Thushfnkiconvergestofinmeasure.21.Fatou"sLemma:LethfnibeasequenceofnonnegativemeasurablefunctionsthatconvergesinRRmeasuretofonE.ThenthereisasubsequencehfnkisuchthatlimEfnk=limEfn.ByQ20,hfnkiconvergesinmeasuretoRRRfwww.hackshp.cnonEsoitinturnhasasubsequenceRhfnkjithatconvergestofa.e.ThusEflimEfnkj=limEfnk=limEfn.MonotoneConvergenceTheorem:Lethfnibeanincreasingsequenceofnonnegativemeasurablefunctionsthatconvergesinmeasuretof.AnysubsequencehfRnkialsoconvergesinmeasuretoRRfsoitinturnhasRasubsequencehfnkjithatconvergestofa.e.Thusf=limfnkj.ByQ2.12,f=limfn.Lebesgue"sDominatedConvergenceTheorem:LetgbeintegrableoverEandlethfnibeasequenceofmeasurablefunctionssuchthatjfnjgonEandconvergesinmeasuretofonE.Anysubsequencehfnkialsoconvergesinmeasuretofsoitinturnhasasubsequencehfnkjithatconvergestofa.e.ThusRRRRf=limfnkj.ByQ2.12,f=limfn.22.LethfnibeasequenceofmeasurablefunctionsonasetEof
nitemeasure.Ifhfniconvergestofinmeasure,thensodoesanysubsequencehfnki.Thusanysubsequenceofhfnkialsoconvergestofinmeasure.Conversely,ifhfnidoesnotconvergeinmeasuretof,thenthereexists">0suchthatforanyNthereexistsnNwithmfx:jfn(x)�f(x)j"g".Thisgivesrisetoasubsequencehfnkisuchthatmfx:jfnk(x)�f(x)"g"forallk.Thissubsequencewillnothaveafurthersubsequencethatconvergesinmeasuretof.23.LethfnibeasequenceofmeasurablefunctionsonasetEof
nitemeasure.Ifkhdaw.comhfniconvergestof21若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
inmeasure,thensodoesanysubsequencehfnki.Thushfnkihasinturnasubsequencethatconvergestofa.e.Conversely,ifeverysubsequencehfnkihasinturnasubsequencehfnkjithatconvergestofa.e.,thenhfnkjiconvergestofinmeasuresobyQ22,hfniconvergestofinmeasure.24.Supposethatfn!finmeasureandthatthereisanintegrablefunctiongsuchthatjfnjgforalln.Let">0begiven.Nowjfn�fjisintegrableforeachnandjfn�fj [�k;k]convergestojfn�fj.RkRByLebesgue"sDominatedConvergenceTheorem,R�kjfn�fjconvergestojfn�fj.ThusthereexistsNsuchthatjfn�fj<"=3.ByProposition14,foreachn,given">0,thereexists>0suchjxjNRthatforanysetAwithmA<,jf�fj<"=3.Wemayassume<"=6N.TherealsoexistsN0Ansuchthatmfx:jf(x)�f(x)jg<forallnN0.LetA=fx:jf(x)�f(x)jg.ThenRRnRRnjfn�fj=RjxjNjfn�fj+A[�N;N]jfn�fj+Ac[�N;N]jfn�fj<"=3+"=3+2N<"forallnN0.i.e.jf�fj!0.n25.LethfnibeaCauchysequenceinmeasure.Thenwemaychoosenv+1>nvsuchthatSmfx:jf(x)�f(x)j1=2vg<1=2v.LetE=fx:jf(x)�f(x)j1=2vgandletF=E.nv+1TnvSvnv+1TnvTkvkvThenm(F)m(E)1=2k�1forallksom(F)=0.Ifx=2F,thenx=2Fforkkvkvkkkkksomeksojf(x)�f(x)j<1=2vforallvkandjf(x)�f(x)j<1=2v�1forwvk.khdaw.comnv+1PnvnwnvThustheseries(fnv+1�fnv)convergesa.e.toafunctiong.Letf=g+fn1.Thenfnv!finmeasuresincethepartialsumsoftheseriesareoftheformfnv�fn1.Given">0,chooseNsuchthatmfx:jfn(x)�fr(x)j"=2g<"=2foralln;rNandmfx:jfnv(x)�f(x)j"=2g<"=2forallvN.Nowfx:jfn(x)�f(x)j"gfx:jfn(x)�fnv(x)j"=2g[fx:jfnv(x)�f(x)j"=2gforalln;vN.Thusmfx:jfn(x)�f(x)j"g<"forallnN.i.e.fn!finmeasure.5Di
erentiationandIntegration5.1Di
erentiationofmonotonefunctions+f(0+h)�f(0)1.Letfbede
nedbyf(0)=0andf(x)=xsin(1=x)forx6=0.ThenDf(0)=limh!0+h=�limh!0+sin(1=h)=1.Similarly,Df(0)=1;D+f(0)=D�f(0)=�1.+[�f(x+h)]�[�f(x)]f(x+h)�f(x)2a.D[�f(x)]=limh!0+h=�limh!0+h=�D+f(x).+g(x+h)�g(x)f(�x�h)�f(�x)2b.Letg(x)=f(�x).ThenDg(x)=limh!0+h=limh!0+h=limh!0+�f(�x)�f(�x�h)=�lim课后答案网f(�x)�f(�x�h)=�Df(�x).hh!0+h�3a.Supposefiscontinuouson[a;b]andassumesalocalmaximumatc2(a;b).Nowthereexistsf(c+h)�f(c)>0suchthatf(c+h)0suchthatf(c)>f(c�h)for00for00forallx2(a;b).Supposethereexistx;y2[a;b]withxg(y).SinceD+g(x)>0forallx2(a;b),ghasnolocalmaximumin(a;b)byQ3.Thusgisdecreasingon(a;y]andD+g(c)0forallc2(a;y).Contradiction.Hencegisnondecreasingon[a;b].Nowforany">0,D+(f(x)+"x)"on(a;b)sof(x)+"xisnondecreasingon[a;b].Letxf(y).Then00.NotethatifD+(fg)()=1,thenD+f(c)=1.NowsupposeD+f(c)<1.Let">0begivenandlet"=min(1;").Thereexists>0such1g0()+1+D+f(c)10thatjg(+h)�g()�g0()j<"for00suchthatf(c+h)�f(c)�D+f(c)<"h112h01for00suchthat213g(+h00)�g()<for00suchthatg(+h)�g()<0for00,thereexists>0suchthatA�"=20,thereexistsx2(x;x+)suchthatf(x)�f(x)>2�n�1nnnnsofisdiscontinuousateachxn.8a.Supposeacb.Leta=x0khdaw.com0.ThenthereexistsNsuchthat23若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
PnPnPnPnb1jf(xi)�f(xi�1)j1jf(xi)�fn(xi)j+1jf(xi�1)�fn(xi�1)j+1jfn(xi)�fn(xi�1)j<"+Ta(fn)fornN.ThusTb(f)"+Tb(f)fornNsoTb(f)"+limTb(f).Since"isarbitrary,aanaanTb(f)limTb(f).aan10a.Letfbede
nedbyf(0)=0andf(x)=x2sin(1=x2)forx6=0.Considerthesubdivisionppp�1<2=n<2=(n�1)<<2=<1of[�1;1].Notethattb(f)!1asn!1.Thusfisanotofboundedvariationon[�1;1].10b.Letgbede
nedbyg(0)=0andg(x)=x2sin(1=x)forx6=0.Notethatgisdi
erentiableon[�1;1]andjg0(x)j3on[�1;1].Thusforanysubdivisiona=x0,continuousat0butnotofboundedvariationon[0;1],thusnotabsolutelycontinuouson[0;1].Supposefisabsolutelycontinuouson[;1]for>0,continuousat0andofboundedvariationon[0;1].ForP2(0;1],let0=x00,thereexists2(0;1]such1ii�10thatT0(f)<"=2.Sincefisabsolutelycontinuouson[P;1],thereexists>0suchthatforany
nitePcollectionf(x;x0)gnofdisjointintervalsin[;1]withnjx0�xj<,wehavenjf(x0)�f(x)j<"=2.ii11iiP1iiNowletf(y;y0)gnbea
nitecollectionofdisjointintervalsin[0;1]withnjy0�yj<.If2[y0;y]ii1PPP1iikk+1forsomek,thennjf(y0)�f(y)jkjf(y0)�f(y)j+njf(y0)�f(y)j0,thereexists>0suchPn0Pn00nthat1jf(xi)�f(xi)jP<"=n2and1jg(xi)�g(Pxi)nj<"=2forany
nitecollectionPf(xni;xi)g1ofdisjointintervalsin[a;b]withjx0�xj<.Thenj(fg)(x0)�(fg)(x)jjf(x0)�f(x)j+P1ii1ii1iinjg(x0)�g(x)j<".Thusf+gandf�gareabsolutelycontinuous.1ii14b.Letfandgbetwoabsolutelycontinuousfunctionson[a;b].ThereexistsMsuchthatjf(x)jMPn0andjPg(x)jMforanyx2[a;b].Given">0,thereexists>0suchthat1jf(xi)�f(xi)j<"=2Mandnjg(x0)�g(x)j<"=2Mforany
nitecollectionf(x;x0)gofdisjointintervalsin[a;b]withPn1iiPnPniiPnjx0�xj<.Thenj(fg)(x0)�(fg)(x)jjf(x0)jjg(x0)�g(x)j+jg(x)jjf(x0)�f(x)j<".1ii1ii1iii1iiiThusfgisabsolutelycontinuous.14c.Supposefisabsolutelycontinuouson[a;b]andisneverzerothere.Letg=1=f.ThereexistsMPn02suchthatjf(x)jMforx2[a;b].Given">0,thereexists>0suchthat1jf(xi)�f(xi)j<"M24khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
0Pn00forany
nitecollectionf(xi;xi)gofdisjointintervalsin[a;b]with1jxi�xij<.Thenjg(xi)�g(xi)j=0jf(xi)�f(xi)j<".Thusgisabsolutelycontinuous.jf(xi)f(x0)ji15.LetfbetheCantorternaryfunction.ByQ2.48,fiscontinuousandmonotoneon[0;1].Notethatf0=0a.e.on[0;1]sincefisconstantoneachintervalinthecomplementoftheCantorsetandR1theCantorsethasmeasurezero.Iffisabsolutelycontinuous,then1=f(1)=f0+f(0)=0.0Contradiction.Thusfisnotabsolutelycontinuous.Rx16a.Letfbeamonotoneincreasingfunctionon[a;b].Letgbede
nedbyg(x)=f0andletRah=f�g.Thengisabsolutelycontinuous,h0(x)=f0(x)�dxf0=0a.e.sohissingular,anddxaf=g+h.16b.Letfbeanondecreasingsingularfunctionon[a;b].Let";>0begiven.Sincefissingularon[a;b],foreachx2[a;b],thereisanarbitrarilysmallinterval[x;x+h][a;b]suchthatjf(x+h)�f(x)j<"h=(b�a).Thenthereexistsa
nitecollectionf[xk;yk]gofnonoverlappingintervalsofthissortwhichcoverallof[a;b]exceptforasetofmeasurelessthanP.LabellingxksuchthatPxkxk+1,wehavenny0=ax1f(b)�f(a)�".16c.Letfbeanondecreasingfunctionon[a;b]withproperty(S).i.e.Given";>0,thereisa
nitePnPncollectionf[yk;xk]gofnonoverlappingintervalsin[a;b]suchthatR1jxk�ykj<and1(f(xk)�x0f(yk))>f(b)�f(a)�".Bypart(a),f=g+hwhereg=fandhissingular.ItsucestoshowPanthatg=0a.e.Lettingx0=aandyn+1=b,wehave1(f(yk+1)�f(xk))<".WemaychoosesuchRS0Rb0Rb0thatnf<".Thenf<2"sof=0andg=0.1[yk;xk]aa16d.Lethfnibeasequenceofnondecreasingsingularfunctionson[a;b]suchthatthefunctionf(x)=PPfn(x)iseverywhere
nite.Let";>0begiven.Nowf(b)�f(a)=(fn(b)�fn(a))<1soP1PNthereexistsNsuchthatN+1(fn(b)�fn(a))<"=2.LetF(x)=1fn(x).ThenFisnondecreasingandsingular.Bypart(b),thereexistsa
nitecollectionPPf[yk;xkP]gofnonoverlappingintervalssuchthatPjxk�ykj<and(F(yk)�F(xk))P>F(b)�F(a)�"=2.Now(f(yk)�f(xk))(F(yk)�F(xk))>1F(b)�F(a)�"=2=f(b)�f(a)�N+1(fn(b)�fn(a))�"=2>f(b)�f(a)�".Bypart(c),fissingular.*16e.LetCbetheCantorternaryfunctionon[0;1].ExtendCtoRbyde
ningC(x)=0forx<0andC(x)=1forx>1.Foreachn,de
nefbyf(x)=2�nC(x�an)wheref[a;b]gisanenumerationofnnbn�annntheintervalswithrationalendpointsin[0;1].Theneachfnisanondecreasingsingularfunctionon[0;1].P课后答案网De
nef(x)=fn(x).Thenfiseverywhere
nite,strictlyincreasingandbypart(d),fissingular.17a.LetFbeabsolutelycontinuouson[c;d].Letgbemonotoneandabsolutelycontinuouson[a;b]withcgd.Given">0,thereexists>0suchthatforany
nitecollectionf(y;y0)gofdisjointPPiiintervalswithnjy0�yj<,wehavenjF(y0)�F(y)j<".Nowthereexists0>0suchthatfor1ii1iPiPany
nitecollectionf(x;x0)gofdisjointintervalswithnjx0�xj<0,wehavenjg(x0)�g(x)j<.ii1iiP1iiNowf(g(x);g(x0))gisa
nitecollectionofdisjointintervalssonjF(g(x0))�F(g(x))j<".Henceiiwww.hackshp.cn1iiFgisabsolutelycontinuous.p(*)Additionalassumptionthatgismonotone.Counterexample:Considerf(x)=xforx2[0;1]andg(0)=0,g(x)=(xsinx�1)2forx2(0;1].Then(fg)(0)=0and(fg)(x)=xsinx�1forx2(0;1].fandgareabsolutelycontinuousbutnotfg.0Rx0Rx0*17b.LetE=fx:g(x)=0g.Notethatjg(x)�g(a)j=jgjjgjforallx2[a;b].Let">0.RaaThereexists>0suchthatjg0j<"=2whenevermA<.Let="=4(b�a).Foranyx2E,Athereexistshx>0suchthatjg(x+h)�g(x)j<hfor00.ThereisanopensetOEsuchthatmO=m(OnE)<whereisgivenbyabsolutecontinuity25khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
SPPofg.NowOSisacountableunionInofdisjointopenintervalssol(In)<andl(g[In[0;1]])<".Nowg[E]g[In[0;1]]som(g[E])<".Since"isarbitrary,m(g[E])=0.Rx19a.LetGbethecomplementofageneralisedCantorsetofpositivemeasureandletg= G.Then0gisabsolutelycontinuousandstrictlymonotoneon[0;1].Also,g0= =0onGc.G19b.Sincefx:g0(x)=0ghaspositivemeasure,ithasanonmeasurablesubsetF.ByQ17(b),m(g[F])=0.Also,g�1[g[F]]=Fisnonmeasurable.20a.SupposefisLipschitz.ThereexistsMsuchthatjf(x)�f(y)jMjx�yjforallx;y.Given0Pn0">0,letP="=M.Forany
nitecollectionPf(xi;xi)gofnonoverlappingintervalswith1jxi�xij<,wehavenjf(x0)�f(x)jMnjx0�xj<".Thusfisabsolutelycontinuous.1ii1ii20b.Letfbeabsolutelycontinuous.SupposefisLipschitz.Nowf0(x)=limf(y)�f(x)sojf0(x)j=y!xy�xf(y)�f(x)limy!xjy�xjMforallx.Conversely,iffisnotLipschitz,thenforanyM,thereexistxandysuchthatjf(x)�f(y)j>Mjx�yj.Thenjf0(c)j>Mforsomec2(x;y)bytheMeanValueTheorem.ThusforanyM,thereexistscsuchthatjf0(c)j>Msojf0jisunbounded.*20c.S21a.LetObeanopensetin[c;d].ThenOisacountableunionInofdisjointopenintervals.Nowkhdaw.comSSforeachn,I=(g(c);g(d))forsomec;d2[c;d].Also,g�1[O]=g�1[I]=(c;d).ThusnnnnnnnnPPPRdn0R0mO=l(In)=(g(dn)�g(cn))=cng=g�1[O]g.21b.LetH=fx:g0(x)6=0g.LetE[c;d]withmE=0andlet>0.ThenthereexistsanopenRRRsetOEwithmO<.Bypart(a),g0<.Thusg0=g0<.Since>0isRg�1[O]g�1[E]Hg�1[E]arbitrary,g0=0.Sinceg0>0ong�1[E]H,thesetg�1[E]Hhasmeasurezero.g�1[E]H21c.LetEbeameasurablesubsetof[c;d]andletF=g�1[E]H.Sincegisabsolutelycontinuous,itiscontinuousandthusmeasurablesog�1[E]ismeasurable.Also,g0ismeasurablesoHismeasurable.ThusF=g�1[E]Hismeasurable.ThereexistsaGsetGEwithm(GnE)=0.WemayassumeG[c;d].Bypart(b),m((g�1[G]nRRTg�1[E])H)=0sog0=g0.NowGisacountableintersectionOofopensets.g�1[G]Hg�1[E]HnTkTLetRGk=n=1On.ThenRG1G2solimRmGk=m(GRk)=mG.NowRmE=mG=limRmGk=limg0=limTg0=Tg0=g0=g0=g0.Also,g�1[G]Hkg�1[O]Hg�1[O]Hg�1[G]Hg�1[E]HFkn=1nnRRRbg0=g0= (g(x))g0(x)dx.Fg�1[E]aE课后答案网21d.Letfbeanonnegativemeasurablefunctionon[c;d].Thenthereisanincreasingsequenceh"iofsimplefunctionson[c;d]withlim"=fsolim"(g(x))g0(x)=f(g(x))g0(x).Sinceeachnnn00Rb0PRb0("ng)gismeasurable,(fg)gismeasurable.Nowa"n(g(x))g(x)dx=ack Ek(g(x))g(x)dx=PRdRRRdckmEk=c"n(y)dy.BytheMonotoneConvergenceTheorem,lim"n=f.Thuscf(y)dy=RdRb0Rb0limc"n(y)dy=lima"nwww.hackshp.cn(g(x))g(x)dx=af(g(x))g(x)dx.22a.Fisabsolutelycontinuouson[c;d],gismonotoneandabsolutelycontinuouson[a;b]withcgd.ByQ17(a),H=Fgisabsolutelycontinuous.WheneverH0andg0existwithg0(x)6=0,wehaveD+F(g(x))=DF(g(x))=D�F(g(x))=DF(g(x))=H0(x)=g0(x)byQ6asoF0(g(x))exists.Now+�H0andg0exista.e.soH0(x)=F0(g(x))g0(x)a.e.exceptonE=fx:g0(x)=0g.22b.Letf0bede
nedbyf0(y)=f(y)ify=2g[E]andf0(y)=0ify2g[E].ByQ17b,m(g[E])=0sof=fa.e.HenceH0(x)=f(g(x))g0(x)=f(g(x))g0(x)a.e.00*22c.*22d.5.5Convexfunctions23a.Let"beconvexona
niteinterval[a;b).Letx02(a;b)andletf(x)=m(x�x0)+"(x0)betheequationofasupportinglineatx0.Then"(x)f(x)forallx2(a;b).Since"iscontinuousata,wehave"(x)f(x)min(f(a);f(b))forallx2[a;b).Hence"isboundedfrombelow.23b.Suppose"isconvexon(a;b).If"ismonotoneon(a;b),then"(x)haslimits(possiblyin
nite)asitapproachesaandbrespectivelyfromwithin(a;b).If"isnotmonotone,thenthereexistsc2(a;b)26khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
suchthatD+"(x)0on(a;c]andD+"(x)0on[c;b)sincetheright-handderivativeof"isincreasingon(a;b).Thus"ismonotoneon(a;c]andon[c;b)anditfollowsthattheright-handandleft-handlimitsexistataandbrespectively.Ifa(orb)is
nite,thenbypart(a),thelimitsata(orb)cannotbe�1.23c.Let"becontinuousonanintervalI(open,closed,half-open)andconvexintheinteriorofI.Then"(tx+(1�t)y)t"(x)+(1�t)"(y)forallx;yintheinteriorofIandallt2[0;1].Since"iscontinuous,theinequalityalsoholdsattheincludedendpoints.24.Let"haveasecondderivativeateachpointof(a;b).If"00(x)0forallx2(a;b),then"0isincreasingon(a;b).Also,"iscontinuouson(a;b).Hence"isconvexon(a;b).Conversely,if"isconvexon(a;b),thenitsleft-andright-handderivativesaremonotoneincreasingon(a;b)so"0ismonotoneincreasingon(a;b).Hence"00(x)0forallx2(a;b).25a.Supposea0andb>0.Let"(t)=(a+bt)p.Then"iscontinuouson[0;1)forallp.Forp=1,"(t)=a+btso"00(t)=0.For10.For00.Hence,byQ24,"isconvexfor1p<1andconcavefor01,"00(t)>0forallt2(0;1)so"0isstrictlyincreasingon(0;1).Nowforx1.Similarly,"isstrictlyconcavefor00andletE=fx2[0;1]:jf(x)j>jjfjj1�"g.Thenjjfjjp=R1R(jfjp)1=p(jfjp)1=p(jjfjj�")(mE)1=p.IfmE=0,thenjjfjjjjfjj�".Contradiction.0E111ThusmE>0andlimp!1jjfjjpjjfjj1�".Since">0isarbitrary,limp!1jjfjjpjjfjj1.Hencelimp!0jjfjjp=jjfjj1.R1R1R1R13.jjf+gjj1=0jf+gj0(jfj+jgj)=R0jfjR+0jgj=jjfjj1+Rjjgjj1.4.Supposef2L1andg2L1.Thenjfgjjfjjjgjj=jjgjjjfj=jjfjjjjgjj.11116.2TheMinkowskiandH•olderinequalities5a.LetfandgbetwononnegativefunctionsinLpwith00andpjjgjjp>0.Let=jjfjjpand=jjgjjpsof=
f0andg=
g0wherejjf0jjp=jjg0jjp=1.Set=
=(+).Then1�=
=(+)andjf+gjp=(f+g)p=(
f+
g)p=(+)p(f+000(1�)g)p(+)p(fp+(1�)gp)byconcavityofthefunction"(t)=tpfor01.Letqbesuchthat1=p+1=q=1.Notethat000q=pp0=(1�p0).Letu=(fg)pandletv=g�p.Thenfg=up,fp=uvandgq=vp=(p�1).RR000pLetqbesuchthat1=p+1=q=1.BytheH•olderinequality,juvjjjujjp0jjvjjq0.i.e.jfjR00R0000RRRRR(jfgjpp)1=p(jgjpp=(1�p))(p�1)=p=(jfgj)p(jgjq)1�p.Hencejfgj(jfjp)1=p(jgjq)1=q=jjfjjpjjgjjq.7a.Forp=1,jjhv+vijj1=supjv+vjsup(jvj+jvj)supjvj+supjvj=jjhvijj1+jjhvijj1.For1p<1,let=jjhijjand=jjhijjsohi=h0iandhi=h0iwherejj0jj=vpvpvvvPvvpjj0jj=1.Set=
=(+).Then1�=
=(+)andjjh+ijj=(j+jp)1=pPvpPPvvpPvv((jj+jj)p)1=p=((j0j+j0j)p)1=p=((+)p[j0j+(1�)j0j]p)1=p((+)p[j0jp+vvvvvvv(1�)j0jp])1=p=+=jjhijj+jjhijj.vvpvpPP7b.Forp=1;q=1,jvvjsupjvjjvj=jjhvijj1jjhvijj1.For10andjjgjj>0.Bypart(a),jfjjgjjfj+jgj=1+1=1.pqjjfjjpqRpjjgjjqpjjfjjpqjjgjjqpqHencejfgjjjfjjjjgjj.Equalityholdsinpart(a)ifandonlyifb=ap�1.Thusequalityholdshereifpqandonlyifjjfjjp�1jgj=jjgjjjfjp�1.Equivalently,jjfjjpjgjq=jjgjjqjfjp.pqpq8c.Suppose01,q0>1andpp0�pq0qqpq1=p0+1=q0=1.Thus(ab)pb�p(ab)+b=pab�pbsoappab�pbandaba+b.p0q0qqpq课后答案网R8d.Byasimilarargumentaspart(b),jfgjjjfjjpjjgjjq.6.3Convergenceandcompleteness9.LethfibeaconvergentsequenceinLp.Thereexistsf2Lpsuchthatforany">0,thereexistsNnsuchthatjjfn�fjjp<"=2fornN.Nowforn;mN,jjfn�fmjjpjjfn�fjjp+jjfm�fjjp<".ThushfniisaCauchysequence.www.hackshp.cn10.LethfibeasequenceoffunctionsinL1.Supposejjf�fjj!0.Given">0,thereexistsNsuchnn1thatinffM:mft:jfn(t)�f(t)j>Mg=0g<"fornN.Thusmft:jfn(t)�f(t)j"g=0fornN.LetE=ft:jf(t)�f(t)j"g.ThenmE=0andhficonvergesuniformlytofonEc.Conversely,nnsupposethereexistsasetEwithmE=0andhficonvergesuniformlytofonEc.Given">0,therenexistsNsuchthatjf(t)�f(t)j<"=2fornNandt2Ec.Thusft:jf(t)�f(t)j>"=2gEfornnnN.HenceinffM:mft:jfn(t)�f(t)j>Mg=0g<"fornN.i.e.jjfn�fjj1!0.11.LethfibeaCauchysequenceinL1.Given">0,thereexistsNsuchthatinffM:mft:njfn(t)�fm(t)j>Mg=0g=jjfn�fmjj1<"=2forn;mN.Thusforn;mN,thereexistsM<"=2suchthatmft:jfn(t)�fm(t)j>Mg=0somft:jfn(t)�fm(t)j>"=2g=0.Thenhfniconvergesa.e.toafunctionfandjf�fj<"=2a.e.fornNsojfjjfj+"=2a.e.andf2L1.Furthermore,nNinffM:mft:jfn(t)�f(t)j>Mg=0g<"fornN.i.e.jjfn�fjj1!0.12.Let1p<1andleth(n)p.Given">0,thereexistsNsuchthatvibeaCauchysequencein`P(n)(m)pp(n)(m)ppjv�vj<"forn;mN.Inparticular,jv�vj<"forn;mNandeachv.Thusfor(n)Pk(n)ppeachv,hviisCauchyinRsoitconvergestosomev.Considerhvi.Thenv=1jv�vj<"forP(n)pp(n)ppeachkandeachnNsojv�vj<"fornN.Thushvkhdaw.com�vi2`fornNsohvi2`28若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
(n)andjjhvi�hvijjp!0.13.LetC=C[0;1]bethespaceofallcontinuousfunctionson[0;1]andde
nejjfjj=maxjf(x)jforf2C.ItisstraightforwardtocheckthatjjjjisanormonC.LethfnibeaCauchysequenceinC.Given">0,thereexistsNsuchthatmaxjfn(x)�fm(x)j<"forn;mNsojfn(x)�fm(x)j<"forn;mNandx2[0;1].Thushfn(x)iconvergestosomef(x)foreachx2[0;1].Furthermore,theconvergenceisuniform.Thusf2C.Also,maxjfn(x)�f(x)j<"fornN.i.e.jjfn�fjj!0.14.Itisstraightforwardtocheckthatjjjjisanormon`1.Leth(n)1.1vibeaCauchysequencein`(n)(m)(n)(m)Given">0,thereexistsNsuchthatsupjv�vj<"forn;mN.Thenjv�vj<"for(n)(n)eachvandn;mN.Thushviconvergestosomevforeachvandjv�vj<"fornN.Thenjjj(N)1.Also,supj(n)(n)vvj+"foreachvandhvi2`v�vj<"fornN.i.e.jjhvi�hvijj1!0.15.Letcbethespaceofallconvergentsequencesofrealnumbersandletc0bethespaceofallsequences(n)whichconvergeto0.Itisstraightforwardtocheckthatjjjj1isanormoncandc0.Lethvibea(n)(m)Cauchysequenceinc.Given">0,thereexistsNsuchthatsupjv�vj<"forn;mN.Then(n)(m)(n)jv�vj<"foreachvandn;mN.Thushviconvergestosomevforeachv.Nowforeachv000000(N)(N)(N)(N)andvkhdaw.com,thereexistsNsuchthatjv�v0jjv�vj+jv�v0j+jv0�v0j<".Thushvi(n)(n)(n)isCauchyinR.Hencehvi2candsupjv�vj<".i.e.jjhvi�hvijj1!0.IfhviisaCauchy(n)(n)sequenceinc0,thenhviconvergesto0sincejvjjv�vj+jvj.16.LethfibeasequenceinLp,1p<1,whichconvergesa.e.toafunctionfinLp.Supposenjjfn�fjjp!0.Thenjjfnjjp!jjfjjpsincejjjfnjjp�jjfjjpjjjfn�fjjp.Conversely,supposeRjjfjj!jjfjj.Now2p(jfjp+jfjp)�jf�fjp0foreachnsobyFatou"sLemma,2p+1jfjpnRppnRnRRRlim2p(jfjp+jfjp)�jf�fjp=2p+1jfjp�limjf�fjp.Thuslimjf�fjp0limjf�fjp.nnnnnHencejjfn�fjjp!0.17.LethfibeasequenceinLp,10,thereexists>0suchthatRnpjgjq<("=4M)qwhenevermE<.ByEgoro
"sTheorem,thereexistsEsuchthatmE<andhfiEnconvergesuniformlytofonEc.ThusthereexistsNsuchthatjf(x)�f(x)j<"=(2(mEc)1=pjjgjj)RRRRnRRqfornNandx2Ec.Nowjfg�fgjjf�fjjgj(jf�fjp)1=p(jgjq)1=q+(jf�RnnEnEERcnfjp)1=p(jgjq)1=q2M("=4m)+("=(2(mEc)1=pjjgjj))(mEc)1=pjjgjj="fornN.i.e.fg=REcqqlimfng.课后答案网Forp=1,itisnottrue.LetRfn=n R[0;1=n]Rforeachn.Thenfn!0andjjfnjj1=1foreachn.Letg= 2L1.Thenfg=0butfg=f=1foreachn.[0;1]nn18.Letf!finLp,1p<1andlethgibeasequenceofmeasurablefunctionssuchthatjgjMnnRnforallnandg!ga.e.Given">0,thereexists>0suchthatjfjp<("=8M)pwhenevernEmE<.ByEgoro
"sTheorem,thereexistsEsuchthatmE<andhgniconvergesuniformlytogonEc.ThusthereexistsNsuchthatjjf�fjj<"=2Mandjg(x)�g(x)j<"=(4(mEc)1=pjjfjj)www.hackshp.cnnpnRpfornNandx2Ec.Nowjjgf�gfjjjjgf�gfjj+jjgf�gfjj=(jgjpjf�fjp)1=p+RnnRpnnnRpnpnn(jg�gjpjfjp)1=pMjjf�fjj+(jg�gjpjfjp)1=p+(jg�gjpjfjp)1=p<"=2+("=8M)(2M)+nnpEnEcn"=(4(mEc)1=pjjfjj)(mEc)1=pjjfjj="fornN.Thusjjgf�gfjj!0.ppnnp6.4ApproximationinLppPm�11Rk+1pPm�11Rk+1p*19.jjT(f)jjp=jjk=0k+1�k(kf) [k;k+1)jjpk=0jjk+1�k(kf) [k;k+1)jjp.Nowjj1(Rk+1f) jjp=Rbj1(Rk+1f) jp=Rk+11jRk+1fjp.Bythek+1�kk[k;k+1)pak+1�kk[k;k+1)k(k+1�k)pkH•olderinequality,jRk+1fjpRk+1jfjp(Rk+11)p=q=Rk+1jfjp(Rk+11)p�1.ThusjjT(f)jjpkkkkkpPm�1Rk+11Rk+1jfjp(Rk+11)p�1=Pm�11Rk+1jfjp(�)p�1=Rbjfjp.k=0k(k+1�k)pkkk=0(k+1�k)p�1kk+1kaHencejjT(f)jjpjjfjjpandjjT(f)jjjjfjj.ppppR*20.ByChebyshev"sinequality,forany">0,j"�fjp"pmfx:j"(x)�f(x)jp>"pg.Thusmfx:j"(x)�f(x)j>"g=mfx:j"(x)�f(x)jp>"pg"�pjj"�fjjp<"forsucientlysmall.p29khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
6.5BoundedlinearfunctionalsontheLpspaces21a.Letgbeanintegrablefunctionon[0RR;1].Ifjjgjj16=0,letf=sgn(g).Thenfisaboundedmeasurablefunction,jjfjj1=1andfg=jgj=jjgjj1jjfRjj1.IfRjjgjj1=0,theng=0a.e.Letf=1.Thenfisaboundedmeasurablefunction,jjfjj1=1andfg=g=0=jjgjj1jjfjj1.21b.Letgbeaboundedmeasurablefunction.GivenRR">0,letE=fx:g(x)>jjgjj1�"gandletf= E.Thenfg=Eg(jjgjj1�")mE=(jjgjj1�")jjfjj1.22.LetFbeaboundedlinearfunctionalon`p.Foreachv,letebethesequencewith1inthePvv-thentryand0elsewhere.Forp=1,notethatjjhi�nejj!0foreachhi2`1soPvv=1vv1vF(hvi)=vF(ev)bylinearityandcontinuityofF.NowjF(ev)Pj=jF(ev)j=jjevjj1jjFjjforallvsohF(e)i2`1andjjhF(e)ijjjjFjj.Conversely,jF(hi)j=jF(e)jjjhijjjjhF(e)ijjsovv1vvvv1v1jjhF(e)ijjjF(hi)j=jjhijjforallnonzerohi2`1.ThusjjhF(e)ijjjjFjj.v1vv1vv1PnpPFor11,choosef=jg�hjq�2(g�h).ThenRRRjfjp=jg�hjp(q�1)=jg�hjqsof2Lp.Nowjg�hjq�2(g�h)g=jg�hjq�2(g�h)hsojg�hjq=0.RRThusg=ha.e.Forp=1,choosef=sgn(g�h).Thenf2L1andf(g�h)=jg�hj.Nowfg=fhRRsojg�hj=f(g�h)=0.Thusg=ha.e.7MetricSpaces课后答案网7.1Introduction1a.Clearly,(x;y)0forallx;y.Now(x;y)=0ifandonlyifjx�yj=0foralliifandonlyifiix=yforalliifandonlyifx=y.Sincejx�yj=jy�xjforeachi,(x;y)=(y;x).Finally,iiPnPniiPniiPn(x;y)=jx�yj(jx�zj+jz�yj)=jx�zj+jz�yj=(x;z)+(z;y).i=1iii=1iiiii=1iii=1iiTheargumentfor+issimilarexceptforthelastproperty.Foranyx;y;z,jx�yjjx�zj+www.hackshp.cnjjjjjjjjz�yjmaxjx�zj+maxjz�yj=+(x;z)++(z;y).Thus+(x;y)+(x;z)++(z;y).jjiiiiii1b.Forn=2(resp.n=3),fx:(x;y)<1gistheinteriorofthecircle(resp.sphere)withcenteryandradius1.fx:(x;y)<1gistheinteriorofthediamond(resp.bi-pyramid)withcenterywithheightandwidth2.fx:+(x;y)<1gistheinteriorofthesquare(resp.cube)withcenteryandsidesoflength1.2.Suppose0<"<�(x;z)andy2Sz;".Then(z;y)<"<�(x;z).Hence(x;y)(x;z)+(z;y)<soy2Sx;.3a.Foranyx,(x;x)=0.If(x;y)=0,then(y;x)=(x;y)=0.If(x;z)=0and(z;y)=0,then0(x;y)(x;z)+(z;y)=0so(x;y)=0.Thus(x;y)=0isanequivalencerelation.LetXbethesetofequivalenceclassesunderthisrelation.Supposexandx0areinthesameequivalenceclass.Alsosupposethatyandy0areinthesameequivalenceclass.Then(x;y)(x;x0)+(x0;y)=(x0;y)(x0;y0)+(y0;y)=(x0;y0).If(x;y)=0,thenxandyareinthesameequivalenceclass.Thusde
nesametriconX.3b.LetbeanextendedmetriconX.Foranyx,(x;x)=0<1.If(x;y)<1,then(y;x)=(x;y)<1.If(x;z)<1and(z;y)<1,then(x;y)(x;z)+(z;y)<1.Thus(x;y)<1isanequivalencerelation.LetX0beapartoftheextendedmetricspace(khdaw.comX;)andletxbearepresentative30若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
ofX0.Ify2X0,thenforanyz2Sy;Swith>0,(x;z)S(x;y)+(y;z)<1soz2X0.i.e.Sy;X0.ThusX0isopen.NowX=XsoX0=Xn6=0X.Sincetheunionisopen,X0isclosed.7.2Openandclosedsets4a.Sincecontinuousfunctionson[0;1]arebounded,CL1.ByQ6.3.13,Ciscomplete.LetgbeapointofclosureofC.Foreveryn,thereexistsfn2Csuchthatjjfn�gjj1<1=n.ThenhfniisaCauchysequenceinCanditconvergestogsog2C.ThusCisaclosedsubsetofL1.4b.Letgbeapointofclosureofthesetofintegrablefunctionsthatvanishfor0t<1=2.ForeachR1=2R1=2R1=2nR,thereexistsfninthesetsuchthatjjfn�gjj1<1=n.Then0jgj0jfn�gj+0jfnj1jfn�gj<1=nforalln.Hencegvanishesa.e.andthesetofintegrablefunctionsthatvanishfor00t<1=2isclosedinL1.RRRR4c.LetRx(t)beameasurablefunctionwithRRx<1.Let=1�x.Foranyy2Sx;R,jy�xj<soy=(y�x)+x<+x<1.Hencethesetofmeasurablefunctionsx(t)withx<1isopeninL1.khdaw.comT5.IfEFandFisclosed,thenEF=F.ThusEFforclosedsetsF.Conversely,sinceTEFEisaclosedsetcontainingE,FE.EFS5a.Fromthede
nition,EisanopensubsetofEsoEO.Conversely,foranyopensubsetOEOEandy2O,thereexists>0suchthatx2OEforallxwith(x;y)<.ThusOEforSanyopensubsetOEsoE.TSOES5b.(E)c=(F)c=Fc=Fc=(Ec).EFEFFcEc6a.ConsidertheballSy;=fx:(x;y)<g.Foranyx2Sy;,let0<"<�(x;y).ByQ2,Sx;"Sy;soSy;isopen.6b.ConsiderthesetS=fx:(x;y)g.Takex2Sc.Then(x;y)>.Let0=(x;y)�.For0ccanyz2Sx;0,(z;y)(x;y)�(x;z)>(x;y)�=soz2S.ThusSisopenandSisclosed.6c.Thesetinpart(b)isnotalwaystheclosureoftheballfx:(x;y)<g.Forexample,letXbeanymetricspacewithjXj>1andbeingthediscretemetric.i.e.(x;y)=1ifx6=yand(x;y)=0ifx=y.Thenfx:(x;y)<1g=fyg,fx:(x;y)<1g=fygandfx:(x;y)1g=X.*7.Rnisseparablewiththesetof课后答案网n-tuplesofrationalnumbersbeingacountabledensesubset.Cisseparablewiththesetofpolynomialson[0;1]withrationalcoecientsbeingacountabledensesubset(Weierstrassapproximationtheorem).L1isnotseparable.Consider and wherex;y2[0;1].Thenjj � jj=1ifx6=y.[0;x][0;y][0;x][0;y]1IfthereexistsacountabledensesubsetD,thenthereexistsd2Dandx;y2[0;1]withx6=ysuchthatjj [0;x]�djj1<1=2andjj [0;y]�djj1<1=2.Thenjj [0;x]� [0;y]jj1<1.Contradiction.L1isseparable.ByProposition6.8,givenwww.hackshp.cnf2L1and">0,thereexistsastepfunction"suchthatjjf�"jj1<".Wemayfurtherapproximate"byastepfunctionwherethepartitionintervalshaverationalendpointsandthecoecientsarerationaltogetacountabledensesubset.7.3Continuousfunctionsandhomeomorphisms8.Lethbethefunctionon[0;1)givenbyh(x)=x=(1�x).Thefunctionh,beingarationalfunction,iscontinuouson[0;1).Ifh(x)=h(y),thenx(1�y)=y(1�x)sox=y.Thushisone-to-one.Foranyy2[0;1),letx=y=(1+y).Thenx2[0;1)andh(x)=y.Thushisonto.Theinversefunctionh�1isgivenbyh�1(x)=x=(1+x),whichiscontinuous.Hencehisahomeomorphismbetween[0;1)and[0;1).9a.Fora
xedsetE,letf(x)=(x;E)=infy2E(x;y).Given">0,let=".When(x;z)<,takeanyy2E.Thenf(x)=(x;E)(x;y)(x;z)+(z;y)<+(z;E)"+f(z).Thusf(x)�f(z)<".Similarly,byinterchangingxandz,f(z)�f(x)<".Thusjf(x)�f(z)j<"andfiscontinuous.9b.If(x;E)=0,thenforany>0,thereexistsy2Esuchthat(x;y)<sox2E.Conversely,if(x;E)>0,say(x;E)=,then(x;y)>
=2forally2Esox=2khdaw.comE.Hencefx:(x;E)=0g=E.31若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
10a.SupposeandareequivalentmetricsonX.Theidentitymappingisahomeomorphismbetween(X;)and(X;).Thusgivenx2Xand">0,thereexists>0suchthatif(x;y)<,then(x;y)<".Byconsideringtheinversefunction,weseethatif(x;y)<,then(x;y)<".Conversely,thetwoimplicationsshowthattheidentitymappingiscontinuousfrom(X;)to(X;)aswellasfrom(X;)to(X;).Sincetheidentitymappingisclearlybijective,itisahomeomorphismsoandareequivalentmetrics.10b.Given">0,let="=n.When+(x;y)<,(x;y)0with0<"=nsuchthat(x;y)<0implies+(x;y)<.Thenwhen(x;y)<0,p(x;y)<".When(x;y)<0,(x;y)(x;y)n<".Thusandareequivalentmetrics.10c.Considerthediscretemetric.Letx=(0;:::;0).Forany>0,wecanchoosey6=xsuchthat(x;y)<but(x;y)=1.Similarlyforand+.Thusisnotequivalenttothemetricsinpart(b).11a.LetbeametriconasetXandlet==(1+).Clearly,(x;y)0with(x;y)=0ifandonlyifx=y.Also,(x;y)=(y;x).Now(x;y)=(x;y)=1�11�1=1+(x;y)1+(x;y)1+(x;z)+(z;y)(x;z)+(z;y)(x;y)1+(x;zkhdaw.com)+(z;y)(x;z)+(z;y).HenceisametriconX.Notethat(x;y)=1�(x;y)=h((x;y))wherehisthefunctioninQ8.Sincehiscontinuousat0,given">0,thereexists0>0suchthath((x;y))<"when(x;y)<0.Nowgiven">0,let0andgivenN,thereexistsN0suchthat(x;x)<"forkN0.Pickkmax(N;N0).nkThennkkNand(x;xnk)<".Thusxisaclusterpointofthesequencehxni.13.Supposethesequence课后答案网hxniconvergestox.Theneverysubsequenceofhxnialsoconvergestoxandsohasxasaclusterpoint.Conversely,supposehxnidoesnotconvergetox.Thereexists">0suchthatforeachN,thereexistsnNwith(x;xn)".Pickn1suchthat(x;xn1)".Supposexn1;:::;xnkhavebeenchosen.Thenpicknk+1nksuchthat(x;xnk+1)".Thesubsequencehxnkidoesnothavexasaclusterpoint.Ifeverysubsequenceofhxnihasinturnasubsequencethatconvergestox,theneverysubsequenceofhxnihasxasaclusterpointbyQ12.Hencethesequencewww.hackshp.cnhxniconvergestox.14.LetEbeasetinametricspaceX.IfxisaclusterpointofasequencefromE,thengiven">0,thereexistsnsuchthat(x;xn)<".Sincexn2E,x2E.Ontheotherhand,ifx2E,thenforeachn,thereexistsxn2Ewith(x;xn)<1=n.Thesequencehxniconvergestox.15.SupposeaCauchysequencehxniinametricspacehasaclusterpointx.ByQ12,thereisasubsequencehxnkithatconvergestox.Given">0,thereexistsNsuchthat(x;xnk)<"=2forkNand(xn;xm)<"=2forn;mN.NowforkN,(x;xk)(x;xnk)+(xnk;xk)<".Thushxniconvergestox.16.LetXandYbemetricspacesandfamappingfromXtoY.SupposefiscontinuousatxandlethxnibeasequenceinXthatconvergestox.Given">0,thereexists>0suchthat(f(x);f(y))<"if(x;y)<.TherealsoexistsNsuchthat(x;xn)<fornN.Thus(f(x);f(xn))<"fornNsothesequencehf(xn)iconvergestof(x)inY.Conversely,supposefisnotcontinuousatx.Thenthereexists">0suchthatforeveryn,thereexistsxnwith(x;xn)<1=nbut(f(x);f(xn))".Thesequencehxniconvergestoxbuthf(xn)idoesnotconvergetof(x).17a.LethxniandhynibeCauchysequencesfromametricspaceX.Given">0,thereexistsNsuchthat(xn;xm)<"=2and(yn;ym)<"=2forn;mN.Now(xn;yn)(xn;xm)+(xm;ym)+(ym;yn)so32khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
(xn;yn)�(xm;ym)(xn;xm)+(yn;ym).Similarly,(xm;ym)�(xn;yn)(xn;xm)+(yn;ym).Thusj(xn;yn)�(xm;ym)j(xn;xm)+(yn;ym)<"forn;mN.Hencethesequenceh(xn;yn)iisCauchyinRandthusconverges.17b.De
ne(hxi;hyi)=lim(x;y)forCauchysequenceshxiandhyi.Then(hxi;hyi)0nnnnnnnnsince(x;y)0foreachn.Also,(hxi;hxi)=lim(x;x)=0.Furthermore,(hxi;hyi)=nnnnnnnnlim(x;y)=lim(y;x)=(hyi;hxi).Finally,(hxi;hyi)=lim(x;y)lim(x;z)+nnnnnnnnnnnnlim(z;y)=(hxi;hzi)+(hzi;hyi).HencethesetofallCauchysequencesfromametricspacennnnnnbecomesapseudometricspaceunder.17c.De
nehxitobeequivalenttohyi(writtenashxihyi)if(hxi;hyi)=0.Ifhxinnnnnnnhx0iandhyihy0i,thenj(hxi;hyi)�(hx0i;hy0i)j(hxi;hx0i)+(hyi;hy0i)=0sonnnnnnnnnnn(hxi;hyi)=(hx0i;hy0i).If(hxi;hyi)=0,thenhxihyisotheyareequalinX.Thusnnnnnnnnthepseudometricspacebecomesametricspace.Associateeachx2XwiththeequivalenceclassinXcontainingtheconstantsequencehx;x;:::i.Thisde
nesamappingTfromXontoT[X].Since(Tx;Ty)=lim(x;y)=(x;y),ifTx=Ty,then(x;y)=0sox=y.ThusTisone-to-one.Also,TiscontinuousonXanditsinverseiscontinuousonT[X].Hencekhdaw.comTisanisometrybetweenXandT[X]X.Furthermore,T[X]isdenseinX.17d.IfhxniisaCauchysequencefromX,wemayassume(bytakingasubsequence)that(xn;xn+1)<2�n.Lethhxi1i1beasequenceofsuchCauchysequenceswhichrepresentsaCauchyse-n;mn=1m=10quenceinX.Given">0,thereexistsNsuchthatform;mN,(hxn;mi;hxn;m0i)<"=2.i.e.limn(xn;m;xn;m0)<"=2.WemayassumethatfornN,(xn;m;xn;m0)<"=2.Inparticular,1(xm;m;xm;m0)<"=2.Wemayalsoassumethat(xm;m0;xm0;m0)<"=2sincethesequencehxn;m0in=10isCauchyinX.Thus(xm;m;xm0;m0)<"form;mNsothesequencehxn;niisCauchyinXandrepresentsthelimitoftheCauchysequenceinX.*17e.TisanisometryfromXontoT[X]andT�1isanisometryfromT[X]ontoX.ThusthereisauniqueisometryT0fromXYontoXthatextendsT.Similarly,thereisauniqueisometryT00fromXontoXYthatextendsT�1.ThenT0j=TandT00j=T�1so(T0T00)j=idandXT[X]T[X]T[X]000000(TT)jX=idX.SinceT[X]isdenseinXandXisdenseinXY,wehaveTT=idXandT00T0=idso(T0=T00)�1.HenceXisisometricwiththeclosureofXinY.XY18.Let(X;)and(Y;)betwocompletemetricspaces.Leth(xn;yn)ibeaCauchysequenceinXY.Since(x;x)((x;x)2+(y;y)2)1=2=
((x;y);(x;y)),thesequencehxiisCauchyinnmnmnmnnmmnX.Similarly,thesequence课后答案网hyniisCauchyinY.SinceXiscomplete,hxniconvergestosomex2X.Similarly,hyniconvergestosomey2Y.Given">0,thereexistsNsuchthat(xn;x)<"=2and(y;y)<"=2fornN.Then
((x;y);(x;y))=((x;x)2+(y;y)2)1=2<"fornN.Hencethennnnnsequenceh(xn;yn)iconvergesto(x;y)2XYandXYiscomplete.7.5Uniformcontinuityanduniformity19.(hx;yi;hx0;y0i)=(www.hackshp.cnx;x0)+(y;y0)0.(hx;yi;hx0;y0i)=0ifandonlyif(x;x0)=0and11(y;y0)=0ifandonlyifx=x0andy=y0ifandonlyifhx;yi=hx0;y0i.(hx;yi;hx0;y0i)=1(x;x0)+(y;y0)=(x0;x)+(y0;y)=(hx0;y0i;hx;yi).(hx;yi;hx0;y0i)=(x;x0)+(y;y0)11(x;x00)+(x00;x0)+(y;y00)+(y00;y0)=(hx;yi;hx00;y00i)+(hx00;y00i;hx0;y0i).Henceisa111metric.Similarly,1isametric.Given">0,let="=2.When
(hx;yi;hx0;y0i)<,(x;x0)2<"2=4and(y;y0)2<"2=4so(hx;yi;hx0;y0i)=(x;x0)+(y;y0)<".Also,(hx;yi;hx0;y0i)=max((x;x0);(y;y0))<".When11p(hx;yi;hx0;y0i)<,(x;x0)<"=2and(y;y0)<"=2so
(hx;yi;hx0;y0i)<"2=4+"2=4<".When1p<,(x;x0)<"=2and(y;y0)<"=2so
(hx;yi;hx0;y0i)<"2=4+"2=4<".Henceand111areuniformlyequivalenttotheusualproductmetric
.20.LetfbeauniformlycontinuousmappingofametricspaceXintoametricspaceYandlethxnibeaCauchysequenceinX.Given">0,thereexists>0suchthat(x;y)<implies(f(x);f(y))<".ThereexistsNsuchthat(xn;xm)<forn;mN.Thus(f(xn);f(xm))<"forn;mN.Hencehf(xn)iisaCauchysequenceinY.21a.LethxnibeasequencefromEthatconvergestoapointx2E.ThenhxniisCauchyinXsohf(xn)iisCauchyinY.SinceYiscomplete,hf(xn)iconvergestosomey2Y.33khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
21b.Supposehxiandhx0ibothconvergetox.Supposehf(x)iconvergestoyandhf(x0)iconvergestonnnny0withy6=y0.Let"=(y;y0)=4>0.Thereexists>0suchthat(x;x0)<implies(f(x);f(x0))<".TherealsoexistsNsuchthat(x;x)<=2and(x0;x)<=2fornN.Thus(x;x0)<nnnmforn;mNso(f(x);f(x0))<"forn;mN.Wemayassumethat(f(x);y)<"andnmn(f(x0);y0)<"fornN.Then(y;y0)(y;f(x))+(f(x);f(x0))+(f(x0);y0)<3"=nnnnn3(y;y0)=4.Contradiction.Hencehf(x)iandhf(x0)iconvergetothesamepoint.Byde
ningy=g(x),nnwegetafunctiononEextendingf.21c.Given">0,thereexists>0suchthat(x;x0)<implies(f(x);f(x0))<"=3forx;x02E.Suppose(x;x0)<=3withx;x02E.LethxibeasequenceinEconvergingtoxandlethx0ibeannsequenceinEconvergingtox0.ThereexistsNsuchthat(x;x)<=3and(x0;x0)<=3fornN.nnThen(x;x0)<fornNso(f(x);f(x0))<"=3fornN.Also,hf(x)iconvergestosomennnnny=g(x)2Yandhf(x0)iconvergestosomey0=g(x0)2Y.Wemayassume(f(x);g(x))<"=3andnn(f(x0);g(x0))<"=3fornN.Thus(g(x);g(x0))<".HencegisuniformlycontinuousonE.n21d.LethbeacontinuousfunctionfromEtoYthatagreeswithfonE.Letx2EandlethxnibeasequenceinEconvergingtox.Thenhh(xn)iconvergestoh(x)andhg(xn)iconvergestog(x).Sinceh(xn)=g(xn)foralln,g(x)=h(x).Hencehg.22a.khdaw.comGiven">0,let="=n.When+(x;y)<,(x;y)0with0<"=sqrtnsuchthat(x;y)<0implies+(x;y)<.pThenwhen(x;y)<0,(x;y)<".When(x;y)<0,(x;y)(x;y)n<".Thusandareuniformlyequivalentmetrics.033Pn0*22b.De
ne(x;y)=jx1�y1j+i=2jxi�yij.Thenisametriconthesetofn-tuplesofrealnumbers.Givenx2Rnand">0,choose0,choosenlargeenoughsothat3n2>1.Letx=hn;0;:::;0iandlety=hn+;0;:::;0i.Then(x;y)=and0(x;y)=(n+)3�n3>课后答案网3n2>1.(x;y)22c.Notethat(x;y)==h((x;y))wherehisthefunctioninQ8.Sincehiscontinuousat1�(x;y)0,given">0,thereexists0>0suchthath((x;y))<"when(x;y)<0.Nowgiven">0,let0,thereexists>0suchthat(x;x0)<implies(f(x);f(x0))<".ThereSkexist
nitelymanyballsSxn;thatcoverX.i.e.X=n=1Sxn;.Takey2Y.Theny=f(x)forsomeSkx2X.Nowx2Sxn;forsomenso(x;xn)<and(f(x);f(xn))<".HenceY=n=1Sf(xn);"soYistotallybounded.Thustotalboundednessisauniformproperty.23c.ByQ8,thefunctionh(x)=x=(1�x)isahomeomorphismbetween[0;1)and[0;1).Let">0begiven.ChooseNsuchthatN>2="andletxn=(n�1)=Nforn=1;:::;N.Theintervals(xn�1=N;xn+1=N)[0;1)areballsofradius"thatcover[0;1).Thus[0;1)istotallybounded.Suppose[0;1)istotallybounded.Thentherearea
nitenumberofballsofradius1thatcover[0;1),Sksay[0;1)=n=1Sxn;1.Wemayassumethatx1;:::;xkarearrangedinincreasingorder.Butthenxk+2isnotinanyoftheballsSxn;1.Contradiction.Hence[0;1)isnottotallybounded.Thustotalboundednessisnotatopologicalproperty.23d.Let(X;)beatotallyboundedmetricspace.Foreachn,therearea
nitenumberofballsof34khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
radius1=nthatcoverSX.LetSnbethesetofthecentresoftheseballs.TheneachSnisa
nitesetandS=Snisacountableset.Given">0,chooseNsuchthatN>1=".Foranyx2X,(x;x0)<1=N<"forsomex02SS.ThusSisadensesubsetofX.HenceXisseparable.NQ124a.LethPXk;kibeasequenceofmetricspacesandde
netheirdirectproductZ=k=1Xk.De
ne
(x;y)=12�k(x;y)where==(1+).Then
(x;y)0since(x;y)0forallk.k=1kkkkkkkkkAlso,
(x;y)=0ifandonlyif(x;y)=0forallkifandonlyifx=yforallkifandonlyifkkkkkx=y.Furthermore,
(x;y)=
(y;x)since(x;y)=(y;x)forallk.Nowforeachn,
(x;y)=PnPnPnkkkkkPk1P12�k(x;y)2�k(x;z)+2�k(z;y)2�k(x;z)+2�k(z;y)=k=1kk=1kk=1kk=1kk=1k
(x;z)+
(z;y).Hence
(x;y)
(x;z)+
(z;y).Thus
isametriconZ.Supposeasequencehx(n)iinZconvergestox2Z.Given">0,let"0=min(2�k�1";2�k�2).ThereexistsNsuchthat
(x(n);x)<"0fornN.i.e.P12�k(x(n);x)<"0fornN.Thenk=1kkk(x(n);x)<2k0"0(1+(x(n);x))fornNso(1�2k0"0)(x(n);x)<2k0"0fornN.Sincek0k0k0k0k0k0k0k0k02k0"0=min("=2;1=4),wehave3(x(n);x)=4<2k0"0<"=2fornN.Hence(x(n);x)<"fork0k0k0k0k0k0(n)nNandhxkiconvergestoxkforeachk.(n)Conversely,supposehxkiconvergestoxkforeachk.Thengiven">0,thereexistsNsuchthatP1khdaw.com�k(n)k=N+12k(xk;xk)<"=2.Fork=1;:::;N,thereexistsMsuchthatk(xk;xk)<"=2fornM.Thus
(x(n);x)=PN2�k(x;x)+P12�k(x;x)0,thereexists>0suchthatj"(x)�"(y)j<"=2when(x;y)<.Let0=min(;"=2).When(x;y)<0,(f(x);f(y))=(x;y)+j"(x)�"(y)j<".When(hx;"(x)i;hy;"(y)i)<0,(x;y)<0<".Thus11fisauniformhomeomorphismand(O;)iscomplete.Let==(1+).ByQ22c,isuniformlyequivalentto.Henceisaboundedmetricforwhich(O;)isacompletemetricspace.7.7Compactmetricspaces27.LetXbeametricspace,KacompactsubsetandFaclosedsubset.Considerthefunctionf(x)=(x;F)=infy2F(x;y).ByQ9b,fx:(x;F)=0g=F=F.ThusifFK=;,then(x;F)>0forallx2K.ThefunctionfjKiscontinuousonacompactsetsoitattainsaminimum>0.Thus(x;y)>forallx2Kandally2F.Conversely,ifFK6=;,thenthereexistsx2Kandy2Fsuchthat(x;y)=0so(F;K)=0.khdaw.com35若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
28a.LetXbeatotallyboundedmetricspaceandletf:X!YbeauniformlycontinuousmapontoY.Given">0,thereexists>0suchthat(x;x0)<implies(f(x);f(x0))<".Thereexist
nitelymanyballsfBgkthatcoverX.Takey2Y.Theny=f(x)forsomex2X.Nowx2Bforxn;n=2xn;somenso(x;x)<and(f(x);f(x))<".HencetheballsfBgkcoverYandYistotallynnf(xn);"n=1bounded.28b.Thefunctionh(x)=x=(1�x)isacontinuousmapfrom[0;1)onto[0;1).[0;1)istotallyboundedbut[0;1)isnot.29a.WemayassumeX=2U.Set"(x)=supfr:9O2UwithBx;rOg.Foreachx2X,thereexistsO2Usuchthatx2O.SinceOisopen,thereexistsrsuchthatBx;rO.Thus"(x)>0.SinceXiscompact,itisboundedso"(x)<1.029b.SupposeBx;rOforsomeO2U.If0"(y)+(x;y)suchthatBx;rOforsomeO2U.Now"(y)0,let=".When(x;y)<,j"(x)�"(y)j(x;y)<"bypart(b).Thus"iscontinuousonkhdaw.comX.29d.IfXissequentiallycompact,"attainsitsminimumonX.Let"=inf".Then">0since"(x)>0forallx.29e.Let"beasde
nedinpart(d).Foranyx2Xand<",<"(x)sothereexistsr>suchthatBx;rOforsomeO2U.ThenBx;Bx;rO.Q1*30a.LetZ=k=1Xk.SupposeeachXkistotallybounded.Given">0,chooseNsuchthat2N>2=".Fork>N,pickp2X.ForeachkN,thereexistsA=fx(k);:::;x(k)gsuchthatforkkk1Mk(k)(k)anyx2Xk,thereexistsxj2Akwithk(x;xj)<"=2.LetA=fhxni:xk2AkforkN;xk=(k)pkfork>Ng.ThenjAj=M1MN.Ifhxni2Z,foreachkN,thereexistsxj2Aksuch(k)(k)thatk(xk;xj)<"=2.Letyk=xjforkNandletyk=pkfork>N.Thenhyni2Aand
(x;y)=Pn2�kk(xk;yk)0,thereexistsapolygonalfunction"suchthatjg(x)�"(x)j<"=2forallx2[0;1].Therealsoexistsapolygonalfunctionwhoseright-handderivativeiseverywheregreaterthanninabsolutevalueandj"(x)�(x)j<"=2forallx2[0;1].Then2Fcandnjg(x)�(x)j<"forallx2[0;1].HenceFnisnowheredense.38c.ThesetDofcontinuousfunctionswhichhavea
nitederivativeontherightforatleastonepointof[0;1]istheunionoftheFn"ssoDisofthe
rstcategoryinC.38d.SinceDisofthe
rstcategoryinthecompletemetricspaceC,D6=CsothereisafunctioninCnD,thatis,anowheredi
erentiablecontinuousfunctionon[0;1].39.LetFbeafamilyofreal-valuedcontinuousfunctionsonacompletemetricspaceX,andsupposethatforeachx2XthereisanumberTMxsuchthatjf(x)jMxforallf2F.Foreachm,letEm;f=fx:jf(x)jmg,andletEm=FEm;f.Sinceeachfiscontinuous,Em;fisclosedandsoSEmisclosed.Foreachx2X,thereexistsmsuchthatjf(x)jmforallf2F.HenceX=Em.ThenSO=EXisadenseopensetandforeachx2O,x2EforsomemsothereisaneighbourhoodmmUofxsuchthatUE.Inparticular,FisuniformlyboundedonU.m40a.Supposethatgiven">0,thereexistNandaneighbourhoodUofxsuchthat(f(x0);f(x))<"nfornNandallx02U.Lethxibeasequencewithx=limx.Wemayassumethatx2Ufornnkhdaw.comn37若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
allnNso(fn(xn);f(x))<"fornNandhfniconvergescontinuouslytofatx.Conversely,supposethereexists">0suchthatforanyNandanyneighbourhoodUofx,thereexistsnNandx02Uwith(f(x0);f(x))".Foreachn,letU=B.Thereexistsn1andx2Uwithnnx;1=n1n11(fn1(xn1);f(x))".Supposexn1;:::;xnkhavebeenchosen.Thereexistsnk+1nkandxnk2Ukwith(fnk+1(xnk+1);f(x))".Thenx=limxnkbyconstructionbutf(x)6=limfnk(xnk)sohfnidoesnotconvergecontinuouslytof.40b.LetZ=f1=ng[f0g.De
neg:XZ!Ybyg(x;1=n)=fn(x)andg(x;0)=f(x).Supposegiscontinuousathx0;0iintheproductmetric.Lethxnibeasequencewithx0=limxn.Thenhx0;0i=limhxn;1=niandf(x)=g(x0;0)=limg(xn;1=n)=limfn(xn)sohfniconvergescontinuouslytofatx0.Conversely,supposehfniconvergescontinuouslytofatx0.Leth(xn;zn)ibeasequenceinXZconvergingtohx0;0i.Thenx0=limxn,0=limznandf(x0)=limfn(xn).i.e.g(x0;0)=limg(xn;1=n).Since0=limzn,itfollowsthatg(x0;0)=limg(xn;zn)sogiscontinuousathx0;0i.40c.Lethfniconvergecontinuouslytofatx.Bypart(b),thefunctiongiscontinuousathx;0i.Ifhxniisasequenceconvergingtox,thenf(x)=g(x;0)=limg(xn;0)=limf(xn).Hencefiscontinuousatx.*40d.Lethfnibeasequenceofcontinuousmaps.Supposehfniconvergescontinuouslytofatx.Bypart(a),givenkhdaw.com">0,thereexistsNandaneighbourhoodUofxsuchthat(f(x0);f(x))<"=2fornNnandx02U.Bypart(c),fiscontinuousatxsowemayassumethat(f(x0);f(x))<"=2forx02U.Thus(f(x0);f(x0))<"fornNandx02U.Conversely,supposethatgiven">0,thereexistNnandaneighbourhoodUofxsuchthat(f(x0);f(x0))<"=4forallnNandallx02U.Inparticular,n(fN(x);f(x))<"=4.Lethxkibeasequencewithx=limxk.Wemayassumethatxk2Uforallkand(fN(xk);fN(x))<"=4forkN.Then(f(xk);f(x))(f(xk);fN(xk))+(fN(xk);fN(x))+(fN(x);f(x))<3"=4forkN.Thus(fk(xk);f(x))(fk(xk);f(xk))+(f(xk);f(x))<"forkNsof(x)=limfk(xk)andhfniconvergescontinuouslytofatx.40e.Lethfnibeasequenceofcontinousmaps.SupposehfniconvergescontinuouslytofonX.Foreachx2Xandeach">0,thereexistsNandaneighbourhoodUofxsuchthat(f(x0);f(x0))<"xSxnSforeachnNandx02U.ThenX=UsoforanycompactsubsetKX,KmUforxxxi=1xisomex;:::;x.ThusfornmaxNandx02K,wehave(f(x0);f(x0))<"sohficonverges1mxinnuniformlytofonK.Conversely,supposehfniconvergesuniformlytofoneachcompactsubsetofX.Thenhfniconvergesuniformlytofonfxgforeachx2X.Given">0,thereexistsNsuchthat(fn(x);f(x))<"=3fornN.Sinceeachfniscontinuous,fisalsocontinuous.ThenthereexistsaneighbourhoodUofxsuchthat课后答案网(f(x0);f(x))<"=3and(f(x0);f(x))<"=3forx02U.Thusnn(f(x0);f(x0))<"fornNandx02U.HencehficonvergescontinuouslytofonX.nn40f.LetXbeacompletemetricspaceandhfniasequenceofcontinuousmapsofXintoamet-ricspaceYsuchthatf(x)=limfn(x)foreachx2X.Form;n2N,de
neFm;n=fx2X:(f(x);f(x))1=mforallk;lng.Letx0beapointofclosureofF.Thereisasequencehxiklm;niinFconvergingtox0.Forany">0andk;ln,thereexists>0suchthat(x;x0)<impliesm;n(f(x);f(x0))<"and(www.hackshp.cnf(x);f(x0))<".ThenthereexistsNsuchthat(x;x0)<foriN.kklliThus(f(x0);f(x0))(f(x0);f(x))+(f(x);f(x))+(f(x);f(x0))<2"+1=m.Since"klkkNkNlNlNlisarbitrary,(f(x0);f(x0))1=mandx02FsoFisclosed.klm;nm;nForanyx2X,thereexistsnsuchthat(fk(x);f(x))<1=2mforkn.Thenfork;ln,S(fk(x);fl(x))S(fk(x);f(x))+(f(x);fl(x))<1=msox2Fm;n.HenceX=nFm;n.ByProposi-tion31,O=FisopenandsinceXiscomplete,Oisalsodense.mnm;nm40g.Letx2O.Thenx2Fforsomen.ThusthereexistsaneighbourhoodUofxsuchmm;nthatUF.Itfollowsthatforanyk;lnandanyx02U,(f(x0);f(x0))1=m.Sincem;nklf(x0)=limf(x0),wehave(f(x0);f(x0))1=mforanyknandx02U.lkT40h.LetE=Om.SinceeachOmisopenanddense,EisadenseGbyBaire"stheorem.Ifx2E,thenx2Omforallm.Given">0,choosem>1=".ThereexistsaneighbourhoodUofxandannsuchthat(f(x0);f(x0))1=m<"foranyknandx02U.k40i.LetXbeacompletemetricspaceandhfniasequenceofcontinuousfunctionsofXintoametricspaceY.Supposehfniconvergespointwisetof.Byparts(f),(g),(h)and(a),thereexistsadenseGinXonwhichhfniconvergescontinuouslytof.41a.Let(X;)and(Y;)becompletemetricspacesandf:XY!Zbeamappingintoametric38khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
space(Z;
)thatiscontinuousineachvariable.Fixy02Y.SetFm;n=fx2X:
[f(x;y);f(x;y0)]1=mforallywith(y;y)1=ng.Letx0beapointofclosureofF.ThereisasequencehxiinF0m;nkm;nconvergingtox0.Foreachkandanyywith(y;y)1=n,
[f(x;y);f(x;y)]1=m.Given">0,0kk0thereexists>0suchthat(x;x0)<implies
[f(x;y);f(x0;y)]<"and
[f(x;y);f(x0;y)]<".Then00thereexistsNsuchthat(x;x0)<forkN.Thus
[f(x0;y);f(x0;y)]
[f(x0;y);f(x;y)]+k0N
[f(x;y);f(x;y)]+
[f(x;y);f(x0;y)]<2"+1=m.Since"isarbitrary,
[f(x0;y);f(x0;y)]1=mNN0N000andx02FsoFisclosed.m;nm;nForanyx2Xandm,thereexists>0suchthat(y;y0)<implies
[f(x;y);f(x;y0)]<1=m.ChooseSn>1=.Thenforanyywith(y;y0)<1=n,
[f(x;y);f(x;y0)]<1=msox2Fm;n.HenceX=nFm;n.S41b.LetO=F.ByProposition31,OisopenandsinceXiscomplete,Oisalsodense.Letmnm;nmmx2O.Thenx2Fforsomen.ThusthereexistsaneighbourhoodU0ofxsuchthatU0F.mm;nm;nForanyywith(y;y)1=nandanyx02U0,
[f(x0;y);f(x0;y)]1=m.Wemayassumethat00
[f(x0;y);f(x;y)]1=mforx02U0bycontinuity.Then
[f(x0;y);f(x;y)]2=mforx02U0.000HencethereisaneighbourhoodUofhx;y0iinXYsuchthat
[f(p);f(x;y0)]2=mforallp2U.T41c.khdaw.comLetG=Om.SinceeachOmisopenanddense,GisadenseGbyBaire"stheorem.Ifx2G,thenx2Omforeachm.Given">0,choosem>2=".ThereexistsaneighbourhoodUofhx;y0isuchthat
[f(p);f(x;y0)]2=m<"forp2U.Hencefiscontinuousathx;y0iforeachx2G.41d.LetEXYbethesetofpointsatwhichfiscontinuous.Iffiscontinuousatz2XY,SthenforeachTn,thereexistsn;z>0suchthatf[Bz;n;z]Bf(z);1=n.LetEn=z2SBz;z;1=n=2.ThenE=nEn(c.f.Q2.7.53)andEisaGset.Takehx0;y0i2XYandlet">0begiven.Bypart(c),thereexistsadenseGsetGXsuchthatfiscontinuousathx;y0iforeachx2G.SinceGisdense,thereexistsx2Gsuchthat(x;x)<".Thenhx;yi2Eand
0(hx;yi;hx;yi)=(x;x)<".10110100010ThusEisdenseinXY.41e.Givena
niteproductX1Xpofcompletemetricspaces,wemayregardtheproductas(X1Xp�1)XnwhereX1Xp�1isacompletemetricspace.Letf:X1Xp!ZbeamappingintoametricspaceZthatiscontinuousineachvariable.Byasimilarargumentasinpart(d),thereexistsadenseGX1Xponwhichfiscontinuous.42a.LetXandYbecompletemetricspaces.AlsoletGXandHYbedenseG"s.ThenTTTTTG=GnandH=HnwhereeachGnandHnisopen.ThusGH=GnHn=(GnHn)whereeachGnHnisopenin课后答案网XY.HenceGHisaGinXY.Given">0andhx;yi2XY,thereexistx02Gandy02Hsuchthat(x;x0)<"=2and(y;y0)<"=2.Then
(hx;yi;hx0;y0i)<".HenceGHisdenseinXY.*42b.T42c.LetEbeadenseGinXY.ThenE=OnwhereeachOnisadenseopensetinXY.Bypart(b),foreachn,thereexistsadenseTGsetGnXsuchthatfy:hx;yi2OngisadenseopensubsetofYforeachx2Gnwww.hackshp.cn.LetG=Gn.ThenGisstillacountableintersectionofdenseopensetsTinX.ThusGisadenseGsetinXsuchthatfy:hx;yi2Eg=fy:hx;yi2OngisadenseGforeachx2G.*42d.*43.Let(X;)beacompletemetricspaceandf:X!Rbeanuppersemicontinuousfunction.LetEbethesetofpointsatwhichfiscontinuous.ThenEisaG.Supposefisdiscontinuousatx.Thenfisnotlowersemicontinuousatxsof(x)>limf(y).Thereexistsq2Qsuchthatf(x)q>limf(y).y!xy!xLetF=fx:f(x)qg.Thenx2FnF.Conversely,ifx2FnFforsomeq2Q,thenf(x)qandqqqqqSforany>0,thereexistsywith(x;y)<andf(y)limf(y).ThusEc=FnF.q2Qqqy!xSinceeachFnFisnowheredense,Ecisofthe
rstcategoryandEisresidual.HenceEisadenseqqGinX7.9AbsoluteG"sPPP44.Let=2�n.Then(x;y)=2�n(x;y)2�n=1forallx;y2E.Since0nnnforalln,0.Also,(x;y)=0ifandonlyifn(x;y)=0forallkhdaw.comnifandonlyifx=y.Since39若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
PP(x;y)=(y;x)foralln,(x;y)=(y;x).Also,(x;y)=2�n(x;y)2�n(x;z)+Pnnnn2�n(z;y)=(x;z)+(z;y).ThusisametriconE.nLet">0and
xx2E.Foreachn,thereexistsn>0suchthatn(x;yP)<nimplies(x;y)<"=2and(x;y)<implies(x;y)<"=2.ThereexistsNsuchthat12�n<"=2.Let=nnn=N+1NPN�nmin(1=2;:::;N=2).If(x;y)<,thenn(x;y)<"=2forn=1;:::;Nson=12n(x;y)<"=2and(x;y)<".If(x;y)<,then1(x;y)<21so(x;y)<".HenceisequivalenttoonE.Ifeachnisuniformlyequivalentto,thenisuniformlyequivalentto.45.LetABAbesubsetsofametricspaceandletgandhbecontinuousmapsofBintoametricspaceX.Supposeg(u)=h(u)forallu2A.Letu2B.Thenu2AsothereisasequencehuniinAconvergingtou.SincegandharecontinuousonB,hg(un)iandhh(un)iconvergetof(u)andg(u)respectively.Butg(un)=h(un)forallnsog(u)=h(u).Hencegh.*46a.46b.StartingfromE,letI1;I2;:::bedisjointopenintervalssatisfyingtheconditionsofpart(a)with"=1=2.ForEIj=EIj,repeattheprocesswith"=1=4,gettingintervalsIj;1;Ij;2;:::.Continuing,atthen-thstagegettingintervalsIwith"=2�n.Givenx2E,thereisauniqueintegerksuchj;k;:::;l1thatxkhdaw.com2Ik1.Thenx2EIk1andthereisauniqueintegerk2suchthatx2Ik1;k2andsoon.Thusthereisauniquesequenceofintegersk1;k2;:::suchthatforeachnwehavex2Ik1;:::;kn.46c.Givenasequenceofintegersk1;k2;:::,supposetherearex;y2Esuchthatx;y2Ik1;:::;knforalln.Byconstruction,thediameterofIislessthan2�nforeachn.Thus(x;y)<2�nforallnsok1;:::;kn(x;y)=0andx=y.46d.LetN!bethespaceofin
nitesequencesofintegersandmakeNintoametricspacebyset-ting(i;j)=.Let
betheproductmetriconN!.Given">0,chooseNsuchthat2�N0,thereexistsNsuchthat(f(x0);f(x0))<"=3foranyx02K.Also,thereexistsN0suchNthat(f(x);f(x))<"=3forwww.hackshp.cnkN0.Thus(f(x);f(x))(f(x);f(x))+(f(x);f(x))+NkNkkNkNkN(f(x);f(x))<"forkN0.HencefiscontinuousonX.N48a.LetXbeaseparable,locallycompactmetricspace,and(Y;)anymetricspace.LetfxngbeacountabledensesubsetofSX.ForeachSn,thereisanopensetUnsuchthatxn2UnandUniscompact.ThenX=fxngUnsoX=SUn.Foreachnandanyx2Un,thereisanopensetVxcontainingxwithVxcompact.ThenUnx2UnVxandsinceUniscompact,thereisa
nitenumberofSVx"scoveringUn.LetOnbetheunionofthe
nitenumberofVx"s.ThenX=On.48b.Let(f;g)=P2�n(f;g)where(f;g)=sup(f(x);g(x)).Since(f;g)1forallnnOn1+(f(x);g(x))nn,(f;g)1<1andsince(f;g)0foralln,(f;g)0.Since(f;g)=(g;f)fornnnalln,(f;g)=(g;f).Foreachn,(f;g)=sup(f(x);g(x))=sup[1�1]nOn1+(f(x);g(x))On1+(f(x);g(x))sup[1�1]=sup(f(x);h(x))+(h(x);g(x))(f;h)+(h;g).ThusOn1+(f(x);h(x))+(h(x);g(x))On1+(f(x);h(x))+(h(x);g(x))nn(f;g)(f;h)+(h;g).HencethesetoffunctionsfromXintoYbecomesametricspaceunder.49.LetFbeanequicontinuousfamilyoffunctionsfromXtoY,andletF+bethefamilyofallpointwiselimitsoffunctionsinF,thatisoffforwhichthereisasequencehfnifromFsuchthat40khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
f(x)=limfn(x)foreachx2X.Givenx2Xand">0,thereisanopensetOcontainingxsuchthat(f(x);f(y))<"=3forally2Oandf2F.Nowiff2F+andy2O,thenthereisasequencehfifromnFsuchthatf(x)=limfn(x)sothereisanNsuchthat(fN(x);f(x))<"=3and(fN(y);f(y))<"=3.Then(f(x);f(y))(f(x);f(x))+(f(x);f(y))+(f(y);f(y))<".HenceF+isalsoanNNNNequicontinuousfamilyoffunctions.50.Let0<
1andletF=ff:jjfjj1g.Iff2F,thenmaxjf(x)j+supjf(x)�f(y)j=jx�yj1.Inparticular,jf(x)�f(y)jjx�yjforallx;y.Thusf2C[0;1].Also,Fisanequicontinuousfamilyoffunctionsontheseparablespace[0;1]andeachf2Fisbounded.BytheAscoli-ArzelaTheorem,eachsequencehfniinFhasasubsequencethatconvergespointwisetoacontinuousfunction.HenceFisasequentiallycompact,andthuscompact,subsetofC[0;1].*51a.LetFbethefamilyoffunctionsthatareholomorphicontheunitdisk=fz:jzj<1gwithjf(z)j1.Letf2Fand
xz02.LetUbeanopenballofradiusmin(jz0j;1�jz0j)centredatz0.ThenU.LetCbethecircleofradiusr=2centredatz0.Foreveryzwithinr=4ofz0,01Rf(w)dwf(w)dwz�z0Rf(w)dwf(z)�f(z)=2iC[w�z�w�z0]=2iC(w�z)(w�z0)byCauchy"sintegralformula.Sincejf(z)j1forallf2Fandallz2,jf(z)�f(z0)j4r�1jz�z0jforallf2F.ItfollowsthatFisequicontinuous.khdaw.com*51b.BytheAscoli-ArzelaTheorem,anysequencehfniinFhasasubsequencethatconvergesuniformlytoafunctionfoneachcompactsubsetof.Furthermorefisholomorphicon.*51c.Lethfnibeasequenceofholomorphicfunctionsonsuchthatfn(Tz)!f(z)forallz2.Foreachz2,thereisanMzsuchthatjfn(Sz)jMzforalln.LetEm=nEm;nwhereEm;n=fz:jfn(z)jmg.ThenEmisclosedand=SmEm.ThereisanEmthatisnotnowheredensesoithasnonemptyinterior.ThenO=EisadenseopensubsetofandhfiislocallyboundedonO.mmnByanargumentsimilartothatinpart(a),hfniisequicontinuousonOsothereisasubsequencethatconvergesuniformlytoafunctionfoneachcompactsubsetofO.Furthermore,fisholomorphiconO.8TopologicalSpaces8.1Fundamentalnotions1a.GivenasetX,de
neonXXby(x;y)=0ifx=yand(x;y)=1otherwise.ThenforanySsetAX,A=x2ABx;课后答案网1=2soAisopen.Thustheassociatedtopologicalspaceisdiscrete.IfXhasmorethanonepoint,thenthereisnometriconXsuchthattheassociatedtopologicalspaceistrivialbecauseinametricspace,anytwodistinctpointscanbeenclosedindisjointopensets.1b.LetXbeaspacewithatrivialtopology.IffisacontinuousmappingofXintoR,thenf�1[I]iseither;orXforanyopenintervalI.Takec2R.Thenf�1[c]=f�1[(c�";c+")]iseither;orX.Thusfmustbeaconstantfunction.Conversely,iff(x)=cforallx2X,thenforanyopenintervalI,f�1[I]=;ifc=2Iandfwww.hackshp.cn�1[I]=Xifc2I.Sinceanyopensetofrealnumbersisacountableunionofdisjointopenintervals,itfollowsthatfiscontinuous.HencetheonlycontinuousmappingsfromXintoRaretheconstantfunctions.1c.LetXbeaspacewithadiscretetopology.LetfbeamappingofXintoR.Sincef�1[O]XforanyopensetOR,fiscontinuous.HenceallmappingsofXintoRarecontinuous.2.EistheintersectionofallclosedsetscontainingEsoEE.Also,EE.Ontheotherhand,EistheintersectionofallclosedsetscontainingE,oneofwhichisEitself,soEE.ThusE=E.A[BisaclosedsetcontainingA[BsoA[BA[B.Ontheotherhand,AA[BandBA[BsoAA[BandBA[B.ThusA[BA[B.HenceA[BA[B.IfFisclosed,thenFisaclosedsetcontainingFsoFF.SincewealwayshaveFF,F=F.Conversely,ifF=F,thensinceFisclosed,Fisclosed.Ifx2E,thenxisineveryclosedsetcontainingE.LetObeanopensetcontainingx.IfOE=;,thenEOc.SinceOcisclosed,x2Oc.Contradiction.HenceOE6=;andxisapointofclosureofE.Conversely,supposexisapointofclosureofE.Ifx=2E,thenxisnotinsomeclosedsetFcontainingEsox2Fc.SinceFcisanopensetcontainingx,FcE6=;.Contradiction.Hencex2E.EistheunionofallopensetscontainedinEsoEE.Also,EE.Ontheotherhand,Eis41khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
theunionofallopensetscontainedinE,oneofwhichisEitself,soEE.ThusE=E.ABisanopensetcontainedinABsoAB(AB).Ontheotherhand,ABAandABBso(AB)Aand(AB)B.Thus(AB)AB.Hence(AB)=AB.Ifx2E,thenxisinsomeopensetOcontainedinEsoxisaninteriorpointofE.Conversely,ifxisaninteriorpointofE,thenx2OEforsomeopensetOsox2E.Ifx2(Ec),thenxisinsomeopensetOEcsox=2OcEandthusx2Ec.Conversely,ifx2Ec,thenxisnotinsomeclosedsetFEsox2FcEcandthusx2(Ec).Hence(Ec)=Ec.3.SupposeAXisopen.Thengivenx2A,x2OAwithO=A.Conversely,supposethatgivenSx2A,thereisanopensetOxsuchthatx2OxA.ThenA=x2AOx,whichisopen.4.LetfbeamappingofXintoY.Supposefiscontinuous.ForanyclosedsetF,f�1[Fc]isopen.Butf�1[Fc]=(f�1[F])csof�1[F]isclosed.Conversely,supposetheinverseimageofeveryclosedsetisclosed.ForanyopensetO,f�1[Oc]isclosed.Butf�1[Oc]=(f�1[O])csof�1[O]isopen.Thusfiscontinuous.5.SupposefisacontinuousmappingofXintoYandgisacontinuousmappingofYintoZ.ForanyopensetOZ,g�1[O]isopeninYsof�1[g�1[O]]isopeninX.Butf�1[g�1[O]]=(gf)�1[O].Hencekhdaw.comgfisacontinuousmappingofXintoZ.6.Letfandgbetworeal-valuedcontinuousfunctionsonXandletx2X.Given">0,thereisanopensetOcontainingxsuchthatjf(x)�f(y)j<"=2andjg(x)�g(y)j<"=2whenevery2O.Thenj(f+g)(x)�(f+g)(y)j=jf(x)�f(y)+g(x)�g(y)jjf(x)�f(y)j+jg(x)�g(y)j<"whenevery2O.Thusf+giscontinuousatx.ThereisanopensetO0suchthatjg(x)�g(y)j<"=2jf(x)jandjf(x)�f(y)j<"=2max(jg(x)�"=2jf(x)jj;jg(x)+"=2jf(x)jj)whenevery2O0.Thenj(fg)(x)�(fg)(y)j=jf(x)g(x)�f(x)g(y)+f(x)g(y)�f(y)g(y)jjf(x)jjg(x)�g(y)j+jf(x)�f(y)jjg(y)j<"whenevery2O0.Thusfgiscontinuousatx.7a.LetFbeaclosedsubsetofatopologicalspaceandhxniasequenceofpointsfromF.Ifxisaclusterpointofhxni,thenforanyopensetOcontainingxandanyN,thereexistsnNsuchthatx2O.Supposex=2F.ThenxisintheopensetFcsothereexistsx2Fc.Contradiction.Hencennx2F.7b.Supposefiscontinuousandx=limxn.ForanyopensetUcontainingf(x),thereisanopensetOcontainingxsuchthatf[O]U.TherealsoexistsNsuchthatxn2OfornNsof(xn)2UfornN.Hencef(x)=lim课后答案网f(xn).7c.Supposefiscontinuousandxisaclusterpointofhxni.ForanyopensetUcontainingf(x),thereisanopensetOcontainingxsuchthatf[O]U.ForanyN,thereexistsnNsuchthatxn2Osof(xn)2U.Hencef(x)isaclusterpointofhf(xn)i.*8a.LetEbeanarbitrarysetinatopologicalspaceX.(E)istheintersectionofallclosedsetscontaining(E),oneofwhichisE,so(E)E.Ontheotherhand,EistheintersectionofallclosedsetscontainingEwww.hackshp.cn,oneofwhichis(E)sinceE=E(E),soE(E).Hence(E)=E.ccNowsinceE=Eand(E)=E,newsetscanonlybeobtainediftheoperationsareperformedccalternately.Also,(E)c=(Ec)so(E)c=(E)c=(Ec)=(Ec)=(E)c.HencetheccccdistinctsetsthatcanbeobtainedareatmostE,E,(E)c,(E)c,(E)c,(E)c,(E)c,Ec,ccccEc,(Ec)c,(Ec)c,(Ec)c,(Ec)c,(Ec)c.*8b.LetE=f(0;0)g[fz:1ag=f�1[(a;1)].Since(�1;a)and(a;1)areopen,thesetsfx:f(x)agareopen.Conversely,supposethesetsfx:f(x)agareopenforanyrealnumbera.SinceanyopensetORisaunionofopenintervalsandanyopenintervalisanintersectionofatmosttwoofthesets,f�1[O]isopensofiscontinuous.44khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
Sincefx:f(x)agc=fx:f(x)agisopenandthesetfx:f(x)agisclosed.21.Supposefandgarecontinuousreal-valuedfunctionsonatopologicalspaceX.ForanyrealSnumbera,fx:f(x)+g(x)ag=(fx:g(x)>qgfx:f(x)>a�qg),whichisopen,sof+giscontinuous.Sq2Qfx:f(x)g(x)ag=Sq2Q(fx:g(x)>qg)fx:f(x)>a=qg,whichisopen,sofgiscontinuous.fx:(f_g)(x)ag=fx:f(x)>ag[fx:g(x)>ag,whichisopen,sof_giscontinuous.fx:(f^g)(x)ag=fx:f(x)>agfx:g(x)>ag,whichisopen,sof^giscontinuous.22.LethfnibeasequenceofcontinuousfunctionsfromatopologicalspaceXtoametricspaceY.Supposehfniconvergesuniformlytoafunctionf.Let">0andletx2X.ThereexistsNsuchthat(fn(x);f(x))<"=3forallnNandallx2X.SincefNiscontinuous,thereisanopensetOcontainingxsuchthatfN[O]BfN(x);"=3.Ify2O,then(f(y);f(x))(f(y);fN(y))+(fN(y);fN(x))+(fN(x);f(x))<".Thusf[O]Bf(x);".SinceanyopensetinYisaunionofopenballs,khdaw.comfiscontinuous.23a.LetXbeaHausdor
space.SupposeXisnormal.GivenaclosedsetFandanopensetOcontainingF,thereexistdisjointopensetsUandVsuchthatFUandOcV.NowUisdisjointfromOcsinceify2Oc,thenVisanopensetcontainingythatisdisjointfromU.ThusUO.Conversely,supposethatgivenaclosedsetFandanopensetOcontainingF,thereisanopensetUsuchthatFUandUO.LetFandGbedisjointclosedsets.ThenFGcandthereisanopensetUsuchthatFUandUGc.Equivalently,FUandGUc.SinceUandUcaredisjointopensets,Xisnormal.*23b.LetFbeaclosedsubsetofanormalspacecontainedinanopensetO.Arrangetherationalsin(0;1)oftheformr=p2�ninasequencehri.LetU=O.Bypart(a),thereexistsanopensetn1U0suchthatFU0andU0O=U1.LetPnbethesetcontainingthe
rstntermsofthesequence.SinceU0U1,thereexistsanopensetUr1suchthatU0Ur1andUr1U1.SupposeopensetsUrhavebeende
nedforrationalsrinPnsuchthatUpUqwheneverpr1sof(x)r1.Sincef(x)>r1,x=2Ur1.Thusx2U.Ify2U,theny2Ur2sof(y)r2c.Thusf[U](c;d).Hencefiscontinuous.23d.LetXbeaHausdor
space.SupposeXisnormal.ForanypairofdisjointclosedsetsAandBonX,BcisanopensetcontainingA.Bytheconstructionsinparts(b)and(c),thereisacontinuousreal-valuedfunctionfonXsuchthat0f1,f0onAandf1on(Bc)c=B.(*)ProofofUrysohn"sLemma.24a.LetAbeaclosedsubsetofanormaltopologicalspaceXandletfbeacontinuousreal-valuedfunctiononA.Leth=f=(1+jfj).Thenjhj=jfj=(1+jfj)<1.24b.LetB=fx:h(x)�1=3gandletC=fx:h(x)1=3g.ByUrysohn"sLemma,thereisacontinuousfunctionh1whichis�1=3onBand1=3onCwhilejh1(x)j1=3forallx2X.Thenjh(x)�h1(x)j2=3forallx2A.24c.SupposewehavecontinuousfunctionshonXsuchthatjh(x)j<2n�1=3nforallx2XandPnnPjh(x)�nh(x)j<2n=3nforallx2A.LetB0=fx:h(x)�nh(x)�2n=3n+1gandi=1iPi=1iletC0=fx:h(x)�nh(x)2n=3n+1g.ByUrysohn"sLemma,thereisacontinuousfunctioni=1ihwhichis�2n=3n+1onB0and2n=3n+1onC0whilejh(x)j2n=3n+1forallx2X.Thenn+1n+1khdaw.com45若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
Pn+1n+1n+1jh(x)�i=1hi(x)j<2=3forallx2A.Pnnn24d.Letkn=i=1hi.Theneachkniscontinuousandjh(x)�kn(x)j<2=3forallPx2A.Also,jk(x)�k(x)j<2n�1=3nforallnandallx2X.Ifm>n,thenjk(x)�k(x)j=jmh(x)jPnn�1Pmni=n+1im2i�1=3i<(2=3)n.Letk(x)=1h(x).Thenjk(x)�k(x)j(2=3)nforallx2X.Thusi=n+1i=1inhkniconvergesuniformlytok.i.e.hhniisuniformlysummabletokandsinceeachkniscontinuous,kiscontinuous.Also,sincejkj1foreachn,jkj1.Nowjh(x)�k(x)j<2n=3nforallnandallx2Annsolettingn!1,jh(x)�k(x)j=0forallx2A.i.e.k=honA.24e.Sincejkj=jhj<1onA,Aandfx:k(x)=1garedisjointclosedsets.ByUrysohn"sLemma,thereisacontinuousfunction"onXwhichis1onAand0onfx:k(x)=1g.24f.Setg="k=(1�j"kj).Thengiscontinuousandg=k=(1�jkj)=h=(1�jhj)=fonA.(*)ProofofTietze"sExtensionTheorem.25.LetFbeafamilyofreal-valuedfunctionsonasetX.Considerthesetsoftheformfx:jfi(x)�fi(y)j<"forsome">0;somey2X;andsome
nitesetf1;:::;fnoffunctionsinFg.TheweaktopologyonXgeneratedbyFhasff�1[O]:f2F;OopeninRgasabase.Nowifx02f�1[O]forsomef2FandOopeninR,wemayassumeOisanopeninterval(c;d).Ifx2fx:jf(x)�f(x0)jii0;somey2X;andsome
nitesetf1;:::;fnoffunctionsinFgisabasefortheweaktopologyonXgeneratedbyF.SupposethistopologyisHausdor
.Foranypairfx;ygofdistinctpointsinX,therearedisjointopensetsOxandOysuchthatx2Oxandy2Oy.ThentherearesetsBxandByoftheformfx:jfi(x)�fi(z)j<"forsome">0;somez2X;andsome
nitesetf1;:::;fnoffunctionsinFgsuchthatx2BxOxandy2ByOy.Supposef(x)=f(y)forallf2F.Thenx;y2BxBy.Contradiction.Hencethereisafunctionf2Fsuchthatf(x)6=f(y).Conversely,supposethatforeachpairfx;ygofdistinctpointsinXthereexistsf2Fsuchthatf(x)6=f(y).Thenx2fx0:jf(x0)�f(x)j0sinceOisopen.Thereexistsz>d�"suchthat(a;z)O.Then(a;d+")=(a;z)[(d�";d+")O.Contradiction.Ifd=2O,then(d�";d+")Ocforsome">0sinceOcisopen.Butthereexistsz>d�"suchthat(d�";z)(a;z)O.Contradiction.Henced=b.Thusforanyccsuchthat(a;c0)O.i.e.c2O.HenceO=IandIisconnected.ItfollowsfromQ33thatintervalsoftheform(a;b],[a;b),[a;b]arealsoconnected.35a.LetXbeanarcwiseconnectedspace.SupposeO1andO2isaseparationofX.Takex2O1andy2O2.Thereisacontinuousfunctionf:[0;1]!Xwithf(0)=xandf(1)=y.Since[0;1]isconnected,f[0;1]isconnectedsowemayassumef[0;1]O1.Theny2O1O2.Contradiction.HenceXisconnected.*35b.ConsiderX=fhx;yi:x=0;�1y1g[fhx;yi:y=sin(1=x);00,choosensuchthat1=2n<".Sincesin1(4n+1)=1andsin课后答案网1(4n+3)=�1,sin(1=x)takesoneveryvaluebetween-1and1in22theinterval[2=(4n+3);2=(4n+1)].Inparticular,sin(1=x0)=yforsomex0intheinterval.Then[h0;yi;hx0;yi]=x0<"sohx0;yi2Bh0;yi;"S.HenceISandbyQ33,X=I[Sisconnected.SupposeXisarcwiseconnected.Thenthereisapathffromh0;0itosomepointofS.Thesetoftsuchthatf(t)2Iisclosedsoithasalargestelement.Wemayassumethatt=0.Letf(t)=hx(t);y(t)i.Thenx(0)=0whilex(t)>0andy(t)=sin(1=x(t))fort>0.Foreachn,chooseuwith00suchthatBx;G.Fory2Bx;,f(t)=(1�t)x+tyisa0pathconnectingxandy.ThusH6=;.Foreachy2H,thereexists>0suchthatBy;0G.Thereisapathfconnectingytox.Foreachz2By;0,thereisapathgconnectingztoy.Thenhgivenbyh(t)=g(2t)fort2[0;1=2]andh(t)=f(2t�1)fort2[1=2;1]isapathconnectingztox.ThusH,theny2BBy;0HandHisopen.Ify2y;Gforsome>0andBy;containsapointzofH.Nowthereisapathconnectingztoxandthereisapathconnectingytoz.Thusthereisapathconnectingytoxsoy2HandHisclosed.HenceH=G.Sincexisarbitrary,Gisarcwiseconnected.36.LetXbealocallyconnectedspaceandletCbeacomponentofX.Ifx2C,thenthereisaconnectedbasicsetBsuchthatx2B.SinceBisconnected,BC.ThusCisopen.*37.LetXbeasinQ35b.Sucientlysmallballscentredath0;0idonotcontainconnectedopensetssoXisnotlocallyconnected.47khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
8.5ProductsanddirectunionsoftopologicalspacesS38a.LetZ=X.Supposef:Z!Yiscontinuous.ForanyopensetOY,f�1[O]isopeninZsof�1[O]XisopeninXforeach.i.e.fj�1[O]isopeninXforeach.ThuseachrestrictionXfjXiscontinuous.Conversely,supposeeachrestrictionSfjXiscontinuous.ForanyopensetOY,f�1[O]=fj�1[O].Eachofthesetsfj�1[O]isopensof�1[O]isopenandfiscontinuous.XX38b.FZisclosedifandonlyifFcisopenifandonlyifFcXisopeninXforeachifandonlyifFXisclosedinXforeach.38c.SupposeZisHausdor
.Givenx;y2X,x;y2ZsotherearedisjointopensetsO1;O2Zsuchthatx2O1andy2O2.ThenO1XandO2XaredisjointopensetsinXcontainingxandyrespectively.ThusXisHausdor
.Conversely,supposeeachXisHausdor
.Givenx;y2Z,x2Xandy2Xforsomeand.If=,thensinceXisHausdor
,therearedisjointopensetsO1;O2Xsuchthatx2O1andy2O2.ThesetsO1andO2arealsoopeninZsoitfollowsthatZisHausdor
.If6=,thensinceXX=;andthesetsXandXareopeninZ,itfollowsthatZisHausdor
.38d.khdaw.comSupposeZisnormal.GivendisjointclosedsetsF1;F2X,F1andF2areclosedinZsotherearedisjointopensetsO1;O2ZsuchthatF1O1andF2O2.ThenF1O1XandF2O2XwithO1XandO2XbeingdisjointopensetsinX.ThusXisnormal.Conversely,supposeeachXisnormal.GivendisjointclosedsetsF1;F2Z,thesetsF1XandF2XareclosedinXforeachsotherearedisjointopensetsSSO1;
S;O2;
XSsuchthatF1XO1;
andF2XO2;
.ThenF1O1;
andF2O2;
withO1;
andO2;
beingdisjointopensetsinZ.HenceZisnormal.39a.LetX1Xbeadirectsummand.Thenitfollowsfromthede
nitionthatX1isopen.LetX2beanotherdirectsummand.ThenXisopenandXXc.NowXcisclosedsoX=XcXisclosed.212212139b.LetX1beasubsetofXthatisbothopenandclosed.LetX2=XnX1.ThenX2isopen,X1X2=;andX=X1[X2.IfOisopeninX,thenOXiisopeninXifori=1;2.Ontheotherhand,ifOisopeninX1[X2,thenOXiisopeninXifori=1;2andthusopeninX.ThenO=(OX1)[(OX2)isopeninX.HenceX=X1[X2.*40a.IfXhasabase,eachelementbeingTychono
,letx;ybedistinctpointsinX.ThereisabasicelementBcontainingx.Since课后答案网BisTychono
,thereexistsOopeninBsuchthatx2Obuty=2O.ThenOisalsoopeninXsoXisTychono
.ConsiderE=Rf0;1g=whereisthesmallestequivalencerelationwithhx;0ihx;1iforx2Rnf0g.Letq:Rf0;1g!Ebethequotientmapandconsiderthebaseq[(a;b)feg]fora0sox2U.ThusWF[U].ThusF[U]isopeninIF.HenceFisahomeomorphism.*48b.SupposeXisanormalspacesatisfyingthesecondaxiomofcountability.LetfBngbeacountablebaseforX.Foreachpairofindicesn;msuchthatBnBm,byUrysohn"sLemma,thereexistsacontinuousfunctiongonXsuchthatg1onBandg0onBc.GivenaclosedsetFn;mn;mnn;mmandx=2F,chooseabasicelementBsuchthatx2BFc.ByQ23a,thereexistsBsuchthatmmnx2BnandBnBm.Thenwww.hackshp.cngn;misde
nedwithgn;m(x)=1andgn;m[F]=0.Furthermorethefamilyfgn;mgiscountable.48c.SupposeXisanormalspacesatisfyingthesecondaxiomofcountability.Bypart(b),thereisacountablefamilyFofcontinuousfunctionswiththepropertyinpart(a).ThenthereisahomeomorphismbetweenXandIF.ByQ45,IFismetrizableandthusXismetrizable.*49.Firstweconsider
niteproductsofconnectedspaces.SupposeXandYareconnectedandchooseha;bi2XY.ThesubspacesXfbgandfxgYareconnected,beinghomeomorphictoXandYSrespectively.ThusTx=(Xfbg)[(fxgY)isconnectedforeachx2X.Thenx2XTxistheunionofacollectionofconnectedspaceshavingthepointha;biincommonsoitisconnected.ButthisunionisXYsoXYisconnected.Theresultforany
niteproductfollowsbyinduction.QLetfXg2AbeacollectionofconnectedspacesandletX=X.Fixapointa2X.Forany
nitesubsetKA,letXKbethesubspaceconsistingofpointsQxsuchthatx=afor
=2K.ThenXKishomeomorphictothe
niteproduct2KXsoitisconnected.LetYbetheunionofthesetsQXK.Sinceanytwoofthemhaveapointincommon,byQ32,Yisconnected.Letx2XandletU=Obeabasicelementcontainingx.NowO=Xforallbut
nitelymanysoletKbethat
niteset.Letybetheelementwithy=xfor2Kandy=afor
=2K.Theny2XKYandy2U.HenceX=YsoXisconnected.khdaw.com49若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
8.6Topologicalanduniformproperties50a.LethfnibeasequenceofcontinuousmapsfromatopologicalspaceXtoametricspace(Y;)thatconvergesuniformlytoamapf.Given">0,thereexistsNsuchthat(fn(x);f(x))<"=3fornNandx2X.Givenx2X,thereisanopensetOcontainingxsuchthat(fN(x);fN(y))<"=3fory2O.Then(f(x);f(y))(f(x);fN(x))+(fN(x);fN(y))+(fN(y);f(y))<"fory2O.Hencefiscontinuous.50b.LethfnibeasequenceofcontinuousmapsfromatopologicalspaceXtoametricspace(Y;)thatisuniformlyCauchy.SupposeYiscomplete.Foreachx2X,thesequencehfn(x)iisCauchysoitconvergessinceYiscomplete.Letf(x)bethelimitofthesequence.Thenhfniconvergestof.Given">0,thereexistsNsuchthat(fn(x);fm(x))<"=2forn;mNandx2X.Foreachx2X,thereexistsNxNsuchthat(fNx(x);f(x))<"=2.ThenfornNandx2X,wehave(fn(x);f(x))(fn(x);fNx(x))+(fNx(x);f(x))<".Thushfniconvergesuniformlytofandbypart(a),fiscontinuous.51.TheproofsofLemmas7.37-39remainvalidwhenXisaseparabletopologicalspace.ThustheAscoli-ArzelaTheoremanditscorollaryarestilltrue.khdaw.com8.7Nets52.SupposeXisHausdor
andsupposeanethxiinXhastwolimitsx;y.ThentherearedisjointopensetsUandVsuchthatx2Uandy2V.Sincexandyarelimits,thereexist0and1suchthatx2Ufor andx2Vfor .Choose0suchthat0 and0 .Then0101x2UVfor 0.Contradiction.HenceeverynetinXhasatmostonelimit.Conversely,supposeXisnotHausdor
.Letx;ybetwopointsthatcannotbeseparatedandletthedirectedsystembethecollectionofallpairshA;Biofopensetswithx2A,y2B.ChoosexhA;Bi2AB.LetObeanopensetcontainingxandletO0beanopensetcontainingy.ForhA;Bi hO;O0i,wehaveAOandBO0sox2ABOO0.Thusbothxandyarelimits.hA;Bi53.Supposefiscontinuous.Lethxibeanetthatconvergestox.ForanyopensetOcontainingf(x),wehavex2f�1[O],whichisopen.Thereexistssuchthatx2f�1[O]for .Thenf(x)2O00for 0.Hencehf(x)iconvergestof(x).Conversely,supposethatforeachnethxiconvergingtoxthenethf(x)iconvergestof(x).Theninparticularthestatementholdsforallsequences.Itfollowsthatfiscontinuous.课后答案网*54.LetXbeanysetandfareal-valuedfunctiononX.LetAbethesystemconsistingofall
nitePsubsetsofX,withF
GmeaningFG.ForeachPF2A,letyF=x2Ff(x).Supposethatf(x)=0PexceptforxinacountablesubsetPfxngandjf(xn)j<1.Given">0,thereexistsNsuchthat1n=N+1jf(xn)j<"P.Lety=f(xn).Foranyopeninterval(y�";y+"),letF0=fx1;:::;xNg.For1F F0,jy�yFjn=N+1jf(xn)j<"soyF2(y�";y+").ThuslimyF=y.Conversely,iff(x)6=0onanuncountablesetG,thenforsomewww.hackshp.cnn,jf(x)j>1=nforuncountablymanyx.Thusbyconsideringarbitrarilylarge
nitesubsetsofG,weseethathyFidoesnotconverge.Hencef(x)=0exceptonaPPcountableset.NowifPjf(xn)j=1,thenweonlyhavef(xn)<1anditfollowsthatthelimitisnotunique.Hencejf(xn)j<1.Q55.LetX=X.SupposeanethxiinXconvergestox.Sinceeachprojectioniscontinuous,eachcoordinateofxconvergestothecorrespondingcoordinateofx.Conversely,supposeeachcoordinateofQxconvergestothecorrespondingcoordinateofx.LetObeabasicelementcontainingx.Then(x)2Oforeach.Foreach,thereexistssuchthat(x)2Ofor .Sinceallbut
nitelymanyoftheOareX,weonlyneedtoconsidera
niteset1;:::;
n.Inparticular,wemaychoose0suchthat0 iforallQi.For 0,wehavei(x)2Oi.For6=i,wealsohave(x)2O=X.Thusx2Ofor 0.Hencethenethxiconvergestox.50khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
9CompactandLocallyCompactSpaces9.1Compactspaces1.SupposeXiscompact.Theneveryopencoverhasa
nitesubcovering.Inparticular,ithasa
nitere
nement.Conversely,supposeeveryopencoverhasa
nitere
nement.Sinceeveryelementinthere
nementisasubsetofanelementintheopencover,theopencoverhasa
nitesubcoveringsoXiscompact.*2.LetThKnibeadecreasingsequenceofcompactsetswithK0Hausdor
.LetObeanopensetwithKnO.SupposeKnisnotasubsetofOforanyn.ThenKnnOisnonemptyandclosedinKn.SinceKnisacompactsubsetoftheHausdor
spaceK0,KnisclosedinK0soKnnOisclosedinTK0.Also,ThKnnOiisadecreasingsequencesoithasthe
niteintersectionproperty.NowT;6=(Kn)nO=(KnnO)KnO.Contradiction.HenceKnOforsomen.(*)AssumeK0isHausdor
.3.SupposeXisacompactHausdor
space.LetFbeaclosedsubsetandletx=2F.Foreachy2F,therearedisjointopensetsUyandVywithx2Uyandy2Vy.NowFiscompactandTfVy:y2SFgisannnopencoverforkhdaw.comF.Thusthereisa
nitesubcoveringfVy1;:::;Vyng.LetU=i=1UyiandV=i=1Vyi.ThenUandVaredisjointopensetswithx2UandFV.HenceXisregular.4.SupposeXisacompactHausdor
space.LetFandGbedisjointclosedsubsets.ByQ3,foreachy2G,therearedisjointopensetsUyandVysuchthatFUyandy2Vy.NowGiscompactandTfV:y2GgisanopencoverforG.Thusthereisa
nitesubcoveringfV;:::;Vg.LetU0=nUySy1yni=1yiandV0=nV.ThenU0andV0aredisjointopensetswithFU0andGV0.HenceXisnormal.i=1yi5a.If(X;T)isacompactspace,thenforT1weakerthanT,anyopencoverfromT1isanopencoverfromTsoithasa
nitesubcovering.Thus(X;T1)iscompact.5b.If(X;T)isaHausdor
space,thenforT2strongerthanT,anytwodistinctpointsinXcanbeseparatedbydisjointsetsinT,whicharealsosetsinT2.Thus(X;T2)isHausdor
.5c.Suppose(X;T)isacompactHausdor
space.IfT1isaweakertopology,thenid:(X;T)!(X;T1)isacontinuousbijection.If(X;T1)isHausdor
,thenidisahomeomorphism.Contradiction.Hence(X;T1)isnotHausdor
.IfT2isastrongertopology,thenid:(X;T2)!(X;T)isacontinuousbijection.If(X;T2)iscompact,thenidisahomeomorphism.Contradiction.Hence(X;T2)isnotcompact.6.LetXbeacompactspaceand课后答案网FanequicontinuousfamilyofmapsfromXtoametricspace(Y;).LethfnibeasequencefromFsuchthatfn(x)!f(x)forallx2X.Foreachx2X,given">0,thereexistsNxsuchthat(fn(x);f(x))<"=3fornNx.Also,thereexistsanopensetOxcontainingxsuchthat(fn(x);fn(y))<"=3fory2Oxandalln.Thenwealsohave(f(x);f(y))<"=3fory2Ox.NowfOx:x2XgisanopencoverforXsothereisa
nitesubcoveringfOx1;:::;Oxkg.LetN=max1ikNxi.FornN,(fn(xi);f(xi))<"=3for1ik.Foreachx2X,x2Oxiforsomeiso(fn(x);f(x))www.hackshp.cn(fn(x);fn(xi))+(fn(xi);f(xi))+(f(xi);f(x))<"fornN.HencehfniconvergesuniformlytofonX.*7.LetXbeaHausdor
spaceandhCniadecreasingsequenceofcompactandconnectedsets.LetTC=Cn.ForanyopencoverUofC,UisanopencoverofsomeCnbyQ2.Thusithasa
nitesubcovering,whichalsocoversC.HenceCiscompact.SupposeCisdisconnectedwithAandBbeingaseparationforC.ThenAandBarenonemptydisjointclosedsubsetsofC.SinceCisanintersectionofclosedsets,Cisclosed.ThusAandBareclosedinC0.SinceC0iscompactandHausdor
,itisnormal.ThustherearedisjointopensetsU;VC0suchthatAUandBV.ThenC=A[BU[V.ByQ2,CnU[Vforsomen.HenceCnisdisconnected.Contradiction.HenceCisconnected.(*)AssumeXisHausdor
8a.LethfnibeasequenceofmapsfromXtoYthatconvergeinthecompact-opentopologytof.Forx2X,letObeanopensetcontainingf(x).ThenNfxg;Oisopeninthecompact-opentopologyandcontainsf.ThereexistsN0suchthatf2NfornN0.i.e.f(x)2OfornN0.Hencenfxg;Onf(x)=limfn(x).*8b.LethfnibeasequenceofcontinuousmapsfromatopologicalspaceXtoametricspace(Y;).SupposehfniconvergestofuniformlyoneachcompactsubsetCofkhdaw.comX.LetNK;Obeasubbasicelement51若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
containingf.Thenf[K]isacompactsetdisjointfromOcso(f[K];Oc)>0.Let"=(f[K];Oc).ThereexistsN0suchthat(f(x);f(x))<"fornN0andx2K.Thenf(x)2OfornN0andnnx2K.i.e.f2NfornN0.Hencehficonvergestofinthecompact-opentopology.nK;OnConversely,supposehfniconvergestofinthecompact-opentopology.LetCbeacompactsubsetofXandlet">0begiven.SinceCiscompactandfiscontinuous,f[C]iscompact.Thusthereexistx1;:::;xn2CsuchthattheopenballsBf(x1);"=4;:::;Bf(xn);"=4coverf[C].Foreachi,let�1TnCi=Cf[Bf(xi);"=4]andOi=BTf(xi);"=4.ThenCiiscompactandf[Ci]Oi.Thusf2i=1NCi;Oi.ThereexistsN0suchthatf2nNfornN0.Foranyx2C,x2Cforsomeisoni=1Ci;Oii(f(x);f(x))<"=4.Also,f(x)2OfornN0so(f(x);f(x))<"=4fornN0.Thusinini(f(x);f(x))<"fornN0andx2C.HencehficonvergesuniformlytofonC.nn(*)Assumeftobecontinuous.9.2CountablecompactnessandtheBolzano-Weierstrassproperty9a.Areal-valuedfunctionfonXiscontinuousifandonlyiffx:f(x)<
gandfx:f(x)>
gareopenforanyrealnumberkhdaw.comifandonlyiffisbothuppersemicontinuousandlowersemicontinuous.9b.Supposefandgareuppersemicontinuous.Thenfx:f(x)<
gandfx:g(x)<
gareopenforSanyrealnumber.Nowforanyrealnumber,fx:f(x)+g(x)<
g=[fx:g(x)0,thereexistsNsuchthatjfN(x)�f(x)j<"=3forallx2X.Sincefx:fN(x)0suchthatjx�yj<impliesfN(x)�fN(y)<"=3.Nowifjx�yj<,thenf(x)�f(y)=[f(x)�fN(x)]+[fN(x)�fN(y)]+[fN(y)�f(y)]<".Given2R,picky2fx:f(x)<
g.Thereexists>0suchthatjx�yj<impliesf(x)�f(y)<
�f(y).i.e.f(x)<
.Hencefx:f(x)<
gisopenandfisuppersemicontinuous.*10(i))(iii)byProposition9.Supposethateveryboundedcontinuousreal-valuedfunctiononXassumesitsmaximum.Letfbeacontinuousfunctionandsupposeitisunbounded.Wemayassume,bytakingmax(1;f),thatfwww.hackshp.cn1.Then�1=fisaboundedcontinuousfunctionwithnomaximum.Thus(iii))(ii).SupposeXisnocountablycompact.ThenitdoesnothavetheBolzano-Weierstrassproperty.ThereisasequencehxniinXwithnoclusterpoint.Thusthesequencehasnolimitpoints.LetA=fxng.ThenAisclosedandsinceallsubsetsofAarealsoclosed,Aisdiscrete.De
nef(xn)=nforxn2A.ThenfiscontinuousontheclosedsetAandsinceXisnormal,byTietze"sExtensionTheorem,thereisacontinuousfunctiongonXsuchthatgjA=f.ThengisanunboundedcontinuousfunctiononX.Thus(ii))(i).11a.LetXbethesetofordinalslessthanthe
rstuncountableordinalandletBbethecollectionofsetsoftheformfx:xxn.Ifnosuchsequenceexists,wemayconstructforsomekhdaw.com52若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
Nanincreasingsequencehznisuchthatjf(zn)�f(zn�1)j1=Nforeachn.Thenhzniconvergestoitssupremumbuthf(zn)idoesnotconverge,contradictingthecontinuityoff.Nowletx0=supxn.Thenf(x)=f(x0)forxx0.12a.SimilarargumentasQ11a.*12b.LetUbeanopencoverforYconsistingofbasicsets.LetLa=fx:xb0suchthatLa12U.Sincey>b0oryang.Thereexistsaanforalln.Thentheopensetfx:x>agdoesnotcontainanyUn.Contradiction.ThusYisnot
rstcountable.9.3khdaw.comProductsofcompactspaces13.EachclosedandboundedsubsetXofRniscontainedinacubeInwhereI=[a;b].EachIiscompactinRsoIniscompactinRnbyTychono
"sTheorem.NowXisclosedinInsoXiscompact.*14.SupposeXiscompactandIisaclosedinterval.LetUbeanopencoverofXIandlett2I.SinceXftgishomeomorphictoSX,Xftgiscompactsothereisa
nitesubcoveringfU1;:::;UngnofUsuchthatU=i=1UiXftg.NowXftgcanbecoveredby
nitelymanybasicsetsA1B1;:::;AkBkU.ThenB=B1BkisanopensetcontainingTt.Ifhx;yi2XB,nthenhx;tSi2AjBjforsomejsox2Ajandy2i=1BiBj.Thushx;yi2AjBjandnXBi=1(AiBi)U.Thusforeacht2I,thereisanopensetBtcontainingtsuchthatXBtcanbecoveredby
nitelymanyelementsofU.ThecollectionofthesetsBtformsanopencoverofXsoSmthereisa
nitesubcollectionfBt1;:::;BtmgcoveringX.NowXI=i=1(BtiI),whichcanthenbecoveredby
nitelymanyelementsofU.Q15.LethXnibeacountablecollectionofsequentiallycompactspacesandletX=Xn.Givena(1)sequencehxniinX,thereisasubsequencehxniwhose
rstcoordinateconverges.Thenthereisa(2)(1)(n)subsequencehxniofhxniwhosesecondcoordinateconverges.Considerthediagonalsequencehxni.Eachcoordinateofthissequenceconvergessothesequenceconvergesin课后答案网X.HenceXissequentiallycompact.16.LetXbeacompactHausdor
space.LetFbethefamilyofcontinuousreal-valuedfunctionsonQXwithvaluesin[0;1].LetQ=f2FIf.ConsiderthemappinggofXintoQmappingxtothepointwhosef-thcoordinateisf(x).Ifx6=y,thensinceXiscompactHausdor
,andthusnormal,byUrysohn"sLemma,thereexistsf2Fsuchthatf(x)=0andf(y)=1.Thusgisone-to-one.Sincefiscontinuousforeachf2www.hackshp.cnF,giscontinuous.Nowg[X]isacompactsubsetoftheHausdor
spaceQsog[X]isclosedinQ.Furthermore,gisacontinuousbijectionfromthecompactspaceXontotheHausdor
spaceg[X]soXishomeomorphictog[X].*17.LetQ=IAbeacubeandletfbeacontinuousreal-valuedfunctiononQ.Given">0,coverf[Q]by
nitelymanyopenintervalsI;:::;Ioflength".Considerf�1[I]forj=1;:::;n.Thesesets1nj�1Q(j)(j)coverQandwemayassumeeachofthemisabasicset,thatis,f[Ij]=UwhereUisopenin(j)(j)SnIandallbut
nitelymanyoftheUareI.Foreachj,letFj=f:U6=IgandletF=j=1Fj,whichisa
niteset.De
neh:Q!Qbyh(x)=xfor2Fand0otherwise.De
neg=fh.ThengdependsonlyonFandjf�gj<".9.4Locallycompactspaces18.LetXbealocallycompactspaceandKacompactsubsetofX.Foreachx2K,thereisanopensetOxcontainingxwithSOxcompact.SinceKiscompact,thereisa
nitesubcollectionSfOx1;:::;OxngnnthatcoversK.LetO=i=1Oxi.ThenOKandO=i=1Oxiiscompact.*19a.LetXbealocallycompactHausdor
spaceandKacompactsubset.ThenKisclosedin53khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
X.ByQ8.23a,thereexistsaclosedsetDcontaining!withDK=;.ByUrysohn"sLemma,thereisacontinuousfunctiongonXwith0g1thatis1onKand0onD.De
nef(x)=min(2(g(x)�1=2);0).Thenfx:f(x)>0g=fx:g(x)>1=2g,whichiscompactbecausegiscontinuousonX.(*)Alternatively,useQ16toregardKasaclosedsubsetofthecompactHausdor
spaceQ.*19b.LetKbeacompactsubsetofalocallycompactHausdor
spaceX.ThenbyQ18,thereisanopensetOKwithOcompact.NowXnOandKaredisjointclosedsubsetsofXsobyUrysohn"sLemma,thereisacontinuousfunctiongonXwith0g1thatis1onKand0onXnO.Thenf=gjXistherequiredfunction.20a.LetXbetheAlexandro
one-pointcompacti
cationofalocallycompactHausdor
spaceX.ConsiderthecollectionofopensetsofXandcomplementsofcompactsubsetsofX.Then;andXareinthecollection.IfU1andU2areopeninX,thensoisU1U2.IfK1andK2arecompactsubsetsofX,then(XnK)(XnK)=Xn(K[K)whereK[Kiscompact.Also,U(XnK)=12121211U1(XnK1),whichisopeninX.Thusthecollectionofsetsisclosedunder
niteintersection.IfSfUgisacollectionofopensetsinSX,thenTUisopeninX.IffKgisacollectionofcompactsubsetsofX,then(XnK)=XnK.SinceeachKiscompactintheHausdor
spaceX,eachKkhdaw.comTTTisclosedinSSXandKisclosedinX.ThusKisclosedineachSKsoKiscompact.Finally,TU[(XnK)=U[(XnK)=Xn(KnU),whereU=UandK=K.SinceKnUisclosedinthecompactsetK,KnUiscompact.Thusthecollectionofsetsisclosedunderarbitraryunion.HencethecollectionofsetsformsatopologyforX.20b.LetidbetheidentitymappingfromXtoXnf!g.Clearlyidisabijection.IfUisopeninXnf!g,thenU=(Xnf!g)U0forsomeU0openinX.IfU0isopeninX,thenU=U0soUisopeninX.IfU0=XnKforsomecompactKX,thenU=XnK,whichisopeninX.Thusidiscontinuous.Also,anyopensetinXisopeninXsoidisanopenmapping.Henceidisahomeomorphism.20c.LetUbeanopencoverofX.ThenUcontainsasetoftheformXnKforsomecompactKX.TaketheotherelementsofUandintersecteachofthemwithXtogetanopencoverofK.Thenthereisa
nitesubcollectionthatcoversK.Thecorresponding
nitesubcollectionofUtogetherwithXnKthencoversX.HenceXiscompact.Letx;ybedistinctpointsinX.Ifx;y2X,thentherearedisjointopensetsinX,andthusinX,thatseparatexandy.If课后答案网x2Xandy=!,thenthereisanopensetOcontainingxwithOcompact.ThesetsOandXnOaredisjointopensetsinXseparatingxandy.HenceXisHausdor
.*21a.LetSndenotetheunitsphereinRn+1.Letp=h0;:::;0;1i2Rn+1.De
nef:Sn�p!Rnbyf(x)=1hx;:::;xi.Themapg:Rn!Sn�pde
nedbyg(y)=ht(y)y;:::;t(y)y;1�t(y)i1�xn+11n1nwheret(y)=2=(1+jjyjj2)istheinverseoff.ThusRnishomeomorphictoSn�pandtheAlexandro
one-pointcompacti
cationofRnishomeomorphictotheAlexandro
one-pointcompacti
cationofSn�p,whichisSn.www.hackshp.cn21b.LetXbethespaceinQ11andYbethespaceinQ12.De
nef:X!Ybyf(x)=xforx2Xandf(!)=!.Thenfisclearlyabijection.ConsiderthebasicsetsinY.Nowf�1[fx:x0onK,supportfO,f+g>0onOandg0onK.Thusf=(f+g)2Fiscontinuousand1onK.Thefunctions"i=fi=(f+g)2F;i=1;:::;nforma
nitecollectionoffunctionssubordinatetothecollectionfOgandsuchthat"1+"n1onK.*26.Lemma:LetXbealocallycompactHausdor
spaceandUbeanopensetcontainingx2X.ThenthereisanopensetVcontainingxsuchthatViscompactandVU.Proof:ThereisanopensetOXcontainingxsuchthatOiscompact.ThenUOisopeninOandcontainsx.ThusthereisanopensetO0Osuchthatx2O0andO0OUO.NotethatO0OO0=O0.ThusO0UO.LetV=OO0.Thenx2V.Furthermore,sinceO0isopeninO=O,itisopeninOandthusopenin课后答案网XsoVisopeninX.Also,VO0UOU.SinceVisclosedinO,Viscompact.LetXbealocallycompactHausdor
spaceandfOngacountablecollectionofdenseopensets.GivenanopensetU,letx1beapointinO1U.LetV1beanopensetcontainingx1suchthatV1iscompactandV1O1U.Supposex1;:::;xnandV1;:::;Vnhavebeenchosen.Letxn+12On+1VnandletVn+1beanopensetcontainingxn+1suchthatVn+1iscompactandVn+1On+1Vn.ThenV1V2isadecreasingsequenceofclosedsetsinthecompactsetV1.Thiscollectionofclosedsetshasthe
niteTwww.hackshp.cnTTTintersectionpropertysoVn6=;.Lety2Vn.Theny2OnU.HenceOnisdenseinX.(*)AssumethatXisHausdor
.*27.LetXbealocallycompactHausdor
spaceandletObeanopensubsetcontainedinacountableSunionFnofclosedsets.NotethatOisalocallycompactHausdor
space(seeQ29b).Also,O=SS(OF),whichisaunionofsetsclosedinO.If(OF)=;,then(OF)=;foralln(withnnTntheinteriortakeninTOS)soOnFnisdenseandopeninOSforalln.ByQ26,(OnSFn)isdenseinSO.But(OnF)=On(F)c=;.Contradiction.Hence(OF)6=;.NowOF(OF)SnSnnnnsoOF6=;andFisanopensetdenseinO.nn(*)AssumethatXisHausdor
.*28.LetYbeadensesubsetofaHausdor
spaceX,andsupposethatYwithitssubspacetopologyislocallycompact.Giveny2Y,thereisanopensetUYcontainingywithUY=YUcompact.SinceXisHausdor
,YUisclosed.ThensinceUYU,wehaveUYUY.NowU=VYforsomeopensetVX.NotethatsinceYisdense,V=VY.Thenx2VandVV=VY=UY.HenceYisopeninX.29a.SupposeFisclosedinalocallycompactspaceX.Givenx2F,thereisanopensetOX55khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
containingxwithOcompact.ThenOFisanopensetinFcontainingyandOFF=FOFisclosedinOandthuscompact.HenceFislocallycompact.*29b.SupposeOisopeninalocallycompactHausdor
spaceX.Takex2O.BythelemmainQ26,thereisanopensetUcontainingxsuchthatUiscompactandUO.NowUOisanopensetinOcontainingxwithUOO=OUO=UObeingcompactsinceitisclosedinthecompactsetU.HenceOislocallycompact.29c.SupposeasubsetYofalocallycompactHausdor
spaceXislocallycompactinitssubspacetopology.ThenYisdenseintheHausdor
spaceY.ByQ28,YisopeninY.Conversely,supposeYisopeninY.Bypart(a),YislocallycompactsinceYisclosedinX.Bypart(b),YislocallycompactsinceYisopeninY.9.5-compactspaces30.LetXbealocallycompactHausdor
space.SupposethereisasequencehOniofopensetswithSOncompact,OnOn+1andX=On.Foreachn,let"nbeacontinuousreal-valuedfunctionwithP"nkhdaw.com1onOn�1andsupport"nOn.De
ne":X![0;1)by"=(1�"n).Giveny2Xand">0,y2OforsomeNandthusy2OfornN.ThereexistsN0suchthatNnPN0000"(y)�n=1(1�"n(y))<"=2.LetN=max(N;N).Takex2ON00.Then"N00+1(x)=1.Infact,PN00"(x)=1fornN00+1so"(x)=(1�"(x)).Foreachn=1;:::;N00,thereisanopensetUnn=1nnTN0000containingysuchthatj"n(y)�"n(x)j<"=2Nforx2Un.LetU=n=1UnON00.ThenUisanopenPN00PN00setcontainingyandforx2U,j"(y)�"(x)j["(y)�n=1(1�"n(y))]+jn=1(1�"n(y))�"(x)jPN00PN00["(y)�n=1(1�"n(y))]+n=1j"n(y)�"n(x)j<".Hence"iscontinuous.Toshowthat"isproper,weconsiderclosedboundedintervalsin[0;1).ByconsideringON00�1andopensetsVnwithVncompactandVnUn,wethenapplyasimilarargumentasabove.31a.Let(X;)beaproperlocallycompactmetricspace.IfKisacompactsubset,thenKisclosedandboundedbyProposition7.22.Conversely,supposeasubsetKisclosedandbounded.SinceXisproper,theclosedballsfx:(x;x0)agarecompactforsomex0andalla2(0;1).SinceKisbounded,thereexistx1andbsuchthat(x;x1)bforallx2K.Then(x;x0)b+(x1;x0)forallx2K.ThusKisaclosedsubsetofthecompactsetfx:(x;x0)b+(x1;x0)gsoKiscompact.31b.Let(X;)beaproperlocallycompactmetricspace.Thentheclosedballs课后答案网fx:(x;x0)agarecompactforsomex0andallx2(0;1).NotethatacompactsubsetK[0;1)isclosedandbounded.Also,thefunctionf(x)=(x;x)iscontinuousfromXto[0;1).Nowf�1[K]isboundedsinceKis0boundedandclosedsincefiscontinuousandKisclosed.Thusbypart(a),f�1[K]iscompact.Hencef:X![0;1)isapropercontinuousmapandXis-compact.31c.Let(X;)bea-compactandlocallycompactmetricspace.Thenthereisapropercontinuousmap":X![0;1).De
newww.hackshp.cn(x;y)=(x;y)+j"(x)�"(y)j.ThenisametriconX.Givenx2Xand">0,thereexists0>0suchthat(x;y)<0impliesj"(x)�"(y)j<"=2.Choose0,thereisa
niteFourierseries"suchthatj"(x)�f(x)j<"forallx.*43.LetAbeanalgebraofcontinuousreal-valuedfunctionsonacompactspaceX,andassumethatAseparatesthepointsofX.Ifforeachx2Xthereisanfx2Awithfx(x)6=0,thenbycontinuity,thereisanopenneighbourhoodOxofxsuchthatfx(y)6=0fory2Ox.ThesetsfOxgcoverXsobycompactness,
nitelymanyofthemcover课后答案网X,sayfO;:::;Og.Letg=f2++f2.Theng2Ax1xnx1xnandg6=0everywhere.TheclosureoftherangeofgisacompactsetKnotcontaining0.Thefunctionhgivenbyh(t)=1=tfort2Kandh(0)=0iscontinuousonK[f0gsoitcanbeuniformlyapproximatedbypolynomialshnsothathng2Aandhng!1=g.Notethatifhnisuniformlywithin"=2ofh,thenjhn(0)j<"=2buthn(0)istheconstanttermofhnsosubtractingtheconstanttermresultsinapolynomialstillwithin"ofh.Thuswemayassumethatthepolynomialshnhavenoconstantterm.Thus1=g2Aso12Aandwww.hackshp.cnAcontainstheconstantfunctions.HenceA=C(X).44.LetFbeafamilyofcontinuousreal-valuedfunctionsonacompactHausdor
spaceX,andsupposethatFseparatesthepointsofX.LetAbethesetofpolynomialsina
nitenumberoffunctionsofF.ThenAisasubalgebraofC(X)thatseparatesthepointsofXandcontainstheconstantfunctions.BytheStone-WeierstrassTheorem,AisdenseinC(X).Henceeverycontinuousreal-valuedfunctiononXcanbeuniformlyapproximatedbyapolynomialina
nitenumberoffunctionsofF.45a.LetXbeatopologicalspaceandAasetofreal-valuedfunctionsonX.De
nexyiff(x)=f(y)forallf2A.Clearly,xxforallx2Xandyxifxy.Ifxyandyz,thenf(x)=f(y)=f(z)forallf2Asoxz.Henceisanequivalencerelation.45b.LetXebethesetofequivalenceclassesofand"thenaturalmapofXintoXe.Givenf2A,de
nef~onXebyf~(~x)=f(x).If~x=~y,thenxysof(x)=f(y)andf~(~x)=f~(~y).Thusf~iswell-de
nedanditistheuniquefunctionsuchthatf=f~".45c.LetXehavetheweaktopologygeneratedbythefunctionsf~inpart(b).Considerabasicsetf~�1[O].Now"�1[f~�1[O]]=fx:f~("(x))2Og=fx:f(x)2Og=f�1[O],whichisopensincefiscontinuous.Hence"iscontinuous.45d.Since"iscontinuousandmapsXontoXe,ifXiscompact,thensoisXe.Byde
nitionofthe58khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
weaktopologyonXe,thefunctionsf~arecontinuous.*45e.LetXbeacompactspaceandAaclosedsubalgebraofC(X)containingtheconstantfunctions.De
neXeand"asabove.If~xand~yaredistinctpointsinXe,thenf(x)6=f(y)forsomef2AsotherearedisjointopensetsOandOinRwithf(x)2Oandf(y)2O.Then~x2f~�1[O]andxyxyxy~2f~�1[O].ThusXeisacompactHausdor
space.Furthermore,"inducesa(continuous)mappingyof":A!C(Xe);f7!f~,wheref=f~".TheimageofAinC(Xe)isasubalgebracontainingtheconstantfunctionsandseparatingthepointsofXe.Supposehg~iisasequencein"[A]thatconvergesnto~ginC(Xe).Thenthesequencehgni,wheregn=~gn",convergesto~g".Sinceeachgn2AandAisclosed,~g"2Aso~g2"[A]byuniqueness.Thus"[A]isclosed.BytheStone-WeierstrassTheorem,theimageofAisC(Xe).HenceAisthesetofallfunctionsoftheformf~"withf~2C(Xe).46.LetXandYbecompactspaces.Thesetof
nitesumsoffunctionsoftheformf(x)g(y)wheref2C(X)andg2C(Y)isanalgebraofcontinuousreal-valuedfunctionsonXYthatcontainstheconstantfunctionsandseparatespointsinXY.BytheStone-WeierstrassTheorem,thissetisdenseinC(XY).Thusforeachcontinuousreal-valuedfunctionfonXYandeach">0,thereexistPncontinuousfunctionsg1;:::;gnonXandh1;:::;hnonYsuchthatjf(x;y)�i=1gi(x)hi(y)j<"forallhx;ykhdaw.comi2XY.47.Thefunctionsofnorm1inthealgebraAgiveamappingofXintothein
nite-dimensionalcubeQfIf:f2A;jjfjj=1g.BytheTietzeExtensionTheorem,eachcontinuousfunctionfontheimageofXcanbeextendedtoacontinuousfunctiongonthecubeandbyQ17,gcanbeapproximatedbyacontinuousfunctionhofonlya
nitenumberofcoordinates.ThenhcanberegardedasacontinuousfunctiononacubeinRn,whichcanbeuniformlyapproximatedbyapolynomialin(a
nitenumberof)thecoordinatefunctions.48a.Let"bethepolynomialde
nedby"(x)=x+x(1�2x)(1�x).Then"0(x)=6x2�6x+2>0forallx.Thus"ismonotoneincreasingandits
xedpointsare0;1;1.2*48b.Choose">0.Notethat"(x)>xon(0;1)and"(x)0,itsucestoconsiderthecasewhereisarationalnumbera.De
ne"(x)=课后答案网x+x(a�bx)(1�x).Thenisa
xedpointof".Byparts(a)and(b),somebiterate="nisapolynomialwithintegralcoecients(andnoconstantterm)suchthat0(x)1in[0;1]andj(x)�j<"forallx2[";1�"].*48d.LetPbeapolynomialwithintegralcoecients,andsupposethatP(�1)=P(0)=P(1)=0.Letbeanyrealnumber.Wemayassumethat0<
<1.Forany">0,thereexists>0suchthatjP(x)j<"=2forx2(�;),x2(1�;1]andx2[�1;�1+).Wemayassumethat<"=jjPjj.Bypart(c),thereisapolynomialwww.hackshp.cnwithintegralcoecientsandnoconstanttermsuchthatj(x2)�j<forallx2[;1�].ThenjP(x)(x2)�
P(x)j<jjPjj<"forallx2[�1;1].*48e.LetI=[�1;1]andfacontinuousreal-valuedfunctiononIsuchthatf(�1);f(0);f(1)areintegersandf(1)f(�1)mod2.Letf(�1)=a;f(0)=b;f(1)=a+2c,wherea;b;careintegers.LetQ(x)=(a�b+c)x2+cx+b.Replacingfbyf�Q,wemayassumethata=b=c=0.ThenusetheStone-WeierstrassTheoremtoapproximatefbyapolynomialRwithrationalcoecientssuchthatR(�1)=R(0)=R(1)=0.LetNbetheleastcommonmultipleofthedenominatorsofthecoecientsofRsothatNRhasintegralcoecientsandvanishesat-1,0,1.Let=1=Nandapplypart(d)tothepolynomialNRsothatR,andthusf,canbeapproximatedbyapolynomialPwithintegralcoecients.*49a.*49b.*50a.*50b.59khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
10BanachSpaces10.1Introduction1.Supposexn!x.Thenjjjxnjj�jjxjjjjjxn�xjj!0.Hencejjxnjj!jjxjj.2.Themetric(x;y)=[Pn(x�y)2]1=2isderivedfromthenormjjxjj=(Pnx2)1=2.ThemetricPi=1iiPi=1i(x;y)=njx�yjisderivedfromthenormjjxjj=njxj.Themetric+(x;y)=maxjx�yji=1iii=1iiiisderivedfromthenormjjxjj+=maxjxj.Nown�1jjxjj+jjxjj+jjxjjnjjxjj+sojjxjjandjjxjj+pipareequivalent.Also,(n)�1jjxjj+jjxjj+jjxjj(n(jjxjj+)2)1=2=njjxjj+sojjxjjandjjxjj+areequivalent.Thusjjxjjandjjxjjarealsoequivalent.3.Consider+asafunctionfromXXintoX.Sincejj(x1+y1)�(x2+y2)jjjjx1�x2jj+jjy1�y2jj,+iscontinuous.ConsiderasafunctionfromRXintoX.Sincejjcx�cyjj=jcjjjx�yjj,iscontinuous.4.LetMbeanonemptyset.ThenMM+Msincem=m+forallm,whereisthezerovector.Also,MMsincem=1mforallm.IfMisalinearmanifold,thenM+MMandMMforeachsoM+M=MandM=M.Conversely,supposeM+M=MandM=M.Thenx2Mforeach2Randx2M.Thusalso1x1+2x22Mfor1;22Randx1;x22M.HenceMisalinearmanifold.khdaw.comT5a.LetfMi:i2IgbeafamilyoflinearmanifoldsandletM=Mi.Forany1;22Randx1;x22M,wehavex1;x22Miforalli.SinceeachMiisalinearmanifold,1x1+2x22Miforeachi.i.e.1x1+2x22M.HenceMisalinearmanifold.5b.GivenasetAinavectorspaceX,XisalinearmanifoldcontainingA.ConsiderthefamilyoflinearmanifoldscontainingA.TheintersectionfAgofthisfamilyisalinearmanifoldcontainingAanditisthesmallestsuchlinearmanifold.5c.ConsiderthesetMofall
nitelinearcombinationsoftheform1x1++nxnwithxi2A.ThenMisalinearmanifoldcontainingA.Also,anylinearmanifoldcontainingAwillcontainM.HenceMisthesmallestlinearmanifoldcontainingA.i.e.fAg=M.6a.LetMandNbelinearmanifolds.For1;22R,m1;m22Mandn1;n22N,1(m1+n1)+2(m2+n2)=(1m1+2m2)+(1n1+2n2)2M+N.HenceM+Nisalinearmanifold.NotethatMM+NandNM+NsoM+NcontainsM[N.Also,anymanifoldcontainingM[NwillalsocontainM+N.HenceM+N=fM[Ng.6b.LetMbealinearmanifold.For课后答案网1;22R,x1;x22Mand>0,thereexisty1;y22Msuchthatjjx1�y1jj<=21andjjx2�y2jj<=22(Wemayassume1;26=0).Thenjj(1x1+2x2)�(1y1+2y2)jjj1jjjx1�y1jj+j2jjjx2�y2jj<.Thus1x1+2x22MsoMisalinearmanifold.7.LetPbethesetofallpolynomialson[0;1].ThenPC[0;1].For1;22Randp1;p22P,1p1+2p2isstillapolynomialon[0;1]so1p1+2p22P.ThusPisalinearmanifoldinC[0;1].ThesetPisnotclosedinC[0;1]becausebytheWeierstrassApproximationTheorem,everycontinuousfunctionon[0;1]canbeuniformlyapproximatedbypolynomialson[0www.hackshp.cn;1].i.e.Pcontainsacontinuousfunctionthatisnotapolynomial.Thesetofcontinuousfunctionsfwithf(0)=0isaclosedlinearmanifoldinC[0;1].8.LetMbea
nite-dimensionallinearmanifoldinanormedvectorspaceXwithM=fx1;:::;xng.Eachx2PXcanbewrittenasauniquelinearcombination1x1++nxn.Wemayde
neanormnjjxjj1=i=1jijandseethatjjjj1isequivalenttotheoriginalnormonX.ThusconvergenceunderthePn(k)originalnormonXisequivalenttoconvergenceofeachsequenceofcoecientsinR.Lethi=1ixiik(k)beasequenceinMconvergingtox2X.Leti=limkiforeachi.BycontinuityofadditionandPn(k)Pnscalarmultiplication,x=limki=1ixi=i=1ixi2M.HenceMisclosed.9.LetS=fx:jjxjj<1g.Givenx2S,let=(1�jjxjj)=2.Whenjjy�xjj<,wehavejjyjjjjy�xjj+jjxjj<(1�jjxjj)=2+jjxjj<1soy2S.HenceSisopen.ForanysequencehxniinSthatconvergestosomex,wehavejjxnjj!jjxjjsojjxjj1.ThusSfx:jjxjj1g.Ontheotherhand,ifjjxjj=1and>0,let=max(1�=2;0).Thenjjxjj2Sandjjx�xjj=j1�j<.Thusx2Sandfx:jjxjj1gS.HenceS=fx:jjxjj1g.10.De
nexyifjjx�yjj=0.Thenxxsincejj0xjj=0jjxjj=0.Also,xyimpliesyxsincejjy�xjj=jj�(x�y)jj=j�1jjjx�yjj=jjx�yjj.Finally,ifxyandyz,then60khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
jjx�zjjjjx�yjj+jjy�zjj=0soxz.Thusisanequivalencerelation.Ifx1y1andx2y2,thenjj(x1+x2)�(y1+y2)jjjjx1�y1jj+jjx2�y2jj=0sox1+x2y1+y2.Ifxy,thenjjcx�cyjj=jcjjjx�yjj=0socxcyforc2R.Henceiscompatiblewithadditionandscalarmultiplication.Ifxy,thenjjjxjj�jjyjjjjjx�yjj=0sojjxjj=jjyjj.LetX0bethesetofequivalenceclassesunder.De
ne
x0+
y0asthe(unique)equivalenceclasswhichcontains
x+
yforx2x0andy2y0andde
nejjx0jj=jjxjjforx2x0.ThenX0becomesanormedvectorspace.Themapping"ofXontoX0thattakeseachelementofXintotheequivalenceclasstowhichitbelongsisahomomorphismofXontoX0since"(
x+
y)=
x0+
y0=
"(x)+
"(y).Thekernelof"consistsoftheelementsofXthatbelongtotheequivalenceclasscontainingthezerovector.Thesearetheelementsxwithjjxjj=0.R1=2ROntheLpspaceson[0;1]wehavethepseudonormjjfjj=1jfjp.Thenfgif1jf�gjp=0.p00i.e.f=ga.e.Thekernelofthemapping"consistsofthefunctionsthatare0a.e.11.LetXbeanormedlinearspace(withnormjjjj)andMalinearmanifoldinX.Letjjxjj1=infm2Mjjx�mjj.Sincejjx�mjj0forallx2Xandm2M,jjxjj10forallx2X.Forx;y2Xand">0,thereexistm;n2Msuchthatjjx�mjj0isarbitrary,jjx+yjj1jjxjj1+jjyjj1.Also,forx2Xand2R,jj
xjj1=infm2Mjj
x�mjj=jjinfm2Mjjx�mjj=jjjjxjj1.Hencejjjj1isapseudonormonX.LetX0bethenormedlinearspacederivedfromXandthepseudonormjjjjusingtheprocessin1Q10.Thenaturalmapping"ofXontoX0haskernelMsinceitconsistsoftheelementsxwithjjxjj1=infm2Mjjx�mjj=0.LetObeanopensetinX.Takex2O.Thenthereexists>0suchthaty2Oifjjy�xjj1<.Nowifjjz�"(x)jj<,wherez="(y)forsomey2X,thenjjy�xjj1<soy2Oandz2"[O].Hence"[O]isopen.i.e."mapsopensetsintoopensets.12.SupposeXiscompleteandMisaclosedlinearmanifoldinX.Leth"(xn)ibeanabsolutelyPPsummablesequenceinX=M.Thenjj"(xn)jj<1soPjjxnjj1<1.GivenP">0,foreachn,thereexistsm2Msuchthatjjx�mjj0andx2X,thereexistsm2Msuchthatjjx�mjj1�".Sincejj"jj=supjjxjj=1jj"(x)jj,wehavejj"jj>1�"forall">0.Thusjj"jj1.15b.LetXandYbenormedlinearspacesandAaboundedlinearoperatorfromXintoYwhosekernelisM.De
neamappingBfromX=MintoYbyBx0=Axwherex0istheequivalenceclasscontainingx.Ifx0=y0,thenjjx�yjj=0.Thusforany">0,thereexistsm2Msuchthatjjx�y�mjj<".1ThenjjAx�Ayjj=jjA(x�y�m)jjjjAjj".Since">0isarbitrary,jjAx�Ayjj=0.i.e.Ax=Ay.ThusBiswell-de
nedandA=B".Furthermore,itistheuniquesuchmapping.Ifx0;y02X=Mand
;
2R,thenB(
x0+
y0)=A(
x+
y)=
Ax+
Ay=
Bx0+
By0soBisalinearoperator.Also,jjBx0jj=jjAxjjjjAjjjjxjj=jjAjjjjx�mjjforallm2M.ThusjjBx0jjjjAjjjjxjj=jjAjjjjx0jj1sojjBjjjjAjjandBisbounded.Forany">0,thereexistsx2Xwithjjxjj=1andjjAxjj>jjAjj�".61khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
Thenjjx0jj1andjjBx0jj>jjAjj�".SincejjBjj=supjjBx0jj,wehavejjBjjjjAjj.Hencejjx0jj1jjAjj=jjBjj.16.LetXbeametricspaceandYthespaceofreal-valuedfunctionsfonXvanishingata
xedpointx02Xandsatisfyingjf(x)�f(y)jM(x;y)forsomeM(dependingonf).De
nejjfjj=jf(x)�f(y)jsup.Clearlyjjfjj0.Also,jjfjj=0ifandonlyiff(x)=f(y)forallx;y2Xifandonlyiffis(x;y)thezerofunction.Furthermore,jjf+gjjjjfjj+jjgjjsincej(f+g)x�(f+g)yjjf(x)�f(y)j+jg(x)�g(y)jandsupA+BsupA+supB.Similarly,jj
fjj=jjjjfjj.Thusjjjjde
nesanormonY.Foreachx2X,de
nethefunctionalFxbyFx(f)=f(x).ThenFx(
f+
g)=
f(x)+
g(x)=
Fx(f)+
Fx(g)soFxisalinearfunctionalonY.Also,jjFx(f)jj=jf(x)j=jf(x)�f(x0)j(x;x0)jjfjjsoFxisbounded.Furthermore,jjFxjj(x;x0)sojjFx�Fyjj(x;x0)+(y;x0)(x;y).Ifjjfjj=1jf(x)�f(y)jand">0,thenthereexistx;y2Xsuchthat>1�".i.e.jf(x)�f(y)j>(1�")(x;y).(x;y)SincejjFx�Fyjj=supjjfjj=1j(Fx�Fy)fj=supjjfjj=1jf(x)�f(y)j>(1�")(x;y)forall">0,wehavejjFx�Fyjj(x;y).HencejjFx�Fyjj=(x;y).ThusXisisometrictoasubsetofthespaceYofboundedlinearoperatorsfromYtoR.SinceYiscomplete,theclosureofthissubsetgivesacompletionofkhdaw.comX.10.3LinearfunctionalsandtheHahn-BanachTheorem17.Letfbealinearfunctionalonanormedlinearspace.Iffisbounded,thenitisuniformlycontinuousandbyQ14,itskernelisclosed.Conversely,iffisunbounded,thenthereisasequencehxniwithjjxnjj1forallnandf(xn)!1.Takex=2kerfandconsideryn=x�(f(x)=f(xn))xn.Eachynisinkerfandyn!x.Thuskerfisnotclosed.18.LetTbealinearsubspaceofanormedlinearspaceXandyagivenelementofX.Ify2T,theninft2Tjjy�tjj=0=supff(y):jjfjj=1;f(t)=0forallt2Tg.Thuswemayassumey=2T.Let=inft2Tjjy�tjj.Thenjjy�tjjforallt2T.ThereisaboundedlinearfunctionalfonXsuchthatjjfjj=1,f(y)=andf(t)=0forallt2T.Thussupff(y):jjfjj=1;f(t)=0forallt2Tg.Ifsothereexistst2Tsuchthatf(y)>jjy�tjj.Butthenjjy�tjj=jjfjjjjy�tjjf(y�t)=f(y)>jjy�tjj.Contradiction.Thussupff(y):jjfjj=1;f(t)=0forallt2Tg.Henceinft2Tjjy�tjj=supff(y):jjfjj=1;f(t)=0forallt2Tg.19.LetTbealinearsubspaceofanormedlinearspace课后答案网XandyanelementofXwhosedistancetoTisatleast.LetSbethesubspaceconsistingofmultiplesofy.De
nef(y)=.ThenfisalinearfunctionalonS.Letp(x)=inft2Tjjx�tjj.Thenf(y)=p(y)p(y).BytheHahn-BanachTheorem,wemayextendftoallofXsothatf(x)p(x)forallx2X.Inparticular,f(y)=andf(t)=0forallt2T.Also,f(x)p(x)=inft2Tjjx�tjjjjxjjsojjfjj1.20.Let`1bethespaceofallboundedsequencesandletSbethesubspaceconsistingoftheconstantsequences.LetGbetheAbeliansemigroupofoperatorsgeneratedbytheshiftoperatorwww.hackshp.cnAgivenbyA[hni]=hn+1i.Ifhni2S,sayn=foralln,de
nef[hni]=.ThenfisalinearfunctionalonS.De
nep[hi]=lim.Thenf[hi]=p[hi]onS.Also,p(Anx)=p(x)forallx2X.Ifhi2S,nnnnnthenAn[hi]=hi2Sandf(An[hi])=f[hi].ByProposition5,thereisanextensionFofftoannnnlinearfunctionalonXsuchthatF(x)p(x)andF(Ax)=F(x)forallx2X.Inparticular,F[hni]limn.Also,�F[hni]=F[h�ni]lim(�n)=�limnsolimnF[hni].Bylinearity,F[hn+ni]=F[hni+hni]=F[hni]+F[hni]andF[h
ni]=F[hni]=
F[hni].Finally,ifn=n+1,thenF[hni]=F[A[hni]]=F[hni].(*)ThefunctionalFiscalledaBanachlimitandisoftendenotedbyLim.*21.LetXbethespaceofboundedreal-valuedfunctionsontheunitcircleandletSbethesubspaceRofboundedLebesguemeasurablefunctionsontheunitcircle.Fors2S,de
nef(s)=s.Alsode
nep(x)=infxsf(s).ThenfisalinearfunctionalonSwithfponS.LetGconsistoftherotationssothatitisanAbeliansemigroupofoperatorsonXsuchthatforeveryA2Gwehavep(Ax)p(x)forx2Xwhilefors2SwehaveAs2Sandf(As)=f(s).ThenthereisanextensionofftoalinearfunctionalFonXsuchthatF(x)p(x)andF(Ax)=F(x)forx2X.ForasubsetPoftheunitcircle,let(P)=F( P).Thiswillbearotationallyinvariantmeasureon[0;2].Thenextendittothe62khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
boundedsubsetsofRtogettherequiredsetfunction.22.LetXbeaBanachspace.SupposeXisre
exive.IfXisnotre
exive,thenthereisanonzerofunctiony2Xsuchthaty(x0)=0forallx02"[X].Butthereexistsx2Xsuchthaty="(x).Ifx2X,then0=y("(x))=("(x))("(x))=("(x))(x)=x(x).Thusx=0andsoy=0.Contradiction.ThusXisre
exive.Conversely,supposeXisre
exive.Letx2X.De
nex2Xbyx(x)=x("(x)).Then"(x)(x)=x(x)=("(x))(x)=x(x)=x("(x))=x(x).ThusXisre
exive.23a.Ifx;y2Sand
;
2R,then(
x+
y)(s)=
x(s)+
y(s)=0foralls2SsoSisalinearsubspaceofX.LethyibeasequenceinSthatconvergestosomey2X.ByQ13,foreachs2S,ny(s)!y(s).Sincey(s)=0foralln,wehavey(s)=0.Thusy2SandSisclosed.nn*23b.Ifx2S,thenthereisasequencehsiinSconvergingtox.Lety2S.Theny(s)=0fornnallnsoy(x)=0.ThusSS.Supposethereexistsx2SnS.Thenthereisalinearfunctionalfwithjjfjj1,f(x)=infjjx�tjj>0andf(t)=0fort2S.Thusf2(S)=Ssof(x)=0.t2SContradiction.HenceS=S.23c.LetSbeaclosedsubspaceofXandlet":X!X=Sbethenaturalhomomorphism.De
neA:X!SbyAy=yj.ThenAisaboundedlinearoperatorwithkernelS.ByQ15b,thereisakhdaw.comSuniqueboundedlinearoperatorB:X=S!SsuchthatA=B".BytheHahn-BanachTheorem,Aisonto.ThussoisB.Ifyj=zj,theny�z2Sso"(y)="(z)andBisone-to-one.HenceBisSSanisomorphismbetweenSandX=S.23d.LetSbeaclosedsubspaceofare
exiveBanachspaceX.Let":X!Xbethenaturalisomorphismandde
neA:X!SbyAy=yj.Lets2S.ThensA2XsosA="(x)Sforsomex2X.Ifx=2S,thenthereexistsx2Xsuchthatx(x)>0andx(s)=0fors2S.ThenA(x)=0sox(x)=("(x))(x)=(sA)(x)=0.Contradiction.Thusx2S.Nowforanys2S,thereexistsx2XsuchthatA(x)=s.Thens(s)=(sA)(x)=("(x))(x)=x(x)=s(x)=("(x))(s).i.e.s="(x).HenceSisre
exive.SS24.LetXbeavectorspaceandPasubsetofXsuchthatx;y2Pimpliesx+y2Pand
x2Pfor
>0.De
neapartialorderinXbyde
ningxytomeany�x2P.AlinearfunctionalfonXissaidtobepositive(withrespecttoP)iff(x)0forallx2P.LetSbeanysubspaceofXwiththepropertythatforeachx2Xthereisans2Swithxs.LetfbeapositivelinearfunctionalonS.ThefamilyofpositivelinearfunctionalsonSispartiallyorderedbysettingf
gifgisanextensionoff.BytheHausdor
MaximalPrinciple,thereisamaximallinearlyorderedsubfamily课后答案网fggcontainingf.De
neafunctionalFontheunionofthedomainsofthegbysettingF(x)=g(x)ifxisinthedomainofg.Sincethesubfamilyislinearlyordered,Fiswell-de
ned.Also,Fisapositivelinearfunctionalextendingf.Furthermore,FisamaximalextensionsinceifGisanyextensionofF,theng
F
GimpliesthatGmustbelongtofggbymaximalityoffgg.ThusG
FsoG=F.LetTbeapropersubspaceofXwiththepropertythatforeachx2Xthereisat2Twithxt.Weshowthateachpositivelinearfunctionalwww.hackshp.cngonThasaproperextensionh.Lety2XnTandletUbethesubspacespannedbyTandy.Ifhisanextensionofg,thenh(y+t)=h(y)+h(t)=h(y)+g(t).Thereexistst02Twithyt0.i.e.t0�y2T.Then(t0�y)+t2Tandg((t0�y)+t)0.De
neh(y)=g(t0�y).Thenh(y+t)=h(y)+g(t)=g((t0�y))+g(t)=g((t0�y)+t)0.Thushisaproperextensionofg.SinceFisamaximalextension,itfollowsthatFisde
nedonX.*25.LetfbeamappingoftheunitballS=fx:jjxjj1gintoRsuchthatf(
x+
y)=
f(x)+
f(y)wheneverx;yand
x+
yareinS.De
neg(x)=jjxjjf(x).Ifjjxjj1,theng(x)=jjxjj1f(x)=jjxjjjjxjjf(x).Ifx;y2X,theng(x+y)=jjx+yjjf(x+y)=jjx+yjjf(jjxjjx+jjyjjy)=jjx+jjx+yjjjjx+yjjjjxjjjjx+yjjjjyjjyjj[jjxjjf(x)+jjyjjf(y)]=jjxjjf(x)+jjyjjf(y)=g(x)+g(y).If2Randx2X,thenjjx+yjjjjxjjjjx+yjjjjyjjjjxjjjjyjjg(
x)=jj
xjjf(
x)=jjjjxjjf(
x)=jjjjxjjf(x)=
g(x).Thusgisalinearfunctionalonjj
xjjjjjjxjjjjjjxjjXextendingf.10.4TheClosedGraphTheorem26.LethTnibeasequenceofcontinuouslinearoperatorsfromaBanachspaceXtoanormedvectorspaceY.Supposethatforeachx2XthesequencehTnxiconvergestoavalueTx.Nowforeach63khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
x2XthereexistsMxsuchthatjjTnxjjMxforalln.ThusthereexistsMsuchthatjjTnjjMforalln.Given">0,foreachx2X,thereexistsNsuchthatjjTNx�Txjj<".ThenjjTxjjjjTNx�Txjj+jjTNxjj<"+Mjjxjj.ThusjjTxjjMjjxjjforallx2X.i.e.Tisaboundedlinearoperator.27.LetAbeaboundedlineartransformationfromaBanachspaceXtoaBanachspaceY,andletMbethekernelandStherangeofA.SupposeSisisomorphictoX=M.SinceXiscompleteandMisclosed,X=MiscompletebyQ12.ThusX=MisclosedandsinceSisisomorphictoX=M,Sisalsoclosed.Conversely,supposeSisclosed.ThensinceYiscomplete,soisS.Let"bethenaturalhomomorphismfromXtoX=M.ThereisauniqueboundedlinearoperatorB:X=M!SsuchthatA=B".SinceAand"areonto,soisB.ThusBisanopenmapping.ItremainstoshowthatBisone-to-one.SupposeBx0=By0.Thenx0="(x)andy0="(y)forsomex;y2XsoB("(x))=B("(y)).ThenAx=Aysox�y2Mandx0=y0.HenceBisone-to-oneandisthusanisomorphism.28a.LetSbealinearsubspaceofC[0;1]thatisclosedasasubspaceofL2[0;1].LethfibeasequenceninSconvergingtofinC[0;1].i.e.jjfn�fjj1!0.Thensincejjfn�fjj2jjfn�fjj1,wehavejjfn�fjj2!0.Thusf2S.HenceSisclosedasasubspaceofC[0;1].RR28b.Foranyf2S,wehavejjfjj=(f2)1=2(jjfjj2)1=2=jjfjj.SinceSisclosedinbothC[0;1]211andLkhdaw.com2[0;1],itiscompleteinbothnorms.ThusthereexistsMsuchthatjjfjjMjjfjj.12*28c.Lety2[0;1]andde
neF(f)=f(y).ThenFisalinearfunctionalonL2[0;1].Also,jF(f)j=jf(y)jjjfjjMjjfjjsoFisbounded.BytheRieszRepresentationTheorem,thereexistsk2L212Rysuchthatf(y)=F(f)=ky(x)f(x)dx.*29a.LetY=C[0;1]andletXbethesubspaceoffunctionswhichhaveacontinuousderivative.LetAbethedi
erentialoperator.Letx(t)=tn.Thenjjxjj=1andAx(t)=ntn�1sojjAxjj=n.ThusnnnnAisunboundedandthusdiscontinuous.Letx2Xsuchthatx!xandx0=Ax!y.SinceweRRnRnnRnhaveuniformconvergence,y=limx0=limx0=x(t)�x(0)sox(t)=x(0)+y.Thusx2XnnandAx=x0=y.HenceAhasaclosedgraph.*29b.ConsiderA:R!RgivenbyA(x)=1=xifx6=0andA(0)=0.ThenAisadiscontinuousoperatorfromaBanachspacetoanormedlinearspacewithaclosedgraph.10.5Topologicalvectorspaces30a.LetBbeacollectionofsubsetscontaining课后答案网.SupposeBisabaseatforatranslationinvarianttopology.Byde
nitionofabase,ifU;V2B,thereexistsW2BsuchthatWUVso(i)holds.IfU2Bandx2U,thenU�xisopensothereexistsV2BsuchthatVU�x.Thenx+VUso(ii)holds.Conversely,suppose(i)and(ii)hold.LetT=fO:x2O)9y2XandU2Bsuchthatx2y+UOg.ItfollowsthatTcontains;andX,andisclosedunderunion.Ifx2O1O2,thenthereexisty1;y22XandU1;U22Bsuchthatxwww.hackshp.cn2yi+Ui2Oi;i=1;2.Nowx�yi2Uisoby(ii),thereexistsVi2Bsuchthatx�yi+ViUi.i.e.x+Viyi+UiOi.Nowby(i),thereexistsW2BsuchthatWV1V2sox2x+WO1O2.ThusTisclosedunder
niteintersection.IfO2Tandy2x+O,theny�x2Osothereexistsz2XandU2Bsuchthaty�x2z+UO.Thusy2x+z+Ux+Osox+O2T.HenceTisatranslationinvarianttopology.Furthermore,if2O,thenthereexistx2XandU2Bsuchthat2x+UO.Thus�x2UsothereexistsV2Bsuchthat�x+VU.i.e.Vx+U.Then2VO.ThusBisabaseat.30b.LetBbeabaseatforatranslationinvarianttopology.SupposeadditioniscontinuousfromXXtoX.Inparticular,additioniscontinuousath;i.ThusforeachU2B,thereexistsV1;V22BsuchthatV1+V2U.TakeV2BwithVV1V2.ThenV+VU.Conversely,suppose(iii)holds.Forx0;y02X,fx0+y0+U:U2Bgisabaseatx0+y0.NowforeachU2B,pickV2BsuchthatV+VU.Ifx2x0+Vandy2y0+V,thenx+y2x0+y0+U.ThusadditioniscontinuousfromXXtoX.30c.Supposescalarmultiplicationiscontinuous(ath0;i)fromRXtoX.GivenU2Bandx2X,thereexist">0andV2Bsuchthat(x+V)Uforjj<".Let=2=".Then1(x+V)Usox+V
U.Inparticular,x2
U.30d.LetXbeatopologicalvectorspaceandletBbethefamilyofallopensetsUthatcontainand64khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
suchthat
UUforallwithjj<1.IfOisanopensetcontaining,thencontinuityofscalarmultiplicationimpliesthatthereisanopensetVcontainingandan">0suchthatVOforallSjj<".LetU=V.ThenVisopen,2UO,and
UUforwithjj<1.ThusBisajj<"localbaseforthetopologyanditsatis
es(v)byitsde
nition.*30e.SupposeBsatis
estheconditionsoftheproposition.Bypart(a),Bisabaseatforatranslationinvarianttopologyandbypart(b),additioniscontinuous.GivenU2B,thereexistsV2BsuchthatV+VU.Nowgivenx2X,thereexists2Rsuchthatx2
V.Let"=1=jj.Ifjjjj.Let"=jjn�jj.Nowwhenj�j<",wehavejj0andW;W02Bsuchthat(x+W)Vwhenjj<"and
W0Vwhenj�j<".Then00
x�x=(x�x)+(�)x2V+VUwhenj�j<"andx2x+W0.Hencescalar0000000multiplicationiscontinuousfromRXtoX.khdaw.comT30f.SupposeXisT1.Ifx6=andx2fU2Bg,thenanyopensetcontainingwillalsocontainx.Contradiction.Hence(vi)holds.Conversely,suppose(vi)holds.Giventwodistinctpointsxandy,thereexistsU2Bsuchthatx�y=2U.Also,thereexistsV2BsuchthatV+VU.If(x+V)(y+V)6=;,thenx�y2V�V.By(v),�VVsox�y2V+VU.Contradiction.Thusx+Vandy+VaredisjointopensetsseparatingxandysoXisHausdor
.(*)ProofofProposition1431a.SupposealineartransformationffromonetopologicalvectorspaceXtoatopologicalvectorspaceYiscontinuousatonepoint.Wemayassumefiscontinuousattheorigin.LetObeanopensetcontainingtheorigininY.ThereexistsanopensetUcontainingtheorigininXsuchthatf[U]O.Sincefislinear,foranyx2X,f[x+U]=f(x)+f[U]f(x)+O.Hencefisuniformlycontinuous.*31b.LetfbealinearfunctionalonatopologicalvectorspaceX.Supposefiscontinuous.LetIbeaboundedopenintervalcontaining0.ThereexistsanopensetOcontainingtheorigininXsuchthatf[O]I.Thusf[O]6=R.Conversely,supposethereisanonemptyopensetOsuchthatf[O]6=R.Takex2O.ThenO�xisanopenneighbourhoodofsothereisanopenneighbourhoodUofsuchthatUO�xand
U课后答案网Uforwithjj<1.Nowf[U]6=Rand
f[U]=f[
U]f[U]ifjj<1sof[U]isaboundedinterval.Thusfiscontinuousatandthuscontinuouseverywhere.32.LetXbeatopologicalvectorspaceandMaclosedlinearsubspace.Let"bethenaturalhomo-morphismofXontoX=M,andde
neatopologyonX=MbytakingOtobeopenifandonlyif"�1[O]SisopeninX.Clearly,"iscontinuous.IfUisopeninX,then"�1["[U]]=(m+U),whichism2Mopenso"[U]isopen.NowletObeanopensetcontainingx0+y02X=M.Then"�1[O]isanopensetcontainingx+y2X.Thereexistopensetswww.hackshp.cnUandVcontainingxandyrespectivelysuchthatU+V"�1[O].Since"isopen,"[U]and"[V]areopensetscontainingx0andy0respectively.Then"[U]+"[V]"["�1[O]]=O.ThusadditioniscontinuousfromX=MX=MtoX=M.NowletObeanopensetcontainingcx02X=M.Then"�1[O]isanopensetcontainingcx2X.ThereexistopensetsUandVcontainingcandxrespectivelysuchthatUV"�1[O].Since"isopen,"[V]isanopensetcontainingx0.ThenU"[V]"["�1[O]]=O.ThusscalarmultiplicationiscontinuousfromRX=MtoX=M.HenceX=Misatopologicalvectorspace.33a.SupposeXis
nitedimensionaltopologicalvectorspace.Letx1;:::;xnbeavectorspacebasisofPXandlete;:::;ebethestandardbasisofRn.De
nealinearmap"ofRntoXsothat"(nae)=P1nPi=1Piinax.Then"isone-to-oneandthusonto.Ifasequenceha(j)eiinRnconvergestoae,i=1iiiiii(j)thenhaiiconvergestoaiforeachi.SinceadditionandscalarmultiplicationarecontinuousonX,theP(j)Psequencehaixiiconvergestoaixi.Thus"iscontinuous.33b.SupposeXisHausdor
.LetSandBbethesubsetsofRnde
nedbyS=fy:jjyjj=1gandB=fy:jjyjj<1g.SinceSiscompactand"iscontinuous,"[S]iscompactinXandthusclosed.ThenXn"[S]isopen.33c.LetBbeabaseatsatisfyingtheconditionsofProposition14.SinceXn"[S]isanopenset65khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
containing,thereexistsU2BsuchthatUXn"[S].Furthermore,
UUforeachjj<1bycondition(v)ofProposition14.33d.Supposeu="(y)2Uforsomeywithjjyjj>1.Then1<1so1u2Ubut1u=jjyjjjjyjjjjyjj1"(y)="(y)wherejjyjj=1so1u2"[S].Contradiction.HenceU"[B].Thus"[B]isjjyjjjjyjjjjyjjjjyjjopenand"�1iscontinuous.(*)ProofofProposition15.34.LetMbea
nitedimensionalsubspaceofaHausdor
topologicalvectorspaceX.Ifx=2M,letNbethe
nitedimensionalsubspacespannedbyxandM.ThenNhastheusualtopologysoxisnotapointofclosureofM.HenceMisclosed.35.LetAbealinearmappingofa
nitedimensionalHausdor
vectorspaceXintoatopologicalvectorspaceY.TherangeofAisa
nitedimensionalsubspaceofYsoithastheusualtopology.Ifxn!xinP(n)P(n)Xwherexn=aieiandx=aiei,thenai!aiforeachi.SincetherangeofAhastheusualP(n)Ptopology,Axn=aiAei!aiAei=Ax.HenceAiscontinuous.*36.LetAbealinearmappingfromatopologicalvectorspaceXtoa
nitedimensionaltopologicalspacekhdaw.comY.IfAiscontinuous,thenitskernelMisclosedbyQ14.Conversely,supposeMisclosed.ThereisauniquelinearmappingB:X=M!YsuchthatA=B"where":X!X=Misthenaturalhomomorphism,whichisacontinuousopenmapbyQ32.Thenker"isclosedand"isanopenmapsoX=MisaHausdor
topologicalvectorspace.Furthermore,Bisone-to-oneandYis
nitedimensionalsoX=Mis
nitedimensional.ByQ35,Biscontinuous.HenceAiscontinuous.*37.LetXbealocallycompactHausdor
vectorspace.LetVbeaneighbourhoodofwithVcompactand
VVforeachwithjj<1.Thesetfx+1V:x2VgisanopencoverofVsoVcanbecovered3bya
nitenumberoftranslatesx+1V;:::;x+1V.Toshowthatx;:::;xspanX,itsucesto13n31nshowthattheyspanV.Letx2V.Thenx=x+1uforsomek2f1;:::;ngandu2U.Now1Uk131113iscoveredby1(x+1V);:::;1(x+1V)sox=x+1x+1u.Continuinginthisway,wehave3133n3k13k292x2x+1x++1x+1Uforeachr.Lety=x+1x++1x.Thenyisinthek13k23r�2kr3rrk13k23r�2krrspanofS,whichis
nitedimensionalandthusclosedbyQ34.Nowforeachy2V,thereexists"y>0andanopenneighbourhoodUyofysuchthatUyVwheneverjj<"ysincescalarmultiplicationiscontinuousath0;yi.TheopensetsUycoverVsoVUy1[[Uyn.ThenVVwheneverjj0begiven.ChooseMsuchthat0课后答案网03�M�1<3�N.WhenmM,wehave3�m�1<3�Nso3�m�1VV.i.e.3�mVV.Thusx�ym2VformM.Thusx�ym!andym!x.HencexisinthespanofS.Itfollowsthatx1;:::;xnspanXsoXis
nitedimensional.10.6Weaktopologieswww.hackshp.cn38a.Supposex!xweakly.Thenf(x)!f(x)foreachf2X.Thusjf(x)jCforeachf2Xnnnfandeachn.Nowlet":X!Xbethenaturalhomomorphismsothat"(x)(f)=f(x).Thennnj"(x)(f)jCforeachf2Xandeachn.SinceXisaBanachspace,hjj"(x)jjiisboundedbutnfnjj"(xn)jj=jjxnjjsohjjxnjjiisbounded.*38b.Lethxibeasequencein`p;10,thereexistsNsuchthatjjFN�Fjj<"=3C.SinceFN2spanfengandm;n!mforeachn,thereisanMsuchthatjFN(xn)�FN(x)j<"=3fornM.ThusfornM,wehavejF(xn)�F(x)jjF(xn)�FN(xn)j+jFN(xn)�FN(x)j+jFN(x)�F(x)j<".Hencehxniconvergesweaklytox.66khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
38c.LethxibeasequenceinLp[0;1];1ng.NotethatthedistancebetweenanytwopointsinFisatleast1sotherearenononconstantsequencesinFthatconvergeinthestrongtopology.AnysequenceinFthatconvergesmustbeaconstantsequencesoitslimitisinF.HenceFisstronglyclosed.38g.LetFbeasinpart(f).Thesetsfx:jf(x)j<";i=1;:::;ngwhere">0andf;:::;f2(`p)i1nformabaseatfortheweaktopology.Given">0andf;:::;f2(`p),f(y)isoftheform1nim;ni+niwherehii2`q.Choosensuchthatjij<"=2foralli.Thenchoosem>nsuchthatnmnnjij<"=2nforalli.Thenjf(y)jjij+njij<".ThusFfx:jf(x)j<";i=1;:::;ng6=;mim;nnmiandisaweakclosurepointofF.Supposehzki=hymk;nki=hxnk+nkxmkiisasequencefromFthatconvergesweaklytozero.Given">0andhi2`q,thereexistsNsuchthatj+nj<"forkN.Supposefmgisboundednnkkmkkabove.Thensomemisrepeatedin
nitelymanytimes.Letm=1andn=0otherwise.ForeachNthereexistskNsuchthatmk=msojnk+nkmkj=jnkj1.Thusfmkgisnotboundedaboveandwemayassumethesequence课后答案网hmkiisstrictlyincreasing.Nowsupposefnkgisboundedabove.Thensomenisrepeatedin
nitelymanytimes.Letn=1andm=0otherwise.ForeachNthereexistskNsuchthatnk=nsojnk+nkmkj=1.Thusfnkgisnotboundedaboveandwemayassumethesequencehnkiisstrictlyincreasing.Nowletmk=1=nkforeachkandm=0ifm6=mkforanyk.Thenhi2`qandj+nj1forallk.Contradiction.HencethereisnosequencehzifromnnkkmkkFthatconvergesweaklytozero.38h.Theweaktopologyof`1istheweakesttopologysuchthatallfunctionalsin(`1)=`1arecontinuous.Abaseatisgivenbythesetswww.hackshp.cnfx2`1:jf(x)j<";i=1;:::;ngwhere">0andi1()11P()f1;:::;fPn2`.Aneth(xn)iin`convergesweaklyto(xn)2`ifandonlyifnxnynconvergestoxyforeach(y)2`1.nnnnIfaneth(x()1convergesweaklyto(x)2`1,thenforeachn,taking(y)2`1wherey=1andn)iin`nnn()ym=0form6=n,wehavexnconvergingtoxnforeachn.()1()PIftheneth(xn)iin`isbounded,saybyM,andxnconvergestoxnforeachn,thennjxnjP()P()11P()P()njxn�xnj+njxnjMso(xn)2`.If(yn)2`,thenjn(xn�xn)ynjjj(yn)jj1njxn�P()P()xnj!0sonxnynconvergestonxnynandh(xn)iconvergesweaklyto(xn).Fork2N,let(x(k)1wherex(k)=kandx(k)(k)n)2`kn=0ifn6=k.Thenthesequenceh(xn)iisnotboundedandx(k)1wherey=1foralln,wehavenconvergesto0foreachn.However,taking(yn)2`nP(k)(k)nxnyn=k,whichdoesnotconvergeto0soh(xn)idoesnotconvergeweaklyto.Theweak*topologyon`1asthedualofcistheweakesttopologysuchthatallfunctionalsin"[c]`100arecontinuous.Abaseatisgivenbythesetsff2`1:jf(x)j<";i=1;:::;ngwhere">0andi()11P()x1;:::;xn2c0.Aneth(xn)iin`isweak*convergentto(xn)2`ifandonlyifnxnynconverges67khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
Ptonxnynforall(yn)2c0.Usingthesameargumentsasaboveandreplacing`1byc,weseethatifaneth(x()1isweak*0n)iin`convergentto(x)2`1,thenx()nnconvergestoxnforeachn.Wealsoseethatifthenetisbounded()andxnconvergestoxn,thenthenetisweak*convergentto(xn).Fork2N,let(x(k)1wherex(k)=kandx(k)(k)n)2`kn=0ifn6=k.Thenthesequenceh(xn)iisnot(k)boundedandxnconvergesto0foreachn.However,taking(yn)2c0whereyn=1=nforalln,weP(k)(k)havenxnyn=1,whichdoesnotconvergeto0soh(xn)iisnotweak*convergentto.39a.LetX=c;F=`1andFthesetofsequenceswith
nitelymanynonzeroterms,whichisdense00in`1.Considerthesequenceh(x(k)(k)=k2andx(k)n)iinc0wherexkn=0ifn6=k.ForanysequenceP(k)2(k)(yn)2F0,wehavenxnyn=kyk!0.Thusthesequenceh(xn)iconvergestozerointheweak(k)topologygeneratedbyF0.Nowifthesequenceh(xn)iconvergesintheweaktopologygeneratedbyF,theweaklimitmustthenbezero.Let(z)bethesequenceinFwherez=1=n2foreachn.ThennnP(k)(k)nxnzn=1.Thusthesequenceh(xn)idoesnotconvergeintheweaktopologygeneratedbyF.Hencekhdaw.comFandF0generatedi
erentweaktopologiesforX.NowsupposeSisaboundedsubsetofX.WemayassumethatScontains.LetFbeasetoffunctionalsinXandletFbeadensesubsetofF(inthenormtopologyonX).Notethatingeneral,theweak0topologygeneratedbyF0isweakerthantheweaktopologygeneratedbyF.AbaseatfortheweaktopologyonSgeneratedbyFisgivenbythesetsfx2S:jfi(x)j<";i=1;:::;ngwhere">0andf1;:::;fn2F.AsetinabaseatfortheweaktopologyonSgeneratedbyF0isalsoinabaseatfortheweaktopologygeneratedbyF.Supposex2SsothatjjxjjMandjfi(x)j<"forsome">0andf1;:::;fn2F.Foreachi,thereexistsgi2F0suchthatjjfi�gijj<"=2M.Ifjgi(x)j<"=2fori=1;:::;n,thenjfi(x)jjfi(x)�gi(x)j+jgi(x)jjjfi�gijjjjxjj+jgi(x)j<"fori=1;:::;n.ThusanysetinabaseatfortheweaktopologyonSgeneratedbyFcontainsasetinabaseatfortheweaktopologygeneratedbyF0.HencethetwoweaktopologiesarethesameonS.*39b.LetSbetheunitsphereinthedualXofaseparableBanachspaceX.LetfxgbeacountablendensesubsetofX.Thenf"(xn)gisacountabledensesubsetof"[X].Bypart(a),f"(xn)ggeneratesthesameweaktopologyonSas"[X].i.e.f"(x)ggeneratestheweak*topologyonS.Nowde
nenP(f;g)=2�njf(xn)�g(xn)j.ThenisametriconS.Furthermore(f;f)!0ifandonlyif1+jf(xn)�g(xn)jnjfn(xk)�f(xk)j!0foreachk(seeQ7.24a)ifandonlyifj"(xk)(fn)�"(xk)(f)j!0foreachkifandonlyiff!fintheweak*topology.Hence课后答案网Sismetrizable.n40.SupposeXisaweaklycompactset.Everyx2Xiscontinuoussox[X]iscompactinR,andthusbounded,foreachx2X.Foreachx2Xandx2X,thereisaconstantMxsuchthatj"(x)(x)j=jx(x)jMx.Thusfjj"(x)jj:x2Xgisbounded.Sincejj"(x)jj=jjxjjforeachx,wehavefjjxjj:x2Xgisbounded.41a.LetSbethelinearsubspaceofwww.hackshp.cnC[0;1]giveninQ28(SisclosedasasubspaceofL2[0;1].SupposehfiisasequenceinSsuchthatf!fweaklyinL2.ByQ28c,foreachy2[0;1],thereexistsnnRRRk2L2suchthatforallf2Swehavef(y)=kf.Nowfk!fkforeachy2[0;1]sinceyynyyk2L2=(L2).Thusf(y)!f(y)foreachy2[0;1].yn41b.SupposehfiisasequenceinSsuchthatf!fweaklyinL2.ByQ38a,hjjfjjiisbounded.nnn2ByQ28b,thereexistsMsuchthatjjfjj1Mjjfjj2forallf2S.Inparticular,jjfnjj1Mjjfnjj2foralln.Hencehjjfjjiisbounded.Nowhf2iisasequenceofmeasurablefunctionswithjfj2M0onn1nn[0;1]andf2(y)!f2(y)foreachy2[0;1]asaconsequenceofpart(a).BytheLebesgueConvergencenTheorem,jjfjj2!jjfjj2andsojjfjj!jjfjj.ByQ6.16,f!fstronglyinL2.n22n22n*41c.SinceL2isre
exiveandSisaclosedlinearsubspace,Sisare
exiveBanachspacebyQ23d.ByAlaoglu"sTheorem,theunitballofSisweak*compact.ThentheunitballofSisweaklycompactsincetheweak*topologyonSinducestheweaktopologyonSwhenSisregardedasasubspaceofS.Bypart(b),theunitballofSiscompact.ThusSislocallycompactHausdor
sobyQ37,Sis
nitedimensional.68khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
10.7Convexity42.LetAbealinearoperatorfromthevectorspaceXtothevectorspaceY.LetKbeaconvexsetinX.Ifx;y2Xand01,thenAx+(1�)Ay=A(x)+A((1�)y)=A(x+(1�)y)2A[X].ThusA[X]isaconvexsetinY.LetK0beaconvexsetinY.Ifx;y2A�1[K0],thenAx;Ay2K0soif01,thenAx+(1�)Ay2K0.NowAx+(1�)Ay=A(x+(1�)y)sox+(1�)y2A�1[K0].ThusA�1[K0]isaconvexsetinX.ByusingthelinearityofA,itcanbeshownthatasimilarresultholdswhenconvexset"isreplacedbylinearmanifold".De
nethelinearoperatorA:R2!RbyA(hx;yi)=x+y.Takethenon-convexsetfh0;1i;h1;0igR2.ItsimageunderAistheconvessetf1gR.43.LetKbeaconvexsetinatopologicalvectorspace.Letx;y2Kand01.LetObeanopensetcontainingx+(1�)y.Sinceadditionandscalarmultiplicationarecontinuous,thereareopensetsUandVcontainingxandyrespectively,aswellas">0,suchthatU+VOwheneverj�j<"andj�(1�)j<".Nowthereexistx02KUandy02KV.Thenx0+(1�)y02KO.Hencex+(1�)y2KandKisconvex.44a.Letx0beaninteriorpointofasubsetKofatopologicalvectorspaceX.ThereisanopensetOsuchthatkhdaw.comx02OK.Letx2X.Bycontinuityofadditionathx0;i,thereexistsanopensetUcontainingsuchthatx0+UO.Bycontinuityofscalarmultiplicationath0;xi,thereexists">0suchthatx2Uwheneverjj<".Thusx0+x2OKwheneverjj<"andx0isaninternalpointofK.44b.InR,aconvexsetmustbeanintervalandaninternalpointmustnotbeanendpointoftheintervalsoitisaninteriorpoint.InRnforn2,letfe:i=1;:::;ngbethestandardbasis.iSupposex=hx1;:::;xniisaninternalpointofaconvexsetK.Foreachi,thereexists"i>0suchthatx+ei2Kifjj<"i.Let"=min1in"i.Ifjj<",thenx+ei2Kforalli.NowsupposePjjy�xjj<"=n.Thenjyi�xij<"=nforallisox+n(yi�xi)ei2Kforalli.Notethaty=n1(x+n(y�x)e),whichbelongstoKsinceKisconvex.Thusthereisanopenballcentredi=1niiiatxandcontainedinKsoxisaninteriorpointofK.*44c.ConsiderK=B[B[fhx;0i:jxj<1gR2.Thenh0;0iisaninternalpointofKh0;1i;1h0;�1i;1butnotaninteriorpoint.44d.SupposeaconvexsetKinatopologicalvectorspacehasaninteriorpointx.LetybeaninternalpointofK.Thereexists">0suchthaty+"(y�x)2K.NowthereisanopensetOsuchthatx2O2K.Let="=2.Theny=课后答案网x+1[y+(y�x)]2O+1[y+(y�x)]K+1KK.1+1+1+1+1+1+SinceO+1[y+(y�x)]isanopenneighbourhoodofycontainedinK,yisaninteriorpointof1+1+K.*44e.LetXbeatopologicalvectorspacethatisofsecondBairecategorywithrespecttoitself.SupposeaclosedconvexsubsetKofXhasaninternalpointy.LetXn=fx2X:y+tx2Cforallt2[0;1=n]g.EachXnisaclosedsubsetofXsinceadditionandscalarmultiplicationarecontinuousandCisclosed.Swww.hackshp.cnNowX=XnsosomeXnhasaninteriorpointx.Thenx2OXnforsomeopensetO.Thusy+1x2y+1OCsoy+1xisaninteriorpointofC.nnn(*)Assumptionofconvexitynotnecessary?45.LetKbeaconvexsetcontainingandsupposethatxisaninternalpointofK.Sincexisaninternalpoint,thereexists">0suchthatx+x2Kforjj<".Choosesuchthat0<<1and(1�)�1x2K.Notethat0<1�<1.Fory2K,x+y=(1�)[(1�)�1x]+y2KsinceKisconvex.46.LetNbeafamilyofconvexsets(containing)inavectorspaceX.SupposeNsatis
es(i),(ii)and(iii).ToshowthatthetranslatesofsetsinNformabaseforatopologythatmakesXintoalocallyconvextopologicalvectorspace,itsucestoshowthatNisabaseat.IfN1;N22N,thereisanN32NwithN3N1N2.Thuscondition(i)ofProposition14issatis
ed.IfN2Nandx2N,thenxisinternal.ByQ45,thereexistswith0<<1andx+NN.NotethatN2N.Thuscondition(ii)ofProposition14issatis
ed.IfN2N,then1N+1NN.Notethat1N2N.222Thuscondition(iii)ofProposition14issatis
ed.IfN2Nandx2X,thenx2Nforsome2Rsince2Nandisinternal.Nowx21Nsocondition(iv)ofProposition14issatis
ed.IfN2Nand00.Lety=(sgnx)(jxj�jxj)andii121112y2=2x2.Also,letz1=x1+(sgnx1)jx2jandz2=0.Theny1+z1=2x1andy2+z2=2x2.Also,jyj+jyj=jxj+jxjandjzj+jzjjxj+jxj.Fori>2,de
ney=z=x.Thenhxi=1(hyi+hzi)12121212iiii2iiwithhyi;hzi2S.Thustheunitspherein`1hasnoextremepoints.iiLethxi2`1withjxj=1foralli.If1=x=y+(1�)zwithjyj1,jzj1and0<<1,ii课后答案网iiiiithenyi=zi=1.Similarly,if�1=xi=yi+(1�)ziwithjyij1,jzij1and0<<1,theny=z=�1.Thushxiisanextremepointoftheunitspherein`1.Ifjxj<1forsomeN,thende
neiiiNy=x+1�jxijandz=x�1�jxijforallisothathxi=1(hyi+hzi).Furthermore,hyi;hzi2S.ii2ii2i2iiiiHencetheextremepointsoftheunitspherein`1arethosehxiwithjxj=1foralli.ii*48f.Theconstantfunctions1areextremepointsoftheunitsphereinC(X)whereXisacompactHausdor
space.Letf2Cwww.hackshp.cn(X)withjjfjj=1andsupposejf(x0)j<1forsomex02X.Fix">0suchthat00,thereexistsNsuchthatforallnNwehavemft:70khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
R1jxn(t)�x(t)jRjRxn(t)�x(t)j"=2g<"=2.ThenfornN,(xn;x)=01+jxn(t)�x(t)jdtft:jxn(t)�x(t)j"=2g1dt+ft:jxn(t)�x(t)j<"=2gjxn(t)�x(t)jdt<".Thusxn!xinthemetric.Conversely,supposehxnidoesnotconvergetoxinmeasure.Thereexists">0suchthatforallNthereisnNwithmft:jxn(t)�x(t)j"g".Thusthereisasubsequencehxnkisuchthatmft:jxnk(t)�x(t)j"g"R1jxnk(t)�x(t)jRjxnk(t)�x(t)j"2forallk.Now(xnk;x)=01+jxn(t)�x(t)jdtfjxn�xj"g1+jxn(t)�x(t)jdt1+"forallksothekkksubsequencehxnkidoesnotconvergetoxinthemetric.Hencehxnidoesnotconvergetoxinthemetric.49c.LethxnibeCauchyinthemetric.SupposehxniisnotCauchyinmeasure.Thereexists">0suchthatforallNthereisn;mNwithmft:jxn(t)�xm(t)j"g".Thusthereisasubsequencehxnkisuchthatmft:jxn2k�1(t)�xn2k(t)j"g"forallk.Now(xn2k�1;xn2k)=R1jxn2k�1(t)�xn2k(t)jRjxn2k�1(t)�xn2k(t)j"2dtdtforallksothesubsequence01+jxn2k�1(t)�xn2k(t)jfjxn2k�1�xn2kj"g1+jxn2k�1(t)�xn2k(t)j1+"hxnkiisnotCauchyinthemetric.Contradiction.HencehxniisCauchyinmeasure.ByQ4.25,hxniconvergesinmeasureandbypart(b),itconvergesinthemetric.HenceXisacompletemetricspace.49d.Given">0,let="=2.When
(hx;yi;hx0;y0i)<,wehave(x;x0)<and(y;y0)<.Now(x+khdaw.comy;x0+y0)=(x�x0+y�y0)(x�x0)+(y�y0)=(x;x0)+(y;y0)<".HenceadditionisacontinuousmappingofXXintoX.49e.Givena2R,x2Xand">0,byQ3.23a,thereexistsMsuchthatjxjMexceptonasetofmeasurelessthan"=3.Let=min(1;"=3(jaj+1);"=3M).When
(ha;xi;hc;x0i)<,wehavejc�aj<00R1jax(t)�cx0(t)jR1ja�cjjx(t)jR1jcjjx(t)�x0(t)jand(x;x)<.Now(ax;cx)=01+jax(t)�cx0(t)jdt01+ja�cjjx(t)jdt+01+jcjjx(t)�x0(t)jdt0,thereisastepfunctionssuchthatjx�sj<"=2exceptonasetofR1jx(t)�s(t)jmeasurelessthan"=2.i.e.mft:jx(t)�s(t)j"=2g<"=2.Now(x;s)=dt=01+jx(t)�s(t)jRjx(t)�s(t)jRjx(t)�s(t)jR1Rdt+dt<"=2dt+1dt<".Hencethefjx�sj<"=2g1+jx(t)�s(t)jfjx�sj"=2g1+jx(t)�s(t)j0fjx�sj"=2gsetofstepfunctionsisdenseinX.*49g.49h.Byparts(d)and(e),Xisatopologicalvectorspace.SincetherearenononzerocontinuouslinearfunctionalsonXbypart(g),Xcannotbelocallyconvex.P2�vjvj49i.Letsbethespaceofallsequencesofrealnumbersandde
ne课后答案网(hvi)=1+jvj.Analoguesofparts(a)and(c)followfromQ7.24.Theanalogueofpart(d)followsfromthesameargumentasabove.Fortheanalogueofpart(e),supposea2R,hvi2sand">0aregiven.Let=min(1;"=2jaj;"=2((hvi)+1)).When
(ha;hvii;hc;hvii)<,wehavejc�aj<andP�vP2�vjajjP�v2jav�cvjv�vj2ja�cjjvj(hvi�hvi)<.Now(ahvi�chvi)=1+jav�cvj1+jajjv�vj+1+ja�cjjvj=jaj(hvi�hvi)+ja�cj(hvi)N.Thusf(hvi)=v=1vf(ev).10.8Hilbertspace50.Supposexn!xandyn!y.ThenthereexistsMsuchthatjjxnjjMforalln.Given">0,chooseNsuchthatjjyn�yjj<"=2Mandjjxn�xjj<"=2jjyjjfornN.Nowj(xn;yn)�(x;y)jj(xn;yn�y)j+j(xn�x;y)jjjxnjjjjyn�yjj+jjxn�xjjjjyjj<"fornN.Hence(xn;yn)!(x;y).*51a.Theuseoftrigonometricidentitiesshowsthatfp1;cospvt;sinpvtgisanorthonormalsystemfor2L2[0;2].Supposex2L2[0;2]suchthat(x;cospvt)=0and(x;sinpvt)=0forallv.Let">0.ByPropo-PNPNsition6.8andQ9.42,thereisa
niteFourierseriesP"=a0+nP=1ancosnt+n=1bvPsinntsuchthatjjx�"jj<".Nowjj"jj2=(";")=2a2+N(a2+b2)andj(";")j2=2a2+N((pan)2+0n=1nnvv0n=1(pbn)2)sojj"jj2=Pj(";")j2.ThusthereexistsMsuchthatjjj"jj2�Pnj(";")j2j<"2vvkhdaw.comv=�nv71若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
Pn22Pn22forPnnM.NowjjP"n�v=�n(";"v)"vjjPn=jj"jj�v=�nj(";"v)j<"fornM.Also,jj(";")"�(x;")"jj2=j("�x;")j2jj"�xjj2<"2fornM.Thusv=P�nnvvv=�nvvPnv=�nvPnjjx�v=�Pn(x;"v)"vjjjjx�"jj+jjP"�v=�n(";"v)"vjj+jjv=�n("�x;"v)"vjj<3"fornM.Hencex=(x;")"andjjxjj2=j(x;")j2=0sox=0.Hencefp1;cospvt;sinpvtgisacompletevvvvv2orthonormalsystemforL2[0;2].51b.Bypart(a)andProposition27,everyfunctioninL2[0;2]isthelimitinmean(oforder2)ofitsFourierseries.i.e.theFourierseriesconvergestothefunctioninL2[0;2].S52a.Letx2Handletf"vgbeanorthonormalsystem.NotethatPfv:av6=0g=nfv:av1=ng.ByBessel"sinequality,wehavejaj2jjxjj2<1sofv:a1=ngis
niteforeachn.Hencevvvfv:av6=0giscountable.*52b.LetHbeaHilbertspaceandf"vgacompleteorthonormalsystem.Bypart(a),onlycountablymanyFouriercoecientsPavarenonzerosowemaylistthemasasequencePhavi.ByBessel"sinequality,jaj2jjxjj2<1sogiven">0,thereexistsNsuchthat1jaj2<"2.Form>nN,wevvPPv=N+1vhavejjma"jj2=mja2<"2sothesequenceofpartialsumsisCauchyinHandthusconvergesv=Pnvvv=nvPPinHP.i.e.vav"v2H.Now(x�vav"v;"v)=(x;"v)�av=0forallvsox�vav"v=0.i.e.x=khdaw.comvav"v.PIfacompleteorthonormalsysteminHiscountable,sayh"vi,thenx=vav"vandxisaclusterpointofthesetoflinearcombinationsof"v,whichcontainsthecountabledensesetoflinearcombinationsof"vwithrationalcoecientssoHisseparable.Thusacompleteorthonormalsysteminanon-separableHilbertspaceisuncountable.*52c.LetfbeaboundedlinearfunctionalonH.LetK=kerf.Sincefiscontinuous,KisaclosedlinearsubspaceofH.WemayassumeK?6=f0gsothereexistsx2K?withf(x)=1.De
ne00y=x=jjxjj2.Then0=(x�f(x)x;x)=(x;x)�f(x)jjxjj2forallx2H.i.e.f(x)=(x;y)forall000000x2H.Ify02Hsuchthat(x;y)=(x;y0)forallx2H,theny�y02H?.Inparticular,jjy�y0jj2=(y�y0;y�y0)=0soy=y0.Thisprovestheuniquenessofy.Sincejf(x)j=j(x;y)jjjxjjjjyjj,wehavejjfjjjjyjj.Furthermore,sincef(y=jjyjj)=(y=jjyjj;y)=jjyjj,wehavejjfjj=jjyjj.52d.LetHbeanin
nitedimensionalHilbertspace.Iff"vgisacompleteorthonormalsysteminH,thenthesetof
nitelinearcombinationsof"visadensesubsetofH.Nowifjf"vgj=n,thenjf
nitelinearcombinationsof"vgj=n@0=nsothereisadensesubsetofHwithcardinalitypn.IfSisadensesubsetofH,thenforeach"v,thereexistsxvp2Swithpjjxv�p"vjj<1=2.Ifv6=u,thenjjxv�xujjjj"v�课后答案网"ujj�jjxv�"vjj�jjxu�"ujj>2�1=2�1=2=0soxv6=xu.ThusjSjjf"vgj.HencethenumberofelementsinacompleteorthonormalsysteminHisthesmallestcardinalnsuchthatthereisadensesubsetofHwithnelements.Furthermore,thisprovesthateverycompleteorthonormalsysteminHhasthesamenumberofelements.*52e.SupposetwoHilbertspacesHandH0areisomorphicwithanisomorphism:H!H0.Iff"gisacompleteorthonormalsysteminH,thenf(")gisacompleteorthonormalsysteminH0.vvSimilarlyfor�1.Thusdimwww.hackshp.cnH=dimH0.Conversely,supposedimH=dimH0.LetEbeacompletePorthonormalsysteminH.ConsidertheHilbertspace`2(E)=f(f:E!R):jf(e)j2<1g.IfPPe2Ex2H,de
ne^x:E!Rby^x(e)=(x;e).Thenjx^(e)j2=j(x;e)j2=jjxjj2<1so^x2`2(E).e2Ee2EFurthermore,jjxjj=jjx^jj.De
ne:H!`2(E)by(x)=^x.Thenisalinearisometry.Nowtherangeofcontainsfunctionsfsuchthatf(e)=0forallbut
nitelymanye2Eandisclosedsinceisanisometry.Henceisanisomorphism.IfFisacompleteorthonormalsysteminH0,thenjEj=jFjso`2(E)and`2(F)mustbeisomorphic.HenceHandH0areisomorphic.P*52f.LetAbeanonemptysetandlet`2(A)=f(f:A!R):jf(a)j2<1g.Then`2(A)isaPa2AHilbertspacewith(f;g)=a2Af(a)g(a).Foreacha2A,let abethecharacteristicfunctionoffag.Then 2`2(A)foreacha2A.Furthermoref :a2Agisacompleteorthonormalsystemin`2(A).aaThusdim`2(A)=jf :a2Agj=jAj.HencethereisaHilbertspaceofeachdimension.a53a.LetPbeasubsetofH.Supposey2P?andy!y.Thenforeachx2P,wehavenn(x;y)!(x;y).Since(x;y)=0foreachn,wehave(x;y)=0soy2P?.ThusP?isclosed.Ifnna;b2Randy;z2P?,thenforeachx2P,wehave(ay+bz;x)=a(y;x)+b(z;x)=0soay+bz2P?andP?isalinearmanifold.*53b.Ifx2P,then(x;y)=0forally2P?.ThusP??isaclosedlinearmanifoldcontainingP.SupposePQwhereQisaclosedlinearmanifold.ThenQ?P?andP??Q??.Now72khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
ifQisapropersubsetofQ??,pickx2Q??nQ.Thenthereexistsy2Qsuchthatjjx�yjj=00inffjjx�yjj:y2Qg=.Letz=x�y.Foranya2Randy2Q,wehave2jjz�ayjj2=011jjzjj2�2a(z;y)+a2jjyjj2=2�2a(z;y)+a2jjyjj2soa2jjyjj2�2a(z;y)0.Thus4(z;y)201111111anditfollowsthat(z;y)=0.i.e.z?Q.Thusz2Q??Q?soz=0i.e.x=y2Q.Contradiction.10HenceQ=Q??soP??Q.53c.LetMbeaclosedlinearmanifold.Givenx2H,thereexistsy2Msuchthatx�y?M.Thenx=y+zwherez=x�y2M?.Ifx=y+z=y0+z0wherey;y02Mandz;z02M?,theny�y0=z�z0soy�y0;z�z02MM?.Hencey=y0andz=z0.Furthermorejjxjj2=(y+z;y+z)=jjyjj2+jjzjj2+2(y;z)=jjyjj2+jjzjj2sincez?y.54.LethxnibeaboundedsequenceofelementsinaseparableHilbertspaceH.SupposejjxnjjMforallnandleth"nibeacompleteorthonormalsysteminH.Nowj(xn;"1)jMforallnsothereisasub-sequenceh(xnk;"1)ithatconverges.Thenj(xnk;"2)jMforallksothereisasubsequenceh(xnkl;"2)ithatconverges.Furthermore,h(xnk;"1)ialsoconverges.Continuingtheprocess,weobtainthediagonallsequencehxnnisuchthath(xnn;"k)iconvergesforanyk.ForanyboundedlinearfunctionalPfonH,thereisauniquePy2HsuchthatPf(x)=(x;y)forallx2H.Furthermore,y=k(y;"k)"k.Now(xnn;ykhdaw.com)=(xnn;k(y;"k)"k)=k(xnn;"k)(y;"k).Sinceh(xnn;"k)iconvergesforeachk,h(xnn;y)iconverges.i.e.hxnihasasubsequencewhichconvergesweakly.55.LetSbeasubspaceofL2[0;1]andsupposethatthereisaconstantKsuchthatjf(x)jKjjfjjforallx2P[0n;1].Ifhf1;:::;fniPisany
niteorthonormalsequenceinnPnPnS,thenforanyPna1;:::;an2R,wehave(af(x))2K2jjafjj2=K2(af;af)=K2a2forallx2[0;1].i=1iii=1iii=1Piii=1iiPi=1iPFixx2[0;1].Foreachi,leta=pPfi(x).Then(naf(x))2=nf(x)2andna2=1.inf2i=1iii=1ii=1ii(x)i=1RRThusPnf(x)2K2forallx2[0;1]andn=Pnjjfjj2=1(Pnf(x)2)1K2=K2.Hencei=1ii=1i0i=1i0thedimensionofSisatmostK2.11MeasureandIntegration11.1MeasurespacesSk�11.LetfAngbeacollectionofmeasurablesets.LetB1=A1andBSk=AkSnn=1AnforSk>1.ThenSfBngPisacollectionofpairwisedisjointmeasurablesetssuchthat课后答案网PnSnSnAn=Bn.Now(Ak)=(Bk)=(Bk)=limnk=1(Bk)=limn(k=1Bk)=limn(k=1Ak).2a.Letf(XS;B;)gbeacollectionofmeasurespaces,andsupposethatthesetsPfXgaredisjoint.De
neX=X,B=fB:8
BX2Bgand(B)=(BX).Now;2Bsince;X=;2Bforall.IfB2B,thenBX2BforallsoBcX=Xn(BX)2Bforall.ThusBc2B.IfhBiisasequenceinB,thenforeachn,BX2BforallsoSSnSnBnX=(BnX)2Bforeach.ThusBn2B.HenceBisa-algebra.Pwww.hackshp.cnP2b.S(;)=PS(;X)=PP(;)=0.ForanysequenceofdisjointsetsPPPBi2B,wehave(Bi)=(BiX)=i(BiX)=i(BiX)=i(Bi).Henceisameasure.2c.Supposethatallbutacountablenumberofthearezeroandtheremainderare-
nite.LetShnibethecountablymanythatarenonzeroand-
nite.Thenforeachn,Xn=kXn;kwhereXn;k2SBnandSn(Xn;k)0giscountable.ThusPnf:(YnX)>0giscountable.Foreach,(X)=(XnYn)=n(XYn).If(X)>0,then(XYn)>0forsomen.HenceSf:(X)>0giscountable.i.e.allbutacountablenumberoftheParezero.Furthermore,X=n(XYn)whereXYn2Band(XYn)(XYn)=(Yn)<1foreachn.Hencetheremainingare-
nite.73khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
(*)Unionofmeasurespaces3a.SupposeE1;E22Band(E1E2)=0.Then(E1nE2)=(E2nE1)=0.Hence(E1)=(E1nE2)+(E1E2)=(E2nE1)+(E1E2)=(E2).3b.Supposeiscomplete,E12Band(E1E2)=0.ThenE2nE12BsinceE2nE1E1E2.Also,E1E2=E1n(E1nE2)2B.HenceE2=(E2nE1)[(E1E2)2B.4.Let(X;B;)beameasurespaceandY2B.LetBYconsistofthosesetsofBthatarecontainedinY.SetYE=EifE2BY.Clearly;2BY.IfB2BY,thenB2BandBY.ThusYnB2BandYSnBYsoYSnB2BY.IfShBniisasequenceofsetsinBY,thenBn2BandBnYforalln.ThusBn2BandBnYsoSBn2BYS.Furthermore,PY(;)=P(;)=0andifhBniisasequenceofdisjointsetsinBY,thenY(Bn)=(Bn)=(Bn)=Y(Bn).Hence(Y;BY;Y)isameasurespace.(*)Restrictionofmeasuretomeasurablesubset5a.Let(X;B)beameasurablespace.Supposeandaremeasuresde
nedonBandde
nethesetfunctionE=E+EonB.Then(;)=(;)+(;)=0.Also,ifhEniisasequenceofdisjointsetsSSSPPPinB,then(En)=(En)+(En)=En+En=En.Henceisalsoameasure.*5b.khdaw.comSupposeandaremeasuresonBand.Notethat�isameasurewhenrestrictedtomeasurablesetswith
nite-measure.De
ne(E)=supFE;(F)<1((F)�(F)).Clearly(;)=0.IfE1;E22BwithE1E2=;,thenforanyFE1[E2with(F)<1,wehave(F)�(F)=(�)(FE1)+(�)(FE2)(E1)+(E2).Thus(E1[E2)(E1)+(E2).Ontheotherhand,ifF1E1andF2E2with(F1);(F2)<1,wehave(�)(F1)+(�)(F2)=(�)(F1[F2)(E1[E2).Thus(E1)+(E2)(E1[E2).Henceis
nitelyadditive.SNowsupposePhEniisasequenceofdisjointsetsinPSBP.IfFEnwith(F)<1,then(�)(F)=(�)(FEn)(En).Thus(En)(En).Ontheotherhand,foranyN,wehavePNSNSPSn=1(En)=(n=1En)(En).ThusEn(En).Henceiscountablyadditive.If(E)=1,then(E)=1so(E)+(E)=(E)andif(E)<1,then(E)+(E)=(E)+((E)�(E))=(E).Hence=+.5c.Suppose=+forsomemeasure.Ifis
nite,then=�=.Supposeis-S
nite.ThenX=XnwhereXn2Band(Xn)<1foreachn.WemayassumethattheXnaredisjoint.NowforanysetSPE2B,wehaveP(EXn)=S(EXn)since(EXn)<1.Then(E)=((EXn))=课后答案网(EXn)=(EXn)=((EXn))=(E).Henceinpart(b)istheuniquesuchmeasure.*5d.If=+=+0,then(F)=0(F)foranysetFwith(F)<1.GivenE2B,foranyFEwith(F)<1,wehave(F)�(F)=(F)=0(F)0(E).Hence(E)0(E)andinpart(b)isthesmallestsuchmeasure.S6a.Letbea-
nitemeasuresoX=Xnwhere(Xn)<1foreachn.ForanymeasurablesetESSkofin
nitemeasure,wehave1=((EXn))=limk(n=1(EXn)).ThusforanyN,thereexistsSkwww.hackshp.cnksuchthatN<(n=1(EXn))<1.Henceissemi
nite.6b.Givenameasure,de
ne1(E)=supFE;(F)<1(SF).Then1(;)=(;)=0.IfhEniisasequenceofdisjointmeasurablesets,thenforanyFEnwith(F)<1,wehave(F)=PPSP(FEn)1(En).Thus1(En)1(En).Conversely,ifF1E1andF2E2with(F1);(F2)<1,then(F1)+(F2)=(F1[F2)1(E1[E2)so1(E1)+1(E2)1(E1[E2).ItPkSkSPSfollowsthatforanyk,n=11(En)1(n=1En)1(En).Thus1(En)1(En).Hence1isameasure.Furthermore,if1(E)=1,thenforanyN,thereexistsFEwithN<(F)<1.ThenN<1(F)<1.Hence1issemi
nite.Nowde
ne2(E)=supFE;1(F)<1((F)�1(F)).Then2isameasure(c.f.Q5b).If(F)<1forallFEwith1(F)<1,then(F)=1(F)forallFEwith1(F)<1so2(E)=0.If(F)=1forsomeFEwith1(F)<1,then(F)�1(F)=1so2(E)=1.Hence2onlyassumesthevalues0and1.*6c.7.Givenameasurespace(X;B;),letB0bethecollectionofsetsA[BwhereA2BandBCforsomeC2Bwith(C)=0.Clearly;2B0.IfhEniisasequenceinB0,thenEn=An[Bnforeach74khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
SSSSnwhereAn2BSandBSnCnforsomeSCn2Bwith(Cn)=0.NowEn=An[BnwhereAn2BandBnCnwith(Cn)=0.IfE2B0,thenE=A[BwhereA2BandBCforsomeC2Bwith(C)=0.ThenEc=AcBc=Ac(Cn(CnB))c=Ac(Cc[(CnB))=(AcCc)[(Ac(CnB))whereAcCc2BandAc(CnB)Cwith(C)=0.HenceBisa0-algebra.FurthermoreBB0.IfE2BwithE=A[B=A0[B0withA;A02B,BCandB0C0forsomeC;C02B0with(C)=(C0)=0,thenA[B[C[C0=A0[B0[C[C0soA[C[C0=A0[C[C0.Now(A)(A[C[C0)(A)+(C)+(C0)=(A).Thus(A)=(A[C[C0).Similarly,(A0)=(A0[C[C0).Hence(A)=(A0).ForE2B0,de
ne0(E)=S(A).ThenSS0iswell-de
ned.Clearly,SS0(;)=0.IfPhEniPisasequenceofdisjointsetsinB0,thenEn=An[Bnso0(En)=(An)=(An)=0(En).Thus0isameasure.Furthermore,ifE2B,then0(E)=(E).If0(A[B)=0andEA[B,thenE=A[(EA)whereEABCwith(C)=0soE2B0.Hence(X;B0;0)isacompletemeasurespaceextending(X;B;).8a.Letbea-
nitemeasure.IfEislocallymeasurable,thenEB2BforeachB2BwithSS(B)khdaw.com<1.NowX=XnwithXn2Band(Xn)<1foralln.ThusE=(EXn)whereEXn2Bforalln.ThusE2B.i.e.Eismeasurable.Henceissaturated.8b.LetCbethecollectionoflocallymeasurablesets.Clearly;2C.IfC2C,thenCB2BforeachB2Bwith(B)<1.NowforanysuchsetB,CcB=Bn(CB)2B.ThusCc2C.IfhCinisasequenceofsetsinC,thenCnB2BforeachB2Bwith(B)<1.NowforanysuchsetB,SSSCnB=(CnB)2B.ThusCn2C.HenceCisa-algebra.8c.Let(X;B;)beameasurespaceandCthe-algebraoflocallymeasurablesets.ForE2C,setE=EifE2BandE=1ifE=2B.Clearly(;)=0.IfhEniisasequenceofdisjointsetsinSSPC,thenweconsiderafewcases.IfSEn2=SB,then(EnS)=1.Furthermore,En2=BSforsomenso(En)=S1=(ESn).NowsupposePEPn2B.If(SEn)<1,thenEn=EnEn2BforPeachnso(PEn)=(ESn)=(En)=S(En).If(En)=1,theneitherPEn2BforallSn,so(En)=(En)=(En)=1=(En),orEn2=Bforsomen,so(En)=1=(En).HenceisameasureonC.LetEbealocallymeasurablesetin(X;C;).ThenEC2CforanyC2Cwith(C)<1.i.e.EC2CforanyC2Bwith(C)<1.ThusEC2BforanyC2Bwith(C)<1andEisalocallymeasurablesetin(课后答案网X;B;)soE2C.Hence(X;C;)isasaturatedmeasurespace.*8d.Supposeissemi
niteandE2C.Set(E)=supf(B):B2B;BEg.Clearly(;)=0.SIfhEniisasequenceofdisjointsetsinSPC,foranyPB2BwithBEn,provided(B)<1,wehaveS(B)=((BESn))=P(BEn)(En).Ontheotherhand,if(B)=1,then(En)=1.Thus(En)(En).IfE1;E22CwithE1E2=;andB1;B22BwithB1E1andB2E2,then(E1)+(E2)(B1)+(B2)=(B1[B2)(E1[E2).NowforanyPNSNSPSN,wehaven=1(En)www.hackshp.cn(n=1En)(En).Thus(En)(En).HenceisameasureonC.LetEbealocallymeasurablesetin(X;C;).ThenEC2CforanyC2Cwith(C)<1.IfB2Bwith(B)<1,thenB2Cwith(B)=(B)<1.ThusEB2C.ItfollowsthatEB2BsoE2C.Hence(X;C;)isasaturatedmeasurespace.Furthermore,isanextensionof.9a.LetRbea-ringthatisnota-algebra.LetBbethesmallest-algebracontainingRandsetR0=fE:Ec2Rg.Notethat;2RR[R0.IfA2R[R0,theneitherA2RsoAc2R0R[R0SSSorA2R0soAc2RR[R0.IfhAiisasequenceinR[R0,thenA=fA:A2Rg[fA:nnnnnA2R0g2R[R0.IfAisa-algebracontainingR,thenAalsocontainsR0.ThusAR[R0.HencenR[R0=B.Furthermore,ifE2RR0,thenE;Ec2RsoX=E[Ec2RandRisa-algebra.Contradiction.HenceRR0=;.9b.IfisameasureonR,de
neonBby(E)=(E)ifE2Rand(E)=1ifE2R0.Clearly(;)=(;)=0.LethEibeasequenceofdisjointsetsinB.IfE2RandE2R0,then(E2)c2Rn12so(E)c(E)c=(E)cnE2R.ThusE[E2R0so(E[E)=1=(E)+(E).IfE2R12S21121S212nforalln,thenE2R.Similarly,ifE2R0foralln,thenE2R0.InbothcaseswehaveSPnSnSnSS(E)=(E).Thusingeneral,(E)=(fE:E2Rg[fE:E2R0g)=(fE:nSnPnPnnPnnnE2Rg)+(fE:E2R0g)=(E)+(E).HenceisameasurennnEn2RnEn2R0(En)=khdaw.comn75若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
onB.9c.De
neonBby(E)=(E)ifE2Rand(E)=supf(A):AE;A2RgifE2R0.Clearly(;)=(;)=0.LethEibeasequenceofdisjointsetsinB.NotethatifE2RforallnorE2R0SnPSPnnforalln,then(En)=(En).Itfollowsthatingeneral(En)=(En).HenceisameasureonB.*9d.*9e.11.2Measurablefunctions10.Letfbeanonnegativemeasurablefunction.Foreachpairhn;kiofintegers,setE=fx:k2�nn;kP22n�n�n2n�nf(x)<(k+1)2gandset"n=2k=0k En;k+(2+1)2 ff(22n+1)2�ng.TheneachEn;kismeasurableandeach"nisasimplefunction.NotethatEn;k=En+1;2k[En+1;2k+1forallhn;ki.Supposex2E.Then"(x)=k2�n.Ifx2E,then"(x)=(2k)2�(n+1)=k2�n="(x).n;knn+1;2kn+1nIfx2E,then"(x)=(2k+1)2�(n+1)>(2k)2�(n+1)"(x).Also,iff(x)(22n+1)2�n,n+1;2k+1n+1nthen"(x)=(22n+1)2�n"(x).Thus"".Forx2X,iff(x)<1,thenforsucientlykhdaw.comnn+1nn+1largen,f(x)�"(x)2�n.Iff(x)=1,then"(x)=(22n+1)2�n>2n!1.Thusf=lim"atnnneachpointofX.SIffisde
nedona-
nitemeasurespace(X;B;),thenX=Xnwhere(Xn)<1foreachn.P22n�nS2n�nSDe
ne"n==2k=0k En;knXm+(2+1)2 ff(22n+1)2�ngnXm.Then"n+1"nm=1Snm=1andf=lim"n.Furthermore,each"nvanishesoutsidem=1Xm,asetof
nitemeasure.(*)ProofofProposition711.Supposeisacompletemeasureandfbeameasurablefunction.Supposef=ga.e.Thenfx:g(x)<
g=fx:f=g;f(x)<
g[fx:f6=g;g(x)<
g=(fx:f(x)<
gnfx:f6=g;f(x)1=2g=Abutm(A)=0so课后答案网 A=0a.e.andtheconstantzerofunctionismeasurable.(*)ProofofProposition812.LethfnibeasequenceofmeasurablefunctionsthatconvergetoafunctionfexceptatthepointsofasetEofmeasurezero.Supposeiscomplete.Given,fx:f(x)>
g=fx=2E:limfn(x)>
g[fx2E:f(x)>
g.Since(E)=0,fx2E:f(x)>
gismeasurable.Also,fx=2E:limfn(x)>
g=Ecfx:limf(x)>
gsoitismeasurable.Hencefx:f(x)>
gismeasurableandfismeasurable.nNote:Iffn(x)!f(x)forallwww.hackshp.cnx,thencompletenessisnotrequired.13a.Lethfnibeasequenceofmeasurablereal-valuedfunctionsthatconvergetofinmeasure.Foranyk,thereexistsnandameasurablesetEwith(E)<2�ksuchthatjf(x)�f(x)j<2�kkS1kknkTS1forx=2E.Ifx=2E,thenjf(x)�f(x)j<2�kforkm.Thusifx=2E,kk=mknkmk=mkthenforsomem,wehavejf(x)�f(x)j<2�kforkmsohficonvergestof.Furthermore,TS1S1nkP1P1nkTS1(E)(E)(E)<2�k=2�m+1forallmso(E)=mk=mkk=mkk=mkk=mmk=mk0andhfnkiconvergestofa.e.(c.f.Proposition4.18)13b.Lethfnibeasequenceofmeasurablefunctionseachofwhichvanishesoutsidea
xedmeasurablesetAwith(A)<1.Supposethatfn(x)!f(x)foralmostallx,sayexceptonasetBofmeasurezero.Ifx2AcnB,thenf(x)=0forallnsof(x)=0.Given">0,letG=fx2AnB:jf(x)�f(x)j"gandSnnn1letEN=n=NGn.NotethatEN+1EN.IfTx2AnB,thenthereexistsNsuchthatjfn(x)�f(x)j<"fornN.i.e.x=2ENforsomeN.ThusEN=;andlim(EN)=0sothereexistsNsuchthat(E)<".Furthermore,(E[B)<"andifx=2E[B,theneitherx2Aandx=2GcforallNNNnnNsojfn(x)�f(x)j<"forallnNorx=2Asojfn(x)�f(x)j=0foralln.Hencehfniconvergestofinmeasure.76khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
13c.LethfnibeasequencethatisCauchyinmeasure.Wemaychoosenk+1>nksuchthatSfx:jf(x)�f(x)j2�kg<2�k.LetE=fx:jf(x)�f(x)j2�kgandletF=1E.nk+1TnkS1knTk+1nkTmk=mkThen(F)(E)2�k+1forallkso(F)=0.Ifx=2F,thenx=2Fforsomemmk=mkmmmsojf(x)�f(x)j<2�kforallkmandjf(x)�f(x)j<2�k+1forlkm.ThustheseriesPnk+1nknlnk(fnk+1�fnk)convergesa.e.toafunctiong.Letf=g+fn1.Thenfnk!finmeasuresincethepartialsumsareoftheformfnk�fn1.Given">0,chooseNsuchthatfx:jfn(x)�fm(x)j"=2g<"=2forn>mNandfx:jfnk(x)�f(x)j"=2g<"=2forkN.Thenfx:jfn(x)�f(x)j"gfx:jfn(x)�fnk(x)j"=2g[fx:jfnk(x)�f(x)j"=2gforalln;kN.Thusfx:jfn(x)�f(x)j"g<"forallnNandhfniconvergestofinmeasure.(c.f.Q4.25)14.Let(X;B;)beameasurespaceand(X;B0;0)itscompletion.SupposegismeasurablewithrespecttoBandthereisasetE2Bwith(E)=0andf=gonXnE.Forany,fx:g(x)<
g2B.Nowfx:f(x)<
g=fx2XnE:g(x)<
g[fx2E:f(x)<
gwherefx2XnE:g(x)<
g2Bandfx2E:f(x)<
gE.Thusfx:f(x)<
g2B0andfismeasurablewithrespecttoB0.Fortheconverse,
rstconsiderthecaseofcharacteristicfunctions.Suppose AismeasurablewithrespecttoB.ThenA2BsoA=A0[B0whereA02BandB0C0withC02Band(C0)=0.00De
nekhdaw.comf(x)= (x)ifx=2C0andf(x)=1ifx2C0.If
<0,thenfx:f(x)>
g=X.If1,Athenfx:f(x)>
g=;.If0
<1,thenfx:f(x)>
g=fx:f(x)=1g=A[C0=A0[C0.Thusfx:f(x)>
g2BforallandfismeasurablewithrespecttoB.NextconsiderthecasePnofsimplefunctions.Supposeg=i=1ci AiismeasurablewithrespecttoB0.Theneach AiismeasurablewithrespecttoB0.Foreachi,thereisafunctionhimeasurablewithrespecttoPSBandnnSasetnEi2BwithS(Eni)=0and Ai=hionXPnnEi.Theng=i=1cihionXni=1Eiwherei=1Ei2Band(i=1Ei)=0.Furthermore,i=1cihiismeasurablewithrespecttoB.Nowforanonnegativemeasurablefunctionf,thereisasequenceofsimplefunctionsh"niconvergingpointwisetofonX.Foreachn,thereisafunctionnmeasurablewithrespecttoSSBandasetEnS2Bwith(En)=0and"n=nonXnEn.Thenf=limnonXnEnwhereEn2Band(En)=0.Furthermore,limnismeasurablewithrespecttoB.Finally,forgeneralameasurablefunctionf,wehavef=f+�f�wheref+andf�arenonnegativemeasurablefunctionsandtheresultfollowsfromthepreviouscase.15.LetDbetherationals.Supposethattoeach2DthereisassignedaB2BsuchthatBBfor
<
.Thenthereisauniquemeasurablefunction课后答案网SfonXsuchthatTSfonBandfonXnB.NowTfx:f(x)<
g=
<
BandTfx:f(x)Tg=
>
<
B.Similarly,fTx:fS(x)>
g=TXn
>
TBandfx:f(x)g=
<
(Xn
>
B).Also,fx:f(x)=g=
>
<
B
<
(Xn
>
B).16.Egoro
"sTheorem:Lethfnibeasequenceofmeasurablefunctionseachofwhichvanishesoutsidea
xedmeasurablesetAof
nitemeasure.Supposethatfn(x)!f(x)foralmostallx.ByQ13b,hfniconvergestofinmeasure.Given">0andn,thereexistNandameasurablesetEwith(E)<"2�nnSSnPnsuchthatjfn(x)�f(x)j<1www.hackshp.cn=nfornNnandx=2En.LetE=En.Then(En)(En)<".Choosen0suchthat1=n0<".Ifx=2EandnNn0,wehavejfn(x)�f(x)j<1=n0<".ThusfnconvergesuniformlytofonXnE.11.3Integration17.LetfandgbemeasurablefunctionsandEameasurableset.R(i)Foraconstantc,notethatifc0,then(cf)+=cf+and(cf)�=cf�socf=RR1RR1R1111E1cf+�cf�=cf+�cf�=cf.Ontheotherhand,ifc<0,then(cf)+=�cf�E1E11RE1RE1ERR1R1R1and(cf)�=�cf+socf=(�cf�)�(�cf+)=�cf��(�c)f+=cf.Note11E1E1E11E1E1Ethatiff=f�fwheref;farenonnegativeintegrablefunctions,thenf++f=f�+fsoRR1R2R1R2RRRR21f++f=f�+fandf=f+�f�=f�f.Nowsincefandgareintegrable,so2112RRaref++g+andf�+g�.Furthermore,f+g=(f++g+)�(f�+g�).Thus(f+g)=(f++g+)�RRRRRRRRRR(f�+g�)=f++g+�f��g�=f+g.Together,wehave(cf+cg)=cf+cg.E121E2E(ii)Supposejhjjfjandhismeasurable.Sincefisintegrable,soaref+andf�.Thusjfj=f++f�RRRisintegrable.By(iii),wehavehjhjjfj<1.Thushisintegrable.77khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
RRRR(iii)Supposefga.e.Thenf�g0a.e.and(f�g)0.By(i),wehave(f�g)=f�gsoRRRRf�g0.i.e.fg.(*)ProofofProposition1518.Supposethatisnotcomplete.De
neaboundedfunctionftobeintegrableoverasetEof
niteRRmeasureifsup"fE"d=inffEdforallsimplefunctions"andR.Iffisintegrable,thenRgivenn,therearesimplefunctions"nandnsuchthat"nfnandnd�"nd<1=n.Thenthefunctions=infand"=sup"aremeasurableand"f.Nowthesetnn=fx:"(x)<(x)gistheunionofthesets=fx:"(x)<(x)�1=vg.Eachiscontainedvvinthesetfx:"n(x)0begiven.SupposefisRnonnegativeandboundedbyM.If(E)<"=M,thenf<".Inparticular,theresultholdsforallEsimplefunctions.Iffisnonnegative,thereisanincreasingsequenceh"niofnonnegativesimplefunctionsRRconvergingtoRfonX.BytheMonotoneConvergenceTheorem,wehaveRf=limR"nsothereexistsRNsuchthat(f�fN)<"=2.Choose<"=2supj"Nj.If(E)<,thenEf=E(f�fN)+EfN<"=2+"=2="sotheresultholdsfornonnegativemeasurablefunctions.Foranintegrablefunctionf,RRRthereexists>0suchthatif(E)<,thenjfj<".Thusjfjjfj<".EEE20.Fatou"sLemma:LethfnibeasequenceofnonnegativemeasurablefunctionsthatconvergeinRRmeasureonasetEtoafunctionf.ThereisasubsequencehfnkisuchthatlimEfnk=limEfn.NowhfnkiconvergestofinmeasureonEsobyQ13aithasasubsequencehfnkjithatconvergestofalmostRRRReverywhereonE.ThusEflimEfnkj=limEfnk=limEfn.MonotoneConvergenceTheorem:LethfnibeasequenceofnonnegativemeasurablefunctionswhichRRconvergeinmeasuretoafunctionRfandsupposethatRRfnRfforalln.Sincefnf,wehaveRRfnf.ByFatou"sLemma,wehaveflimfnlimfnfsoequalityholdsandf=limfn.LebesgueConvergenceTheorem:Let课后答案网gbeintegrableoverEandsupposethathfniisasequenceofmeasurablefunctionssuchthatonEwehavejfn(x)jg(x)andsuchthathfniconvergesinmeasureRtofalmosteverywhereonE.Thefunctionsg�fnarenonnegativesobyFatou"sLemma,(g�f)RElimE(g�fn).ThereisasubsequenceRRhfRnkithatconvergestoRfRalmosteverywhereonREsojfjjgjonEandfintegrable.ThusEgR�EfREg�limEfnandlimEfnREf.Similarly,byconsideringRthefunctionsg+fn,wehavewww.hackshp.cnEflimEfn.ThusequalityholdsandEf=limEfn.21a.Supposefisintegrable.Thenjfjisintegrable.Nowfx:f(x)6=0g=fx:jf(x)j>0g=Sfx:jf(x)j1=ng.Eachofthesetsfx:jf(x)j1=ngismeasurable.Iffx:jf(x)j1=ng=1RRforsomen,thenjfjjfj(1=n)fx:jf(x)j1=ng=1.Contradiction.Thusfx:jf(x)j1=ngfx:jf(x)j1=ng<1forallnandfx:f(x)6=0gisof-
nitemeasure.21b.Supposefisintegrableandf0.Thereisanincreasingsequenceh"niofsimplefunctionssuchthatf=lim"n.Wemayrede
neeach"ntobezeroonfx:f(x)=0g.Sincefx:f(x)6=0gis-
nite,wemayfurtherrede
neeach"ntovanishoutsideasetof
nitemeasurebyProposition7(c.f.Q10).21c.Supposefisintegrablewithrespectto.Thenf+andf�arenonnegativeintegrablefunctionssobypart(b),thereareincreasingsequencesh"niandhniofsimplefunctionseachofwhichvanishesoutsideasetof
nitemeasuresuchthatlim"=f+andlim=f�.BytheMonotoneConvergenceRRRnRnTheorem,f+d=lim"dandf�d=limd.Given">0,thereisasimplefunction"RRnnRRN+�suchthatfd�"nd<"=2andthereisasimplefunctionRN0suchthatRfd�N0d<"=2.+�LetR"="N�NR0.Then"isasimplefunctionandRRjf�R"jd=Rjf�"N�f+N0jd+�+�jf�"Njd+jf�N0jd=(fd�"Nd)+(fd�N0d)<".22a.Let(X;B;)beameasurespaceandganonnegativemeasurablefunctiononX.Set(E)=RSREgd.Clearly(;)=0.LethEnibeasequenceofdisjointsetsinB.Then(En)=SEgd=khdaw.comn78若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
RRPPRPRPg SEd=g End=g End=gd=(En).HenceisameasureonB.nEnPn22b.LetRfbeanonnegativemeasurablefunctiononPnPnRPXn.If"RisasimplefunctiongivenbyRPni=1Rci Ei.Then"d=i=1ci(Ei)=i=1ciEigd=i=1cig Eid=i=1cig Eid="gd.Nowiffisanonnegativemeasurablefunction,thereisanincreasingsequenceh"niofsimplefunctionssuchthatf=lim"n.Thenh"ngiisanincreasingsequenceofnonnegativemeasurablefunctionssuchRRthatRfg=limR"ng.BytheMonotoneConvergenceTheorem,wehavefgd=lim"ngd=lim"nd=fd.23a.Let(X;B;)beameasurespace.Supposefislocallymeasurable.ForanyandanyE2Bwith(E)<1,fx:f(x)>
gE=fx:f E(x)>
gif0andfx:f(x)>
gE=fx:f E(x)>0g[(fx:f E(x)=0gE)[fx:0>f E(x)>
gif
<0.Thusfx:f(x)>
gEismeasurablesofx:f(x)>
gislocallymeasurableandfismeasurablewithrespecttothe-algebraoflocallymeasurablesets.Conversely,supposefismeasurablewithrespecttothe-algebraoflocallymeasurablesets.ForanyandanyE2Bwith(E)<1,fx:f E(x)>
g=fx:f(x)>
gEif0andfx:f (x)>
g=(fx:f(x)>
gE)[Ecif
<0.Thusfx:f (x)>
gismeasurableandfisEElocallymeasurable.khdaw.com23b.Letbea-
nitemeasure.De
neintegrationfornonnegativelocallymeasurablefunctionsfbyRRtakingftobethesupremumof"as"rangesoverallsimplefunctionslessthanf.ForasimplePnRPnPnRfunction"=i=1ci Ei,wehave"=i=1ci(Ei)=i=1ci(Ei)=R"dR.RPSLetX=XnwhereeachXnismeasurableand(Xn)<1.Thenf=f SXn=f X.nn(n)Nowf Xnismeasurableforeachnsothereisanincreasingsequenceh"kiofsimplefunctionscon-RRPPRPR(n)PR(n)vergingtoPRf Xn.ThusRPf=Rnf Xn=nRf Xn=nlimk"k=nlimk"kd=f Xnd=f Xnd=f SXnd=fd.nn11.4Generalconvergencetheorems24.Let(X;B)beameasurablespaceandhniasequenceofmeasuresonBsuchthatforeachE2B,n+1(E)n(SE).Let(E)=limSn(E).ClearlyP(;)=0.LetPhEkibeasequenceofdisjointsetsPinB.Then(kEk)=limnn(kEk)=limnkn(Ek)=klimnn(Ek)=k(Ek)wheretheinterchangingofthelimitandthesummationisvalidbecausen(Ek)isincreasinginnforeachk.Henceisameasure.课后答案网*25.LetmbeLebesguemeasure.Foreachn,de
neby(E)=m(E)2�n.ThenhiisaSnnSSndecreasingsequenceofmeasures.NotethatPR=Pk2Z[k;k+1).Now(k[k;kP+1)=limnn(k[k;k+1))=limm(R)2�n=1but([k;k+1))=lim([k;k+1))=limm([k;k+1))2�n=Pnkknnknlim2�n=0.Henceisnotameasure.kn*26.Let(X;B)beameasurablespaceandwww.hackshp.cnhniasequenceofmeasuresonBthatconvergesetwisetotoasetfunction.Clearly(;)=0.IfE1;E22BandE1E2=;,then(E1[E2)=limn(E1[E2)=lim(n(E1)+n(E2))=limn(E1)+limn(E2)=(E1)+(E2).Thusis
nitelyadditive.IfTisnotameasure,thenitisnot;-continuous.i.e.thereisadecreasingsequencehEniofsetinBwithEn=;andlim(En)=">0.De
ne1=1=1.Ifjandjhavebeende
nedforjk,letk+1>
ksuchthatk+1(Ek)7"=8.Thenletk+1>
ksuchthat"=8k+1(EkP+1).De
neFn=EnnEn+1.Thenn+1(Fn)P3"=4.Nowforjoddand1k0,then(Ec)=0so(X)=(E)+(Ec)=0.Thus=0andwestillhave?.11.6TheRadon-NikodymTheorem33a.Let(X;B;)bea-
nitemeasurespaceandletbeameasureonBwhichisabsolutelyScontinuouswithrespectto.LetX=Xiwith(Xi)<1foreachi.WemayassumetheXiarepairwisedisjoint.Foreachi,letBi=fE2B:EEig,i=jBiandi=jBi.Then(Xi;Bi;i)isa
nitemeasurespaceandi<<i.Thusforeachithereisanonnegativei-measurablefunctionfisuch80khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
Rthati(E)=EfidiforallPE2Bi.De
nePfbyf(x)=PfiR(x)ifx2Xi.IfPERX,thenERXi2Biforeachi.Thus(E)=(EXi)=i(EXi)=EXifidi=EXifd=Efd.RR33b.Iffandgarenonnegativemeasurablefunctionssuchthat(E)=fd=gdforanyRREEmeasurablesetE,thenfd=gdsof=ga.e.34a.Radon-Nikodymderivatives:Suppose<<andfisanonnegativemeasurablefunction.ForhiPnRPnPnRdanonnegativesimplefunction"=i=1ci Ei,wehave"d=i=1ci(Ei)=i=1ci(Eidd)=RPhiRhinc dd="dd.Foranonnegativemeasurablefunctionf,leth"ibeanincreas-i=1iEiddiRRingsequenceofnonnegativesimplefunctionsconvergingpointwisetof.Thenfd=lim"id=RhiRhilim"dd=fdd.iddR34b.hIfi1<<and2R<<h,theni1+2<<.ForanymeasurablesetRhihiE,wehave1(E)=d1dand(E)=d2d.Thus(+)(E)=d1+d2d.ByuniquenessoftheEd2Ed12EddhihihiRadon-Nikodymderivative,wehaved(1+2)=d1+d2.dddRhiRhihi34c.Suppose<<<<.ForanymeasurablesetE,wehave(E)=dd=dddkhdaw.comhihiEdEddwherethelastequalityfollowsfrompart(a).Henced=dd.dddhihihihi�134d.Suppose<<and<<.Thend=ddbypart(c).Butd1sod=d.dddddd35a.Supposeisasignedmeasuresuchthat?and<<.TherearedisjointmeasurablesetsAandBsuchthatX=A[Bandjj(B)=0=jj(A).Thenj(A)j=0sojj(X)=jj(A)+jj(B)=0.Hence+=0=�so=0.35b.Suppose1and2aresingularwithrespectto.TherearedisjointmeasurablesetsA1andB1suchthatX=A1[B1and(B1)=0=1(A1).Similarly,therearedisjointmeasurablesetsA2andB2suchthatX=A2[B2and(B2)=0=2(A2).NowX=(A1A2)[(B1[B2),(A1A2)(B1[B2)=;and(c11+c22)(A1A2)=0=(B1[B2).Hencec11+c22?.35c.Suppose1<<and2<<.If(E)=0,then1(E)=0=2(E).Thus(c11+c22)(E)=0andc11+c22<<.35d.Let(X;B;)bea-
nitemeasurespaceandletbea-
nitemeasureonB.Suppose=+=0+0where?,0?,<<and<<.Now�0=0�where�0and0101课后答案网00120011000�aresignedmeasuressuchthat�0?and0�<<byparts(b)and(c).Thenbypart110011(a),wehave�0=0=0�so=0and=0.0011001136.Let(X;B;)bea-
nitemeasurespaceandsupposeisasignedmeasureonBwhichisabsolutelycontinuouswithrespectto.ConsidertheJordandecomposition=�whereeither+or�must1R2Rbe
nite.Therearemeasurablefunctionsfandgsuchthat+(E)=fdand�(E)=gd.REEThen(E)=+(E)��(Ewww.hackshp.cn)=(f�g)d.E37a.Complexmeasures:Givenacomplexmeasure,itsrealandimaginarypartsare
nitesignedmeasuresandsohaveJordandecompositions1�2and3�4respectively.Hence=1�2+i3�i4where1;2;3;4are
nitemeasures.*37b.*37c.*37d.*37e.(*)Polardecompositionforcomplexmeasures38a.Letandbe
nitemeasuresonameasurablespace(X;B)andset=+.De
neF(f)=RRRRfd.NotethatL2()L1()L1()sincejfjd((X))1=2(jfj2d)1=2andjfjdRjfjd.ThusFiswell-de
ned.ClearlyFislinear.FurthermorejF(f)j((X))1=2jjfjj.HenceFis2aboundedlinearfunctionalonL2().S38b.Thereexistsauniquefunctiong2L2()suchthatF(f)=(f;g).Notethatfg>1g=fgR1+1=ng.LetEn=fg1+1=ng.Then(En)=F( En)=Engd(1+1=n)(En)so81khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
(1=n)(En)+(En)0.Itfollowsthat(En)=0=(En)andR(En)=0.Thus(fg>1g)=0.Similarly,R(fg<0g=0.Now(E)=F( E)=( E;g)=Egdand(E)=(E)�(E)=(1�g)d.E38c.Suppose<<.If(E)=0,then(E)=0so(E)=0.Thus<<.LetE=fx:g(x)=0g.RThen(E)=gd=0.Thusg=0onlyonasetof-measurezero.Inthiscase,wehaveRERR(E)=1d=gg�1d=g�1dbyQ22.EERERR38d.Suppose<<.Then(1�g)g�1d=g�1d�gg�1d=(E)�(E)=(E).InEEEparticular,(1�g)g�1isintegrablewithrespectto.(*)AlternateproofoftheRadon-NikodymTheorem39.LetX=[0;1],BtheclassofLebesguemeasurablesubsetsof[0;1]andtaketobeLebesguemeasureandtobethecountingmeasureonB.Thenis
niteandabsolutelycontinuouswithrespectRto.Supposethereisafunctionfsuchthat(E)=fdforallE2B.Sinceis
nite,fisERintegrablewithrespectto.ThusE0=fx:f(x)6=0giscountable.Now0=(E0)=Efd.0Contradiction.Hencethereisnosuchfunctionf.40a.Decomposablemeasures:LetfXgbeadecompositionforameasureandEameasurableset.SSSNotethatkhdaw.com(fXE:(XE)=0g)=0and(EnX)=0.Thus(E)=(fXE:(XE)>0g).If(XE)>0forcountablymany,thenwehaveacountableunionanditfollowsthatPP(E)=(XE).IfS(XnE)>0foruncountablymanySn,thenS(XE)=1.IfS(E)<1,thenforany
niteunionkP=1(XkE),wehave(k=1(XkE))((XE))P(fXE:(XE)>0g)=(E)so(XE)(E)<1.Contradiction.Thus(E)=1=(XE).40b.LetfXgbeadecompositionforacompletemeasure.Iffislocallymeasurable,thensince(X)<1foreach,therestrictionofftoeachXismeasurable.Conversely,supposetherestrictionSofftoeachXismeasurable.GivenameasurablesetSEwithS(E)<1,letA=fXE:(XE)>0g,B=fXE:(XE)=0gandC=EnX).For0,fx:f E(x)>g=fx2A:f(x)>
g[fx2B:f(x)>
g[fx2C:f(x)>
g.Thelasttwosetsaremeasurablesincetheyaresubsetsofsetsofmeasurezero.The
rstsetismeasurablesinceitissimplyfx:f (x)>
gEforsome.For
<0,fx:f (x)>
g=Ec[(Efx:f(x)>
g).ByaXEsimilarargumentasbefore,fx:f E(x)>
gismeasurable.Hencefislocallymeasurable.RRPnRLetfbeanonnegativelocallymeasurablefunctionofX.NowfdSnfd=i=1fdXXiXiRPRi=1forany
nitesetPfX1;:::;Xng.ThusXRfdPXfd.Ontheotherhand,foranysimplefunctionSPPn课后答案网nn"P=Pni=1ci Eiwith"fP,wehaveRX"dR=i=1ciP(EiRX)=i=1ci((EiX))=(i=1ci(EiX))=X"d.ThusXfdXfd.*40c.LetbeabsolutelycontinuouswithrespecttoandsupposethatthereisacollectionfXgwhichisadecompositionforbothand.Letbede
nedbyR(E)=(XE)foreachP.ThenthereisanonnegativemeasurablefunctionPfsuchthatP(E)=PREfd.ThefunctionPRf=Rfislocallymeasurableand(E)=www.hackshp.cn(XE)=(E)=Efd=XEfd=Efd.40d.IfinsteadofassumingfXgtobeadecompositionfor,wemerelyassumethatifE2Band(EX)=0forall,then(E)=0,thereverseimplicationinpart(b)remainsvalidalthoughtheforwardimplicationmaynotbetrue.Thustheargumentinpart(c)remainsvalidandsodoestheconclusion.11.7TheLpspaces41.Letf2Lp();1p<1,and">0.Firstassumethatf0.Leth"ibeanincreasingsequencenofnonnegativesimplefunctionsconvergingpointwisetof,eachofwhichvanishesoutsideasetof
nitemeasure.Thenthesequencehjf�"jpiconvergespointwisetozeroandisboundedby2fp.BytheRnLebesgueConvergenceTheorem,limjf�"jpd=0.i.e.limjjf�"jj=0.Thusjjf�"jj<"fornnppsome".Nowforageneralf,therearesimplefunctions"andvanishingoutsidesetsof
nitemeasuresuchthatjjf+�"jj<"=2andjjf��jj<"=2.Thenjjf�("+)jj<".ppp(*)ProofofProposition2642.Let(X;B;)bea
nitemeasurespaceandganintegrablefunctionsuchthatforsomeconstant82khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
RM,jg"djMjj"jjR1forallsimplefunctionsR".LetE=fx:jg(x)jM+"gandlet"=(sgng) E.Then(M+")(E)jgjd=jg"djMjj"jj=M(E).Thus(E)=0andg2L1.E1(*)ProofofLemma27forp=143.Let(X;B;)bea-
nitemeasurespaceandganintegrablefunctionsuchthatforsomeconstantRSM,jg"djMjj"jjpforallsimplefunctions".NowX=Xnwhere(Xn)<1foreachn.LetSqqgn=g nXi.Thengn2L,gn!gandjgnjjgjforeachn.Thuslimjg�gnjd=0.Itfollowsi=1thatlimjjg�gjj=0andg2Lq.nq44.LethEibeasequenceofdisjointmeasurablesetsandforeachnletfbeafunctioninLp;1nPRRPnRPPRp<1,thatvanishesoutsideE.Setf=f.Thenjfjp=jfjp=jfjp=jfjp=PnPnnPnnjjfjjp.Hencef2Lpifandonlyifjjfjjp<1.Inthiscase,jjfjjp=jjfjjp.Also,sincethenPnPnnnnormiscontinuous,wehavejji=1fijjp!jjfjjpsojjf�i=1fijjp!0(c.f.Q6.16).R45.Forg2Lq,letFbethelinearfunctionalonLpde
nedbyF(f)=fgd.BytheH•olderRRinequality,wehavejF(f)j=jfgdjjfgjdjjfjjpjjgjjqsojjFjjjjgjjq.For10.qqpqRR1Letf=sgng.Thenf2L1,jjfjj=1andjF(f)j=jfgdj=jgjd=jjgjj=jjfjjjjgjjsokhdaw.com1111jjFjjjjgjj1.IfRq=1andp=1,given">0,letRE=fxR:g(x)>jjgjj1�"gandletf= E.Thenf2L1,jjfjj=jfjd=(E)andjF(f)j=jfgdj=jgdj(jjgjj�")jjfjjsojjFjjjjgjj.1E11146a.LetbethecountingmeasureonacountablesetX.WemayenumeratetheelementsofXbyPhxi.Byconsideringsimplefunctions,weseethatjfjpisintegrableifandonlyifjf(x)jp<1.nnHenceLp()=`p.*46b.*47a.*47b.*48.LetAandBbeuncountablesetswithdi
erentnumbersofelementsandletX=AB.LetBbethecollectionofsubsetsEofXsuchthatforeveryhorizontalorverticallineLeitherELorEcLiscountable.Clearly;2B.SupposeE2B.Bythesymmetryinthede
nition,Ec2B.SupposeSShSEniisasequenceofsetsinTB.ForeveryhorizontalorverticallineSL,(En)L=(EnL)and(E)cL=EcL.IfELiscountableforalln,then(E)Liscountable.Otherwise,nnnSSnEcLiscountableforsomenand(E)cLiscountable.ThusE2B.HenceBisa-algebra.n课后答案网nnLet(E)bethenumberofhorizontalandverticallinesLforwhichEcLiscountableand(E)bethenumberofhorizontallineswithEcLcountable.Clearly(;)=0=(;)sinceAandBareSuncountable.SupposehEniisasequenceofdisjointsetsinTB.Then(En)isthenumberofhorizontalandverticallinesLforwhichEcLiscountable.NotethatifEcLiscountable,thenELisnTnmcountableform6=nsinceEEc.IfEcLiscountable,thenEcLiscountable.OntheotherTmnnnShand,ifEcLiscountable,thenEcLiscountableforsomen,forotherwisewehave(E)Lnwww.hackshp.cnSnPnbeingcountable.Itfollowsthat(En)=(En).ThusisameasureonBandsimilarly,isameasureonB.RDe
neaboundedlinearfunctionalFonL1()bysettingF(f)=fd.12MeasureandOuterMeasure12.1Outermeasureandmeasurability1.Suppose(E)=(E)=0.ForanysetA,wehave(AE)=0sinceAEE.Also,AEcEso(E)(AEc)=(AE)+(AEc).ThusEismeasurable.IfFE,then(F)=0soFismeasurable.Henceiscomplete.S2.SupposethatPhEiiisasequenceofdisjointmeasurablesetsandE=Ei.ForanysetA,wehave(AE)(AE)bycountablesubadditivity.Now(A(E[E))(A(E[E)i1212E)+(A(E[E)Ec)=(AE)+(AE)bymeasurabilityofE.Byinductionwehave1Sn1P2n112Sn1Pn(AE)(AE)foralln.Thus(AE)(AE)(AE)i=1ii=1PiPi=1ii=1iforallnso(AE)(AE).Hence(AE)=(AE).ii83khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
12.2Theextensiontheorem*3.LetXbethesetofrationalnumbersandAbethealgebraof
niteunionsofintervalsoftheform(a;b]with(a;b]=1and(;)=0.Notethatthesmallest-algebracontainingAwillcontainone-pointsets.Letkbeapositivenumber.De
nek(A)=kjAj.Thenkisameasureonthesmallest-algebracontainingAandextends.4a.IfAistheunionofeachoftwo
nitedisjointcollectionsPfCigandfDjgofsetsinPC,thenforPeachi,wehavePP(Ci)=j(CiPDPj).Similarly,foreachPj,wehave(Dj)=i(CiDj).Thusi(Ci)=ij(CiDj)=ji(CiDj)=j(Dj).Pn4b.De
ning(A)=i=1(Ci)wheneverSAisthedisjointunionofthesetsSnPnCi2C,wehavea
nitelySPadditivesetfunctiononA.Thus(Ci)S(i=1CPi)=i=1(Ci)forallnso(Ci)(Ci).Condition(ii)givesthereverseinequality(Ci)(Ci)soiscountablyadditive.(*)ProofofProposition95a.LetCbeasemialgebraofsetsandAthesmallestalgebraofsetscontainingC.Theunionoftwo
niteSncTncunionsofsetsinCisstilla
niteunionofsetsinC.Also,(i=1Ci)=i=1Ciisa
niteintersectionof
niteunionsofsetsinC,whichisthena
niteunionofsetsinC.Thusthecollectionof
niteunionsofsetsinkhdaw.comCisanalgebracontainingC.Furthermore,ifA0isanalgebracontainingC,thenitcontainsallSn
niteunionsofsetsinC.HenceAiscomprisedofsetsoftheformA=i=1Ci.5b.ClearlyCA.Ontheotherhand,sinceeachsetinAisa
niteunionofsetsinC,wehaveAC.HenceA=C.6a.LetAbeacollectionofsetswhichisclosedunder
niteunionsand
niteintersections.CountableSSSunionsofsetsinAarestillcountableunionsofsetsinA.Also,ifAi;Bj2A,then(iAi)(jBj)=i;j(AiBj),whichisacountableunionofsetsinA.HenceAisclosedundercountableunionsand
niteintersections.TTTTnTnTnTn+16b.IfBi2A,thenBi=ni=1Biwherei=1Bi2Aandi=1Bii=1Biforeachn.HenceeachsetinAistheintersectionofadecreasingsequenceofsetsinA.7.Letbea
nitemeasureonanalgebraA,andtheinducedoutermeasure.Supposethatforeach">0thereisasetA2A,AE,suchthat(EnA)<".NotethatAismeasurable.ThusforanysetB,wehave(B)=(BA)+(BAc)(BE)�(B(EnA))+(BEc)>(BE)+(BEc)�".Thus(B)(BE)+(BEc)andEismeasurable.Conversely,supposeEismeasurable.Given课后答案网">0,thereisasetB2AsuchthatEcBand(B)(Ec)+".LetA=Bc.ThenA2AandAE.Furthermore,(EnA)=(EB)=(B)�(BEc)=(B)�(Ec)".8a.IfwestartwithanoutermeasureonXandformtheinducedmeasureonthe-measurablePsets,wecanusetoinduceanoutermeasure+.ForeachsetE,wehave(E)(A)foranySisequenceof-measurablesetsAwithEA.Takingthein
mumoverallsuchsequences,wehavePPii(E)inf(A)=inf(A)=+(E).iwww.hackshp.cni8b.Supposethereisa-measurablesetAEwith(A)=(E).Then+(E)(A)=(A)=(E).Thusbypart(a),wehave+(E)=(E).Conversely,suppose+(E)=(E).Foreachn,thereisa-measurableset(acountableunionof-Tmeasurablesets)AwithEAand(A)=(A)+(E)+1=n=(E)+1=n.LetA=A.nnnnnThenAis-measurable,EAand(A)(E)+1=nforallnso(A)=(E).8c.If+(E)=(E)foreachsetE,thenbypart(b),foreachsetE,thereisa-measurablesetAwithAEand(A)=(E).Inparticular,(A)(E)+"soisregular.Conversely,ifisregular,thenforeachsetEandeachn,thereisa-measurablesetAwithAETnnand(A)(E)+1=n.LetA=A.ThenAis-measurable,AEand(A)=(E).Bynnpart(b),+(E)=(E)foreachsetE.8d.Ifisregular,then+(E)=(E)foreveryEbypart(c).Inparticular,isinducedbythemeasureonthe-algebraof-measurablesets.Conversely,supposeisinducedbyameasureonanalgebraA.ForeachsetEandany">0,thereSSisasequencehAiofsetsinAwithEAand(A)(E)+".EachAis-measurablesoSiiiiAis-measurable.Henceisregular.ikhdaw.com84若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
8e.LetXbeasetconsistingoftwopointsaandb.De
ne(;)=(fag)=0and(fbg)=(X)=1.ThenisanoutermeasureonX.ThesetXistheonly-measurablesetcontainingfagand(X)=1>(fag)+1=2.Henceisnotregular.9a.Letbearegularoutermeasure.ThemeasureinducedbyiscompletebyQ1.LetEbelocally-measurable.ThenEBis-measurableforany-measurablesetBwith(B)=(B)<1.Wemayassume(E)<1.Sinceisregular,itisinducedbyameasureonanalgebraAsothereisasetB2AwithEBand(B)(E)+1<1.ThusE=EBis-measurable.*9b.10.LetbeameasureonanalgebraAandtheextensionofitgivenbytheCaratheodoryprocess.LetEbemeasurablewithrespecttoand(E)<1.Given">0,thereisacountablecollectionSPfAgofsetsinAsuchthatEAand(A)(E)+"=2.ThereexistsNsuchthatnnnP1SNPn=N+1(An)<"=P2.LetA=n=1(An).ThenA2Aand(AE)=(AnE)+(EnA)(A)�(E)+1(A)<".nn=N+1n11a.LetbeameasureonAanditsextension.Let">0.Iffis-integrable,thenthereisPnRPnasimplefunctioni=1ci EiwhereeachEiis-measurableandjf�i=1ci Eijd<"=2.Thesimplefunctionmaybetakentovanishoutsideasetof
nitemeasuresowemayassumeeachkhdaw.comEihas
nite-measure.ForeachE,thereexistsA2Asuchthat(AE)<"=2n.ConsidertheA-simplePiRiiinfunction"=i=1ci Ai.Thenjf�"jd<".*11b.12.3TheLebesgue-StieltjesintegralS112.LetFbeamonotoneincreasingfunctioncontinuousontheright.Suppose(a;b]i=1(ai;bi].Let">0.Thereexists>0suchthatF(b+)0suchthatiiiiF(a+)F(b+)�F(a)>F(b)�F(a+).k�1k�111P1kk1课后答案网1NowPF(b)�F(a)=(F(b)�F(a+))+(F(a+)�F(a))0,thereexists>0suchthatF(y)�F(x)<"whenevery2(x;x+).Nowwhenz2(y;y+0)where0<00,thereexists>0suchthat(E)<"whenever0Pn0m(E)<S.Forany
nitecollectionPf(xi;xi)gofnonoverlappingintervalswithi=1jxi�xij<,wehave(n(x;x0))<".i.e.njF(x0)�F(x)j<".ThusFisabsolutelycontinuous.i=1iii=1iiPConversely,supposeFisabsolutelycontinuous.Given">0,thereexists>0suchthatnjf(x0)�Pi=1if(x)j<"forany
nitecollectionf(x;x0)gofnonoverlappingintervalswithnjx0�xj<.LetEiiii=1iiSbeameasurablesetwithPm(E)<=2.ThereisasequenceofopenintervalshInisuchthatSPEInandm(In)<.Wemayassumetheintervalsarenonoverlapping.Now(E)(In)=(In)=PPkPkP(F(bn)�F(an))whereIn=(an;bn).Sincen=1P(bn�an)=n=1m(In)<foreachn,wehavekn=1(F(bn)�F(an))<"课后答案网foreachn.Thus(E)(F(bn)�F(an))<"and<<2�2�xifx=y;f(x;y)=�2+2�xifx=y+1;>:0otherwise:PPPPPPThenf(x;y)=f(1;1)=3butf(x;y)=(2�2�y)+(�2+2�y�1)=2�y�1�2�y=Pxy2yxyy�2�y�1=�1.y2课后答案网(*)WecannotremovethehypothesisthatfbenonnegativefromTonelli"sTheoremorthatfbeinte-grablefromFubini"sTheorem.25.LetX=Ybetheinterval[0;1],withA=BtheclassofBorelsets.LetbeLebesguemeasurej�1jandthecountingmeasure.Let=Sfhx;yi2XYT:x=ygbethediagonal.LetIj;n=[n;n]nforj=1;:::;nRRandletIn=jR=1Ij;n.Notethat=RnIn.Henceismeasurable(andinfactanRRRR).NowXR[Y d]dwww.hackshp.cn=X(Sfy:y=xg)d=X1d=1butY[X d]d=Y(fx:x=yg)d=Y0d=0.Let(AnPBn)whereAn2AandBn2B.ThensomeBnmustbein
nitesothat(R)(AnBn)=1.Thus()(AnBn)=1.Byde
nitionofoutermeasure,itfollowsthat d()=()()=1.XY(*)WecannotremovethehypothesisthatfbeintegrablefromFubini"sTheoremorthatandbe-
nitefromTonelli"sTheorem.26.LetX=Ybethesetofordinalslessthanorequaltothe
rstuncountableordinal.LetA=Bbethe-algebraconsistingofallcountablesetsandtheircomplements.De
ne=bysetting(E)=0ifEiscountableand(E)=1otherwise.De
neasubsetSofXYbyS=fhx;yi:xxgandSy=RfxR:x
g2ABsoEx2Bforalmostallx.NowEx=fy:f(x;y)>
g=fy:fx(y)>
g.ThusfxismeasurablewithrespecttoBforalmostallx.29a.LetX=Y=Randlet=beLebesguemeasure.Thenistwo-dimensionalLebesguemeasureonXY=R2.ForeachmeasurablesubsetEofR,let(E)=fhx;yi:x�y2Eg.IfEisanTopenset,thenT(E)isopenandthusmeasurable.IfEisaGwithE=EiwhereeachEiisopen,then(E)=(Ei),whichismeasurable.IfEisasetofmeasurezero,then(E)isasetofmeasurezeroandisthusmeasurable.Ageneralmeasurablesetkhdaw.comEisthhedi
erenceofaGsetAandasetBofmeasurezeroand(E)=(A)n(B)so(E)ismeasurable.29b.LetfbeameasurablefunctiononRandde
nethefunctionFbyF(x;y)=f(x�y).Forany,wehavefhx;yi:F(x;y)>
g=fhx;yi:f(x�y)>
g=fhx;yi:x�y2f�1[(
;1)]g=(f�1[(
;1)]).Theinterval(
;1)isaBorelsetsof�1[(
;1)]ismeasurable.Itfollowsfrompart(a)thatfhx;yi:F(x;y)>
gismeasurable.HenceFisameasurablefunctiononR2.29c.LetfandgbeintegrablefunctionsonRandde
nethefunction"by"(y)=f(x�y)g(y).RRRRRByTonelli"sTheorem,jf(x�y)g(y)jdxdy=[jf(x�y)g(y)jdx]dy=jg(y)j[jf(x�RRXYRRYXYXy)jdx]dy=jg(y)j[jf(x)jdx]dy=jfjjgj.Thusthefunctionjf(x�y)g(y)jisintegrable.ByYXRRRRFubini"sTheorem,foralmostallx,thefunction"isintegrable.Leth=".Thenjhj=j"jRRRRRYXYj"j=j"jjfjjgj.XYXYR30a.LetfandgbefunctionsinL1(�1;1)andde
nefgtobethefunctionde
nedbyf(y�x)g(x)dx.Iff(y�x)g(x)isintegrableaty,thende
neF(x)=f(y�x)g(x)andG(x)=F(y�x).RRRRThenGisintegrableandG(x)dx=F(x)dx.i.e.f(x)g(y�x)dx=f(y�x)g(x)dx.Thusfory2R,f(y�x)g(x)isintegrableifandonlyiff(x)g(y�x)isintegrableandtheirintegralsarethesameinthiscase.Whenf课后答案网(y�x)g(x)isnotintegrable,(fg)(y)=(gf)(y)=0sincethefunctionisintegrableforalmostally.Hencefg=gf.30b.Forx;y2Rsuchthatf(y�x�u)g(u)isintegrable,de
neF(u)=f(y�x�u)g(u).ConsiderRRRG(u)=F(u�x).ThenGisintegrableandf(y�u)g(u�x)du=G(u)du=F(u)du=(fg)(y�x).RThefunctionH(u;x)=f(y�u)g(u�x)h(x)isintegrable.Then((fg)h)(y)=(fg)(y�x)h(x)dx=RRRRR[f(y�u)g(u�x)du]h(x)dx=f(y�u)g(u�x)h(x)dudx=[f(y�u)g(u�x)h(x)dx]du=Rf(y�u)(gh)(u)du=(fwww.hackshp.cn(gh))(y).RR30c.Forf2L1,de
nef^byf^(s)=eistf(t)dt.Thenjf^jjfjsof^isaboundedcomplexfunction.RRRRRFurthermore,foranys2R,wehavef[g(s)=eist(fg)(t)dt=eist[f(t�x)g(x)dx]dt=[f(t�RRRRx)eis(t�x)g(x)eisxdx]dt=[f(t�x)eis(t�x)g(x)eisxdt]dx=[f(t�x)eis(t�x)dt]g(x)eisxdx=RR[f(u)eisudu]g(x)eisxdx=f^(s)^g(s).31.Letfbeanonnegativeintegrablefunctionon(�1;1)andletm2betwo-dimensionalLebesguemeasureonR2.Thereisanincreasingsequenceofnonnegativesimplefunctionsh"iconvergingpoint-nSwisetof.LetEn=fhx;yi:00foreachi.Theni(n)(n)(n)(n)En=(F1(0;a1))[[(Fkn(0;akn)).HenceEnismeasurablesofhx;yi:0k;j(1n)(FkGj).NowifE(khdaw.comXX)(XX),thenERand(()())(E)+">P1pp+1nPPi1pp+1n(()())(R)>()(FG)�"0()(E)�".i1pp+1niik;j1nkj1nThus(()())(E)()(E).1pp+1n1nHence(()())=.Itthenfollowsthat()1pp+1n1n1p(p+1n)=1n.*33.Letf(X;A;)gbeacollectionofprobabilitymeasurespaces.12.5Integraloperators*34.ByProposition21,wehavejjTjjM
;
.35.Letk(x;y)beameasurablefunctiononXYofabsoluteoperatortype(p;q)andg2Lq().ThenRjkjisofoperatortype(p;q)sobyProposition21,foralmostallx,theintegraljk(x;y)jg(y)dexists.RYThusforalmostallx,theintegralf(x)=k(x;y)g(y)dexists.Furthermore,thefunctionfbelongsRYtoLp()anjjfjjjjjk(x;y)jg(y)djjjjjkjjjjjgjj.pYpp;qq(*)ProofofCorollary22课后答案网36.Letg,handkbefunctionsonRnofclassLq,LpandLrrespectively,with1=p+1=q+1=r=2.Wemaywrite1=p=1�(1�)=rand1=q=1�=rforsome01.ThenkisofcovarianttypeR(p;q)sotheintegralf(x)=k(x;y)g(y)dyexistsforalmostallxandthefunctionfbelongstoLpRnRRRwithjjfjjpjjkjjrjjgjjqbyProposition21.NowR2njh(x)k(x�y)g(y)jdxdy=Rnjh(x)f(x)jdxjjhjjpjjkjjrjjgjjq.(*)ProofofProposition25www.hackshp.cn37.Letg2Lqandk2Lr,with1=q+1=r>1.Let1=p=1=q+1=r�1.Wemaywrite1=q=1�=rand1=p=1�(1�)=rwhere01.Thenkisofcovarianttype(p;q)sothefunctionf(x)=RRnk(x�y)g(y)dyisde
nedforalmostallxandjjfjjpjjkjjrjjgjjqbyProposition21.(*)ProofofProposition26*38.Letg,handkbefunctionsonRnofclassLq,LpandLrwith1=p+1=q+1=r2.12.6Innermeasure39a.Suppose(X)<1.Byde
nition,(E)(X)�(Ec).Conversely,sinceEandEcaredisjoint,wehave(E)+(Ec)(E[Ec)=(X).Hence(E)=(X)�(Ec).39b.SupposeAisa-algebra.IfA2AandEA,then(E)(A).Thus(E)inff(A):SEA;A2Ag.Conversely,foranysequenceSPhAiiofsetsinAcoveringE,wehaveAi2Asoinff(A):EA;A2Ag(A)(A).Thusinff(A):EA;A2Ag(E).Henceii(E)=inff(A):EA;A2Ag.IfA2AandAE,then(A)=(A)�(AnE).Thussupf(A):AE;A2Ag(E).khdaw.com89若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
Conversely,ifA2Aand(AnE)<1,thenforany">0,thereisasequencehAiofsetsinAsuchthatSPSiAnEAand(A)<(AnE)+".LetB=A.ThenB2Aand(B)<(AnE)+".Also,iiiAnB2AandAnBE.Thus(A)�(AnE)�"=(A)�(B)=(AnB)supf(A):AE;A2Ag.Itfollowsthat(E)supf(A):AE;A2Ag.Hence(E)=supf(A):AE;A2Ag.39c.LetbeLebesguemeasureonR.Bypart(c),wehave(E)=supf(A):AE;Ameasurableg.IfAismeasurableandAE,thengiven">0,thereisaclosedsetFAwith(AnF)<".Thus(A)=(F)+(AnF)<(F)+".Itfollowsthat(E)supf(F):FE;Fclosedg.Conversely,ifFEandFisclosed,thenFismeasurableso(F)(E).Thussupf(F):FE;Fclosedg(E).Hence(E)=supf(F):FE;Fclosedg.SSS40.LethBibeasequenceofdisjointsetsinB.Then(B)=(BE)+(BEc)=PiPPSSiiSPi(BE)+(BEc)=(B).Also,(B)=(BE)+(BEc)=(BPiPiiiiiiE)+(BEc)=(B).HencethemeasuresandinTheorem38arecountablyadditiveoniiB.41.LetbeameasureonanalgebraA,andletEbea-measurableset.IfB2B,thenBisoftheform(AE)[(A0Ec)whereA;A02A.Thus(B)=(AE)+(A0Ec)and(B)=(AE)+(A0Ec).If(A0Ec)=1,then(A0Ec)=1and(B)=(B).If(A0khdaw.comEc)<1,thensinceA0Ecis-measurable,(A0Ec)=(A0Ec)=(A0Ec)whereisthemeasureinducedby.Thus(B)=(B).42a.LetGandHbetwomeasurablekernelsforEsoGE,HEand(EnG)=0=(EnH).Then(GnH)=0=(HnG)sinceGnHEnHandHnGEnG.Inparticular,GHismeasurableand(GH)=0.LetG0andH0betwomeasurablecoversforEsoG0E,H0Eand(G0nE)=0=(H0nE).Then(G0nH0)=0=(H0nG0)sinceG0nH0G0nEandH0nG0H0nE.Inparticular,G0H0ismeasurableand(G0H0)=0.S42b.SupposeEisasetof-
niteoutermeasure.ThenE=EwhereeachEis-measurableandnn(E)<1.Then(E)<1sothereexistG2AsuchthatGEand(G)=(E)=nSnnnnPnn(E).LetG=G.ThenGismeasurable,GEand(EnG)(EnG)(EnH)=0.nnnnHence(EnG)=0andGisameasurablekernelforE.SAlso,thereexistH2AsuchthatEHand(H)=(E).LetH=H.ThenHisnnnPnnnmeasurable,EHand(HnE)(HnE)(HnE)=0.Hence(HnE)=0andHisnnameasurablecoverforE.43a.LetPbethenonmeasurablesetinSection3.4.Notethatm(P)m(P)1.ByQ3.15,课后答案网foranymeasurablesetEwithEP,wehavem(E)=0.Hencem(P)=supfm(E):EP;Emeasurable;m(E)<1g=0.S43b.LetE=[0;1]nP.LethInibeasequenceofopenintervalssuchthatSSEIn.WemaySassumethatIn[0;1]foralln.Then[0;1]nInPsom([0;1]nIn)=0.Thusm(In)=1.Hencem(E)=1.SupposeA[0;1]isameasurableset.Notethatm(AE)m(A[0;1])andm(EnA)m([0;1]nA).Thus1=www.hackshp.cnm(E)=m(AE)+m(EnA)m(A[0;1])+m([0;1]nA)=1som(AE)=m(A[0;1]).*43c.*44.LetbeameasureonanalgebraAandEasetwith(E)<1.Letbearealnumberwith(E)(E).*45a.*45b.46a.LetAbethealgebraof
niteunionsofhalf-openintervalsofRandlet(;)=0and(A)=1SforA6=;.IfE6=P;andhAiiisasequenceofsetsinAwithEAi,thenAi6=;forsomeiand(A)=1.Thus(A)=1so(E)=1.ii46b.IfEcontainsnointerval,thentheonlyA2Awithm(AnE)<1is;.Thus(E)=0.IfEcontainsanintervalI,then(E)(I)�(InE)=(I)=1.46c.NotethatR=Q[Qc,(R)=1,(Q)=(Qc)=0.ThusrestrictedtoBisnotameasureandthereisnosmallestextensionoftoB.46d.ThecountingmeasureisameasureonBandcountingmeasurerestrictedtoAequals.HencethecountingmeasureonBisanextensionoftoB.khdaw.com90若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
46e.LetEbeaninterval.Then(E)=1but(EQ)=0and(EQc)=0.HenceLemma37failsifwereplacesetsinA"bymeasurablesets".47a.LetX=fa;b;cgandset(X)=2,(;)=0and(E)=1ifEisnotXor;.Setting(E)=(X)�(Ec),wehave(X)=2,(;)=0and(E)=1ifEisnotXor;.47b.;andXaretheonlymeasurablesubsetsofX.47c.(E)=(E)forallsubsetsEofXbutallsubsetsexcept;andXarenonmeasurable.47d.BytakingE=fagandF=fbg,wehave(E)+(F)=(E)+(F)=2but(E[F)=(E[F)=1.Hencethe
rstandthirdinequalitiesofTheorem35fail.(*)Ifisnotaregularoutermeasure(i.e.itdoesnotcomefromameasureonanalgebra),thenwedonotgetareasonabletheoryofinnermeasurebysetting(E)=(X)�(Ec).48a.LetX=R2andAthealgebraconsistingofalldisjointunionsofverticalintervalsoftheformI=fhx;yi:a0.Let�bethesetoffunctions"oftheform"(x)=(x;E).SupposeAandBareseparatedbysome"2�.Thentherearenumbersaandbwitha>b,(x;E)>aonAand(x;E)0so(A[B)=(A)+(B)andisaCaratheodoryoutermeasurewithrespectto�.NowforaclosedsetF,wehaveF=fx:(x;F)0g,whichismeasurablesince"(x)=(x;F)is-measurable.Thuseveryclosedset(andhenceeveryBorelset)ismeasurablewithrespectto.www.hackshp.cn(*)ProofofProposition4112.9Hausdor
measuresS52.SupposeEEn.If">0andhBi;niiisasequenceofballscoveringEnwithradiiri;n<",(")PPP(")thenhBi;nii;nisasequenceofballscoveringEso(E)i;nri;n=niri;n.Thus(E)P(")Pn(En).Letting"!0,wehavem(E)nm(En)somiscountablysubadditive.53a.IfEisaBorelsetandhBiiisasequenceofballscoveringEwithradiiri<",thenhBi+yiisasequenceofballscoveringE+ywithradiiri.Conversely,ifhBiiisasequenceofballscoveringE+ywithradiiri<",thenhBi�yiisasequenceofballscoveringEwithradiiri.Itfollowsthat(")(")(E+y)=(E).Letting"!0,wehavem(E+y)=m(E).53b.Sincemisinvariantundertranslations,itsucestoconsiderrotationsabout0.LetTdenoterotationabout0.IfhBiiisasequenceofballscoveringEwithradiiri<",thenhT(Bi)iisasequence(")(")ofballscoveringT(E)withradiiri.Itfollowsthat(E)=(T(E))forall">0.Letting"!0,91khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com
wehavem(E)=m(T(E)).*54.*55a.LetEbeaBorelsubsetofsomemetricspaceX.Supposem(E)is
niteforsome.55b.Supposem(E)>0forsome.Ifm(E)<1forsome
>
,thenbypart(a),m(E)=0.Contradiction.Hencem(E)=1forall
>
.55c.LetI=inff:m(E)=1g.If
>I,thenm(E)=1bypart(a).ThusIisanupperboundforf:m(E)=0g.IfU0,choosensuchthat3�n<".ThensincetheCantorternarysetCcanbecoveredby2nintervalsoflength3�n,wehave(")n(3�n)=1.Thusm(C)1.(C)2Conversely,given">0,ifhIniisanysequenceofopenintervalscoveringCandwithlengthslessthan",thenwemayenlargeeachintervalslightlyandusecompactnessofCtoreducetothecaseofa
nitecollectionofclosedintervals.WemayfurthertakeeachItobethesmallestintervalcontainingsomepairofintervalsJ;J0fromtheconstructionofC.IfJ;J0arethelargestsuchintervals,thenthereisanintervalKCcbetweenthem.Nowl(I)s(l(J)+l(K)+l(J0))s(3(l(J)+l(J0)))s=2(1(l(J))s+1(l(J0))s)222(l(J))khdaw.coms+(l(J0))s.Proceedinthiswayuntil,aftera
nitenumberofsteps,wereachacoveringofCbyequalintervalsoflength3�j.Thismustincludeallintervalsoflength3�jintheconstructionofC.ItP(")followsthatl(In)1.Thus(C)1forall">0andm(C)1.Sincem(C)=1,byparts(a)and(b),wehavem(C)=0for0<
<
andm(C)=1for
>
.HencetheHausdor
dimensionofCis=log2=log3.13MeasureandTopology13.1BairesetsandBorelsets1.[Incomplete:Q5.20c,Q5.22c,d,Q7.42b,d,Q7.46a,Q8.17b,Q8.29,Q8.40b,c,Q9.38,Q9.49,Q9.50,Q10.47,Q10.48b,Q10.49g,Q11.5d,Q11.6c,Q11.8d,Q11.9d,e,Q11.37b,c,d,e,Q11.46b,Q11.47a,b,Q11.48,Q12.9b,Q12.11b,Q12.18,Q12.27,Q12.33,Q12.34,Q12.38,Q12.43c,Q12.44,Q12.45a,b,Q12.48c,Q12.50,Q12.54,Q12.55a]课后答案网www.hackshp.cn92khdaw.com若侵犯了您的版权利益,敬请来信通知我们!℡www.khdaw.com'
您可能关注的文档
- 商务与经济统计 第8版 英文版 (戴维 R. 安德森 著) 机械工业出版社 课后答案
- 商务与经济统统计 第八版 (安德森 著) 中信出版社 课后答案
- 商业银行经营学 第三版 (戴国强 著) 高等教育出版社 课后答案
- 射频通信电路 第二版 (陈邦媛 著) 科学出版社 课后答案
- 渗流力学课后题答案
- 生物化学解题指导与测验_张楚富_高等教育出版社
- 声学基础 (许肖梅 著) 科学出版社 课后答案
- 石油化学工程原理 (李阳初 刘雪暖 着)
- 实变函数与反函分析 (宋国柱 著) 科学出版社 课后答案
- 实分析与复分析 (Walter Rudin 著) 机械工业出版社 课后答案
- 实用大学英语 第一册 (谢利霞 刘菊玲 郝帅 著) 武汉大学出版社 听力原文和课后答案
- 实用数据结构 (C++描述) 第二版 (徐士良 葛兵 著) 清华大学出版社 课后答案
- 实用英语综合教程 1 (教育部《实用英语》教材编写组 著) 高等教育出版社 课后答案
- 世纪商务英语 外贸英语实务 (侯雁慧 著) 大连理工大学出版社 课后答案
- 世界经济史 (高德步 著) 人民大学出版社 课后答案
- 市场调查与预测 (闫秀荣 著) 上海财经大学出版社 课后答案
- 数据结构 (戴敏 著) 机械工业出版社 课后答案
- 数据结构 (熊回香 王伟军 著) 清华大学出版社 北京交通大学出版社 部分答案 课后答案
相关文档
- 施工规范CECS140-2002给水排水工程埋地管芯缠丝预应力混凝土管和预应力钢筒混凝土管管道结构设计规程
- 施工规范CECS141-2002给水排水工程埋地钢管管道结构设计规程
- 施工规范CECS142-2002给水排水工程埋地铸铁管管道结构设计规程
- 施工规范CECS143-2002给水排水工程埋地预制混凝土圆形管管道结构设计规程
- 施工规范CECS145-2002给水排水工程埋地矩形管管道结构设计规程
- 施工规范CECS190-2005给水排水工程埋地玻璃纤维增强塑料夹砂管管道结构设计规程
- cecs 140:2002 给水排水工程埋地管芯缠丝预应力混凝土管和预应力钢筒混凝土管管道结构设计规程(含条文说明)
- cecs 141:2002 给水排水工程埋地钢管管道结构设计规程 条文说明
- cecs 140:2002 给水排水工程埋地管芯缠丝预应力混凝土管和预应力钢筒混凝土管管道结构设计规程 条文说明
- cecs 142:2002 给水排水工程埋地铸铁管管道结构设计规程 条文说明