- 3.57 MB
- 2022-04-22 11:41:06 发布
- 1、本文档共5页，可阅读全部内容。
- 2、本文档内容版权归属内容提供方，所产生的收益全部归内容提供方所有。如果您对本文有版权争议，可选择认领，认领后既往收益都归您。
- 3、本文档由用户上传，本站不保证质量和数量令人满意，可能有诸多瑕疵，付费之前，请仔细先通过免费阅读内容等途径辨别内容交易风险。如存在严重挂羊头卖狗肉之情形，可联系本站下载客服投诉处理。
- 文档侵权举报电话：19940600175。
'SOLUTIONSMANUALtoaccompanyDigitalSignalProcessing:AComputer-BasedApproachSecondEditionSanjitK.MitraPreparedbyRajeevGandhi,SerkanHatipoglu,ZhihaiHe,LucaLucchese,MichaelMoore,andMyleneQueirozdeFarias1
ErrataListof"DigitalSignalProcessing:AComputer-BasedApproach",SecondEditionChapter21.Page48,Eq.(2.17):Replace"y[n]"with"xu[n]".12.Page,51:Eq.(2.24a):Delete"(x[n]+x*[N-n])".Eq.(2.24b):Delete21"(x[n]-x*[N-n])".23.Page59,Line5fromtopandline2frombottom:Replace"-cos((w1+w2-p)n)"with"cos((2p–w1–w2)n)".4.Page61,Eq.(2.52):Replace"Acos((Wo+kWT)t+f)"with"Acos(±(Wot+f)+kWTt)".5.Page62,line11frombottom:Replace"WT>2Wo"with"WT>2Wo".6.Page62,line8frombottom:Replace"2pWo/wT"with"2pWo/WT".7.Page65,Program2_4,line7:Replace"x=s+d;"with"x=s+d";".¥¥nn8.Page79,line5belowEq.(2.76):Replace"åa"with"åa".n=0n=0nnnn9.Page81,Eq.(2.88):Replace"aL+1l2+aNlN-L"with"aL+1l2+L+aNlN-L".10.Page93,Eq.(2.116):Replacethelowerlimit"n=–M+1"onallsummationsignswith"n=0".11.Page100,linebelowEq.(2.140)andcaptionofFigure2.38:Replace"wo=0.03"with"wo=0.06p".12.Page110,Problem2.44:Replace"{y[n]}={-1,-1,11,-3,-10,20,-16}"with"{y[n]}={-1,-1,11,-3,30,28,48}",and"{y[n]}={-14-j5,-3-j17,-2+j5,-26+j22,9+j12}"with"{y[n]}={-14-j5,-3-j17,-2+j5,-9.73+j12.5,5.8+j5.67}".13.Page116,ExerciseM2.15:Replace"randn"with"rand".Chapter31.Page118,line10belowEq.(3.4):Replace"real"with"even".2
¥¥nn2.Page121,Line5belowEq.(3.9):Replace"åa"with"åa".n=0n=03.Page125,Eq.(3.16):Deletethestraya.4.Page138,line2belowEq.(3.48):Replace"frequencyresponse"with"discrete-timeFouriertransform".5.Page139,Eq.(3.53):Replace"x(n+mN)"with"x[n+mN]".6.Page139,lin22,Example3.14:Replace"x[n]={012345}"with"{x[n]}={012345}".7.Page139,line3,Example3.14:Replace"x[n]"with"{x[n]}",and"pk/4"with"2pk/4".8.Page139,line6frombottom:Replace"y[n]={462346}"with"{y[n]}={4623}".9.Page141,Table3.5:Replace"N[g<-k>N]"with"Ng[<-k>N]".10.Page142,Table3.7:Replace"argX[<-k>N]"with"–argX[<-k>N]".é1111ùé1111ùêúêú1j-1-j1-j-1j11.Page147,Eq.(3.86):Replace"êú"with"êú".ê1-11-1úê1-11-1úêúêúë1-j-1jûë1j-1-jû-1-1n-nn-n12.Page158,Eq.(3.112):Replace"åaz"with"-åaz".n=-¥n=-¥13.Page165,line4aboveEq.(3.125);Replace"0.0667"with"0.6667".14.Page165,line3aboveEq.(3.125):Replace"10.0000"with"1.000",and"20.0000"with"2.0000".15.Page165,lineaboveEq.(3.125):Replace"0.0667"with"0.6667","10.0"with"1.0",and"20.0"with"2.0".16.Page165,Eq.(3.125):Replace"0.667"with"0.6667".17.Page168,linebelowEq.(3.132):Replace"z>ll"with"z>ll".18.Page176,linebelowEq.(3.143):Replace"Rh"with"1/Rh".-jw/2jw/219.Page182,Problem3.18:Replace"X(e)"with"X(-e)".3
20.Page186,Problem3.42,Part(e):Replace"argX[<-k>N]"with"–argX[<-k>N]".21.Page187,Problem3.53:Replace"N-pointDFT"with"MN-pointDFT",replace"0£k£N-1"with"0£k£MN-1",andreplace"x[M]"with"x[N]".22.Page191,Problem3.83:Replace"lim"with"lim".n®¥z®¥P(z)P(z)23.Page193,Problem3.100:Replace""with"-ll".D"(z)D"(z)24.Page194,Problem3.106,Parts(b)and(d):Replace"z1/a".mnm25.page199,Problem3.128:Replace"(0.6)[n]"with"(0.6)m[n]",andreplace"(0.8)[n]"nwith"(0.8)m[n]".26.Page199,ExerciseM3.5:Delete"following".Chapter41.Page217,firstline:Replace"xN"with"xM".2.Page230,line2belowEq.(4.88):Replace"qg(w)"with"q(w)".3.Page236,line2belowEq.(4.109):Replace"decreases"with"increases".jw4.Page246,line4belowEq.(4.132):Replace"qc(e)"with"qc(w)".5.Page265,Eq.(4.202):Replace"1,2,K,3"with"1,2,3".j0jp/46.Page279,Problem4.18:Replace"H(e)"with"H(e)".7.Page286,Problem4.71:Replace"z3=-j0.3"with"z3=-0.3".8.Page291,Problem4.102:Replace-1-2-3-4-50.4+0.5z+1.2z+1.2z+0.5z+0.4z"H(z)="with1+0.9z-2+0.2z-4-1-2-3-4-50.1+0.5z+0.45z+0.45z+0.5z+0.1z"H(z)=".1+0.9z-2+0.2z-49.Page295,Problem4.125:Insertacomma","before"theautocorrelation".Chapter51.Page302,line7belowEq.(5.9):Replace"response"with"spectrum".4
2.Page309,Example5.2,line4:Replace"10Hzto20Hz"with"5Hzto10Hz".Line6:Replace"5k+15"with"5k+5".Line7:Replace"10k+6"with"5k+3",andreplace"10k–6"with"5(k+1)–3".3.Page311,Eq.(5.24):Replace"Ga(jW-2k(DW))"with"Ga(j(W-2k(DW)))".4.Page318,Eq.(5.40):Replace"H(s)"with"Ha(s)",andreplace""with"".5.Page321,Eq.(5.54):Replace""with"".6.Page333,firstline:Replace"Wp1"with"Wˆp1",and"Wp2"with"Wˆp2".7.Page349,line9frombottom:Replace"1/T"with"2p/T".8.Page354,Problem5.8:Interchange"W1"and"W2".9.Page355,Problem5.23:Replace"1Hz"inthefirstlinewith"0.2Hz".10.Page355,Problem5.24:Replace"1Hz"inthefirstlinewith"0.16Hz".Chapter61.Page394,line4frombottom:Replace"alpha1"with"fliplr(alpha1)".2.Page413,Problem6.16:Replaceæ-1-1-1-1-1-1ö"H(z)=b0+b1èz+b2z(z+b3z(1+L+bN-1z(1+bNz)))ø"with-1æ-1-1-1-1-1ö"H(z)=b0+b1zè1+b2z(z+b3z(1+L+bN-1z(1+bNz)))ø".23z+18.5z+17.53.Page415,Problem6.27:Replace"H(z)="with(2z+1)(z+2)23z+18.5z+17.5"H(z)=".(z+0.5)(z+2)4.Page415,Problem6.28:Replacethemultipliervalue"0.4"inFigureP6.12with"–9.75".-3-35.Page421,ExerciseM6.1:Replace"-7.6185z"with"-71.6185z".6.Page422,ExerciseM6.4:Replace"Program6_3"with"Program6_4".7.Page422,ExerciseM6.5:Replace"Program6_3"with"Program6_4".8.Page422,ExerciseM6.6:Replace"Program6_4"with"Program6_6".5
Chapter71.Page426,Eq.(7.11):Replace"h[n–N]"with"h[N–n]".2.Page436,line14fromtop:Replace"(5.32b)"with"(5.32a)".3.Page438,line17frombottom:Replace"(5.60)"with"(5.59)".4.Page439,line7frombottom:Replace"50"with"40".-15.Page442,linebelowEq.(7.42):Replace"F(ˆz)"with"1/F(ˆz)".-16.Page442,lineaboveEq.(7.43):Replace"F(ˆz)"with"F(ˆz)".Læzˆ-aö7.Page442,Eq.(7.43):Replaceitwith"F(ˆz)=±lÕç÷".l=1è1-al*ˆzø8.Page442,linebelowEq.(7.43):Replace"whereal"with"whereal".9.Page446,Eq.(7.51):Replace"b(1-a)"with"b(1+a)".10.Page448,Eq.(7.58):Replace"wc1.nSincebbecomesarbitrarilylargeasnincreaseshence{h[n]}isnotaboundedsequence.8
(d){g[n]}=4sin(wn).Since-4£g[n]£4forallvaluesofn,{g[n]}isaboundedasequence.22(e){v[n]}=3cos(wn).Since-3£v[n]£3forallvaluesofn,{v[n]}isaboundedbsequence.12.8(a)Recall,x[n]=(x[n]+x[-n]).ev2Sincex[n]isacausalsequence,thusx[–n]=0"n>0.Hence,x[n]=x[n]+x[-n]=2x[n],"n>0.Forn=0,x[0]=xev[0].evevevThusx[n]canbecompletelyrecoveredfromitsevenpart.1ìï1x[n],n>0,Likewise,x[n]=(x[n]–x[-n])=í2od20,n=0.îïThusx[n]canberecoveredfromitsoddpart"nexceptn=0.(b)2y[n]=y[n]-y*[-n].Sincey[n]isacausalsequencey[n]=2y[n]"n>0.cacaForn=0,Im{y[0]}=y[0].Hencerealpartofy[0]cannotbefullyrecoveredfromy[n].cacaThereforey[n]cannotbefullyrecoveredfromy[n].ca2y[n]=y[n]+y*[-n].Hence,y[n]=2y[n]"n>0.cscsForn=0,Re{y[0]}=y[0].Henceimaginarypartofy[0]cannotberecoveredfromy[n].cscsThereforey[n]cannotbefullyrecoveredfromy[n].cs112.9x[n]=(x[n]+x[-n]).Thisimplies,x[–n]=(x[–n]+x[n])=x[n].ev2ev2evHenceevenpartofarealsequenceiseven.11x[n]=(x[n]–x[–n]).Thisimplies,x[–n]=(x[–n]–x[n])=–x[n].od2od2odHencetheoddpartofarealsequenceisodd.112.10RHSofEq.(2.176a)isx[n]+x[n-N]=(x[n]+x*[-n])+(x[n-N]+x*[N-n]).cscs22Sincex[n]=0"n<0,Hence1x[n]+x[n-N]=(x[n]+x*[N-n])=x[n],0£n£N–1.cscs2pcsRHSofEq.(2.176b)is11x[n]+x[n-N]=(x[n]-x*[-n])+(x[n-N]-x*[n-N])caca221=(x[n]-x*[N-n])=x[n],0£n£N–1.2pca9
12.11x[n]=(x[n]+x*[<-n>])for0£n£N–1,Since,x[<-n>]=x[N-n],itfollowspcs2NN1thatx[n]=(x[n]+x*[N-n]),1£n£N–1.pcs21Forn=0,x[0]=(x[0]+x*[0])=Re{x[0]}.pcs211Similarlyx[n]=(x[n]-x*[<-n>])=(x[n]-x*[N-n]),1£n£N–1.Hence,pca2N21forn=0,xpca[0]=(x[0]-x*[0])=jIm{x[0]}.2¥2.12(a)Givenåx[n]<¥.Therefore,bySchwartzinequality,n=-¥¥2æ¥öæ¥öån=-¥x[n]£çån=-¥x[n]÷çån=-¥x[n]÷<¥.èøèø1/n,n³1,(b)Considerx[n]=Theconvergenceofaninfiniteseriescanbeshown{0,otherwise.viatheintegraltest.Letan=f(x),wheref(x)isacontinuous,positiveanddecreasing¥¥functionforallx³1.Thentheseriesån=1anandtheintegralò1f(x)dxbothconvergeor¥1¥bothdiverge.Foran=1/n,f(x)=1/n.Butò1dx=(lnx)1=¥-0=¥.Hence,x¥¥1ån=-¥x[n]=ån=1ndoesnotconverge,andasaresult,x[n]isnotabsolutely1summable.Toshow{x[n]}issquare-summable,weobserveherean=2,andthus,n¥1¥1æ1ö11¥1f(x)=2.Now,ò12dx=çè-x÷ø=-¥+1=1.Hence,ån=12converges,orinotherxx1nwords,x[n]=1/nissquare-summable.2.13SeeProblem2.12,Part(b)solution.coswn¥æcoswnö2¥1¥1p2cc2.14x2[n]=pn,1£n£¥.Now,ån=1çpn÷£ån=1p2n2.Since,ån=1n2=6,èø¥æcoswnö21cån=1ç÷£.Thereforex2[n]issquare-summable.èpnø6Usingintegraltestwenowshowthatx[n]isnotabsolutelysummable.2¥coswxc¥coswcx1xdx=×x×cosint(wx)wherecosintisthecosineintegralfunction.ò1pxpcoswxcc1¥coswx¥coswnccSinceòdxdiverges,ån=1alsodiverges.1pxpn10
¥¥222.15åx[n]=å(xev[n]+xod[n])n=-¥n=-¥¥¥¥¥¥2222=åxev[n]+åxod[n]+2åxev[n]xod[n]=åxev[n]+åxod[n]n=-¥n=-¥n=-¥n=-¥n=-¥¥asån=–¥xev[n]xod[n]=0sincexev[n]xod[n]isanoddsequence.2.16x[n]=cos(2pkn/N),0£n£N–1.Hence,N-1N-1N-121N1Ex=åcos(2pkn/N)=2å(1+cos(4pkn/N))=2+2åcos(4pkn/N).n=0n=0n=0N-1N-1LetC=åcos(4pkn/N),andS=åsin(4pkn/N).n=0n=0N-1j4pkj4pkn/Ne-1ThereforeC+jS=åe=j4pk/N=0.Thus,C=Re{C+jS}=0.e-1n=0NAsC=Re{C+jS}=0,itfollowsthatE=.x2¥2¥22.17(a)x1[n]=m[n].Energy=ån=-¥m[n]=ån=-¥1=¥.1K21K2K1Averagepower=lim2K+1ån=-K(m[n])=lim2K+1ån=01=lim2K+1=2.K®¥K®¥K®¥¥2¥2(b)x2[n]=nm[n].Energy=ån=-¥(nm[n])=ån=-¥n=¥.1K21K2Aveeragepower=lim2K+1ån=-K(nm[n])=lim2K+1ån=0n=¥.K®¥K®¥jwn¥jwn2¥2(c)xo.Energy=Ao=A3[n]=Aoeòn=-¥oeån=-¥o=¥.Averagepower=12122KA2KjwonKo2lim2K+1ån=-KAoe=lim2K+1ån=-KAo=lim2K+1=Ao.K®¥K®¥K®¥æ2pnöjwon-jwon2pAjf(d)x[n]=Asinç+f÷=Aoe+A1e,wherewo=,Ao=-eandèMøM2A-jf222232A1=e.FromPart(c),energy=¥andaveragepower=Ao+A1+4AoA1=A.24ì1,n³0,ì1,n<0,2.18Now,m[n]=íHence,m[-n-1]=íThus,x[n]=m[n]+m[-n-1].î0,n<0.î0,n³0.n2.19(a)Considerasequencedefinedbyx[n]=åd[k].k=-¥11
Ifn<0thenk=0isnotincludedinthesumandhencex[n]=0,whereasforn³0,k=0isnì1,n³0,includedinthesumhencex[n]=1"n³0.Thusx[n]=åd[k]=í=m[n].îï0,n<0,k=-¥ì1,n³0,ì1,n³1,(b)Sincem[n]=íitfollowsthatm[n–1]=íîï0,n<0,îï0,n£0.1,n=0,Hence,m[n]–m[n-1]==d[n].{0,n¹0,2.20Nowx[n]=Asin(w0n+f).(a)Givenx[n]={0-2-2-20222}.ThefundamentalperiodisN=4,hencewo=2p/8=p/4.Nextfromx[0]=Asin(f)=0wegetf=0,andsolvingpx[1]=Asin(+f)=Asin(p/4)=-2wegetA=–2.4(b)Givenx[n]={22-2-2}.ThefundamentalperiodisN=4,hencew=2p/4=p/2.Nextfromx[0]=Asin(f)=2andx[1]=Asin(p/2+f)=Acos(f)=2it0canbeseenthatA=2andf=p/4isonesolutionsatisfyingtheaboveequations.(c)x[n]={3-3}.HerethefundamentalperiodisN=2,hencew=p.Nextfromx[0]0=Asin(f)=3andx[1]=Asin(f+p)=-Asin(f)=-3observethatA=3andf=p/2thatA=3andf=p/2isonesolutionsatisfyingthesetwoequations.(d)Givenx[n]={01.50-1.5},itfollowsthatthefundamentalperiodofx[n]isN=4.Hencew0=2p/4=p/2.Solvingx[0]=Asin(f)=0wegetf=0,andsolvingx[1]=Asin(p/2)=1.5,wegetA=1.5.-j0.4pn2.21(a)x˜1[n]=e.Here,wo=0.4p.FromEq.(2.44a),wethusget2pr2prN===5r=5forr=1.wo0.4p(b)x˜2[n]=sin(0.6pn+0.6p).Here,wo=0.6p.FromEq.(2.44a),wethusget2pr2pr10N===r=10forr=3.wo0.6p3(c)x˜3[n]=2cos(1.1pn-0.5p)+2sin(0.7pn).Here,w1=1.1pandw2=0.7p.FromEq.2pr12pr1202pr22pr220(2.44a),wethusgetN1===r1andN2===r2.Tobeperiodicw11.1p11w20.7p712
2020wemusthaveN1=N2.Thisimplies,r1=r2.Thisequalityholdsforr1=11andr2=7,117andhenceN=N1=N2=20.2pr1202pr220(d)N1==r1andN2==r2.ItfollowsfromtheresultsofPart(c),N=201.3p130.3p3withr1=13andr2=3.2pr152pr25(e)N1==r1,N2==r2andN3=N2.Therefore,N=N1=N2=N2=5for1.2p30.8p2r1=3andr2=2.(f)x˜6[n]=nmodulo6.Sincex˜6[n+6]=(n+6)modulo6=nmodulo6=x˜6[n].HenceN=6isthefundamentalperiodofthesequencex˜[n].62pr2pr1002.22(a)wo=0.14p.Therefore,N===r=100forr=7.wo0.14p72pr2pr25(b)wo=0.24p.Therefore,N===r=25forr=3.wo0.24p32pr2pr100(c)wo=0.34p.Therefore,N===r=100forr=17.wo0.34p172pr2pr8(d)wo=0.75p.Therefore,N===r=8forr=3.wo0.75p32.23x[n]=xa(nT)=cos(WonT)isaperiodicsequenceforallvaluesofTsatisfyingWoT×N=2prforrandNtakingintegervalues.Since,WoT=2pr/Nandr/Nisarationalnumber,WoTmustalsoberationalnumber.ForWo=18andT=p/6,wegetN=2r/3.Hence,thesmallestvalueofN=3forr=3.2.24(a)x[n]=3d[n+3]-2d[n+2]+d[n]+4d[n-1]+5d[n-2]+2d[n-3](b)y[n]=7d[n+2]+d[n+1]-3d[n]+4d[n-1]+9d[n-2]-2d[n-3](c)w[n]=-5d[n+2]+4d[n+2]+3d[n+1]+6d[n]-5d[n-1]+d[n-3]2.25(a)Foraninputxi[n],i=1,2,theoutputisyi[n]=a1xi[n]+a2xi[n-1]+a3xi[n-2]+a4xi[n-4],fori=1,2.Then,foraninputx[n]=Ax1[n]+Bx2[n],theoutputisy[n]=a1(Ax1[n]+Bx2[n])+a2(Ax1[n-1]+Bx2[n-1])+a3(Ax1[n-2]+Bx2[n-2])+a4(Ax1[n-3]+Bx2[n-3])=A(a1x1[n]+a2x1[n-1]+a3x1[n-2]+a4x1[n-4])+B(a1x2[n]+a2x2[n-1]+a3x2[n-2]+a4x2[n-4])=Ay1[n]+By2[n].Hence,thesystemislinear.13
(b)Foraninputxi[n],i=1,2,theoutputisyi[n]=b0xi[n]+b1xi[n-1]+b2xi[n-2]+a1yi[n-1]+a2yi[n-2],i=1,2.Then,foraninputx[n]=Ax1[n]+Bx2[n],theoutputisy[n]=A(b0x1[n]+b1x1[n-1]+b2x1[n-2]+a1y1[n-1]+a2y1[n-2])+B(b0x2[n]+b1x2[n-1]+b2x2[n-2]+a1y2[n-1]+a2y2[n-2])=Ay1[n]+By2[n].Hence,thesystemislinear.ìxi[n/L],n=0,±L,±2L,K(c)Foraninputxi[n],i=1,2,theoutputisyi[n]=,íî0,otherwise,Considertheinputx[n]=Ax1[n]+Bx2[n],Thentheoutputy[n]forn=0,±L,±2L,Kisgivenbyy[n]=x[n/L]=Ax1[n/L]+Bx2[n/L]=Ay1[n]+By2[n].Forallothervaluesofn,y[n]=A×0+B×0=0.Hence,thesystemislinear.(d)Foraninputxi[n],i=1,2,theoutputisyi[n]=xi[n/M].Considertheinputx[n]=Ax1[n]+Bx2[n],Thentheoutputy[n]=Ax1[n/M]+Bx2[n/M]=Ay1[n]+By2[n].Hence,thesystemislinear.1M-1(e)Foraninputxi[n],i=1,2,theoutputisyi[n]=Måk=0xi[n-k].Then,foran1M-1inputx[n]=Ax1[n]+Bx2[n],theoutputisyi[n]=Måk=0(Ax1[n-k]+Bx2[n-k])æ1M-1öæ1M-1ö=AçèMåk=0x1[n-k]÷ø+BçèMåk=0x2[n-k]÷ø=Ay1[n]+By2[n].Hence,thesystemislinear.(f)ThefirsttermontheRHSofEq.(2.58)istheoutputofanup-sampler.ThesecondtermontheRHSofEq.(2.58)issimplytheoutputofanunitdelayfollowedbyanup-sampler,whereas,thethirdtermistheoutputofanunitadavanceoperatorfollowedbyanup-samplerWehaveshowninPart(c)thattheup-samplerisalinearsystem.Moreover,thedelayandtheadvanceoperatorsarelinearsystems.Acascadeoftwolinearsystemsislinearandalinearcombinationoflinearsystemsisalsolinear.Hence,thefactor-of-2interpolatorisalinearsystem.(g)FollowingtheargumentsgiveninPart(f),itcanbeshownthatthefactor-of-3interpolatorisalinearsystem.2.26(a)y[n]=n2x[n].Foraninputxi[n]theoutputisyi[n]=n2xi[n],i=1,2.Thus,foraninputx3[n]=Ax1[n]2+Bx2[n],theoutputy3[n]isgivenbyy3[n]=n(Ax1[n]+Bx2[n])=Ay1[n]+By2[n].Hencethesystemislinear.Sincethereisnooutputbeforetheinputhencethesystemiscausal.However,y[n]beingproportionalton,itispossiblethataboundedinputcanresultinanunboundedoutput.Letx[n]=1"n,theny[n]=n2.Hencey[n]®¥asn®¥,hencenotBIBOstable.Lety[n]betheoutputforaninputx[n],andlety1[n]betheoutputforaninputx1[n].If222x[n]=x[n-n]theny[n]=nx[n]=nx[n-n].However,y[n-n]=(n-n)x[n-n].10110000Sincey[n]¹y[n-n],thesystemisnottime-invariant.1014
4(b)y[n]=x[n].Foraninputx1[n]theoutputisyi[n]=xi4[n],i=1,2.Thus,foraninputx3[n]=Ax1[n]+44444Bx2[n],theoutputy3[n]isgivenbyy3[n]=(Ax1[n]+Bx2[n])¹Ax1[n]+Ax2[n]Hencethesystemisnotlinear.Sincethereisnooutputbeforetheinputhencethesystemiscausal.Here,aboundedinputproducesboundedoutputhencethesystemisBIBOstabletoo.Lety[n]betheoutputforaninputx[n],andlety1[n]betheoutputforaninputx1[n].If44x1[n]=x[n-n0]theny1[n]=x1[n]=x[n-n0]=y[n-n0].Hence,thesystemistime-invariant.3(c)y[n]=b+åx[n-l].l=03Foraninputxi[n]theoutputisyi[n]=b+åxi[n-l],i=1,2.Thus,foraninputx3[n]=l=0Ax1[n]+Bx2[n],theoutputy3[n]isgivenby333y[n]=b+å(Ax1[n-l]+Bx2[n-l])=b+åAx1[n-l]+åBx2[n-l]l=0l=0l=0¹Ay1[n]+By2[n].Sinceb¹0hencethesystemisnotlinear.Sincethereisnooutputbeforetheinputhencethesystemiscausal.Here,aboundedinputproducesboundedoutputhencethesystemisBIBOstabletoo.Alsofollowingananalysissimilartothatinpart(a)itiseasytoshowthatthesystemistime-invariant.3(d)y[n]=b+åx[n-l]l=–33Foraninputxi[n]theoutputisyi[n]=b+åxi[n-l],i=1,2.Thus,foraninputx3[n]=l=–3Ax1[n]+Bx2[n],theoutputy3[n]isgivenby333y[n]=b+å(Ax1[n-l]+Bx2[n-l])=b+åAx1[n-l]+åBx2[n-l]l=-3l=-3l=-3¹Ay1[n]+By2[n].Sinceb¹0hencethesystemisnotlinear.Sincethereisoutputbeforetheinputhencethesystemisnon-causal.Here,aboundedinputproducesboundedoutputhencethesystemisBIBOstable.15
Lety[n]betheoutputforaninputx[n],andlety1[n]betheoutputforaninputx1[n].If33x1[n]=x[n-n0]theny1[n]=b+åx1[n-l]=b+åx1[n-n0-l]=y[n-n0].Hencethel=–3l=–3systemistime-invariant.(e)y[n]=ax[-n]Thesystemislinear,stable,noncausal.Lety[n]betheoutputforaninputx[n]andy[n]1betheoutputforaninputx1[n].Theny[n]=ax[-n]andy1[n]=ax1[-n].Letx1[n]=x[n-n0],theny1[n]=ax1[-n]=ax[-n-n0],whereasy[n-n0]=ax[n0-n].Hencethesystemistime-varying.(f)y[n]=x[n–5]Thegivensystemislinear,causal,stableandtime-invariant.2.27y[n]=x[n+1]–2x[n]+x[n–1].Lety[n]betheoutputforaninputx[n]andy[n]betheoutputforaninputx[n].Then1122foraninputx[n]=ax[n]+bx[n]theoutputy[n]isgivenby3123y[n]=x[n+1]-2x[n]+x[n-1]3333=ax[n+1]-2ax[n]+ax[n-1]+bx[n+1]-2bx[n]+bx[n-1]111222=ay[n]+by[n].12Hencethesystemislinear.Ifx[n]=x[n-n]theny[n]=y[n-n].Hencethesystemis1010time-inavariant.Alsothesystem"simpulseresponseisgivenbyìï-2,n=0,h[n]=í1,n=1,-1,îï0,elsewhere.Sinceh[n]¹0"n<0thesystemisnon-causal.2.28Medianfilteringisanonlinearoperation.Considerthefollowingsequencesastheinputtoamedianfilter:x[n]={3,4,5}andx[n]={2,-2,-2}.Thecorrespondingoutputsofthe12medianfilterarey[n]=4andy[n]=-2.Nowconsideranotherinputsequencex3[n]=12x1[n]+x2[n].Thenthecorrespondingoutputisy[n]=3,Ontheotherhand,3y[n]+y[n]=2.Hencemedianfilteringisnotalinearoperation.Totestthetime-12invarianceproperty,letx[n]andx1[n]bethetwoinputstothesystemwithcorrepondingoutputsy[n]andy1[n].Ifx[n]=x[n-n]then10y[n]=median{x[n-k],.......,x[n],.......x[n+k]}1111=median{x[n-k-n],.......,x[n-n],.......x[n+k-n]}=y[n-n].0000Hencethesystemistimeinvariant.16
1æx[n]ö2.29y[n]=ççy[n-1]+÷÷2èy[n-1]øNowforaninputx[n]=am[n],theouputy[n]convergestosomeconstantKasn®¥.1æaöTheabovedifferenceequationasn®¥reducestoK=çK+÷whichisequivalentto2èKø2K=aorinotherwords,K=a.Itiseasytoshowthatthesystemisnon-linear.Nowassumey1[n]betheoutputforan1æx1[n]öinputx1[n].Theny[n]=çy[n-1]+÷12çè1y[n-1]÷ø11æx[n-n0]öIfx[n]=x[n-n].Then,y[n]=çy[n-1]+÷.1012çè1y[n-1]÷ø1Thusy[n]=y[n-n].Hencetheabovesystemistimeinvariant.102.30Foraninputxi[n],i=1,2,theinput-outputrelationisgivenby2yi[n]=xi[n]-yi[n-1]+yi[n-1].Thus,foraninputAx1[n]+Bx2[n],iftheoutputisAy1[n]+By2[n],thentheinput-outputrelationisgivenbyAy1[n]+By2[n]=2Ax1[n]+Bx2[n]-(Ay1[n-1]+By2[n-1])+Ay1[n-1]+By2[n-1]=Ax1[n]+Bx2[n]2222-Ay1[n-1]-By2[n-1]+2ABy1[n-1]y2[n-1]+Ay1[n-1]+By2[n-1]2222¹Ax1[n]-Ay1[n-1]+Ay1[n-1]+Bx2[n]-By2[n-1]+By2[n-1].Hence,thesystemisnonlinear.Lety[n]betheoutputforaninputx[n]whichisrelatedtox[n]through2y[n]=x[n]-y[n-1]+y[n-1].Thentheinput-outputrealtionforaninputx[n-no]isgiven2byy[n-no]=x[n-no]-y[n-no-1]+y[n-no-1],orinotherwords,thesystemistime-invariant.Nowforaninputx[n]=am[n],theouputy[n]convergestosomeconstantKasn®¥.22Theabovedifferenceequationasn®¥reducestoK=a-K+K,orK=a,i.e.K=a.2.31Asd[n]=m[n]-m[n-1],T{d[n]}=T{m[n]}-T{m[n-1]}Þh[n]=s[n]-s[n-1]ForadiscreteLTIsystem¥¥¥¥y[n]=åh[k]x[n-k]=å(s[k]-s[k-1])x[n-k]=ås[k]x[n-k]-ås[k-1]x[n-k]k=-¥k=-¥k=-¥k=-¥¥¥2.32y[n]=åm=-¥h[m]x˜[n-m].Hence,y[n+kN]=åm=-¥h[m]x˜[n+kN-m]¥=åm=-¥h[m]x˜[n-m]=y[n].Thus,y[n]isalsoaperiodicsequencewithaperiodN.17
¥2.33Nowd[n-r]*d[n-s]=åm=-¥d[m-r]d[n-s-m]=d[n-r-s](a)y1[n]=x1[n]*h1[n]=(2d[n-1]-0.5d[n-3])*(2d[n]+d[n-1]-3d[n-3])=4d[n-1]*d[n]–d[n-3]*d[n]+2d[n-1]*d[n-1]–0.5d[n-3]*d[n-1]–6d[n-1]*d[n-3]+1.5d[n-3]*d[n-3]=4d[n-1]–d[n-3]+2d[n-1]–0.5d[n-4]–6d[n-4]+1.5d[n-6]=4d[n-1]+2d[n-1]–d[n-3]–6.5d[n-4]+1.5d[n-6](b)y2[n]=x2[n]*h2[n]=(-3d[n-1]+d[n+2])*(-d[n-2]-0.5d[n-1]+3d[n-3])=-0.5d[n+1]-d[n]+3d[n-1]+1.5d[n-2]+3d[n-3]-9d[n-4](c)y3[n]=x1[n]*h2[n]=(2d[n-1]-0.5d[n-3])*(-d[n-2]-0.5d[n-1]+3d[n-3])=-d[n-2]-2d[n-3]-6.25d[n-4]+0.5d[n-5]–1.5d[n-6](d)y4[n]=x2[n]*h1[n]=(-3d[n-1]+d[n+2])*(2d[n]+d[n-1]-3d[n-3])=2d[n+2]+d[n+1]-d[n-1]-3d[n-2]–9d[n-4]N22.34y[n]=åm=N1g[m]h[n-m].Now,h[n–m]isdefinedforM1£n-m£M2.Thus,form=N1,h[n–m]isdefinedforM1£n-N1£M2,orequivalently,forM1+N1£n£M2+N1.Likewise,form=N2,h[n–m]isdefinedforM1£n-N2£M2,orequivalently,forM1+N2£n£M2+N2.(a)Thelengthofy[n]isM2+N2-M1–N1+1.(b)Therangeofnfory[n]¹0ismin(M1+N1,M1+N2)£n£max(M2+N1,M2+N2),i.e.,M1+N1£n£M2+N2.¥2.35y[n]=x1[n]*x2[n]=åk=-¥x1[n-k]x2[k].¥Now,v[n]=x1[n–N1]*x2[n–N2]=åk=-¥x1[n-N1-k]x2[k-N2].Let¥k-N2=m.Thenv[n]=åm=-¥x1[n-N1-N2-m]x2[m]=y[n-N1-N2],2.36g[n]=x1[n]*x2[n]*x3[n]=y[n]*x3[n]wherey[n]=x1[n]*x2[n].Definev[n]=x1[n–N1]*x2[n–N2].Then,h[n]=v[n]*x3[n–N3].FromtheresultsofProblem2.32,v[n]=y[n-N1-N2].Hence,h[n]=y[n-N1-N2]*x3[n–N3].Therefore,usingtheresultsofProblem2.32againwegeth[n]=g[n–N1–N2–N3].18
¥2.37y[n]=x[n]*h[n]=åx[n-k]h[k].Substitutingkbyn-mintheaboveexpression,wek=-¥¥gety[n]=åx[m]h[n-m]=h[n]*x[n].Henceconvolutionoperationiscommutative.m=-¥¥Lety[n]=x[n]*(h1[n]+h2[n])==åx[n-k](h1[k]+h2[k])k=-¥¥k=¥=åx[n-k]h1[k]+åx[n-k]h2[k]=x[n]*h1[n]+x[n]*h2[n].Henceconvolutionisk=-¥k=-¥distributive.2.38x3[n]*x2[n]*x1[n]=x3[n]*(x2[n]*x1[n])Asx2[n]*x1[n]isanunboundedsequencehencetheresultofthisconvolutioncannotbedetermined.Butx2[n]*x3[n]*x1[n]=x2[n]*(x3[n]*x1[n]).Nowx3[n]*x1[n]=0forallvaluesofnhencetheoverallresultiszero.Henceforthegivensequencesx3[n]*x2[n]*x1[n]¹x2[n]*x3[n]*x1[n].2.39w[n]=x[n]*h[n]*g[n].Definey[n]=x[n]*h[n]=åx[k]h[n-k]andf[n]=kh[n]*g[n]=åg[k]h[n-k].Considerw1[n]=(x[n]*h[n])*g[n]=y[n]*g[n]k=åg[m]åx[k]h[n-m-k].Nextconsiderw2[n]=x[n]*(h[n]*g[n])=x[n]*f[n]mk=åx[k]åg[m]h[n-k-m].Differencebetweentheexpressionsforw1[n]andw2[n]iskmthattheorderofthesummationsischanged.A)Assumptions:h[n]andg[n]arecausalfilters,andx[n]=0forn<0.Thisimpliesìï0,form<0,y[m]=ímåx[k]h[m-k],form³0.îïk=0nnn-mThus,w[n]=åg[m]y[n-m]=åg[m]åx[k]h[n-m-k].m=0m=0k=0Allsumshaveonlyafinitenumberofterms.Hence,interchangeoftheorderofsummationsisjustifiedandwillgivecorrectresults.B)Assumptions:h[n]andg[n]arestablefilters,andx[n]isaboundedsequencewith¥k2x[n]£X.Here,y[m]=åk=-¥h[k]x[m-k]=åk=kh[k]x[m-k]+ek,k[m]with112e[m]£eX.k1,k2n19
Inthiscaseallsumshaveeffectivelyonlyafinitenumberoftermsandtheerrorcanbereducedbychoosingk1andk2sufficientlylarge.Henceinthiscasetheproblemisagaineffectivelyreducedtothatoftheone-sidedsequences.Here,againaninterchangeofsummationsisjustifiedandwillgivecorrectresults.Hencefortheconvolutiontobeassociative,itissufficientthatthesequencesbestableandsingle-sided.¥2.40y[n]=åx[n-k]h[k].Sinceh[k]isoflengthManddefinedfor0£k£M–1,thek=-¥(M-1)convolutionsumreducestoy[n]=åx[n-k]h[k].y[n]willbenon-zeroforallthosek=0valuesofnandkforwhichn–ksatisfies0£n-k£N-1.Minimumvalueofn–k=0andoccursforlowestnatn=0andk=0.Maximumvalueofn–k=N–1andoccursformaximumvalueofkatM–1.Thusn–k=M–1Þn=N+M-2.Hencethetotalnumberofnon-zerosamples=N+M–1.¥2.41y[n]=x[n]*x[n]=åx[n-k]x[k].k=-¥Sincex[n–k]=0ifn–k<0andx[k]=0ifk<0hencetheabovesummationreducestoN-1ìïn+1,0£n£N-1,y[n]=åx[n-k]x[k]=íîï2N-n,N£n£2N-2.k=nHencetheoutputisatriangularsequencewithamaximumvalueofN.LocationsoftheNN7Noutputsampleswithvaluesaren=–1and–1.Locationsoftheoutputsamples444NN3Nwithvaluesaren=–1and–1.Note:ItistacitlyassumedthatNisdivisibleby4222Notherwiseisnotaninteger.4N-12.42y[n]=åk=0h[k]x[n-k].Themaximumvalueofy[n]occursatn=N–1whenalltermsintheconvolutionsumarenon-zeroandisgivenbyN-1NN(N+1)y[N-1]=åk=0h[k]=åk=1k=2.¥¥2.43(a)y[n]=gev[n]*hev[n]=åhev[n-k]gev[k].Hence,y[–n]=åhev[-n-k]gev[k].k=-¥k=-¥Replacekby–k.Thenabovesummationbecomes¥¥¥y[-n]=åhev[-n+k]gev[-k]=åhev[-(n-k)]gev[-k]=åhev[(n-k)]gev[k]k=-¥k=-¥k=-¥=y[n].Hencegev[n]*hev[n]iseven.20
¥(b)y[n]=gev[n]*hod[n]=åhod[(n-k)]gev[k].Asaresult,k=-¥¥¥¥y[–n]=åhod[(-n-k)]gev[k]=åhod[-(n-k)]gev[-k]=-åhod[(n-k)]gev[k].k=-¥k=-¥k=-¥Hencegev[n]*hod[n]isodd.¥(c)y[n]=god[n]*hod[n]=åhod[n-k]god[k].Asaresult,k=-¥¥¥¥y[–n]=åhod[-n-k]god[k]=åhod[-(n-k)]god[-k]=åhod[(n-k)]god[k].k=-¥k=-¥k=-¥Hencegod[n]*hod[n]iseven.172.44(a)Thelengthofx[n]is7–4+1=4.Usingx[n]=h[0]{y[n]-åk=1h[k]x[n-k]},wearriveatx[n]={13–212},0£n£319(b)Thelengthofx[n]is9–5+1=5.Usingx[n]=h[0]{y[n]-åk=1h[k]x[n-k]},wearriveatx[n]={11111},0£n£4.15(c)Thelengthofx[n]is5–3+1=3.Usingx[n]=h[0]{y[n]-åk=1h[k]x[n-k]},wegetx[n]={-4+j,-0.6923+j0.4615,3.4556+j1.1065},0£n£2.2.45y[n]=ay[n–1]+bx[n].Hence,y[0]=ay[–1]+bx[0].Next,2y[1]=ay[0]+bx[1]=ay[-1]+abx[0]+bx[1].Continuingfurtherinsimilarwaywenn+1n-kobtainy[n]=ay[-1]+åabx[k].k=0nn+1n-k(a)Lety1[n]betheoutputduetoaninputx1[n].Theny1[n]=ay[-1]+åabx1[k].k=0Ifx[n]=x[n–n]then10nn-n0n+1n-kn+1n-n-ry[n]=ay[-1]+åabx[k-n]=ay[-1]+åa0bx[r].10k=nr=00n-n0n-n+1n-n-rHowever,y[n-n]=a0y[-1]+åa0bx[r].0r=0Hencey[n]¹y[n-n]unlessy[–1]=0.Forexample,ify[–1]=1thenthesystemistime10variant.Howeverify[–1]=0thenthesystemistime-invariant.21
(b)Lety1[n]andy2[n]betheoutputsduetotheinputsx1[n]andx2[n].Lety[n]betheoutputforaninputax[n]+bx[n].However,12nnn+1n+1n-kn-kay1[n]+by2[n]=aay[-1]+bay[-1]+aåabx1[k]+båabx2[k]k=0k=0whereasnnn+1n-kn-ky[n]=ay[-1]+aåabx1[k]+båabx2[k].k=0k=0Hencethesystemisnotlinearify[–1]=1butislinearify[–1]=0.(c)GeneralizingtheaboveresultitcanbeshownthatforanN-thordercausaldiscretetimesystemtobelinearandtimeinvariantwerequirey[–N]=y[–N+1]=L=y[–1]=0.nn2.46ystep[n]=åk=0h[k]m[n-k]=åk=0h[k],n³0,andystep[n]=0,n<0.Sinceh[k]isnonnegative,ystep[n]isamonotonicallyincreasingfunctionofnforn³0,andisnotoscillatory.Hence,noovershoot.n2.47(a)f[n]=f[n–1]+f[n–2].Letf[n]=ar,thenthedifferenceequationreducestonn-1n-221±5ar-ar-ar=0whichreducestor-r-1=0resultinginr=.2nnæ1+5öæ1-5öThus,f[n]=aç÷+aç÷.1çè2÷ø2çè2÷øa+aa-a1212Sincef[0]=0hencea+a=0.Alsof[1]=1hence+5=1.1222nn11æ1+5ö1æ1-5öSolvingforaanda,wegeta=–a=.Hence,f[n]=ç÷-ç÷.121255çè2÷ø5çè2÷ø(b)y[n]=y[n–1]+y[n–2]+x[n–1].AssystemisLTI,theinitialconditionsareequaltozero.Letx[n]=d[n].Then,y[n]=y[n–1]+y[n–2]+d[n-1].Hence,y[0]=y[–1]+y[–2]=0andy[1]=1.Forn>1thecorrespondingdifferenceequationisy[n]=y[n–1]+y[n–2]withinitialconditionsy[0]=0andy[1]=1,whicharethesameasthoseforthesolutionofFibonacci"ssequence.Hencethesolutionforn>1isgivenbynn1æ1+5ö1æ1-5öy[n]=çç÷÷-çç÷÷5è2ø5è2øThusf[n]denotestheimpulseresponseofacausalLTIsystemdescribedbythedifferenceequationy[n]=y[n–1]+y[n–2]+x[n–1].2.48y[n]=ay[n-1]+x[n].Denoting,y[n]=yre[n]+jyim[n],anda=a+jb,weget,y[n]+jy[n]=(a+jb)(y[n-1]+jy[n-1])+x[n].reimreim22
Equatingtherealandtheimaginaryparts,andnotingthatx[n]isreal,wegety[n]=ay[n-1]-by[n-1]+x[n],(1)rereimy[n]=by[n-1]+ay[n-1]imreimTherefore1by[n-1]=y[n]-y[n-1]imaimareHenceasingleinput,twooutputdifferenceequationis2bby[n]=ay[n-1]-y[n]+y[n-1]+x[n]rereaimare22thusby[n-1]=-ay[n-1]+(a+b)y[n-2]+ax[n-1].imrereSubstitutingtheaboveinEq.(1)weget22y[n]=2ay[n-1]-(a+b)y[n-2]+x[n]-ax[n-1]rererewhichisasecond-orderdifferenceequationrepresentingy[n]intermsofx[n].re2.49FromEq.(2.59),theoutputy[n]ofthefactor-of-3interpolatorisgivenby12y[n]=xu[n]+(xu[n-1]+xu[n+2])+(xu[n-2]+xu[n+1])wherexu[n]istheoutputof33thefactor-of-3up-samplerforaninputx[n].Tocomputetheimpulseresponsewesetx[n]=d[n],inwhichcase,xu[n]=d[3n].Asaresult,theimpulseresponseisgivenby12h[n]=d[3n]+(d[3n-3]+d[3n+6])+(d[3n-6]+d[3n+3])or331212=d[n+2]+d[n+1]+d[n]+d[n-1]+d[n-2].33332.50Theoutputy[n]ofthefactor-of-Linterpolatorisgivenby12y[n]=xu[n]+(xu[n-1]+xu[n+L-1])+(xu[n-2]+xu[n+L-2])LLL-1+K+(xu[n-L+1]+xu[n+1])wherexu[n]istheoutputofthefactor-of-Lup-Lsamplerforaninputx[n].Tocomputetheimpulseresponsewesetx[n]=d[n],inwhichcase,xu[n]=d[Ln].Asaresult,theimpulseresponseisgivenby12h[n]=d[Ln]+(d[Ln-L]+d[Ln+L(L-1)])+(d[Ln-2L]+d[Ln+L(L-2)])LLL-112+K+(d[Ln-L(L–1)]+d[Ln+L])=d[n+(L-1)]+d[n+(L-2)]+KLLLL-112L-1+d[n+1]+d[n]+d[n-1]+d[n-2]+d[n-(L-1)]LLLL2.51Thefirst-ordercausalLTIsystemischaracterizedbythedifferenceequationy[n]=px[n]+px[n-1]-dy[n-1].Lettingx[n]=d[n]weobtaintheexpressionforits011impulseresponseh[n]=p0d[n]+p1d[n-1]-d1h[n-1].Solvingitforn=0,1,and2,wegeth[0]=p0,h[1]=p1-d1h[0]=p1-d1p0,andh[2]=-d1h[1]==-d1(p1-d1p0).Solvingtheseh[2]h[2]h[0]equationswegetp0=h[0],d1=-,andp1=h[1]-.h[1]h[1]23
MN2.52åpkx[n-k]=ådky[n-k].k=0k=0MNLettheinputtothesystembex[n]=d[n].Then,åpkd[n-k]=ådkh[n-k].Thus,k=0k=0Npr=ådkh[r-k].Sincethesystemisassumedtobecausal,h[r–k]=0"k>r.k=0rrpr=ådkh[r-k]=åh[k]dr-k.k=0k=02.53Theimpulseresponseofthecascadeisgivenbyh[n]=h1[n]*h2[n]whereænönnçkn-k÷h1[n]=am[n]andh2[n]=bm[n].Hence,h[n]=çåab÷m[n].èk=0ø¥¥nk2.54Now,h[n]=am[n].Thereforey[n]=åh[k]x[n-k]=åax[n-k]k=-¥k=0¥¥kk=x[n]+åax[n-k]=x[n]+aåax[n-1-k]=x[n]+ay[n-1].k=1k=0Hence,x[n]=y[n]–ay[n-1].Thustheinversesystemisgivenbyy[n]=x[n]–ax[n-1].ìüTheimpulseresponseoftheinversesystemisgivenbyg[n]=í1-aý.îþ2.55y[n]=y[n-1]+y[n-2]+x[n-1].Hence,x[n–1]=y[n]–y[n–1]–y[n–2],i.e.x[n]=y[n+1]–y[n]–y[n–1].Hencetheinversesystemischaracterisedbyìüy[n]=x[n+1]–x[n]–x[n–1]withanimpulseresponsegivenbyg[n]=í1–1–1ý.îþ1d1p12.56y[n]=px[n]+px[n-1]-dy[n-1]whichleadstox[n]=y[n]+y[n-1]-x[n-1]011ppp000Hencetheinversesystemischaracterisedbythedifferenceequation1d1p1y[n]=x[n]+x[n-1]-y[n-1].1p1p1p10002.57(a)Fromthefigureshownbelowweobservev[n]¯x[n]h1[n]h2[n]y[n]h5[n]h3[n]h4[n]24
v[n]=(h1[n]+h3[n]*h5[n])*x[n]andy[n]=h2[n]*v[n]+h3[n]*h4[n]*x[n].Thus,y[n]=(h2[n]*h1[n]+h2[n]*h3[n]*h5[n]+h3[n]*h4[n])*x[n].Hencetheimpulseresponseisgivenbyh[n]=h2[n]*h1[n]+h2[n]*h3[n]*h5[n]+h3[n]*h4[n](b)Fromthefigureshownbelowweobserveh4[n]v[n]¯x[n]h1[n]h2[n]h3[n]y[n]h5[n]v[n]=h4[n]*x[n]+h1[n]*h2[n]*x[n].Thus,y[n]=h3[n]*v[n]+h1[n]*h5[n]*x[n]=h3[n]*h4[n]*x[n]+h3[n]*h1[n]*h2[n]*x[n]+h1[n]*h5[n]*x[n]Hencetheimpulseresponseisgivenbyh[n]=h3[n]*h4[n]+h3[n]*h1[n]*h2[n]+h1[n]*h5[n]2.58h[n]=h1[n]*h2[n]+h3[n]Nowh1[n]*h2[n]=(2d[n-2]-3d[n+1])*(d[n-1]+2d[n+2])=2d[n-2]*d[n-1]–3d[n+1]*d[n-1]+2d[n-2]*2d[n+2]–3d[n+1]*2d[n+2]=2d[n-3]–3d[n]+4d[n]–6d[n+3]=2d[n-3]+d[n]–6d[n+3].Therefore,y[n]=2d[n-3]+d[n]–6d[n+3]+5d[n-5]+7d[n-3]+2d[n-1]-d[n]+3d[n+1]=5d[n-5]+9d[n-3]+2d[n-1]+3d[n+1]-6d[n+3]2.59Forafilterwithcompleximpulseresponse,thefirstpartoftheproofissameasthatfora¥filterwithrealimpulseresponse.Since,y[n]=åh[k]x[n-k],k=-¥¥¥y[n]=åh[k]x[n-k]£åh[k]x[n-k].k=-¥k=-¥¥Sincetheinputisboundedhence0£x[n]£B.Therefore,y[n]£Bh[k].xxåk=-¥¥Soifåh[k]=S<¥theny[n]£BxSindicatingthaty[n]isalsobounded.k=-¥25
Toproovetheconverseweneedtoshowthatifaboundedoutputisproducedbyaboundedh*[-n]inputthenS<¥.Considerthefollowingboundedinputdefinedbyx[n]=.h[-n]¥¥h*[k]h[k]Theny[0]=å=åh[k]=S.NowsincetheoutputisboundedthusS<¥.h[k]k=-¥k=-¥¥ThusforafilterwithcomplexresponsetooisBIBOstableifandonlyifåh[k]=S<¥.k=-¥2.60Theimpulseresponseofthecascadeisg[n]=h1[n]*h2[n]orequivalently,¥g[k]=åh1[k-r]h2[r].Thus,r=–¥¥¥¥æ¥öæ¥öåg[k]=ååh[k–r]h[r]£çåh[k]÷çåh[r]÷.12ç1÷ç2÷k=–¥k=–¥r=–¥èk=–¥øèr=–¥øSinceh1[n]andh2[n]arestable,åh1[k]<¥andåh2[k]<¥.Hence,åg[k]<¥.kkkHencethecascadeoftwostableLTIsystemsisalsostable.2.61Theimpulseresponseoftheparallelstructureg[n]=h1[n]+h2[n].Now,åg[k]=åh1[k]+h2[k]£åh1[k]+åh2[k].Sinceh1[n]andh2[n]arestable,kkkkåh1[k]<¥andåh2[k]<¥.Hence,åg[k]<¥.HencetheparallelconnectionofkkktwostableLTIsystemsisalsostable.2.62Consideracascadeoftwopassivesystems.Lety1[n]betheoutputofthefirstsystemwhichistheinputtothesecondsysteminthecascade.Lety[n]betheoveralloutputofthecascade.¥¥22Thefirstsystembeingpassivewehaveåy1[n]£åx[n].n=-¥n=-¥¥¥¥222Likewisethesecondsystembeingalsopassivewehaveåy[n]£åy1[n]£åx[n],n=-¥n=-¥n=-¥indicatingthatcascadeoftwopassivesystemsisalsoapassivesystem.Similarlyonecanprovethatcascadeoftwolosslesssystemsisalsoalosslesssystem.2.63Consideraparallelconnectionoftwopassivesystemswithaninputx[n]andoutputy[n].Theoutputsofthetwosystemsarey1[n]andy2[n],respectively.Now,¥2¥2¥2¥2ån=-¥y1[n]£ån=-¥x[n],andån=-¥y2[n]£ån=-¥x[n].Lety1[n]=y2[n]=x[n]satisfyingtheaboveinequalities.Theny[n]=y1[n]+y2[n]=2x[n]¥2¥2¥2andasaresult,ån=-¥y[n]=4ån=-¥x[n]>ån=-¥x[n].Hence,theparallelconnectionoftwopassivesystemsmaynotbepassive.26
MN2.64Letåpkx[n-k]=y[n]+ådky[n-k]bethedifferenceequationrepresentingthecausalk=0k=1IIRdigitalfilter.Foraninputx[n]=d[n],thecorrespondingoutputistheny[n]=h[n],theimpulseresponseofthefilter.AsthereareM+1{pk}coefficients,andN{dk}coefficients,thereareatotalofN+M+1unknowns.Todeterminethesecoefficientsfromtheimpulseresponsesamples,wecomputeonlythefirstN+M+1samples.Toillstratethemethod,withoutanylossofgenerality,weassumeN=M=3.Then,fromthedifferenceequationreprsentationwearriveatthefollowingN+M+1=7equations:h[0]=p,0h[1]+h[0]d=p,11h[2]+h[1]d+h[0]d=p,122h[3]+h[2]d+h[1]d+h[0]d=p,1233h[4]+h[3]d+h[2]d+h[1]d=0,123h[5]+h[4]d+h[3]d+h[2]d=0,123h[6]+h[5]d+h[4]d+h[3]d=0.123Writingthelastthreeequationsinmatrixformwearriveatéh[4]ùéh[3]h[2]h[1]ùédùé0ùê1úêh[5]ú+êh[4]h[3]h[2]úêd2ú=ê0ú,êúêúêúëh[6]ûëh[5]h[4]h[3]ûêëd3úûë0ûédùéh[3]h[2]h[1]ù–1éh[4]ùê1úandhence,êd2ú=–êh[4]h[3]h[2]úêh[5]ú.êúêúêëd3úûëh[5]h[4]h[3]ûëh[6]ûSubstitutingthesevaluesof{di}inthefirstfourequationswritteninmatrixformwegetép0ùéh[0]000ùé1ùêpúêh[1]h[0]00úêdúê1ú=êúê1ú.êp2úêh[2]h[1]h[0]0úêd2úêëp3úûëh[3]h[2]h[1]h[0]ûêëd3úûnnnn(n+1)2.65y[n]=y[-1]+ål=0x[l]=y[-1]+ål=0lm[l]=y[-1]+ål=0l=y[-1]+2.n(n+1)(a)Fory[–1]=0,y[n]=22n(n+1)n+n-4(b)Fory[–1]=–2,y[n]=–2+=.22nT2.66y(nT)=y((n–1)T)+òx(t)dt=y((n–1)T)+T×x((n–1)T).Therefore,thedifference(n-1)Tequationrepresentationisgivenbyy[n]=y[n-1]+T×x[n-1],wherey[n]=y(nT)andx[n]=x(nT).27
1n1n-111n-12.67y[n]=nål=1x[l]=nål=1x[l]+nx[n],n³1.y[n-1]=n-1ål=1x[l],n³1.Hence,n-1ål=1x[l]=(n-1)y[n-1].Thus,thedifferenceequationrepresentationisgivenbyæn-1ö1y[n]=ç÷y[n-1]+x[n].n³1.ènøn2.68y[n]+0.5y[n-1]=2m[n],n³0withy[-1]=2.Thetotalsolutionisgivenbyy[n]=yc[n]+yp[n]whereyc[n]isthecomplementarysolutionandyp[n]istheparticularsolution.nyc[n]isobtainednysolvingyc[n]+0.5yc[n-1]=0.Tothisendwesetyc[n]=l,whichnn-1yieldsl+0.5l=0whosesolutiongivesl=-0.5.Thus,thecomplementarysolutionisnoftheformyc[n]=a(-0.5).Fortheparticularsolutionwechooseyp[n]=b.Substitutingthissolutioninthedifferenceequationrepresentationofthesystemwegetb+0.5b=2m[n].Forn=0wegetb(1+0.5)=2orb=4/3.n4Thetotalsolutionisthereforegivenbyy[n]=yc[n]+yp[n]=a(-0.5)+,n³0.3-141Thereforey[–1]=a(-0.5)+=2ora=–.Hence,thetotalsolutionisgivenby331n4y[n]=–(-0.5)+,n³0.33n2.69y[n]+0.1y[n-1]-0.06y[n-2]=2m[n]withy[–1]=1andy[–2]=0.Thecomplementarysolutionyc[n]isobtainedbysolvingyc[n]+0.1yc[n-1]-0.06yc[n-2]=0.Tothisendwennn-1n-2n-22setyc[n]=l,whichyieldsl+0.1l–0.06l=l(l+0.1l–0.06)=0whosesolutiongivesl1=–0.3andl2=0.2.Thus,thecomplementarysolutionisoftheformnnyc[n]=a1(-0.3)+a2(0.2).nFortheparticularsolutionwechooseyp[n]=b(2).ubstitutingthissolutioninthedifferencenn-1n-2nequationrepresentationofthesystemwegetb2+b(0.1)2–b(0.06)2=2m[n].For-1-2n=0wegetb+b(0.1)2–b(0.06)2=1orb=200/207=0.9662.nn200nThetotalsolutionisthereforegivenbyy[n]=yc[n]+yp[n]=a1(-0.3)+a2(0.2)+2.207-1-1200-1Fromtheabovey[-1]=a1(-0.3)+a2(0.2)+2=1and207-2-2200-210107y[-2]=a1(-0.3)+a2(0.2)+2=0orequivalently,–a1+5a2=and207320710050a1+25a2=–whosesolutionyieldsa1=–0.1017anda2=0.0356.Hence,thetotal9207nnnsolutionisgivenbyy[n]=-0.1017(-0.3)+0.0356(0.2)+0.9662(2),forn³0.n2.70y[n]+0.1y[n-1]-0.06y[n-2]=x[n]-2x[n-1]withx[n]=2m[n],andy[–1]=1andy[–2]=0.Forthegiveninput,thedifferenceequationreducestonn-1y[n]+0.1y[n-1]-0.06y[n-2]=2m[n]-2(2)m[n-1]=d[n].Thesolutionofthis28
equationisthusthecomplementarysolutionwiththeconstantsdeterminedfromthegiveninitialconditionsy[–1]=1andy[–2]=0.Fromthesolutionofthepreviousproblemweobservethatthecomplementaryisofthennformyc[n]=a1(-0.3)+a2(0.2).Forthegiveninitialconditionswethushave-1-1-2-2y[-1]=a1(-0.3)+a2(0.2)=1andy[-2]=a1(-0.3)+a2(0.2)=0.Combiningtheseé-1/0.31/0.2ùéa1ùé1ùtwoequationswegetêúêú=êúwhichyieldsa1=-0.18anda2=0.08.ë1/0.091/0.04ûëa2ûë0ûnnTherefore,y[n]=-0.18(-0.3)+0.08(0.2).2.71Theimpulseresponseisgivenbythesolutionofthedifferenceequationy[n]+0.5y[n-1]=d[n].FromthesolutionofProblem2.68,thecomplementarysolutionisngivenbyyc[n]=a(-0.5).Todeterminetheconstantwenotey[0]=1asy[–1]=0.From0thecomplementarysolutiony[0]=a(–0.5)=a,hencea=1.Therefore,theimpulsenresponseisgivenbyh[n]=(-0.5).2.72Theimpulseresponseisgivenbythesolutionofthedifferenceequationy[n]+0.1y[n-1]-0.06y[n-2]=d[n].FromthesolutionofProblem2.69,thecomplementarynnsolutionisgivenbyyc[n]=a1(-0.3)+a2(0.2).Todeterminetheconstantsa1anda2,weobservey[0]=1andy[1]+0.1y[0]=0asy[–1]=y[–2]=0.Fromthecomplementary00solutiony[0]=a1(-0.3)+a2(0.2)=a1+a2=1,and11y[1]=a1(-0.3)+a2(0.2)=–0.3a1+0.2a2=-0.1.Solutionoftheseequationsyielda1=0.6nnanda2=0.4.Therefore,theimpulseresponseisgivenbyh[n]=0.6(-0.3)+0.4(0.2).KKKnAn+1n+1n+12.73LetAn=n(li).Then=li.Nowlim=1.Sinceli<1,thereexistsAnnn®¥nAn+11+li¥apositiveintegerNosuchthatforalln>No,0N-1.Itfollowsfromtheaboverxx[l]isatrinagularfunctionofl,andhenceisevenwithamaximumvalueofNatl=0æpnö2.76(a)x1[n]=cosç÷whereMisapositiveinteger.Periodofx1[n]is2M,henceèMø12M-112M-1æpnöæp(n+l)örxx[l]=2Mån=0x1[n]x1[n+l]=2Mån=0cosçèM÷øcosçèM÷ø12M-1æpnöìæpnöæplöæpnöæplöü1æplö2M-12æpnö=2Mån=0cosçèM÷øícosçèM÷øcosçèM÷ø-sinçèM÷øsinçèM÷øý=2McosçèM÷øån=0cosçèM÷ø.îþ2M-12æpnö2MFromthesolutionofProblem2.16åcosç÷==M.Therefore,n=0èMø21æpnörxx[l]=cosç÷.2èMø(b)x2[n]=nmodulo6={012345},0£n£5.Itisaperidicsequencewithaperiod156.Thus,,rxx[l]=6ån=0x2[n]x2[n+l],0£l£5.Itisalsoaperidicsequencewithaperiod6.155rxx[0]=(x2[0]x2[0]+x2[1]x2[1]+x2[2]x2[2]+x2[3]x2[3]+x2[4]x2[4]+x2[5]x2[5])=,66140rxx[1]=(x2[0]x2[1]+x2[1]x2[2]+x2[2]x2[3]+x2[3]x2[4]+x2[4]x2[5]+x2[5]x2[0])=,66132rxx[2]=(x2[0]x2[2]+x2[1]x2[3]+x2[2]x2[4]+x2[3]x2[5]+x2[4]x2[0]+x2[5]x2[1])=,66128rxx[3]=(x2[0]x2[3]+x2[1]x2[4]+x2[2]x2[5]+x2[3]x2[0]+x2[4]x2[1]+x2[5]x2[2])=,66131rxx[4]=(x2[0]x2[4]+x2[1]x2[5]+x2[2]x2[0]+x2[3]x2[1]+x2[4]x2[2]+x2[5]x2[3])=,66140rxx[5]=(x2[0]x2[5]+x2[1]x2[0]+x2[2]x2[1]+x2[3]x2[2]+x2[4]x2[3]+x2[5]x2[4])=.66n(c)x3[n]=(-1).Itisaperiodicsquencewithaperiod2.Hence,111rxx[l]=2ån=0x3[n]x3[n+l],0£l£1.rxx[0]=2(x2[0]x2[0]+x2[1]x2[1])=1,and1rxx[1]=(x2[0]x2[1]+x2[1]x2[0])=-1.Itisalsoaperiodicsquencewithaperiod2.22.77E{X+Y}=òò(x+y)pXY(x,y)dxdy=òòxpXY(x,y)dxdy+òòypXYy(x,y)dxdy=òx(òpXY(x,y)dy)dx+òy(òpXY(x,y)dx)dy=òxpX(x)dx+òypY(y)dy31
=E{X}+E{Y}.Fromtheaboveresult,E{2X}=E(X+X}=2E{X}.Similarly,E{cX}=E{(c–1)X+X}=E{(c–1)X}+E{X}.Hence,byinduction,E{cX}=cE{x}.22.78C=E{(X-k)}.TofindthevalueofkthatminimizethemeansquareerrorC,wedifferentiateCwithrespecttokandsetittozero.dCThus=E{2(X-k)}=E{2X}-E{2K}=2E{X}-2K=0.whichresultsink=E{X},anddk2theminimumvalueofCiss.x¥2.79mean=m=E{x}=xp(x)dxxò-¥X¥222variance=s=E{(x-m)}=(x-m)p(x)dxxxòxX-¥aæ1öa¥xdxx(a)pX(x)=pç22÷.Now,mx=pò22.Since22isoddhencetheèx+aø-¥x+ax+aintegraliszero.Thusm=0.x¥22axdxs=.Sincethisintegraldoesnotconvergehencevarianceisnotdefinedforxpò-¥x2+a2thisdensityfunction.a-axa¥-ax(b)p(x)=e.Now,m=xedx=0.Next,x2x2ò-¥¥¥ì2-ax¥¥ü2a2-ax2-axïxe2x-axïs=xedx=axedx=aí+edxýx2ò-¥ò0ï-aòaïî00þì-ax¥üï2xe¥2-axï2=aí0++edxý=.ïa-aò0a2ïa2î0þnænöln-l(c)px(x)=åçèl÷øp(1-p)d(x-l).Now,l=0nn¥ænöln-lænöln-lmx=òxåçèl÷øp(1-p)d(x-l)dx=åçèl÷øp(1-p)=np-¥l=0l=0n222¥2ænöln-l2sx=E{x}-mx=òxåçèl÷øp(1-p)d(x-l)dx-(np)-¥l=0n2ænöln-l22=ålçèl÷øp(1-p)-np=np(1-p).l=0¥-alea(d)px(x)=åd(x-l).Now,l!l=032
¥¥e-aal¥e-aalmx=òxål!d(x-l)dx=åll!=a.-¥l=0l=0¥¥-al¥-al222a2ea22ea2sx=E{x}-mx=2òxål!d(x-l)dx-a=ål-a=a.-¥l!l=0l=0x-x2/2a2(e)p(x)=em(x).Now,x2a1¥2-x2/2a21¥2-x2/2a2m=xem(x)dx=xedx=ap/2.xa2ò-¥a2ò01¥22a2pæpö2223-x/2a2sx=E{x}-mx=2òxem(x)dx-=çè2-÷øa.a-¥222.80Recallthatrandomvariablesxandyarelinearlyindependentifandonlyifì2üEía1x+a2yý>0"a1,a2exceptwhena1=a2=0,Now,îþì22üì22ü2222Eíîa1xýþ+Eíîa2yýþ+E{(a1)*a2xy*}+E{a1(a2)*x*y}=a1E{x}+a2E{y}>0"aandaexceptwhena1=a2=0.12Henceifx,yarestatisticallyindependenttheyarealsolinearlyindependent.2222222.81sx=E{(x-mx)}=E{x+mx-2xmx}=E{x}+E{mx}-2E{xmx}.222Sincemisaconstant,henceE{m}=mandE{xm}=mE{x}=m.Thus,xxxxxx222222s=E{x}+m-2m=E{x}-m.xxxx2.82V=aX+bY.Therefore,E{V}=aE{X}+bE{Y}=am+bm.andxy222s=E{(V-m)}=E{(a(X-m)+b(Y-m))}.vvxy22222SinceX,Yarestatisticallyindependenthences=as+bs.vxy2.83v[n]=ax[n]+by[n].Thusfvv[n]=E{v[m+n}v[m]}22=E{ax[m+n}x[m]+by[m+n}y[m]+abx[m+n]y[m]+abx[m]y[m+n]}.Sincex[n]andy[n]areindependent,2222fvv[n]=E{ax[m+n}x[m]}+E{by[m+n}y[m]}=afxx[n]+bfyy[n].fvx[n]=E{v[m+n]x[m]}=E{ax[m+n]x[m]+by[m+n]x[m]}=aE{x[m+n]x[m]}=afxx[n].Likewise,fvy[n]=bfyy[n].2.84fxy[l]=E{x[n+l]y*[n]},fxy[–l]=E{x[n–l]y*[n]},fyx[l]=E{y[n+l]x*[n]}.Therefore,fyx*[l]=E{y*[n+l]x[n]}=E{x[n–l]y*[n]}=fxy[–l].33
Hencefxy[-l]=fyx*[ll].Sincegxy[l]=fxy[l]-mx(my)*.Thus,gxy[l]=fxy[l]-mx(my)*.Hence,gxy[l]=fxy[l]-mx(my)*.Asaresult,gxy*[l]=fxy*[l]-(mx)*my.Hence,gxy[-l]=gyx*[l].Theremainingtwopropertiescanbeprovedinasimilarwaybylettingx=y.22.85E{x[n]-x[n-l]}³0.22E{x[n]}+E{x[n-l]}-E{x[n]x*[n-l]}-E{x*[n]x[n-l]}³02fxx[0]-2fxx[l]³0fxx[0]³fxx[l]2222UsingCauchy"sinequalityE{x}E{y}£E{xy}.Hence,fxx[0]fyy[0]£fxy[l].2Onecanshowsimilarlygxx[0]gyy[0]£gxy[l].2.86Sincetherearenoperiodicitiesin{x[n]}hencex[n],x[n+l]becomeuncorrelatedasl®¥..22Thuslimg[l]=limf[l]-m®0.Hencelimf[l]=m.xxxxxxxxl®¥l®¥l®¥24249+11l+14l29+11l+14l2.87fXX[l]=24.Now,mX[n]=limfXX[l]=lim24=7.1+3l+2ll®¥l®¥1+3l+2l222Hence,mX[n]=m7.E(X[n])=fXX[0]=9.Therefore,sX=fXX[0]-mX=9-7=2.M2.1L=input("Desiredlength=");n=1:L;FT=input("Samplingfrequency=");T=1/FT;imp=[1zeros(1,L-1)];step=ones(1,L);ramp=(n-1).*step;subplot(3,1,1);stem(n-1,imp);xlabel(["Timein",num2str(T),"sec"]);ylabel("Amplitude");subplot(3,1,2);stem(n-1,step);xlabel(["Timein",num2str(T),"sec"]);ylabel("Amplitude");subplot(3,1,3);stem(n-1,ramp);xlabel(["Timein",num2str(T),"sec"]);ylabel("Amplitude");M2.2%GetuserinputsA=input("Thepeakvalue=");L=input("Lengthofsequence=");N=input("Theperiodofsequence=");FT=input("Thedesiredsamplingfrequency=");DC=input("Thesquarewavedutycycle=");%CreatesignalsT=1/FT;34
t=0:L-1;x=A*sawtooth(2*pi*t/N);y=A*square(2*pi*(t/N),DC);%Plotsubplot(211)stem(t,x);ylabel("Amplitude");xlabel(["Timein",num2str(T),"sec"]);subplot(212)stem(t,y);ylabel("Amplitude");xlabel(["Timein",num2str(T),"sec"]);1050-5-10020406080100Timein5e-05sec1050-5-10020406080100Timein5e-05secM2.3(a)TheinputdataenteredduringtheexecutionofProgram2_1areTypeinrealexponent=-1/12Typeinimaginaryexponent=pi/6Typeinthegainconstant=1Typeinlengthofsequence=4135
RealpartImaginarypart110.50.500-0.5-0.5-1-1010203040010203040TimeindexnTimeindexn(b)TheinputdataenteredduringtheexecutionofProgram2_1areTypeinrealexponent=-0.4Typeinimaginaryexponent=pi/5Typeinthegainconstant=2.5Typeinlengthofsequence=101RealpartImaginarypart1.51.5110.50.500-0.5-0.5010203040010203040TimeindexnTimeindexnM2.4(a)L=input("Desiredlength=");A=input("Amplitude=");omega=input("Angularfrequency=");phi=input("Phase=");n=0:L-1;x=A*cos(omega*n+phi);stem(n,x);xlabel("Timeindex");ylabel("Amplitude");title(["omega_{o}=",num2str(omega)]);(b)36
w=0.14po210-1-20102030405060708090100Timeindexnw=0.24po210-1-20510152025303540Timeindexnw=0.68po210-1-20102030405060708090100Timeindexn37
w=0.75po210-1-20510152025303540Timeindexn-j0.4pnM2.5(a)UsingProgram2_1wegeneratethesequencex˜1[n]=eshownbelowRealpart10.50-0.5-10510152025303540TimeindexnImaginarypart10.50-0.5-10510152025303540Timeindexn(b)Codefragmentusedtogeneratex˜2[n]=sin(0.6pn+0.6p)is:x=sin(0.6*pi*n+0.6*pi);38
210-1-20510152025303540Timeindexn(c)Codefragmentusedtogeneratex˜3[n]=2cos(1.1pn-0.5p)+2sin(0.7pn)isx=2*cos(1.1*pi*n-0.5*pi)+2*sin(0.7*pi*n);420-2-40510152025303540Timeindexn(d)Codefragmentusedtogeneratex˜4[n]=3sin(1.3pn)-4cos(0.3pn+0.45p)is:x=3*sin(1.3*pi*n)-4*cos(0.3*pi*n+0.45*pi);6420-2-4-60510152025303540Timeindexn(e)Codefragmentusedtogeneratex˜5[n]=5sin(1.2pn+0.65p)+4sin(0.8pn)-cos(0.8pn)is:x=5*sin(1.2*pi*n+0.65*pi)+4*sin(0.8*pi*n)-cos(0.8*pi*n);39
1050-5-100510152025303540Timeindexn(f)Codefragmentusedtogeneratex˜6[n]=nmodulo6is:x=rem(n,6);6543210-10510152025303540TimeindexnM2.6t=0:0.001:1;fo=input("FrequencyofsinusoidinHz=");FT=input("SampligfrequencyinHz=");g1=cos(2*pi*fo*t);plot(t,g1,"-")xlabel("time");ylabel("Amplitude")holdn=0:1:FT;gs=cos(2*pi*fo*n/FT);plot(n/FT,gs,"o");holdoffM2.7t=0:0.001:0.85;g1=cos(6*pi*t);g2=cos(14*pi*t);g3=cos(26*pi*t);plot(t/0.85,g1,"-",t/0.85,g2,"--",t/0.85,g3,":")xlabel("time");ylabel("Amplitude")holdn=0:1:8;gs=cos(0.6*pi*n);plot(n/8.5,gs,"o");holdoffM2.8Asthelengthofthemovingaveragefilterisincreased,theoutputofthefiltergetsmoresmoother.However,thedelaybetweentheinputandtheoutputsequencesalsoincreases(ThiscanbeseenfromtheplotsgeneratedbyProgram2_4forvariousvaluesofthefilterlength.)40
M2.9alpha=input("Alpha=");yo=1;y1=0.5*(yo+(alpha/yo));whileabs(y1-yo)>0.00001y2=0.5*(y1+(alpha/y1));yo=y1;y1=y2;enddisp("Squarerootofalphais");disp(y1)M2.10alpha=input("Alpha=");yo=0.3;y=zeros(1,61);L=length(y)-1;y(1)=alpha-yo*yo+yo;n=2;whileabs(y(n-1)-yo)>0.00001y2=alpha-y(n-1)*y(n-1)+y(n-1);yo=y(n-1);y(n)=y2;n=n+1;enddisp("Squarerootofalphais");disp(y(n-1))m=0:n-2;err=y(1:n-1)-sqrt(alpha);stem(m,err);axis([0n-2min(err)max(err)]);xlabel("Timeindexn");ylabel("Error");title(["alpha=",num2str(alpha)])ThedisplayedoutputisSquarerootofalphais0.84000349056114a=0.70560.060.040.020-0.02-0.040510152025TimeindexnM2.11N=input("Desiredimpulseresponselength=");p=input("Typeinthevectorp=");d=input("Typeinthevectord=");[h,t]=impz(p,d,N);n=0:N-1;stem(n,h);xlabel("Timeindexn");ylabel("Amplitude");41
1.510.50-0.5-1010203040TimeindexnM2.12x=[3-201452]y=[071-349-2],w=[-5436-501].(a)rxx[n]=[6112-31128592811-32116],ryy[n]=[0-146143-521016010-524361-140],rww[n]=[–5428–44–11–20112–20–11–44284–5].6015050r[n]ryy[n]40xx1003050200100-50-6-4-20246-6-4-20246LagindexLagindex100r[n]ww500-50-6-4-20246Lagindex(b)rxy[n]=[–631–6–191049402742737140].42
rxw[n]=[3–2–152916–351236386–17–10].50100r[n]r[n]xwxy5000-50-50-6-4-20246-6-4-20246LagindexLagindexM2.13N=input("Lengthofsequence=");n=0:N-1;x=exp(-0.8*n);y=randn(1,N)+x;n1=length(x)-1;r=conv(y,fliplr(y));k=(-n1):n1;stem(k,r);xlabel("Lagindex");ylabel("Amplitude");gtext("r_{yy}[n]");3020r[n]yy100-10-30-20-100102030LagindexM2.14(a)n=0:10000;phi=2*pi*rand(1,length(n));A=4*rand(1,length(n));x=A.*cos(0.06*pi*n+phi);stem(n(1:100),x(1:100));%axis([050-44]);xlabel("Timeindexn");ylabel("Amplitude");mean=sum(x)/length(x)var=sum((x-mean).^2)/length(x)43
442200-2-2-4-4020406080100020406080100TimeindexnTimeindexn442200-2-2-4-4020406080100020406080100TimeindexnTimeindexnmean=0.00491782302432var=2.68081196077671FromExample2.44,wenotethatthemean=0andvariance=8/3=2.66667.M2.15n=0:1000;z=2*rand(1,length(n));y=ones(1,length(n));x=z-y;mean=mean(x)var=sum((x-mean).^2)/length(x)mean=0.00102235365812var=0.3421053083095921-12(1+1)1UsingEqs.(2.129)and(2.130)wegetmX==0,andsX==.Itshould2223benotedthatthevaluesofthemeanandthevariancecomputedusingtheaboveMATLABprogramgetclosertothetheoreticalvaluesifthelengthisincreased.Forexample,foralength100001,thevaluesaremean=9.122420593670135e-0444
var=0.3348688851681945
Chapter3(2e)¥jw-jwn3.1X(e)=åx[n]ewherex[n]isarealsequence.Therefore,n=-¥æ¥ö¥¥jw-jwn-jwnXre(e)=Reçåx[n]e÷=åx[n]Re(e)=åx[n]cos(wn),andèn=-¥øn=-¥n=-¥æ¥ö¥¥jw-jwn-jwnXim(e)=Imçåx[n]e÷=åx[n]Im(e)=-åx[n]sin(wn).Sincecos(wn)andèn=-¥øn=-¥n=-¥jwsin(wn)are,respectively,evenandoddfunctionsofw,Xre(e)isanevenfunctionofw,jwandXim(e)isanoddfunctionofw.jw2jw2jw2jwX(e)=Xre(e)+Xim(e).Now,Xre(e)isthesquareofanevenfunctionand2jwXim(e)isthesquareofanoddfunction,theyarebothevenfunctionsofw.Hence,jwX(e)isanevenfunctionofw.æjw)öjw-1Xim(earg{X(e)}=tançjw÷.TheargumentisthequotientofanoddfunctionandanevenèXre(e)øjwfunction,andisthereforeanoddfunction.Hence,arg{X(e)}isanoddfunctionofw.–w–wjw111-ae1-ae1-acosw-jasinw3.2X(e)==×==–jw–jw–w221-ae1-ae1-ae1-2acosw+a1-2acosw+ajw1-acoswjwasinwTherefore,Xre(e)=2andXim(e)=–2.1-2acosw+a1-2acosw+ajw2jwjw111X(e)=X(e)×X*(e)=×=.–jwjw21-ae1-ae1-2acosw+ajw1Therefore,X(e)=.21-2acosw+ajwXim(e)asinw-1æasinwötanq(w)=jw=–.Therefore,q(w)==tanç–÷.Xre(e)1-acoswè1-acoswø11113.3(a)y[n]=m[n]=y[n]+y[n],wherey[n]=(y[n]+y[-n])=(m[n]+m[-n])=+d[n],evodev22221111andy[n]=(y[n]-y[-n])=(m[n]-m[-n])=m[n]-–d[n].od2222é¥ù¥jw1êú11Now,Yev(e)=2ê2påd(w+2pk)ú+2=påd(w+2pk)+2.ëk=–¥ûk=–¥1111Sincey[n]=m[n]-+d[n],y[n]=m[n-1]-+d[n-1].Asaresult,od22od2245
1111y[n]-y[n-1]=m[n]-m[n-1]+d[n-1]-d[n]=d[n]+d[n-1].TakingtheDTFTofodod2222jw-jwjw1–jwbothsideswethengetYod(e)-eYod(e)=2(1+e).or-jwjw11+e11Y(e)==-.Hence,od21-e-jw1-e-jw2¥jwjwjw1Y(e)=Yev(e)+Yod(e)=-jw+påd(w+2pk).1-ek=-¥¥jw(b)Letx[n]bethesequencewiththeDTFTX(e)=å2pd(w-wo+2pk).Itsinversek=-¥p1jwnjwnDTFTisthengivenbyx[n]=2pd(w-w)edw=eo.2pòo-pjw¥3.4LetX(e)=åk=-¥2pd(w+2pk).ItsinverseDTFTisthengivenby1pjwn2px[n]=ò2pd(w)edw==1.2p–p2p¥¥jw-jwn-jwn3.5(a)Lety[n]=g[n–no],ThenY(e)=åy[n]e=åg[n-no]en=-¥n=-¥¥-jwn-jwn-jwnjw=eoåg[n]e=eoG(e).n=-¥¥¥jwnjw-jwnjwn-jwn(b)Leth[n]=eog[n],thenH(e)=åh[n]e=åeog[n]en=-¥n=-¥¥-j(w-w)nj(w-w)=åg[n]eo=G(eo).n=-¥¥d(G(ejw))¥jw-jwn-jwn(c)G(e)=åg[n]e.Hence=å-jng[n]e.dwn=-¥n=-¥d(G(ejw))¥d(G(ejw))-jwnTherefore,j=ång[n]e.ThustheDTFTofng[n]isj.dwdwn=-¥¥¥¥jw-jwn(d)y[n]=g[n]*h[n]=åg[k]h[n-k].HenceY(e)=ååg[k]h[n-k]ek=-¥n=-¥k=-¥¥¥jw-jwkjw-jwkjwjw=åg[k]H(e)e=H(e)åg[k]e=H(e)G(e).k=-¥k=-¥¥jw-jwn(e)y[n]=g[n]h[n].HenceY(e)=åg[n]h[n]en=-¥46
p1jqjqnSinceg[n]=òG(e)edqwecanrewritetheaboveDTFTas2p-p¥pp¥jw1-jwnjqjqn1jq-j(w-q)nY(e)=2påòh[n]eG(e)edq=2pòG(e)åh[n]edqn=-¥-p-pn=-¥p1jqj(w-q)=òG(e)H(e)dq.2p-p¥¥æpöç1jw-jwn÷(f)y[n]=åg[n]h*[n]=åg[n]çòH*(e)edw÷ç2p÷n=-¥n=-¥è-pøpæ¥öp1jwç-jwn÷1jwjw=2pòH*(e)çåg[n]e÷dw=2pòH*(e)G(e)dw.-pèn=-¥ø-p¥jw-jwn3.6DTFT{x[n]}=X(e)=åx[n]e.n=-¥¥¥-jwnjwm-jw(a)DTFT{x[–n]}=åx[-n]e=åx[m]e=X(e).n=-¥m=-¥¥æ¥ö-jwnjwn(b)DTFT{x*[-n]}=åx*[-n]e=çåx[-n]e÷usingtheresultofPart(a).n=-¥èn=-¥øjwThereforeDTFT{x*[-n]}=X*(e).ìx[n]+x*[n]ü1jw-jw(c)DTFT{Re(x[n])}=DTFTíý={X(e)+X*(e)}usingtheresultofPartî2þ2(b).ìx[n]-x*[n]ü1jw-jw(d)DTFT{jIm(x[n])}=DTFTíjý={X(e)-X*(e)}.î2jþ2ìx[n]+x*[-n]ü1jwjwjwjw(e)DTFT{xcs[n]}=DTFTíý={X(e)+X*(e)}=Re{X(e)}=Xre(e).î2þ2ìx[n]-x*[-n]ü1jwjwjw(f)DTFT{xca[n]}=DTFTíý={X(e)-X*(e)}=jXim(e).î2þ2¥jw-jwn3.7X(e)=åx[n]ewherex[n]isarealsequence.Forarealsequence,n=-¥jw–jw–jwX(e)=X*(e),andIDFT{X*(e),}=x*[-n].47
jw1jw–jwjw(a)Xre(e)={X(e)+X*(e)}.Therefore,IDFT{Xre(e)}21jw–jw11=IDFT{X(e)+X*(e)}={x[n]+x*[-n]}={x[n]+x[-n]}=xev[n].222jw1jw–jwjw(b)jXim(e)={X(e)-X*(e)}.Therefore,IDFT{jXim(e)}21jw–jw11=IDFT{X(e)-X*(e)}={x[n]-x*[-n]}={x[n]-x[-n]}=xod[n].222¥¥jw-jwnjwjwn–jw3.8(a)X(e)=åx[n]e.Therefore,X*(e)=åx[n]e=X(e),andhence,n=-¥n=-¥¥–jw–jwnjwX*(e)=åx[n]e=X(e).n=-¥jw–jwjw–jw(b)FromPart(a),X(e)=X*(e).Therefore,Xre(e)=Xre(e).jw–jwjw–jw(c)FromPart(a),X(e)=X*(e).Therefore,Xim(e)=-Xim(e).jw2jw2jw2–jw2–jw-jw(d)X(e)=X(e)+X(e)=X(e)+X(e)=X(e).reimreimX(ejw)X(e-jw)jw-1im-1imjw(e)argX(e)=tan=-tan=-argX(e)X(ejw)X(e-jw)rerepp1jwjwn1jw–jwn3.9x[n]=òX(e)edw.Hence,x*[n]=òX*(e)edw.2p2p-p-pjwjw(a)Sincex[n]isrealandeven,henceX(e)=X*(e).Thus,p1jw-jwnx[–n]=òX(e)edw,2p-pp11jwTherefore,x[n]=(x[n]+x[-n])=òX(e)cos(wn)dw.22p-pjw–jwNowx[n]beingeven,X(e)=X(e).Asaresult,theterminsidetheaboveintegraliseven,p1jwandhencex[n]=òX(e)cos(wn)dwp0(b)Sincex[n]isoddhencex[n]=–x[–n].p1jjwThusx[n]=(x[n]-x[-n])=òX(e)sin(wn)dw.Again,sincex[n]=–x[–n],22p-p48
pjw–jwjjwX(e)=-X(e).Theterminsidetheintegraliseven,hencex[n]=òX(e)sin(wn)dwp0æjwnjf-jwn-jfönne0e+e0e3.10x[n]=acos(wn+f)m[n]=Aaç÷m[n]oçè2÷øAjfjwnA-jf-jw=e(aeo)m[n]+e(aeo)m[n].Therefore,22jwAjf1A-jf1X(e)=e+e.21-ae-jwejwo21-ae-jwe-jwonjw13.11Letx[n]=am[n],a<1.FromTable3.1,DTFT{x[n]}=X(e)=.–jw1-ae¥¥¥jwn-jwnn-jwn-1jwn-jwn(a)X1(e)=åam[n+1]e=åae=ae+åaen=-¥n=-1n=011æejw-aö-1jw=ae+–jw=aç–jw÷.1-aeè1-aeøn(b)x2[n]=nam[n].Notethatx2[n]=nx[n].Hence,usingthedifferentiation-in-frequencyjw-jwjwdX(e)aepropertyinTable3.2,wegetX2(e)=j=-jw2.dw(1-ae)ìnM-1ïa,n£M,jwn-jwn-n-jwn(c)x3[n]=íThen,X3(e)=åae+åaeîï0,otherwise.n=0n=-MM+1-jw(M+1)-M-jwM1-aeMjwM1-ae=+ae.1-ae-jw1-a-1e-jw¥¥jwn–jwnn–jwn-jw2-j2w(d)X4(e)=åae=åae-1-ae-aen=3n=01-jw2-j2w=-1-ae-ae.-jw1-ae¥¥jwn–jwnn–jwn-2j2w-1jw(e)X5(e)=ånae=ånae-2ae-aen=-2n=0-jwae-2j2w-1jw=-2ae-ae.-jw2(1-ae)-1¥¥1ejwjwn–jwn-mjwm-mjwm(f)X6(e)=åae=åae=åae-1=-1jw-1=jw.1-aea-en=-¥m=1m=049
ì1,–N£n£N,N1ï-jw(2N+1))sin(w[N+2])jw-jwnjwN(1-e3.12(a)y1[n]=íThenY1(e)=åe=e-jw=îï0,otherwise.n=-N(1-e)sin(w/2)ìnï1-,-N£n£N,(b)y[n]=íNNowy2[n]=y0[n]*y0[n]where2ïî0,otherwise.2æéN+1ùöìï1,-N/2£n£N/2,sinçwêëúû÷jw2jwè2øy[n]=íThusY(e)=Y(e)=.00,otherwise.20sin2(w/2)îïìïcos(pn/2N),-N£n£N,(c)y[n]=íThen,3îï0,otherwise.NNjw1-j(pn/2N)-jwn1j(pn/2N)-jwnY3(e)=åee+åee22n=-Nn=-NNN1-jæw-pön1–jæw+pön=åeè2Nø+åeè2Nø22n=-Nn=-Np1p11sin((w-2N)(N+2))1sin((w+2N)(N+2))=+.2p2psin((w-2N)/2)sin((w+2N)/2)(n+m-1)!n3.13Denotexm[n]=am[n],a<1.Weshallprovebyinductionthatn!(m-1)!jw1DTFT{xm[n]}=Xm(e)=–jwm.FromTable3.1,itfollowsthatitholdsform=1.(1-ae)(n+1)!nLetm=2.Thenx2[n]=am[n]=(n+1)x1[n]=nx1[n]+x1[n].Therefore,n!(–jwjwae11X2(e)=–jw2+–jw=–jw2usingthedifferentiation-in-frequency(1-ae)1-ae(1-ae)propertyofTable3.2.(n+m)!nNowasuume,theitholdsform.Considernextxm+1[n]=am[n]n!(m)!æn+mö(n+m-1)!næn+mö1=ç÷am[n],=ç÷xm[n]=×n×xm[n]+xm[n].Hence,èmøn!(m-1)!èmøm1dìï1üï1ae–jw1jwXm+1(e)=mjdwíï–jwmýï+–jwm=–jwm+1+–jwmî(1-ae)þ(1-ae)(1-ae)(1-ae)1=.–jwm+1(1-ae)50
¥pjw1jwn3.14(a)Xa(e)=åd(w+2pk).Hence,x[n]=òd(w)edw=1.2pk=-¥-p1-ejw(N+1)Nì1,0£n£N,jw-jwn(b)Xb(e)=-jw=åe.Hence,x[n]=í0,otherwise.1-eîïn=0NNì1,-N£n£N,jw-jwl(c)Xc(e)=1+2åcos(wl)=åe.Hencex[n]=í0,otherwise.îïl=0l=-N-jwjw-jaejw(d)X(e)=,a<1.NowwecanrewriteX(e)asd(1-ae-jw)2djwd1djwjw1X(e)==(X(e))whereX(e)=.ddw(1-ae-jw)dwoo1-ae-jwnnNowx[n]=am[n].Hence,fromTable3.2,x[n]=-jnam[n].odæejw+e–jwöæej2w+e–j2wö33jwjw–jwj2w–j2w3.15(a)H1(e)=1+2ç÷+3ç÷=1+e+e+e+e.è2øè2ø22jwHence,theinverseofH1(e)isalength-5sequencegivenbyh1[n]=[1.51111.5],-2£n£2.éæejw+e–jwöæej2w+e–j2wöùæejw/2+e–jw/2ö(b)Hjw)=ê3+2ú×–jw/22(eç÷+4ç÷ç÷×eêëè2øè2øúûè2ø1j2wjw–jw–j2w–j3wjw=(2e+3e+4+4e+3e+2e).Hence,theinverseofH2(e)isalength-62sequencegivenbyh2[n]=[11.5221.51],-2£n£3.éæejw+e–jwöæej2w+e–j2wöùæejw/2-e–jw/2ö(c)Hjw)=jê3+4ú×3(eç÷+2ç÷ç÷êëè2øè2øúûè2jø1j3wj2wjw–jw–j2w–j3wjw=(e+2e+2e+0-2e-2e-e).Hence,theinverseofH3(e)isa2length-7sequencegivenbyh3[n]=[0.5110–1–1-0.5],-3£n£3.éæejw+e–jwöæej2w+e–j2wöùæejw/2-e–jw/2ö(d)Hjw)=jê4+2ú×jw/24(eç÷+3ç÷ç÷×eêëè2øè2øúûè2jø1æ3j3w1j2wjw1–jw3–j2wöjw=çe-e+3e-3+e-e÷.Hence,theinverseofH4(e)isalength-62è2222øsequencegivenbyh4[n]=[0.75-0.251.5-1.50.25-0.75],-3£n£2.jw33j2wjw5–jw3–j2w3.16(a)H2(e)=1+2cosw+(1+cos2w)=e+e++e+e.242451
jwHence,theinverseofH1(e)isalength-5sequencegivenbyh1[n]=[0.7512.510.75],-2£n£2.jwé4ùjw/2(b)H2(e)=ê1+3cosw+(1+cos2w)úcos(w/2)eë2û1j3w5j2w11jw115–jw1–j2wjw=e+e+e++e+e.Hence,theinverseofH2(e)isalength-6244442sequencegivenbyh2[n]=[0.51.252.752.751.250.5],-3£n£2.jw(c)H3(e)=j[3+4cosw+(1+cos2w)]sin(w)1j3wj2w7jw7–jw–j2w1–j3wjw=e+e+e+0-e-e-e.Hence,theinverseofH3(e)isa4444length-7sequencegivenbyh3[n]=[0.2511.750-1.75-1-0.25],-3£n£3.jwé3ù–jw/2(d)H4(e)=jê4+2cosw+(1+cos2w)úsin(w/2)eë2û1æ3j2w1jw99–jw1–j2w3–j3wöjw=çe+e+-e+e-e÷.Hence,theinverseofH4(e)isa2è442244øé319913ùlength-6sequencegivenbyh4[n]=ê---ú,-2£n£3.ë884488û¥jwj3wjw3jw-jwn3.17Y(e)=X(e)=X((e)).Now,X(e)=åx[n]e.Hence,n=-¥¥¥¥jw-jwnjw3-jwn3-jwmY(e)=ån=-¥y[n]e=X((e))=ån=-¥x[n](e)=åm=-¥x[m/3]e.x[n],n=0,±3,±6,KTherefore,y[n]={0,otherwise.¥jw-jwn3.18X(e)=åx[n]e.n=-¥¥¥jw/2-j(w/2)njw/2n-j(w/2)nX(e)=åx[n]e,andX(-e)=åx[n](-1)e.Thus,n=-¥n=-¥¥¥jw-wn1jw/2jw/21n-j(w/2)nY(e)=åy[n]e={X(e)+X(-e)}=å(x[n]+x[n](-1))e.Thus,22n=-¥n=-¥1nx[n],forneveny[n]=2(x[n]+x[n](-1))={0.fornodd.3.19FromTable3.3,weobservethatevensequenceshavereal-valuedDTFTsandoddsequenceshaveimaginary-valuedDTFTs.(a)Since-n=n,,x[n]isanevensequencewithareal-valuedDTFT.133(b)Since(-n)=-n,x[n]isanoddsequencewithanimaginary-valuedDTFT.252
(c)Sincesin(-wn)=-sin(wn)andw(-n)=-wn,,x[n]isanevensequencewithareal-cccc3valuedDTFT.(d)Sincex[n]isanoddsequenceithasanimaginary-valuedDTFT.4(e)Sincex[n]isanoddsequenceithasanimaginary-valuedDTFT.5jw3.20(a)SinceY(e)isareal-valuedfunctionofw,itsinverseisanevensequence.1jw(b)SinceY(e)isanimaginary-valuedfunctionofw,itsinverseisanoddsequence.2jw(c)SinceY(e)isanimaginary-valuedfunctionofw,itsinverseisanoddsequence.3jw3.21(a)H(e)isareal-valuedfunctionofw.Hence,itsinverseisanevensequence.LLPjw(b)H(e)isareal-valuedfunctionofw.Hence,itsinverseisanevensequence.BLDIFjwjw3.22Letu[n]=x[–n],andletX(e)andU(e)denotetheDTFTsofx[n]andu[n],respectively.FromtheconvolutionpropertyoftheDTFTgiveninTable3.2,theDTFTofy[n]=x[n]*u[n]jwjwjwjw-jwisgivenbyY(e)=X(e)U(e).FromTable3.3,U(e)=X(e).ButfromTable3.4,2-jwjwjwjwjwjwX(e)=X*(e).Hence,Y(e)=X(e)X*(e)=X(e)whichisreal-valuedfunctionofw.3.23Fromthefrequency-shiftingpropertyoftheDTFTgiveninTable3.2,theDTFTof-jpn/3j(w+p/3)x[n]eisgivenbyX(e).AsketchofthisDTFTisshownbelow.j(w+p/3)X(e)1w–p–2p/3–p/30p/32p/3pn3.24TheDTFTofx[n]=–am[–n–1]isgivenby-1¥¥æjwönX(ejw)=å-ane–jwn=-åa–nejwn=-a–1ejwåçe÷.çèa÷øn=–¥n=1n=0jw–1jw11jw21Fora>1,X(e)=-ae=.X(e)=.1-(ejw/a)1-ae-jw1+a2-2acoswp¥1jw22FromParseval"srelation,òX(e)dw=åx[n].2p-pn=-¥53
jw21n(a)X(e)=.Hence,a=-2.Therefoe,x[n]=-(-2)m[-n-1].5+4coswpp¥-1jw2jw22n2Now,4òX(e)dw=2òX(e)dw=4påx[n]=4på(-2)0-pn=-¥n=-¥¥n¥næ1öæ1ö4p=4påç÷=påç÷=.è4øè4ø3n=1n=0jw21n(b)X(e)=.Hence,a=1.5andtherefore,x[n]=-(1.5)m[-n-1].Now,3.25-3coswpp¥-1¥njw21jw22n2æ4öòX(e)dw=2òX(e)dw=påx[n]=på(1.5)=påçè9÷ø0-pn=-¥n=-¥n=1¥n4pæ4ö4p94p=åç÷=×=.9è9ø955n=0(c)Usingthedifferentiation-in-frequencypropertyoftheDTFT,theinverseDTFTofdæ1öae-jwjwnX(e)=jç÷=isx[n]=-nam[-n-1].Hence,theinverseDTFTofdwçè1-ae-jw÷ø(1-ae-jw)21nis-(n+1)am[-n-1].(1-ae-jw)2jw21nY(e)=.Hence,a=2andy[n]=-(n+1)2m[-n-1].Now,(5-4cosw)2pp¥-1jw2jw2222n4òX(e)dw=2òX(e)dw=4påx[n]=4på(n+1)×20-pn=-¥n=-¥¥næ1ö29/4=påç÷×n=p=4p..è4ø9/16n=0¥j03.25(a)X(e)=åx[n]=3+1-2-3+4+1-1=3.n=-¥¥jpjpn(b)X(e)=åx[n]e=-3-1-2+3-4-1+1=-7.n=-¥pjw(c)òX(e)dw=2px[0]=-4p.-p¥p2jw2(d)òX(e)dw=2påx[n]=82p.(UsingParseval"srelation)-pn=-¥54
pjw2¥dX(e)2(e)òdw=2pån×x[n]=378p.(UsingParseval"srelationwithdifferentiation-in--pdwn=-¥frequencyproperty)¥j03.26(a)X(e)=åx[n]=-2+4-1+5-3-2+4+3=8.n=-¥¥jpn(b)X(e)=åx[n](-1)=2+4+1+5+3-2-4+3=14.n=-¥pjw(c)òX(e)dw=2px[0]=-4p.-p¥p2jw2(d)òX(e)dw=2påx[n]=168p.(UsingParseval"srelation)-pn=-¥pjw2¥dX(e)2(e)òdw=2pån×x[n]=1238p.-pdwn=-¥jw3.27LetG(e)denotetheDTFTofg[n].11(b)g[n]=g[n]+g[n-4].Hence,theDTFTofg[n]isgivenby2112jwjw-j4wjw-j4wjwG(e)=G(e)+eG(e)=(1+e)G(e).2111-jw(c)g[n]=g[-(n-3)]+g[n-4].Now,theDTFTofg[-n]isgivenbyG(e).31111jw-j3w-jw-j4wjwHence,theDTFTofg[n]isgivenbyG(e)=eG(e)+eG(e).3311(d)g[n]=g[n]+g[-(n-7)].Hence,theDTFTofg[n]isgivenby4114jwjw-j7w-jwG(e)=G(e)+eG(e).411jwjwjwjw3.28Y(e)=X(e)×X(e)×X(e),i.e.,123¥æ¥öæ¥öæ¥öåy[n]e-jwn=çåx[n]e-jwn÷çåx[n]e-jwn÷çåx[n]e-jwn÷ç1÷ç2÷ç3÷n=-¥èn=-¥øèn=-¥øèn=-¥ø¥æ¥öæ¥öæ¥ö(a)Therefore,settingw=0wegetåy[n]=çåx[n]÷çåx[n]÷çåx[n]÷.ç1÷ç2÷ç3÷n=-¥èn=-¥øèn=-¥øèn=-¥ø¥æ¥öæ¥öæ¥ö(b)Settingw=pwegetå(-1)ny[n]=çå(-1)nx[n]÷çå(-1)nx[n]÷çå(-1)nx[n]÷.ç1÷ç2÷ç3÷n=-¥èn=-¥øèn=-¥øèn=-¥ø55
3.29(a)x[n]=xev[n]+xod[n].Now,foracausalx[n],fromtheresultsofProblem2.4(??),weobservex[n]=2x[n]m[n]-x[0]d[n]=h[n]-x[0]d[n],(1)evx[n]=2x[n]m[n]+x[0]d[n].(2)odTakingtheDTFTofbothsidesofEq.(2)wegetjwjwX(e)=H(e)-x[0],(3)pjw1jqj(w-q)whereH(e)=DTFT{2x[n]m[n]}=X(e)m(e)dq,(4)evpòre–pjwjwNote:TheDTFTofxev[n]isX(e),andtheDTFTofm[n]ism(e).re¥¥jw11jæwöNow,fromTable3.1,m(e)=-jw+påd(w+2pk)=-cotçè÷ø+påd(w+2pk).1-e222k=-¥k=-¥SubstitutingtheaboveinEq.(4)wegetpì¥üjw1jqï1jæqöïH(e)=pòXre(e)í2-2cotçè2÷ø+påd(q+2pk)ýdq–pïîk=-¥ïþppjw1jqjjqæw-qö=Xre(e)+2pòXre(e)dq-2pòXre(e)cotçè2÷ødq.–p–pSubstitutingtheaboveinEq.(3)wegetjwjwjwjwX(e)=X(e)+jX(e)=H(e)-x[0]reimppjw1jqjjqæw-qö=Xre(e)+2pòXre(e)dq-2pòXre(e)cotçè2÷ødq-x[0]–p–ppjwjjqæw-qö=Xre(e)-2pòXre(e)cotçè2÷ødq,(5)–pp1jqsinceX(e)dq=x[0],asx[n]isreal.Comparingtheimaginarypartofbothsidesof2pòre–ppjw1jqæw-qöEq.(5)wethereforegetXim(e)=–2pòXre(e)cotçè2÷ødq.–p(b)TakingtheDTFTofbothsidesofEq.(2)wegetjwjwX(e)=G(e)+x[0],(6)pjwjjqj(w-q)where,G(e)=DTFT{2x[n]m[n]}=X(e)m(e)dq,(7)odpòim–pjwjwasjX(e)istheDTFTofxod[n].Substitutingtheexpressionform(e)givenaboveinimpì¥üjwjjqï1jæqöïEq.(7)wegetG(e)=pòXim(e)í2-2cotçè2÷ø+påd(q+2pk)ýdq–pïîk=-¥ïþ56
ppjwjjq1jqæw-qö=jXim(e)+2pòXim(e)dq+2pòXim(e)cotçè2÷ødq–p–pSubstitutingtheaboveinEq.(6)wegetjwjwjwjwX(e)=X(e)+jX(e)=G(e)+x[0]reimppjwjjq1jqæw-qö=jXim(e)+2pòXim(e)dq+2pòXim(e)cotçè2÷ødq+x[0]–p–ppjw1jqæw-qö=jXim(e)+2pòXim(e)cotçè2÷ødq+x[0](8)–pp1jqjwasX(e)dq=0sinceX(e)isanoddfunctionofw.Comparingtherealpartsof2pòimim–ppjw1jqæw-qöbothsidesofEq.(8)wefinallyarriveatXre(e)=2pòXim(e)cotçè2÷ødq+x[0].–pN-1N-1-(k-l)nj2pn(k-l)/N3.30S=åWN=åen=0n=0j2pn(k-l)1-e1-1Ifk–l¹rNthenS===0.1-ej2pn(k-l)/N1-ej2pn(k-l)/NN-1N-1N-1-rnN-j2pnrIfk–l=rNthenS=åWN=åe=å1=N.n=0n=0n=0N-1-(k-l)nìN,fork–l=rN,raninteger,Hence,åWN=í0,otherwise.în=0N-1N-13.31y˜[n]=å˜x[r]h˜[n-r].Theny˜[n+kN]=åx˜[r]h˜[n+kN-r].Sinceh˜[n]isperiodicinnr=0r=0N-1withaperiodN,h˜[n+kN-r]=h˜[n-r].Thereforey˜[n+kN]=åx˜[r]h˜[n-r]=y˜[n],hencer=0y˜[n]isalsoperiodicinnwithaperiodN.3.32x˜[n]={010-23}andh˜[n]={2010-2}.4Now,y˜[0]=å˜x[r]h˜[0-r]=x˜[0]h˜[0]+x˜[1]h˜[4]+˜x[2]˜h[3]+˜x[3]˜h[2]+x˜[4]h˜[1]=–4.r=03Similarlyy˜[1]=å˜x[r]h˜[1-r]=˜x[0]h˜[1]+x˜[1]h˜[0]+x˜[2]h˜[3]+x˜[3]h˜[2]=5.r=0Continuingtheprocesswecanshowthaty˜[2]=4,y˜[3]=-9,andy˜[4]=6.57
3.33x˜[n]={2-123-2}andh˜[n]={12-30-3}.4Now,y˜[0]=å˜x[r]h˜[0-r]=x˜[0]h˜[0]+x˜[1]h˜[4]+˜x[2]˜h[3]+˜x[3]˜h[2]+x˜[4]h˜[1]=–8.r=03Similarlyy˜[1]=å˜x[r]h˜[1-r]=˜x[0]h˜[1]+x˜[1]h˜[0]+x˜[2]h˜[3]+x˜[3]h˜[2]=3.r=0Continuingtheprocesswecanshowthaty˜[2]=-15,y˜[3]=16,andy˜[4]=-8.3.34Sincey˜[n+rN]=y˜[n],henceallthetermswhicharenotintherange0,1,....N-1canbekkaccumulatedtoy˜[n],where0£k£N–1.HenceinthiscasetheFourierseriesrepresentationkinvolvesonlyNcomplexexponentialsequences.LetN-1x˜[n]=1åX˜[k]ej2pkn/NthenNk=0N-1N-1N-1N-1N-1åx˜[n]e-j2prn/N=1ååX˜[k]ej2p(k-r)n/N=1åX˜[k]åej2p(k-r)n/N.NNn=0n=0k=0k=0n=0NowfromEq.(3.28),theinnersummationisequaltoNifk=r,otherwiseitisequalto0.N-1Thusåx˜[n]e-j2prn/N=X˜[r].n=0N-1N-1N-1X˜[k+lN]=å˜x[n]e-j2p(k+lN)n/N=å˜x[n]e-j2pkn/Ne-j2pln=åx˜[n]e-j2pkn/N=X˜[k].n=0n=0n=0æpnö1jpn/4-jpn/4x˜3.35(a)x˜1[n]=cosçè÷ø={e+e}.Theperiodof1[n]isN=8.42ì77üX˜[k]=1ïíåej2pn/8e-j2pkn/8+åe-j2pn/8e-j2pkn/8ïý12ïïîn=0n=0þì77ü1ï-j2pn(k-1)/8-j2pn(k+1)/8ï=íåe+åeý.Now,fromEq.(3.28)weobserve2ïïîn=0n=0þ77-j2pn(k-1)/88,fork=1,-j2pn(k+1)/88,fork=7,åe={0,otherwise,andåe={0,otherwise.Hence,n=0n=0X˜[k]=4,k=1,7,1{0,otherwise.æpnöæpnö1jpn/3-jpn/33jpn/4-jpn/4(b)x˜2[n]=sinçè÷ø+3cosçè÷ø={e-e}+{e+e}.Theperiodof342j2æpnöæpnösinç÷is6andtheperiodofcosç÷is8.Hence,theperiodofx˜2[n]isthegcmofè3øè4øì2323ü1ïj8pn/24-j2pkn/24-j8pn/24-j2pkn/24ï(6,8)andis24.X˜2[k]=íåee-åeeý2jïïîn=0n=0þ58
ì2323ü3ïj6pn/24-j2pkn/24-j6pn/24-j2pkn/24ï+íåee+åeeý2ïïîn=0n=0þì2323üì2323ü1ï-j2pn(k-3)/24-j2pn(k+3)/24ï3ï-j2pn(k-4)/24-j2pn(k+4)/24ï=íåe-åeý+íåe+åeý.2jïï2ïïîn=0n=0þîn=0n=0þì-j12,k=3,ïj12,k=21,HenceX˜[k]=í2ï36,k=4,20,î0,otherwise.3.36Since˜p[n]isperiodicwithperiodN,thenfromEq.(3.168a)0fProblem3.34,N-1N-1˜p[n]=1åP˜[k]e-j2pkn/NwhereusingEq.(3.168b)wegetP˜[k]=åp˜[n]e-j2pkn/N=1.Nk=0n=0N-11-j2pkn/NHence˜p[n]=åe.Nk=0¥jwj2pk/N-j2pkn/N3.37X˜[k]=X(e)=X(e)=åx[n]e,–¥N].ThereforeX[k]=åg[N]WNn=0n-1N-10nknk=åg[N+n-n0]WN+åg[n-n0]WNn=0n=n0N-1N-n-1N-10(n+n-N)k(n+n)knknknk=åg[n]W0+g[n]W00g[n]W0G[k].NåN=WNåN=WNn=N-nn=0n=00N-1N-1-k0nnk(k-k0)n(c)u[n]=WNg[n].HenceU[k]=åu[n]WN=åg[n]WNn=0n=0ìN-1(k-k)nïW0g[n],ifk³k,ïåN0ï=ín=0N-1ï(N+k-k)nïåW0g[n],ifkN].îG[N+k-k0],ifk].îNg[N-k],ifk>0,NN-1N-1N-1nk(e)u[n]=åg[m]h[N].Therefore,U[k]=ååg[m]h[N]WNm=0n=0m=0N-1N-1N-1nkmk=åg[m]åh[N]WN=åg[m]H[k]WN=H[k]G[k].m=0n=0m=060
N-1N-1N-1nk1nk-nr(f)v[n]=g[n]h[n].Therefore,V[k]=åg[n]h[n]WN=ååh[n]G[r]WW=Nn=0n=0r=0N-1N-1N-11(k-r)n1åG[r]åh[n]W=åG[r]H[N].NNr=0n=0r=0N-1N-11-nk1nk(g)x[n]=åX[k]W.Thusx*[n]=åX*[k]W.Therefore,NNk=0k=0N-1N-1æN-1öæN-1öN-1N-1N-121ç-nr÷çnl÷1*n(l-r)åx[n]=åN2çåX[r]W÷çåX*[l]W÷=N2ååX[r]X[l]åW.n=0n=0èr=0øèl=0ør=0l=0n=0N-1N-1212Sincetheinnersumisnon-zeroonlyifl=r,wegetåx[n]=åX[k].Nn=0k=0N-1nk3.40X[k]=åx[n]W.n=0N-1-nk(a)X*[k]=åx*[n]W.ReplacingkbyN–konbothsidesweobtainn=0N-1N-1-n(N-k)nkX*[N–k]=åx*[n]W=åx*[n]W.Thusx*[n]ÛX*[N–k]=X*[<–k>N].n=0n=0N-1-nk(b)X*[k]=åx*[n]W.ReplacingnbyN–ninthesummationwegetn=0N-1N-1-(N-n)knkX*[k]=åx*[N-n]W=åx*[N-n]W.n=0n=0Thusx*[N–n]=x*[<–n>N]ÛX*[k].1(c)Re{x[n]}={x[n]+x*[n]}.NowtakingDFTofbothsidesandusingresultsofpart(a)21wegetRe{x[n]}Û{X[k]+X*[<–k>N]}.211(d)jIm{x[n]}={x[n]–x*[n]}thisimplesjIm{x[n]}Û{X[k]–X*[<–k>N]}.221(e)xpcs[n]={x[n]+x*[<–n>N]}Usinglinearityandresultsofpart(b)weget21xpcs[n]Û{X[k]+X*[k]}=Re{X[k]}.21(f)xpca[n]={x[n]–x*[<–n>N]}.Againusingresultsofpart(b)andlinearityweget21xpca[n]Û{X[k]–X*[k]}=jIm{X[k]}.261
N-1–j2pkn/N3.41X[k]=Re{X[k]}+jIm{X[k]}=åx[n]e.n=01DFT(a)x[n]={x[n]+x[<-n>]}.FromTable3.6,x*[<-n>]ÛX*[k].Sincex[n]ispe2NNDFT1real,x[<-n>]=x*[<-n>]ÛX*[k].Thus,X[k]={X[k]+X*[k]}=Re{X[k]}.NNpe211(b)x[n]={x[n]-x[<-n>]}.Asaresult,X[k]={X[k]-X*[k]}=jIm{X[k]}.po2Npo23.42Sinceforarealsequence,x[n]=x*[n],takingDFTofbothsideswegetX[k]=X*[<–k>N].Thisimplies,Re{X[k]}+jIm{X[k]}=Re{X[<–k>N}–jIm{X[<–k>N}.ComparingrealandimaginarypartswegetRe{X[k]}=Re{X[<–k>N}andIm{X[k]}=–Im{X[<–k>N}.22AlsoX[k]=(Re{X[k]})+(Im{X[k]})22=(Re{X[<–k>]})+(–Im{X[<–k>]})=X[<–k>]NNNæöæ–Im{X[<-k>]}öandarg{X[k]}=tan-1çIm{X[k]}÷=tan-1çN÷=-arg{X[<-k>]}.èRe{X[k]}øçèRe{X[<-k>]}÷øNN3.43(a)x1[<-n>8]=[11100011]=x1[n].Thus,x1[n]isaperiodicevensequence,andhenceithasareal-valued8-pointDFT.(b)x2[<-n>8]=[1-1-100001]..Thus,x2[n]isneitheraperiodicevenoraperiodicoddsequence.Hence,its8-pointDFTisacomplexsequence.(c)x3[<-n>8]=[0-1-100011]=-x3[n].Thus,x3[n]isaperiodicoddsequence,andhenceithasanimaginary-valued8-pointDFT.(d)x4[<-n>8]=[01100011]=x4[n].Thus,x4[n]isaperiodicevensequence,andhenceithasareal-valued8-pointDFT.N-1N-1nN/2n3.44(a)Now,X[N/2]=åx[n]WN=å(-1)x[n].Henceifx[n]=x[N–1–n]andNisn=0n=0N-1neven,thenå(-1)x[n]=0orX[N/2]=0.n=0N-1(b)X[0]=åx[n]soifx[n]=–x[N–1–n],thenX[0]=0.n=062
N-1N-12N-12nl2nl2nl(c)X[2l]=åx[n]W=åx[n]W+åx[n]Wn=0n=0n=N/2NNN-1-1-12222nlN2nlN2nl=åx[n]W+åx[n+]W=å(x[n]+x[n+])W.22n=0n=0n=0NHenceifx[n]=–x[n+]=–x[n+M],thenX[2l]=0,forl=0,1,....,M–1.2N-1N-12N-12mn2mn2mn3.45X[2m]=åx[n]WN=åx[n]WN+åx[n]WNn=0n=0Nn=2NNNN-1-1N-1-122N2m(n+)22N=x[n]W2mn]W22mn]W2mnmNåN+åx[n+N=åx[n]WN+åx[n+NWN22n=0n=0n=0n=0N-12æNöNN2mn=åçx[n]+x[n+]÷WN=0,0£m£-1.Thisimpliesx[n]+x[n+]=0.è2ø22n=03.46(a)Usingthecirculartime-shiftingpropertyoftheDFTgiveninTable3.5weobservekm1km2DFT{x[N}=WNX[k]andDFT{x[N}=WNX[k].Hence,km1km2km1km2W[k]=DFT{x[n]}=aWNX[k]+bWNX[k]=(aWN+bWN)X[k].Aproofofthecirculartime-shiftingpropertyisgiveninProblem3,39.æNö11-n(b)g[n]=(x[n]+(-1)nx[n])=çx[n]+W2x[n]÷,Usingthecircularfrequency-shiftingN22ç÷èø1ìNüpropertyoftheDFTgiveninTable3.5,wegetG[k]=DFT{g[n]}=íX[k]+X[N]ý.2î2þ(c)UsingthecircularconvolutionpropertyoftheDFTgiveninTable3.5weget2Y[k]=DFT{y[n]}=X[k]×X[k]=X[k].AproofofthecircularconvolutionpropertyisgiveninProblem3,39.NìNük3.47(a)DFTíx[n-]ý=WN2X[k]=-X[k].Hence,î2þìNüu[k]=DFT{u[n]}=DFTíx[n]+x[n-]ý=X[k]-X[k]=0.î2þìNü(b)V[k]=DFT{v[n]}=DFTíx[n]-x[n-]ý=X[k]+X[k]=2X[k].î2þ63
NìNünnïnïN(c)y[n]=(-1)x[n]=WN2x[n].Hence,Y[k]=DFT{y[n]}=DFTíWN2x[n]ý=X[N]ïï2îþusingthecircularfrequency-shiftingpropertyoftheDFTgiveninTable3.5.3.48(a)Fromthecircularfrequency-shiftingpropertyoftheDFTgiveninTable3.5,-m1n-m2nIDFT{X[N]}=WNx[n]andIDFT{X[N]}=WNx[n].Hence,w[n]=IDFT{W[k]}=IDFT{aX[N+bX[N}-m1n-m2n-m1n-m2n=aWNx[n]+bWNx[n]=(aWN+bWN)x[n].æNö11-k(b)G[k]=(X[k]+(-1)kX[k])=çX[k]+W2X[k]÷.Usingthecirculartime-shiftingN22ç÷èø1æNöpropertyoftheDFTgiveninTable3.5,wegetg[n]=IDFT{G[k]}=çx[n]+x[N]÷.2è2ø(c)UsingthemodulationpropertyoftheDFTgiveninTable3.5weget2y[n]=IDFT{Y[k]}=N×x[n]×x[n]=N×x[n].N-1N-12N-12mn2mn2mn3.49(a)X[2m]=åx[n]WN=åx[n]WN+åx[n]WNn=0n=0Nn=2NNNN-1-1N-1-122N2m(n+)22N=x[n]W2mn]W22mn]W2mnmNåN+åx[n+N=åx[n]WN+åx[n+NWN22n=0n=0n=0n=0NN-1-12æNö2N2mn2mn=åçx[n]+x[n+]÷WN=å(x[n]-x[n])WN=0,0£m£-1.è2ø2n=0n=0NN3N-1-1-1N-1424N-14ln4ln4ln4ln4ln(b)X[4l]=åx[n]WN=åx[n]WN+åx[n]WN+åx[n]WN+åx[n]WNn=0n=0NN3Nn=n=n=424N-1NN3Nö4æ4lnN4l(n+)N4l(n+)3N4l(n+)=åçx[n]WN+x[n+]WN4+x[n+]WN2+x[n+]WN4÷ç424÷n=0èøN-14æNlNN2lN3N3lNö4ln=åçx[n]+x[n+]WN+x[n+]WN+x[n+]WN÷WNè424øn=0N-144lnlN2lN3lN=å(x[n]-x[n]+x[n]-x[n])WN=0asWN=WN=WN=1.n=064
N-1N-1(N-k)n-kn3.50(a)X[N-k]=åx[n]WN=åx[n]WN=X*[k].n=0n=0N-1N-10(b)X[0]=åx[n]WN=åx[n]whichisreal.n=0n=0NN-1N-1(N/2)nn(c)X[]=åx[n]WN=å(-1)x[n]whichisreal.2n=0n=06pk-j3.51(a)H[k]=DFT{h[n]}=DFTg[{n-3>3kG[k]=e7G[k]{7]}=W7é6p12p24p30p36pù-j-j-j-j-j=ê1+j2,e7(-2+j3),e7(-1-j2),0,e7(8+j4),e7(-3+j2),e7(2+j5)úêúêëúû8pnj(b)h[n]=IDFT{H[k]}=IDFTG[-4ng[n]=e7g[n]{7]}=W7j8p/7j16p/7j24p/7j32p/7j40p/7j42p/7=[-3.1,2.4e,4.5e,-6e,e,-3e,7e].MN-1N-1N-1nknknk3.52Y[k]=åy[n]WMN=åx[n]WMN.Thus,Y[kM]=åx[n]WN=X[k].n=0n=0n=0Hence,X[k]=Y[kM].3.53NoteX[k]istheMN-pointDFTofthesequencexe[n]obtainedfromx[n]byappendingitwithM(N-1)zeros.Thus,thelength-MNsequencey[n]isgivenbyM-1y[n]=åxe[MN],0£n£MN-1.TakingtheMN-pointDFTofbothsideswel=0æM-1öæM-1öNklklgetY[k]=çåWMN÷X[k]==çåWM÷X[k].èl=0øèl=0ø113.54(a)X[0]=åx[n]=13.n=011n(b)X[6]=å(-1)x[n]=-13.n=011(c)åX[k]=12×x[0]=36.k=0-j(4pk/6)(d)TheinverseDFTofeX[k]isx[12].Thus,11-j(4pk/6)åeX[k]=12×x[<0-4>12]=12×x[8]=-48.k=065
111122(e)FromParseval"srelation,åX[k]=12×åx[n]=1500.k=0n=03.55X[8]=X*[<-8>14]=X*[6]=-2+j3,X[9]=X*[<-9>14]=X*[5]=6-j3,X[10]=X*[<-10>14]=X*[4]=-2-j2,X[11]=X*[<-11>14]=X*[3]=1+j5,X[12]=X*[<-12>14]=X*[2]=3-j4,X[13]=X*[<-13>14]=X*[1]=-1-j3.11332(a)x[0]=åX[k]==2.2857,1414k=011312k(b)x[7]=å(-1)X[k]=-=-0.8571,1414k=013(c)åx[n]=X[0]=12,n=0j(4pn/7)-4n-4n(d)Letg[n]=ex[n]=W14x[n].ThenDFT{g[n]}=DFT{W14x[n]}=X[14]=[X[10]X[11]X[12]X[13]X[0]X[1]X[2]X[3]X[4]X[5]X[6]X[7]X[8]X[9]]1313j(4pn/7)Thus,åg[n]=åex[n]=X[10]=-2–j2,n=0n=01321132498(e)UsingParseval"srelation,åx[n]=åX[k]==35.5714.1414n=0k=063.56Nowyc[n]=åg[k]h[6].Hence,k=0yc[0]=g[0]h[0]+g[1]h[6]+g[2]h[5]+g[3]h[4]+g[4]h[3]+g[5]h[2]+g[6]h[1],yc[1]=g[0]h[1]+g[1]h[0]+g[2]h[6]+g[3]h[5]+g[4]h[4]+g[5]h[3]+g[6]h[2],yc[2]=g[0]h[2]+g[1]h[1]+g[2]h[0]+g[3]h[6]+g[4]h[5]+g[5]h[4]+g[6]h[3],yc[3]=g[0]h[3]+g[1]h[2]+g[2]h[1]+g[3]h[0]+g[4]h[6]+g[5]h[5]+g[6]h[4],yc[4]=g[0]h[4]+g[1]h[3]+g[2]h[2]+g[3]h[1]+g[4]h[0]+g[5]h[6]+g[6]h[5],yc[5]=g[0]h[5]+g[1]h[4]+g[2]h[3]+g[3]h[2]+g[4]h[1]+g[5]h[0]+g[6]h[6],yc[6]=g[0]h[6]+g[1]h[5]+g[2]h[4]+g[3]h[3]+g[4]h[2]+g[5]h[1]+g[6]h[0].6Likewise,yL[n]=åg[k]h[n-k].Hence,k=0y[0]=g[0]h[0],Ly[1]=g[0]h[1]+g[1]h[0],Ly[2]=g[0]h[2]+g[1]h[1]+g[2]h[0],Ly[3]=g[0]h[3]+g[1]h[2]+g[2]h[1]+g[3]h[0],LyL[4]=g[0]h[4]+g[1]h[3]+g[2]h[2]+g[3]h[1]+g[4]h[0],yL[5]=g[0]h[5]+g[1]h[4]+g[2]h[3]+g[3]h[2]+g[4]h[1]+g[5]h[0],yL[6]=g[0]h[6]+g[1]h[5]+g[2]h[4]+g[3]h[3]+g[4]h[2]+g[5]h[1]+g[6]h[0],66
yL[7]=g[1]h[6]+g[2]h[5]+g[3]h[4]+g[4]h[3]+g[5]h[2]+g[6]h[1],yL[8]=g[2]h[6]+g[3]h[5]+g[4]h[4]+g[5]h[3]+g[6]h[2],yL[9]=g[3]h[6]+g[4]h[5]+g[5]h[4]+g[6]h[3],yL[10]=g[4]h[6]+g[5]h[5]+g[6]h[4],yL[11]=g[5]h[6]+g[6]h[5],yL[12]=g[6]h[6].Comparingy10[n]withyL[n]weobservethatyc[0]=yL[0]+yL[7],yc[1]=yL[1]+yL[8],yc[2]=yL[2]+yL[9],yc[3]=yL[3]+yL[10],yc[4]=yL[4]+yL[11],yc[5]=yL[5]+yL[12],yc[6]=yL[6].3.57Sincex[n]isarealsequence,itsDFTsatisfiesX[k]=X*[<-k>N]whereN=11inthiscase.Therefore,X[1]=X*[<-1>11X*[10]=3+j2,X[3]=X*[<-3>11X*[8]=-5+j8,X[5]=X*[<-5>11X*[6]=9+j6,X[7]=X*[<-7>11X*[4]=2-j5,X[9]=X*[<-9>11X*[2]=-1-j3.3.58TheN-pointDFTX[k]ofalength-Nrealsequencex[n]satisfyX[k]=X*[<-k>].HereNN=11.Hence,theremaining5samplesareX[1]=X*[<-1>]=X*[10]=-3.1-j5.2,11X[4]=X*[<-4>]=X*[7]=-4.1-j0.2,X[6]=X*[<-6>]=X*[5]=6.5-j9,1111X[8]=X*[<-8>]=X*[3]=5.3+j4.1,X[9]=X*[<-9>]=X*[2]=-3.2+j2.11113.59Alength-Nperiodicevensequencex[n]satisfyingx[n]=x*[<-n>]hasareal-valuedN-NpointDFTX[k].HereN=10.Hence,theremaining4samplesofx[n]aregivenbyx[6]=x*[<-6>]=x*[4]=2.87-j2,x[7]=x*[<-7>]=x*[3]=-2.1-j4.6,1010x[8]=x*[<-8>]=x*[2]=-3.25-j1.12,andx[9]=x*[<-9>]=x*[1]=0.7+j0.08.10103.60Asx[n]isareal-valuedsequenceoflength498,its498-pointDFTX[k]satisfyX[k]=X*[<-k>498]=X*[498-k](SeeTable3.7).(a)FromthespecifiedDFTsamplesweobservethatX[k1]=X*[412]implyingk1=498-412=86,X[k2]=X*[309]implyingk2=498-309=189,X[k3]=X*[112]implyingk3=498-112=386,X[k4]=X*[11]implyingk4=498-11=487.(b)dcvalueof{x[n]}=X[0]=2.1497-kn1-11n-86n(c)x[n]=åX[k]W498=(X[0]+2Re{X[11]×W498}+2Re{X[86]×W498}498498k=067
-112n-189n-249n+2Re{X[112]×W498}+2Re{X[189]×W498}+X[249]×W498)1æ11pnöæ11pnöæ86pnöæ86pnö={-0.45+7cosç÷-3.1sinç÷-2.2cosç÷+1.5sinç÷249è249øè249øè249øè249øæ112pnöæ112pnöæ189pnöæ189pnö+3cosç÷+0.7sinç÷-4.7cosç÷-1.9sinç÷}.è249øè249øè249øè249ø497149722(d)åx[n]=åX[k]=0.2275.498n=0k=03.61Asx[n]isareal-valuedsequenceoflength316,its316-pointDFTX[k]satisfyX[k]=X*[<-k>]=X*[316-k](SeeTable3.7).316(a)FromthespecifiedDFTsamplesweobservethatX[17]=X*[k4]implyingk=316-17=299,X[k]=X*[210]ife=0,implyingk=316-210=106,X[k]=X*[179]4112ifd=0implyingk=316-179=137,andX[k]=X*[110]ifg=0implying23k=316-110=206.3315(b)Now,X[0]=åx[n]whichisarealnumberasx[n]arerealnumbersimplyinga=0.Sincen=0315nthelengthN=316isanevennumber,X[158]=X[N/2]=å(-1)x[n]isalsoarealnumbern=0implyingb=0.WehavealreadyshowninPart(a),d=e=g=0.(c)Thedcvalueof{x[n]}isX[0]=3.1-17n-106n-137n(d)x[n]={X[0]+2Re(X[17]W316)+2Re(X[106]W316)+2Re(X[137]W316)316-110n1æ17pnöæ106pnö+2Re(X[110]W316)}={3+2(1.5)cosç÷+2(-2.3)sinç÷316è158øè158øæ137pnöæ110pnö+2(4.2)cosç÷+2(1.72)sinç÷-13}è158øè158ø1æ17pnöæ106pnöæ137pnöæ110pnö={-10+3cosç÷-4.6sinç÷+8.4)cosç÷+3.44sinç÷}.316è158øè158øè158øè158ø315131522(e)åx[n]=åX[k]=0.6521.316n=0k=03.62{x[n]}={-4,5,2,-3,0,-2,3,4},0£n£7.LetX[k]denotethe8-pointDFTof3k6kx[n].Considerthe8-pointDFTY[k]=WX[k]=WX[k].Usingthecirculartime-shifting48propertyoftheDFTgiveninTable3.5weobservethattheIDFTy[n]ofY[k]isgivenbyy[n]=x[].Therefore,y[0]=x[<-6>]=x[2]=2,y[1]=x[<1-6>]=x[3]=-3,888y[2]=x[<2-6>]=x[4]=0,y[3]=x[<3-6>]=x[5]=-2,y[4]=x[<4-6>]=x[6]=3,88868
y[5]=x[<5-6>]=x[7]=4,y[6]=x[<6-6>]=x[0]=-4,y[7]=x[<7-6>]=x[1]=5.Thus,888{y[n]}={2,-3,0,-2,3,4,-4,5},0£n£7.3.63{x[n]}={1,-1,2,3,0,0},0£n£5.LetX[k]denotethe6-pointDFTofx[n].2k4kConsiderthe6-pointDFTY[k]=W3X[k]=W6X[k].Usingthecirculartime-shiftingpropertyoftheDFTgiveninTable3.5weobservethattheIDFTy[n]ofY[k]isgivenbyy[n]=x[6].Therefore,y[0]=x[<-4>6]=x[2]=2,y[1]=x[<-3>6]=x[3]=3,y[2]=x[<-2>6]=x[4]=0,y[3]=x[<-1>6]=x[5]=0,y[4]=x[<0>6]=x[0]=1,y[5]=x[<1>6]=x[1]=-1.Thus,{y[n]}={23001-1},0£n£5.3.64(a)yL[0]=g[0]h[0]=-6,yL[1]=g[0]h[1]+g[1]h[0]=16,yL[2]=g[0]h[2]+g[1]h[1]+g[2]h[0]=0,yL[3]=g[0]h[3]+g[1]h[2]+g[2]h[1]=-19,yL[4]=g[1]h[3]+g[2]h[2]=2,yL[5]=g[2]h[3]=4.(b)yc[0]=ge[0]h[0]+ge[1]h[3]+ge[2]h[2]+ge[3]h[1]==g[0]h[0]+g[1]h[3]+g[2]h[2]=-4,yc[1]=ge[0]h[1]+ge[1]h[0]+ge[2]h[3]+ge[3]h[2]==g[0]h[1]+g[1]h[0]+g[2]h[3]=20,yc[2]=ge[0]h[2]+ge[1]h[1]+ge[2]h[0]+ge[3]h[3]==g[0]h[2]+g[1]h[1]+g[2]h[0]=0,yc[3]=ge[0]h[3]+ge[1]h[2]+ge[2]h[1]+ge[3]h[0]==g[0]h[3]+g[1]h[2]+g[2]h[1]=-19.éGe[0]ùé1111ùé-3ùé3ùéH[0]ùé1111ùé2ùé-1ùêúêúêúêúêúêúêúêú(c)êGe[1]ú=ê1-j-1júê2ú=ê-7-j2ú,êH[1]ú=ê1-j-1júê-4ú=ê2+j5ú.êGe[2]úê1-11-1úê4úê-1úêH[2]úê1-11-1úê0úê5úêúêúêúêúêúêúêúêúêëGe[3]úûêë1j-1-júûêë0úûêë-7+j2úûêëH[3]úûêë1j-1-júûêë1úûêë2-j5úûéYc[0]ùéGe[0]×H[0]ùé-3ùêúêúêúêYc[1]ú=.êGe[1]×H[1]ú=ê-4-j39ú.ThereforeêYc[2]úêGe[2]×H[2]úê-5úêúêúêúêëYc[3]úûêëGe[3]×H[3]úûêë-4+j39úûéyc[0]ùé1111ùé-3ùé-4ùêúêúêúêúêyc[1]ú=1ê1j-1-jú×ê-4-j39ú=ê20ú.êyc[2]ú2ê1-11-1úê-5úê0úêúêúêúêúêëyc[3]úûêë1-j-1júûêë-4+j39úûêë-19úû3.65Weneedtoshowg[n]h[n]N=h[n]g[n].NN-1Letx[n]=g[n]h[n]N=åg[m]h[N]m=0N-1andy[n]=h[n]g[n]N=åh[m]g[N]m=069
nN-1=åh[m]g[n-m]+åh[m]g[N+n-m]m=0m=n+1nN-1N-1=åh[n-m]g[m]+åh[N+n-m]g[m]=åh[N]g[m]=x[n].m=0m=n+1m=0Hencecircularconvolutioniscommutative.N-13.66(a)Letg[n]=x[n]1Nx[n]2=åx1[m]x2[N].Thus,m=0N-1N-1N-1æN-1öæN-1öåg[n]=åx1[m]åx2[N]=çåx1[n]÷çåx1[m]÷.Similarlywecanshowthatifn=0m=0n=0èn=0øèm=0øN-1N-1N-1æN-1öæN-1öy[n]=g[n]Nx[n]3,thenåy[n]=åg[m]åx3[N]=çåg[n]÷çåx3[m]÷n=0m=0n=0èn=0øèm=0øæN-1N-1öæN-1ö=çåx1[n]åx2[m]÷çåx3[m]÷.èn=0m=0øèm=0øN-1N-1N-1nn(b)å(-1)g[n]=åx1[m]åx2[N](-1)n=0m=0n=0æN-1öæm-1N-1önn=çåx1[m]÷çåx2[N+n-m](-1)+åx2[n-m](-1)÷.èm=0øèn=0n=møReplacingnbyN+n–minthefirstsumandbyn–minthesecondweobtainN-1æN-1öæm-1N-1önn-N+mn+må(-1)g[n]=çåx1[m]÷çåx2[n](-1)+åx2[n](-1)÷n=0èm=0øèn=0n=0øæN-1öæN-1önn=çå(-1)x1[n]÷çå(-1)x2[n]÷.Similarlywecanshowthatify[n]=g[n]Nx[n]3,thenèn=0øèn=0øN-1æN-1öæN-1öæN-1öæN-1öæN-1önnnnnnå(-1)y[n]=çå(-1)g[n]÷çå(-1)x3[n]÷=çå(-1)x1[n]÷çå(-1)x2[n]÷çå(-1)x3[n]÷.n=0èn=0øèn=0øèn=0øèn=0øèn=0øæ2plnöx[n]-j2pln/Nj2pln/N1nl1-nl3.67y[n]=cosçè÷øx[n]=(e+e)=x[n]WN+x[n]WN.N22211HenceY[k]=X[]+X[].2N2NNN-1-144Nnknk3.68y[n]=x[4n],0£n£-1.Therefore,Y[k]=åy[n]WN/4=åx[4n]WN/4.4n=0n=070
1N-11N-1-4mn-mnNow,x[4n]=åX[m]WN=åX[m]WN/4.Hence,NNm=0m=0NN-1-114N-11N-14-mnnk(k-m)nY[k]=ååX[m]WN/4WN/4=åX[m]åWN/4.Since,NNn=0m=0m=0n=0N-14ìïNN2N3N4N(k-m)n,m=k,k+,k+,k+,k+,åWN/4=í44444Thus,n=0îï0,elsewhere,1N2N3NY[k]=(X[k]+X[k+]+X[k+]+X[k+]).4444V[k]=[-2+j3,1+j5,-4+j7,2+j6,-1-j3,4-j3+j8,j6].Hence,V*[<-k>8]=[-2-j3,-j6,3-j8,4+j,-1+j3,2-j6,-4-j7,1-j5].Therefore,,X[k]=[-2,0.5-j0.5,-0.5-j0.5,3+j3.5,-1,3-j3.5,-0.5+j0.5,0.5+j0.5]andY[k]=[3,5.5-j0.5,7.5+j3.5,2.5+j,-3,2.5-j,7.5-j3.5,5.5+j0.5]13.69v[n]=x[n]+jy[n].Hence,X[k]={V[k]+V*<-k>8]}isthe8-pointDFTofx[n],and21Y[k]={V[k]-V*<-k>8]}isthe8-pointDFTofy[n].Now,2jV[k]=[-2+j3,1+j5,-4+j7,2+j6,-1-j3,4-j,3+j8,j6]V*[<-k>]=[-2-j3,-j6,3-j8,4+j,-1+j3,2-j6,-4-j7,1-j5].Therefiore,8X[k]=[-0.2,0.5-j0.5,-0.5-j0.5,3+j3.5,-1,3-j3.5,-0.5+j0.5,0.5+j0.5]Y[k]=[3,5.5-j0.5,7.5+j3.5,2.5+j-3,2.5-j,7.5-j3.5,5.5+j0.5]3.70v[n]=g[n]+jh[n]=[-3+j2,2-j4,4,j].Therefore,eéV[0]ùé1111ùé-3+j2ùé3-jùêV[1]úê1-j-1júê2-j4úê-12úêV[2]ú=ê1-11-1úê4ú=ê-1+j5ú,i.e.,{V[k]}=[3-j,-12,-1+j5,-2+j4].êúêúêúêúëV[3]ûë1j-1-jûëjûë-2+j4ûThus,{V*[<-k>]}=[3+j,-2-j4,-1-j5,-12]41Therefore,G[k]={V[k]+V*[<-k>]}=[3,-7-j2,-1,-7+j2]ande241H[k]={V[k]-V*[<-k>]}=[-1,2+j5,5,2-j5].2j43.71v[n]=g[n]+jh[n]=[-2+j,1+j2,-3-j3,4+j2].Therefore,éV[0]ùé1111ùé-2+jùéj2ùêV[1]úê1-j-1júê1+j2úê1+j7úêV[2]ú=ê1-11-1úê-3-j3ú=ê-10-j6ú,i.e.,{V[k]}=[j2,1+j7,-10-j6,1+j].êúêúêúêúëV[3]ûë1j-1-jûë4+j2ûë1+jû71
Thus,{V*[<-k>]}=[-j2,1-j,-10+j6,1-j7].41Therefore,G[k]={V[k]+V*[<-k>]}=[0,1+j3,-10,1-j3]ande241H[k]={V[k]-V*[<-k>]}=[2,4,-6,4].2j43.72(a)Letp[n]=IDFT{P[k]}.Thus,ép[0]ùé1111ùé4ùé8ùé2ùêp[1]ú1ê1j-1-júê1+j7ú1ê-12úê-3úêp[2]ú=4ê1-11-1úê2ú=4ê4ú=ê1ú.Similarly,letd[n]=IDFT{D[k]}.êúêúêúêúêúëp[3]ûë1-j-1jûë1-j7ûë16ûë4ûéd[0]ùé1111ùé4.5ùé2ùé0.5ùêd[1]ú1ê1j-1-júê1.5+jú1ê8úê2úêd[2]ú=4ê1-11-1úê-5.5ú=4ê-4ú=ê-1ú.Therefore,êúêúêúêúêúëd[3]ûë1-j-1jûë1.5-jûë12ûë2û-jw-j2w-j3wjw2-3e+e+4eX(e)=.0.5+2e-jw-e-j2w+3e-j3wép[0]ùé1111ùé7ùé3ùêp[1]ú1ê1j-1-júê7+j2úê3ú(b)Letp[n]=IDFT{P[k]}.Thus,êp[2]ú=4ê1-11-1úê-9ú=ê-4ú.Similarly,letêúêúêúêúëp[3]ûë1-j-1jûë7-j2ûë5ûéd[0]ùé1111ùé0ùé1ùêd[1]ú1ê1j-1-júê4+j6úê-2úd[n]=IDFT{D[k]}.Thus,êd[2]ú=4ê1-11-1úê-4ú=ê-3ú.Therefore,êúêúêúêúëd[3]ûë1-j-1jûë4-j6ûë4û-jw-j2w-j3wjw3+3e-4e+5eX(e)=.1-2e-jw-3e-j2w+4e-j3wN-1N-13.73X(ejw)=åx[n]e-jwnandXˆ[k]=åx[n]e-j2pkn/M.n=0n=0M-1M-1N-11ˆ-nk1-j2pkm/M-nkNowxˆ[n]=åX[k]WM=ååx[m]eWMMMk=0k=0m=0N-1M-1¥1-j2pk(m-n)/M=åx[m]åe=åx[n+rM]..Mm=0k=0r=-¥Thusxˆ[n]isobtainedbyshiftingx[n]bymultiplesofMandaddingtheshiftedcopies.SincethenewsequenceisobtainedbyshiftinginmultiplesofM,hencetorecovertheoriginalsequencetakeanyMconsecutivesamples.Thiswouldbetrueonlyiftheshiftedcopiesofx[n]didnotoverlapwitheachother,thatis,ifonlyifM³N.77jw-jwn-j2pkn/103.74(a)X(e)=åx[n]e.Therefore,X1[k]=åx[n]e.Hence,n=0n=072
99æ7ö1j2pkn/101çj2pkm/10÷j2pkn/10x1[n]=10åX1[k]e=10åçåx[m]e÷ek=0k=0èm=0ø79¥1j2pk(n-m)/10=åx[m]åe=åx[n+10r]usingtheresultofProblem3.74.10m=0k=0r=-¥SinceM=10andN=8,M>N,andhencex[n]isrecoverablefromx[n].Infact1{x[n]}={1111111100}andx[n]isgivenbythefirst8samplesofx[n].117-j2pkn/6(b)Here,X2[k]=åx[n]e.Hence,n=055æ7ö1j2pkn/61çj2pkm/6÷j2pkn/6x2[n]=6åX1[k]e=10åçåx[m]e÷ek=0k=0èm=0ø76¥1j2pk(n-m)/6=åx[m]åe=åx[n+6r].SinceM=6andN=8,M40]=åh[k]x[40].k=0k=12Nowforn³27,x[]=x[n-k].Thusu[n]=y[n]for27£n£39.403.77(a)Overlapandaddmethod:Sincetheimpulseresponseisoflength55andtheDFTsizetobeusedis64,hencethenumberofdatasamplesrequiredforeachconvolutionwillbe64–54=10.ThusthetotalnumberofDFT"srequiredforlength-1100datasequenceisé1100ùêú=110.AlsotheDFToftheimpulseresponseneedstobecomputedonce.Hence,theê10útotalnumberofDFT"susedare=110+1=111.ThetotalnumberofIDFT"susedare=110.(b)Overlapandsavemethod:Inthissincethefirst55–1=54pointsarelost,weneedtopadthedatasequencewith54zerosforatotallengthof1154.Againeachconvolutionwillresultin64–54=10correctvalues.ThusthetotalnumberofDFT"srequiredforthedataareé1154ùthusêú=116.Again1DFTisrequiredfortheimpulseresponse.Thusê10úThetotalnumberofDFT"susedare=116+1=117.ThetotalnumberofIDFT"susedare=116.73
ìx[n/L],n=0,L,2L,.....,(N-1)L,3.78(a)y[n]=íî0,elsewhere.NL-1N-1N-1nknLknkY[k]=åy[n]WNL=åx[n]WNL=åx[n]WN.n=0n=0n=0Fork³N,letk=k0+rNwherek0=N.Then,N-1N-1n(k+rN)nkY[k]=Y[k+rN]=åx[n]W000N=åx[n]WN=X[k0]=X[N].n=0n=0(b)SinceY[k]=X[7]fork=0,1,2,.....,20,asketchofY[k]isthusasshownbelow.43Y[k]21k012345678203.79x[n]=x[2n+1]+x[2n],x[n]=x[2n+1]-x[2n],y[n]=y[2n+1]+y[2n],and011Ny[n]=y[2n+1]-y[2n],0£n£-1.Sincex[n]andy[n]arereal,symmetricsequences,it02followsthatx0[n]andy0[n]arereal,symmetricsequences,andx1[n]andy1[n]arereal,anti-symmetricsequences.Nowconsider,the(N/2)-lengthsequenceu[n]=x[n]+y[n]+j(x[n]+y[n]).Itsconjugatesequenceisgivenby0110u*[n]=x[n]+y[n]-j(x[n]+y[n]).Nextweobservethat0110u[<–n>]=x[<–n>]+y[<–n>]+j(x[<–n>]+y[<–n>])N/20N/21N/21N/20N/2=x[n]-y[n]+j(-x[n]+y[n]).Itsconjugatesequenceisgivenby0110u*[<-n>]=x[n]-y[n]-j(-x[n]+y[n]).N/20110Byaddingthelast4sequencesweget4x[n]=u[n]+u*[n]+u[<-n>]+u*[<-n>].0N/2N/2FromTable3.6,ifU[k]=DFT{u[n]},thenU*[<-k>]=DFT{u*[n]},N/2U*[k]=DFT{u*[<-n>]},andU[<-k>]=DFT{u[<-n>]}.Thus,N/2N/2N/21X[k]=DFT{x[n]}=(U[k]+U*[<-k>]+U[<-k>]+U*[k]).Similarly,004N/2N/2j4x[n]=u[n]-u*[n]-u[<-n>]+u*[<-n>].Hence,1N/2N/21X[k]=DFT{x[n]}=(U[k]-U*[<-k>]-U[<-k>]+U*[k]).Likewise,114jN/2N/24y[n]=u[n]-u[<-n>]+u*[n]-u*[<-n>].Thus,1N/2N/21Y[k]=DFT{y[n]}=(U[k]-U[<-k>]+U*[<-k>]-U*[k]).Finally,114N/2N/2j4y[n]=u[n]+u[<-n>]-u*[n]-u*[<-n>].Hence,0N/2N/21Y[k]=DFT{y[n]}=(U[k]+U[<-k>]-U*[<-k>]-U*[k]).004jN/2N/274
11N3.80g[n]=(x[2n]+x[2n+1]),h[n]=(x[2n]–x[2n+1]),0£n£–1.Solvingforx[2n]and222Nx[2n+1],wegetx[2n]=g[n]+h[n]andx[2n+1]=g[n]–h[n],0£n£–1.Therefore,2NN-1-1N-122–n–n-1–nX(z)=åx[n]z=åx[2n]z+zåx[2n+1]zn=0n=0n=0NNNN-1-1-1-12222–n-1–n-1–n-1–n=å(g[n]+h[n])z+zå(g[n]+h[n])z=(1+z)åg[n]z+(1-z)åh[n]z.n=0n=0n=0n=0–k–kHence,X[k]=X(z)k=(1+W)G[]+(1-W)H[],0£k£N–1.z=WNNN/2NN/23.81g[n]=ax[2n]+ax[2n+1]andh[n]=ax[2n]+ax[2n+1],withaa¹aa.Solvingforx[2n]12341423ag[n]-ah[n]-ag[n]+ah[n]4231andx[2n+1],wegetx[2n]=,andx[2n+1]=.Thereforeaa-aaaa-aa14231423NN-1-1N-122–n–n-1–nX(z)=åx[n]z=åx[2n]z+zåx[2n+1]zn=0n=0n=0NN-1-12æag[n]-ah[n]ö2æ-ag[n]+ah[n]ö=åç42÷z–n+z-1åç31÷z–nçèaa-aa3÷øçèaa-aa3÷øn=0142n=0142NN-1-1221-1–n-1–n=a-a(a4-a3z)åg[n]z+(-a2+a1z)åh[n]z.Hence,1a42a3n=0n=01-nk-nkX[k]=(a-aW)G[]+(-a+aW)G[],0£k£N–1.a1a4-a2a343NN/221NN/2N-1æ2p(n+a)(k+b)ö3.82XGDFT[k,a,b]=åx[n]expçè-j÷ø.Nn=0N-11æ2p(n+a)(k+b)öx[n]=åX[k,a,b]expçj÷NèNøk=0N-1N-11æ2p(r+a)(k+b)öæ2p(n+a)(k+b)ö=ååx[r]expç-j÷expçj÷NèNøèNøk=0r=0N-1N-1N-1N-11æ2p(n+a-r-a)(k+b)ö1æ2p(n-r)(k+b)ö=ååx[r]expçj÷=ååx[r]expçj÷NèNøNèNøk=0r=0k=0r=0N-1N-11æ2p(n-r)(k+b)ö1=åx[r]åexpçj÷=×x[n]×N=x[n],NèNøNr=0k=075
N-1æ2p(n-r)(k+b)öN,ifn=r,asfromEq.(3.28),åexpçèjN÷ø={0,otherwise.k=0¥¥-n-n3.83X(z)=åx[n]z=åx[n]z.Therefore,n=-¥n=0¥¥-n-nlimX(z)=limåx[n]z=limx[0]+limåx[n]z=x[0].z®¥z®¥z®¥z®¥n=0n=12(z+0.4)(z-0.91)(z+0.3z+0.4)2.043113.84G(z)=.G(z)haspolesatz=0.3±jandz=±j.(z2-0.6z+0.6)(z2+3z+5)222Hence,therearepossibleROCs.(i)¬1:z£0.6.Theinversez-transformg[n]inthiscaseisaleft-sidedsequence.(ii)¬2:0.6£z£5.Theinversez-transformg[n]inthiscaseisatwo-sidedsequence.(iii)¬3:z³5.Theinversez-transformg[n]inthiscaseisaright-sidedsequence.n3.85(a)(i)x[n]=(0.4)m[n]isaright-sidedsequence.Hence,theROCofitsz-transformis1¥¥-nn-n1exteriortoacircle.Thus,X1(z)=åx1[n]z=å(0.4)z=-1,z>0.41-0.4zn=-¥n=0TheROCofX1(z)isgivenby¬1:z>0.4.n(ii)x[n]=(-0.6)m[n]isaright-sidedsequence.Hence,theROCofitsz-transformisexteriorto2¥¥-nn-n1acircle.Thus,X2(z)=åx2[n]z=å(-0.6)z=-1,z>0.61+0.6zn=-¥n=0TheROCofX2(z)isgivenby¬2:z>0.6.n(iii)x[n]=(0.3)m[n-4]isaright-sidedsequence.Hence,theROCofitsz-transformis3¥¥-nn-nexteriortoacircle.Thus,X3(z)=åx3[n]z=å(0.3)zn=-¥n=4¥¥4-4-nn-n(0.3)zX3(z)=åx3[n]z=å(0.3)z=-1,z>0.3.TheROCofX3(z)isgivenby1-0.3zn=-¥n=4¬3:z>0.2.76
n(iv)x[n]=(-0.3)m[-n-2]isaleft-sidedsequence.Hence,theROCofitsz-transformis4interiortoacircle.Thus,¥-2¥-1-nn-n-mm2+azX4(z)=åx4[n]z=å(-0.3)z=å(-0.3)z=--1,z<0.3.1+0.3zn=-¥n=–¥m=2TheROCofX4(z)isgivenby¬4:z<0.3.(b)(i)Now,theROCofX1(z)isgivenby¬1:z>0.4andtheROCofX2(z)isgivenby¬2:z>0.6.Hence,theROCofY1(z)isgivenby¬1Ǭ2=¬2:z>0.6(ii)TheROCofY2(z)isgivenby¬1Ǭ3=¬1:z>0.4.(iii)TheROCofY3(z)isgivenby¬1Ǭ4=Æ.Hence,thez-transformofthesequencey3[n]doesnotconvergeanywhereinthez-plane.(iv)TheROCofY4(z)isgivenby¬2Ǭ3=¬2:z>0.6.(v)TheROCofY5(z)isgivenby¬2Ǭ4=Æ.Hence,thez-transformofthesequencey5[n]doesnotconvergeanywhereinthez-plane.(vi)TheROCofY6(z)isgivenby¬3Ǭ4=Æ.Hence,thez-transformofthesequencey6[n]doesnotconvergeanywhereinthez-plane.¥-n3.86(a)Z{d[n]}=åd[n]z=d[0]=1,whichconvergeseverywhereinthez-plane.n=-¥¥¥nn-n-1n1(b)Z{am[n]}=åam[n]z=å(az)=-1,"z>a1-azn=-¥n=0(c)SeeExample3.29.nnrjwn-jwn(d)x[n]=rsin(w0n)m[n]=(e0-e0)m[n].Usingtheresultsof(iii)andthe2jlinearitypropertyofthez-transformweobtainn1æ1ö1æ1öZ{rsin(w0n)m[n]}=2jç1-rejw-1÷-ç-jw-1÷è0zø2jè1-re0zørjw-jw-1(e0-e0)z-12jrsin(w0)z==,"z>r1-rz-1(ejw0+e-jw0)+r2z-21-2rcos(w)z-1+r2z-20nnnn3.87(a)x1[n]=6[(0.5)-(0.3)]m[n]=6(0.5)m[n]-6(0.3)m[n].Therefore,-1-1-1666(1-0.3z-1+0.5z)1.2zX(z)=-==,z>0.511-0.5z-11-0.3z-1(1-0.5z-1)(1-0.3z-1)(1-0.5z-1)(1-0.3z-1)77
nnn-6(b)x[n]=-6(0.3)m[n]-6(0.5)m[-n-1].Now,Z{-6(0.3)m[n]}=,z>0.3and21-0.3z-1n6Z{-6(0.5)m[-n-1]}=,z<0.5.Therefore,1-0.5z-1-1661.2zX(z)=-=,0.3a.Notethatx[n]isaright-sidedsequence.Hence,the11n1ROCofitsz-transformisexteriortoacircle.Now,Z{am[n]}=,withROCgiven1-az-1n1byz>aandZ{bm[n]}=,withROCgivenbyz>b.Hence,1-bz-1-1-2111-(a+b)z+zX(z)=+=,z>b11-az-11-bz-1(1-az-1)(1-bz-1)nn(b)x[n]=am[-n-1]+bm[n].Notethatx[n]isatwo-sidedsequence.Now,22n1n1Z{am[-n-1]}=,withROCgivenbyzb.Sincetheregionszbdonotintersect,thez-transformofx[n]2doesnotconverge.nnn1(c)x[n]=am[n]+bm[-n-1].Note,isatwo-sidedsequence.Now,Z{am[n]}=,31-az-1n1withROCgivenbyz>aandZ{bm[-n-1]}=,withROCgivenbyzaandza.TheROCofX1(z)includestheunitcirclesincea<1.aaz(1-az)n=0jw1OntheunitcircleX1(e)=X1(z)z=ejw=ae-jw(1-ae-jw),whichisthesameastheDTFTofx[n].1¥¥nn-nn-n(b)x2[n]=n×am[n],a<1.Therefore,X2(z)=ån×am[n]z=ån×azn=-¥n=0-1az=,z>a.TheROCofX(z)includestheunitcirclesincea<1.Ontheunitcircle,(1-az-1)22-jwjwaeX2(e)=X2(z)z=ejw=(1-ae-jw)2,whichisthesameastheDTFTofx2[n].81
-1Mìan,na.TheROCofX(z)includestheunitcirclesincea<1.411-az-143-j3wjwaeOntheunitcircle,X(e)=,whichisthesameastheDTFTofx[n].31-ae-jw4n(e)x[n]=n×am[n+2],a<1.Therefore,5¥¥-1n-n-22-1n-n-2-1azX5(z)=ån×az=-2az-az+ån×az=-z(2az+a)+-12,z>a.(1-az)n=-2n=0TheROCofX(z)includestheunitcirclesincea<1.Ontheunitcircle,5-jwjwjw-2jw-1aeX(e)=-e(2ae+a)+,whichisthesameastheDTFTofx[n].5(1-ae-jw)25n(f)x[n]=am[-n-1],a>1.Therefore,6-10n-nn-n1X6(z)=åaz=åaz-a=-1-a,z1.Ontheunitcircle,X(e)=-a,whichisthesameastheDTFTof61-a-1ejwx[n].6ìï1,–N£n£N,N-(2N+1)-nN(1-z)3.95(a)y1[n]=íTherefore,Y1(z)=åz=z-1.Sincey1[n]isaîï0,otherwise.n=-N(1-z)finite-lengthsequence,theROCofitsz-transformisthewholez-planeexceptpossiblytheorigin,andthereforeincludestheunitcircle.Ontheunitcircle,N1-jw(2N+1))sin(w[N+2])jw-jwnjwN(1-eY1(e)=åe=e-jw=,whichisthesameDTFTofy1[n].(1-e)sin(w/2)n=-N82
ìnï1-,-N£n£N,(b)y[n]=íNNowy2[n]=y0[n]*y0[n]where2ïî0,otherwise.ìï1,-N/2£n£N/2,-(N+1))22N(1-zy[n]=íTherefore,Y(z)=Y(z)=z.Sincey[n]isa00,otherwise.20(1-z-1)22îïfinite-lengthsequence,theROCofitsz-transformisthewholez-planeexceptpossiblytheorigin,andthereforeincludestheunitcircle.Ontheunitcircle,2æéN+1ùösinçwêú÷jw2jwèë2ûøY(e)=Y(e)=whichisthesameDTFTofy[n].20sin2(w/2)2ìïcos(pn/2N),-N£n£N,(c)y[n]=íTherefore,3îï0,otherwise.NN1-j(pn/2N)-n1j(pn/2N)-nY3(z)=åez+åez22n=-Nn=-Nej(p/2)zNæ1-e-j(2N+1)(p/2N)z-(2N+1)öe-j(p/2)zNæ1-ej(2N+1)(p/2N)z-(2N+1)ö=ç÷+ç÷.Since2çè1-e-j(p/2N)z-1÷ø2çè1-ej(p/2N)z-1÷øy[n]isafinite-lengthsequence,theROCofitsz-transformisthewholez-planeexceptpossibly3theorigin,andthereforeincludestheunitcircle.Ontheunitcircle,p1p1jw1sin((w-2N)(N+2))1sin((w+2N)(N+2))Y(e)=+whichisthesameDTFTofy[n].2p2p3sin((w-2N)/2)sin((w+2N)/2)n3.96(a)x[n]=-am[-n-1].Note,isaleft-sidedsequence.Hence,theROCofitsz-transformis1¥-1¥n-nn-n-mminteriortoacircle.Therefore,X1z)=-åam[-n-1]z=-åaz=-åazn=-¥n=-¥m=1¥æz/aözm=-å(z/a)=-ç÷=,z/a<1.TheROCofX1(z)isthusgivenbyza.83
n(c)x[n]=am[-n].Note,isaleft-sidedsequence.Hence,theROCofitsz-transformis3¥0¥n-nn-n-mminteriortoacircle.Therefore,X3(z)=åam[-n]z=åaz=åazn=-¥n=-¥m=01-1=,az<1.Therefore,theROCofX(z)isgivenbyza(seeTable3.8)and1-az-1-1¥¥-n-n-nmmmm1-azZ{am[-n-1]}=åaz=åaz=åaz-1=-1=-1,az<1.1-aza-zn=-¥m=1m=0-11-azz(-1)Therefore,V(z)=+=,withtheROCofV(z)givenby1-az-1a-z-1(1-az-1)(a-z-1)a1.Since,theROCisexteriortoacircle,theinverse1+z1+(1/3)zz-transformy[n]ofY(z)isaright-sidedsequenceandisgivenby11nny1[n]=3(-1)m[n]-2(-1/3)m[n].3-21(b)Y2(z)=-1+-1,z<.Since,theROCisinteriortoacircle,theinverse1+z1+(1/3)z3z-transformy[n]ofY(z)isaleft-sidedsequenceandisgivenby22nny2[n]=-3(-1)m[-n-1]+2(-1/3)m[-n-1].3-21(c)Y3(z)=-1+-1,3,(z+2)(z-3)è(1+2z)(1-3z)ø(1+2z)(1-3z)84
-1-24-3z+3zLetG(z)=.Apartial-fractionexpansionofG(z)yields(1+2z-1)(1-3z-1)2-1-2ABC4-3z+3z25/4G(z)=++,whereA===1,1+2z-11-3z-1(1-3z-1)2(1-3z-1)2-125/4z=-1/2-1-24-3z+3z10/3C===2,and1+2z-1-15/3z=1/31dé4-3z-1+3z-2ù-25/3B=×êú==1.(2-1)!(-3)2-1dz-1ê1+2z-1ú-25/3ëûz1/3æöæöæö-31-31-32ThereforeX(z)=zç÷+zç÷+zç÷.SincetheROCisz>3,açè1+2z-1÷øçè1-3z-1÷øçè(1-3z-1)2÷øtheinversez-transformx[n]ofX(z)isaright-sidedsequence.aaæöæö-11n-11nThus,Zç÷=(-2)m[n],Zç÷=(3)m[n],andçè1+2z-1÷øçè1-3z-1÷øæ-1ö-13znZç-12÷=n(3)m[n].Thus,è(1-3z)øn-3n-32n-2x[n]=(-2)m[n-3]+(3)m[n-3]+(n-2)(3)m[n-2].a3æöæöæö-31-31-32(b)X(z)=zç÷+zç÷+zç÷,z<2.HeretheROCisz<2.bçè1+2z-1÷øçè1-3z-1÷øçè(1-3z-1)2÷øHence,theinversez-transformx[n]ofX(z)isaright-sidedsequence.Thus,bbæöæö-11n-11nZç÷=-(-2)m[-n-1],Zç÷=-(3)m[-n-1],andçè1+2z-1÷øçè1-3z-1÷øæ-1ö-13znZç-12÷=-n(3)m[-n-1].Therefore,è(1-3z)øn-3n-32n-2xb[n]=-(-2)m[-n-4]-(3)m[-n-4]-n(3)m[-n-3].3æöæöæö-31-31-32(c)X(z)=zç÷+zç÷+zç÷,22,whereas,Zç÷andçè1+2z-1÷øçè1-3z-1÷øæ-1öæöZ-1ç2z÷areright-sidedsequencesasz<3.Thus,Z-1ç1÷=(-2)nm[n],çè(1-3z-1)2÷øçè1+2z-1÷ø85
æöæ-1ö-11n-13znZçç1-3z-1÷÷=-(3)m[-n-1],andZç-12÷=-n(3)m[-n-1].Therefore,èøè(1-3z)øn-3n-32n-2xc[n]=(-2)m[n-3]-(3)m[-n-4]-n(3)m[-n-3].3P(z)P(z)3.100G(z)==.Bydefinitiontheresidueratthepolez=lisgivenbyD(z)(1-lz-1)R(z)lll-1P(z)dD(z)d[(1-llz)R(z)]-1dR(z)r=.Now,D"(z)===-lR(z)+(1-lz).Hence,lR(z)dz-1dz-1lldzz=llP(z)D"(z)=-lR(z).Therefore,r=-l.z=lllz=llllD"(z)z=llP(z)p+pz-1+L+pz-Mp01M03.101G(z)==.Thus,G(¥)=.Nowapartial-fractionexpansionD(z)d+dz-1+L+dz-Md01M0NNr-1lofG(z)inzisgivenbyG(z)=å-1,fromwhichweobtainG(¥)=årl.Therefore,1-lzl=1ll=1Np0G(¥)=årl=.dl=1013.102H(z)=,z>r>0.Byusingpartial-fractionexpansionwewrite1-2rcos(q)z-1+r2z-21ìïejqe-jqüï1ìïejqe-jqüïH(z)=í-ý=í-ý.Thus,(ejq-e-jq)îï1-rejqz-11-re-jqz-1þï2jsin(q)îï1-rejqz-11-re-jqz-1þï1rnìïejq(n+1)-e-jq(n+1)üïjqnjnqn-jq-jnqh[n]={erem[n]-reem[n]}=íým[n]2jsin(q)sin(q)îï2jþïrnsin((n+1)q)=m[n].sin(q)¥-n3.103G(z)=åg[n]zwithaROCgivenbyRg.n=-¥¥¥-n-n(a)ThereforeG*(z)=åg*[n](z*)andG*(z*)=åg*[n]z.n=-¥n=-¥Thusthez-transformofg*[n]isG*(z*).¥m(b)Replacenby–minthesummation.ThisleadstoG(z)=åg[-m]z.Thereforem=-¥86
¥-mG(1/z)=åg[-m]z.Thusthez-transformofg[–n]isG(1/z).Notethatsincezhasbeenm=-¥replacedby1/z,theROCofG(1/z)willbe1/Rg.(c)Lety[n]=ag[n]+bh[n].Then,¥¥¥-n-n-nY(z)=å(ag[n]+bh[n])z=aåg[n]z+båh[n]z=aG(z)+bH(z)n=-¥n=-¥n=-¥InthiscaseY(z)willconvergewhereverbothG(z)andH(z)converge.ThustheROCofY(z)isRgÇRh,whereisRgtheROCofG(z)andRhistheROCofH(z).¥¥¥-n-n-(m+n)(d)y[n]=g[n-n0].HenceY(z)=åy[n]z=åg[n-n]z=åg[m]z00n=-¥n=-¥m=-¥¥-n-m-n=z0åg[m]z=z0G(z).m=-¥InthiscasetheROCofY(z)isthesameasthatofG(z)exceptforthepossibleadditionor-neliminationofthepointz=0orz=¥(duetothefactorz0).¥¥n-n-1-n(e)y[n]=ag[n].Hence,Y(z)=åy[n]z=åg[n](za)=G(z/a).n=-¥n=-¥TheROCofY(z)isaR.g¥-n(f)y[n]=ng[n].HenceY(z)=ång[n]z.n=-¥¥¥¥-ndG(z)-n-1dG(z)-nNowG(z)=åg[n]z.Thus,=-ång[n]zÞz=-ång[n]z.dzdzn=-¥n=-¥n=-¥dG(z)ThusY(z)=–z.dz¥(g)y[n]=g[n]*h[n]=åg[k]h[n-k].Hence,k=-¥¥¥æ¥ö¥¥Y(z)=åy[n]z-n=åçåg[k]h[n-k]÷z-n=åg[k]åh[n-k]z-nç÷n=-¥n=-¥èk=-¥øk=-¥n=-¥¥-k=åg[k]H(z)z=H(z)G(z).k=-¥InthiscasealsoY(z)willconvergewhereverbothH(z)andG(z)converge.ThusROCofY(z)isRgÇRh.¥-n(h)y[n]=g[n]h[n].Hence,Y(z)=åg[n]h[n]z.FromEq.(3.107),n=-¥87
¥æö1n-1ç1n-1÷-ng[n]=2pjòG(v)vdv.Thus,Y(z)=åh[n]ç2pjòG(v)vdv÷zç÷Cn=-¥èCøæ¥ö1ç-nn-1÷1-1=2pjòG(v)çåh[n]zv÷dv=2pjòG(v)H(z/v)vdv.Cèn=-¥øC¥¥1n-11-1(i)åg[n]h*[n]=2pjòG(v)åh*[n]vvdv=2pjòG(v)H*(1/v*)vdv.n=-¥Cn=-¥C113.104x[n]=x[n]+jx[n],wherex[n]=(x[n]+x*[n]),andx[n]=(x[n]-x*[n]).Fromreimre2im2j1Table3.9,Z{x*[n]}=X*(z*),withanROCRx.Therefore,Z{xre[n]}={X(z)+X*(z*)},and21Z{x[n]}={X(z)-X*(z*)}.im2j¥1-3n3.105(a)ExpnadinginapowerserieswegetX1(z)=-3=åz,z>1.1-zn=01,ifn=3kandn³0,Thus,x1[n]=Usingpartialfraction,weget{0,elsewhere.1111333X(z)==++.Therefore,11-z-31-z-11+(1+j3)z-11+(1-j3)z-1222211æ13ön1æ13önx1[n]=m[n]+ç--j÷m[n]+ç-+j÷m[n]33è22ø3è22ø11-j2pn/31j2pn/312=m[n]+em[n]+em[n]=m[n]+cos(2pn/3)m[n].333331,ifn=3kandn³0,Thusx1[n]={0,elsewhere.¥1-2n(b)ExpnadinginapowerserieswegetX2(z)=-2=åz,z>1.1-zn=0ì1,ifn=2kandn³0,Thus,x2[n]=íî0,elsewhere.11122Usingpartialfraction,wegetX(z)==+.Therefore,21-z-21+z-11-z-111nx2[n]=m[n]+(-1)m[n]22ì1,ifn=2kandn³0,Thus,x2[n]=íî0,elsewhere.-1-13.106(a)X1(z)=log(1-az),z>a.Expandinglog(1-az)inapowerseriesweget88
2-23-3¥n-1azaza-nX1(z)=-az---.....=-åz.23nn=1naTherefore,,x[n]=-m[n-1].1næa-z-1ö(b)X(z)=logç÷=log(1-(az)-1),za.ExpandingX3(z)inapowerserieswegetè1-azø2-23-3¥n-1azaza-nX1(z)=az+++.....=åz.23nn=1naTherefore,x[n]=m[n-1].3næaö-1(d)X4(z)=logç-1÷=-log(1-(az)),zl³0FromEq.(3.177),forN=4,wegeté1z-1z-2z-3ùê000úê1z-1z-2z-3úD=ê1-11-21-3ú.ThedeterminantofD4isgivenby4ê1zzzú222ê1z-1z-2z-3úêë333úû1z-1z-2z-31z-1z-2z-30000001z-1z-2z-30z-1-z-1z-2-z-2z-3-z-3det(D)=1-11-21-3=1-10-11-20-21-30-341zzz0z-zz-zz-z2222020201z-1z-2z-30z-1-z-1z-2-z-2z-3-z-3333303030z-1-z-1z-2-z-2z-3-z-31z-1+z-1z-2+z-1z-1+z-2101010101100-1-1-2-2-3-3-1-1-1-1-1-1-1-1-2-1-1-2=z2-z0z1-z0z2-z0=(z1-z0)(z2-z0)(z3-z0)1z2+z0z2+z2z0+z0z-1-z-1z-2-z-2z-3-z-31z-1+z-1z-2+z-1z-1+z-23010303033001z-1+z-1z-2+z-1z-1+z-2101100-1-1-1-1-1-1-1-1-1-1-1-2-1=(z1-z0)(z2-z0)(z3-z0)0z2-z1(z2-z1)(z2+z1+z0)0z-1-z-1(z-1-z-1)(z-1+z-2+z-1)3131310z-1-z-1(z-1-z-1)(z-1+z-1+z-1)=(z-1-z-1)-1-z-1-1-z-1212121010(z20)(z30)z-1-z-1(z-1-z-1)(z-1+z-1+z-1)31313101z-1+z-1+z-1=(z-1-z-1)-1-z-1-1-z-1-1-z-1-1-z-121010(z20)(z30)(z21)(z31)1z-1+z-1+z-1310-1-1-1-1-1-1-1-1-1-1-1-1-1-1=(z1-z0)(z2-z0)(z3-z0)(z2-z1)(z3-z1)(z3-z2)=Õ(zk-zl).3³k>l³0-1-1Hence,inthegeneralcase,det(DN)=Õ(zk-zl).ItfollowsfromthisexpressionN-1³k>l³0thatthedeterminantisnon-zero,i.e.DNisnon-singular,ifthesamplingpointszkaredistinct.90
3.110XNDFT[0]=X(z0)=4+4+12-8=12,XNDFT[1]=X(z1)=4-2+3+1=6,X[2]=X(z)=4-4+12+8=20,X[3]=X(z)=4-4+12+8=52.NDFT2NDFT3-11-11-111-1-21-31I0(z)=(1-z)(1-2z)(1-3z)=1-z+z-z.Thus,I0(-2)=10,661-11-11-11-11-21-31I1(z)=(1+z)(1-z)(1-z)=1-z-z+z.Thus,I1(1)=,223341221-1-11-15-11-21-32I2(z)=(1+z)(1-z)(1-z)=1-z-z+z.Thus,I2(2)=-and2363631-1-11-1-11-21-3æ1ö5I3(z)=(1+2z)(1-z)(1-2z)=1-z-4z+4z.Thus,I3è3ø=2.Therefore,1262052-1-2-3X(z)=I0(z)+I1(z)-I2(z)+I3(z)=4-2z+3z+z.101/22/35/2ìx[n],0£n£N-1,3.111x[n]=íy[n]=xe[n]+xe[2N–1–n].Therefore,eî0,N£n£2N-1.2N-1N-12N-1nknknkY[k]=åy[n]W2N=åx[n]W2N+åx[2N-1-n]W2Nn=0n=0n=NN-1N-1N-1nk(2N-1-n)knk-k-nk=åx[n]W2N+åx[n]W2N=åx[n](W2N+W2NW2N).n=0n=0n=0N-1k/2k(n+1/2)-k(n+1/2)Thus,Cx[k]=W2NY[k]=åx[n](W2N+W2N)n=0N-1æp(2n+1)kö=å2x[n]cosç÷,0£k£N-1.è2Nøn=0ìW-k/2C[k],0£k£N-1,ï2Nx3.112Y[k]=í0,k=N,Thus,ï-W-k/2C[2N-k],N+1£k£2N-1.î2Nx2N-1N-12N-11-nk1-(n+1/2)k1-(n+1/2)ky[n]=åY[k]W2N=åCx[k]W2N-åCx[2N-k]W2N2N2N2Nk=0k=0k=N+1N-1N-11-(n+1/2)k1-(n+1/2)(2N-k)=åCx[k]W2N-åCx[k]W2N2N2Nk=0k=1N-1N-11-(n+1/2)k1(n+1/2)k=åCx[k]W2N+åCx[k]W2N2N2Nk=0k=1N-1Cx[0]1æpk(2n+1)ö=+åCx[k]cosç÷,2NNè2Nøk=1N-1Cx[0]1æpk(2n+1)öì1/2,k=0,Hence,+åCx[k]cosç÷,wherew[k]=í1,1£k£N-1.2NNè2Nøîk=191
ìN-1ìy[n],0£n£N-1,ï1æpk(2n+1)öMoreover,x[n]=í=íåw[k]Cx[k]cosçè÷ø,0£n£N-1,î0,elsewhere,ïNk=02Nïî0,elsewhere.ìN-1ï1(2n+1)pk(b)FromEq.(3.163),x[n]=íNåw[k]Cx[k]cos(2N),0£n£N-1,Hence,ïk=0ïî0,elsewhere.N-1N-1N-1æ(2n+1)pmö1(2n+1)pk(2n+1)pmå2x[n]cosçè÷ø=ååw[k]Cx[k]cos(2N)cos(2N)2NNm=0k=0m=0N-1N-11(2n+1)pk(2n+1)pm=åw[k]Cx[k]åcos(2N)cos(2N).(10)Nk=0m=0N-1æöæöìN,ifk=m=0,(2n+1)pk(2n+1)pmïNow,åcosç÷cosç÷=íN/2,ifk=m,m=0è2Nøè2Nøï0,elsewhere.îThus,Eq.(10)reducestoN-1ì1w[0]C[0]×N,m=0,æ(2n+1)pmöïNxå2x[n]cosç÷=í1è2Nøïw[m]C[m]×N,1£m£N-1,m=0îNxìC[0],m=0,=íx=Cx[m],0£m£N-1.îCx[m],1£m£N-1,3.113y[n]=ag[n]+bh[n].Thus,N-1N-1æpk(2n+1)öæpk(2n+1)öCy[k]=åy[n]cosç÷=å(ag[n]+bh[n])cosç÷=è2Nøè2Nøn=0n=0N-1N-1æpk(2n+1)öæpk(2n+1)ö=aåg[n]cosçè÷ø+båh[n]cosçè÷ø=aCg[k]+bCh[k].2N2Nn=0n=0N-1N-1æpk(2n+1)ö*æpk(2n+1)ö3.114Cx[k]=åx[n]cosçè÷øÞCx[k]=åx*[n]cosçè÷ø.2N2Nn=0n=0*ThustheDCTcoefficientsofx*[n]aregivenbyC[k].xN-1ìN,ifk=m=0,æpk(2n+1)öæpm(2n+1)öï3.115Notethatåcosç÷cosç÷=íN/2,ifk=mandk¹0,è2Nøè2Nøïn=0î0,otherwise.N-1N-11*æp(2n+1)köæp(2n+1)möNow,x[n]x*[n]=2ååa[k]a[m]Cx[m]Cx[k]cosçè÷øcosçè÷øN2N2Nk=0m=0N-1N-1N-1N-121*æp(2n+1)köæp(2n+1)möThus,åx[n]=2ååa[k]a[m]Cx[m]Cx[k]åcosçè÷øcosçè÷øN2N2Nn=0k=0m=0n=092
N-1N-1212Now,usingtheorthogonalitypropertymentionedaboveåx[n]=åa[k]Cx[k].2Nn=0k=0N-1ææ2pnköæ2pnköö3.116XDHT[k]=åx[n]çcosçè÷ø+sinçè÷ø÷.Now,èNNøn=0ææ2pnköæ2pmkööXDHT[k]çcosç÷+sinç÷÷èèNøèNøøN-1ææ2pnköæ2pnkööææ2pmköæ2pmköö=åx[n]çcosç÷+sinç÷÷çcosç÷+sinç÷÷.Therefore,èèNøèNøøèèNøèNøøn=0N-1ææ2pmköæ2pmkööåXDHT[k]çcosçè÷ø+sinçè÷ø÷èNNøk=0N-1N-1ææ2pnköæ2pnkööææ2pmköæ2pmköö=åx[n]åçcosç÷+sinç÷÷çcosç÷+sinç÷÷èèNøèNøøèèNøèNøøn=0k=0ìN,ifm=n=0,N-1æöæöïï2pnk2pmkN/2,ifm=n¹0,Itcanbeshownthatåcosç÷cosç÷=íN/2,ifm=N-n,èNøèNøïk=0ïî0,elsewhere,N-1ìN/2,ifm=n¹0,æ2pnköæ2pmköïåsinç÷sinç÷=í-N/2,ifm=N-n,andèNøèNøïk=0î0,elsewhere,N-1N-1æ2pnköæ2pmköæ2pnköæ2pmköåsinç÷cosç÷=åcosç÷sinç÷=0.èNøèNøèNøèNøk=0k=0N-11ææ2pmköæ2pmkööHence,x[m]=åXDHT[k]çcosçè÷ø+sinçè÷ø÷.NèNNøk=0ìx[n-n+N],0£n£n-1,3.117(a)y[n]=x()=í000Nîx[n-n0],n0£n£N-1.N-1n0-1ææ2pnköæ2pnkööææ2pnköæ2pnkööYDHT[k]=åy[n]çcosçè÷ø+sinçè÷ø÷=åx[n-n0+N]çcosçè÷ø+sinçè÷ø÷èNNøèNNøn=0n=0N-1ææ2pnköæ2pnköö+åx[n-n0]çcosçè÷ø+sinçè÷ø÷.èNNøn=n0Replacingn–n0+Nbyninthefirstsumandn–n0byninthesecondsumwegetN-1ææ2p(n+n)köæ2p(n+n)kööY[k]=åx[n]çcosç0÷+sinç0÷÷DHTçèèNøèNø÷øn=N-n0n-10ææ2p(n+n)köæ2p(n+n)köö+åx[n]çcosç0÷+sinç0÷÷çèèNøèNø÷øn=093
N-1ææ2p(n+n)köæ2p(n+n)köö=åx[n]çcosç0÷+sinç0÷÷çèèNøèNø÷øn=0æ2pnköN-1ææ2pnköæ2pnköö0=cosç÷åx[n]çcosç÷+sinç÷÷èNøèèNøèNøøn=0n-1æ2pnkö0ææ2pnköæ2pnköö0+sinç÷åx[n]çcosç÷-sinç÷÷èNøèèNøèNøøn=0æ2pnköæ2pnkö00=cosç÷X[k]+sinç÷X[-k].èNøDHTèNøDHT(b)TheN-pointDHTofx[<–n>N]isXDHT[–k].N-1N-1N-121(c)åx[n]=2ååXDHT[k]XDHT[l]´Nn=0k=0l=0æN-1öçææ2pnköæ2pnkööææ2pnlöæ2pnlöö÷çåçècosçèN÷ø+sinçèN÷ø÷øçècosçèN÷ø+sinçèN÷ø÷ø÷.èn=0øUsingtheorthogonalityproperty,theproductisnon-zeroifk=landisequaltoN.N-1N-1212Thusåx[n]=åXDHT[k].Nn=0k=0æ2pnkö1nk-nkæ2pnkö1nk-nk3.118cosçè÷ø=(WN+WN),andsinçè÷ø=(WN-WN).N2N2jN-1æj2pnk/N-j2pnk/Nj2pnk/N-j2pnk/Nöe+ee-eXDHT[k]=åx[n]çç+÷÷è22jøn=01ThereforeXDHT[k]=(X[N-k]+X[k]-jX[N-k]+jX[k]).2N-1ææ2pnköæ2pnköö3.119y[n]=x[n]g[n]N.Thus,YDHT[k]=åy[n]çcosçè÷ø+sinçè÷ø÷èNNøn=0N-1N-1ææ2pnköæ2pnköö=åx[r]åg[N]çcosçè÷ø+sinçè÷ø÷.èNNør=0n=0FromresultsofProblem3.117N-1ææ2plköæ2plkööYDHT[k]=åx[l]çGDHT[k]cosçè÷ø+GDHT[<-k>N]sinçè÷ø÷èNNøl=0N-1N-1æ2plköæ2plkö=GDHT[k]åx[l]cosçè÷ø+GDHT[<-k>N]åx[l]sinçè÷øNNl=0l=094
1=GDHT[k](XDHT[k]+XDHT[<-k>N])21+GDHT[<-k>N](XDHT[k]-XDHT[<-k>N]).21orY[k]=X[k](G[k]+G[<-k>])DHT2DHTDHTDHTN1+XDHT[<-k>N](GDHT[k]-GDHT[<-k>N]).2é11111111ùê1-11-11-11-1úêúé1111ùê11-1-111-1-1úé11ùê1-11-1úê1-1-111-1-11ú3.120(a)H2=êú,H4=êú,andH8=êú.ë1-1ûê11-1-1úê1111-1-1-1-1úêë1-1-11úûê1-11-1-11-11úêúê11-1-1-1-111úêë1-1-11-111-1úû(b)FromthestructureofH2,H4andH8itcanbeseenthatéHHùéHHùH=ê22ú,andH=ê44ú.4ëH2-H2û8ëH4-H4û-1T*(c)X=Hx.Thereforex=HX=NHX=NHX.Hence,HTNNHTNHTNHTN-1l-1x[n]=åX[k](-1)åbi(n)bi(k).HTi=0k=0whereb(r)istheithbitinthebinaryrepresentationofr.iN-1N-1N-1222-n-nln-nl/2n/2-(l-n)/23.121X(zl)=åx[n]zl=åx[n]AV=åx[n]AVVVn=0n=0n=0N-122-n2/2l/2-nn/2=Våg[n]h[l-n],whereg[n]=x[n]AVandh[n]=V.n=0Ablock-diagramrepresentationforthecomputationofX(z)usingtheaboveschemeisthuslpreciselyFigureP3.6.l-ljq-jlfl3.122z=a.Hence,AVe0e0=a.Sinceaisreal,wehavel00A0=1,V0=1/a,q0=0andf0=0.3.123(i)N=3.-1-2-1-2X(z)=x[0]+x[1]z+x[2]z,andH(z)=h[0]+h[1]z+h[2]z-1-2Y(z)=h[0]x[0]+(h[1]x[0]+x[1]h[0])z+(h[2]x[0]+h[1]x[1]+h[0]x[2])zL95
-3-4+(h[1]x[2]+h[2]x[1])z+h[2]x[2]z.Ontheotherhand,-1Y(z)=(h[0]x[0]+h[1]x[2]+h[2]x[0])+(h[0]x[1]+h[1]x[0]+h[2]x[2])zc-2+(h[0]x[2]+h[1]x[1]+h[2]x[0])z.-3ItiseasytoseethatinthiscaseYc(z)=YL(z)mod(1-z).(ii)N=4.-1-2-3-1-2-3X(z)=x[0]+x[1]z+x[2]z+x[3]zandH(z)=h[0]+h[1]z+h[2]z+h[3]z.-1-2YL(z)=h[0]x[0]+(h[1]x[0]+h[0]x[1])z+(h[0]x[2]+h[1]x[1]+h[2]x[0])z-3-4+(h[0]x[3]+h[1]x[2]+h[2]x[1]+h[3]x[0])z+(h[1]x[3]+h[2]x[2]+h[3]x[1])z-5-6+(h[2]x[3]+h[3]x[2])z+h[3]x[3]z,whereas,Y(z)=(h[0]x[0]+h[1]x[3]+h[2]x[2]+h[3]x[1])c-1+(h[0]x[1]+h[1]x[0]+h[2]x[3]+h[3]x[2])z-2+(h[0]x[2]+h[1]x[1]+h[2]x[0]+h[3]x[3])z-3+(h[0]x[3]+h[1]x[2]+h[2]x[1]+h[3]x[0])z.-4AgainitcanbeseenthatYc(z)=YL(z)mod(1-z).(ii)N=5.-1-2-3-4X(z)=x[0]+x[1]z+x[2]z+x[3]z+x[4]zand-1-2-3-4H(z)=h[0]+h[1]z+h[2]z+h[3]z+h[4]z.-1-2YL(z)=h[0]x[0]+(h[1]x[0]+h[0]x[1])z+(h[0]x[2]+h[1]x[1]+h[2]x[0])z-3+(h[0]x[3]+h[1]x[2]+h[2]x[1]+h[3]x[0])z-4+(h[0]x[4]+h[1]x[3]+h[2]x[2]+h[3]x[1]+h[4]x[0])z-5-6+(h[1]x[4]+h[2]x[3]+h[3]x[2]+h[4]x[1])z+(h[2]x[4]+h[3]x[3]+h[4]x[2])z-7-8+(h[4]x[3]+h[3]x[4])z+h[4]x[4]z,whereas,Yc(z)=(h[0]x[0]+h[4]x[1]+h[3]x[2]+h[2]x[3]+h[1]x[4])-1+(h[0]x[1]+h[1]x[0]+h[2]x[4]+h[3]x[3]+h[4]x[2])z-2+(h[0]x[2]+h[1]x[1]+h[2]x[0]+h[3]x[4]+h[4]x[3])z-3+(h[0]x[3]+h[1]x[2]+h[2]x[1]+h[3]x[0]+h[4]x[4])z-4+(h[0]x[4]+h[1]x[3]+h[2]x[2]+h[3]x[1]+h[4]x[0])z.-5AgainitcanbeseenthatYc(z)=YL(z)mod(1-z).¥-nˆXˆ(z)jwXˆ(ejw)3.124(a)X(z)=åx[n]z.LetX(z)=log(X(z))ÞX(z)=e.Thus,X(e)=en=-¥96
p1jwjwnjw-jw(b)xˆ[n]=òlog(X(e))edw.Ifx[n]isreal,thenX(e)=X*(e).Therefore,2p-pjw-jwlog(X(e))=log(X*(e))..pp1jw-jwn1-jw-jwnxˆ*[n]=òlog(X*(e))edw=òlog(X(e))edw2p2p-p-pp1jwjwn=òlog(X(e))edw=xˆ[n].2p-ppæjwn-jwnöxˆ[n]+xˆ[-n]1jwe+e(c)xˆ[n]==log(X(e))ç÷dwev22pòçè2÷ø-pp1jw=òlog(X(e))cos(wn)dw,2p-ppæjwn-jwnöxˆ[n]-xˆ[-n]jjwe-eandsimilarly,xˆ[n]==log(X(e))ç÷dwod22pòçè2j÷ø-ppjjw=òlog(X(e))sin(wn)dw.2p-p-13.125x[n]=ad[n]+bd[n-1]andX(z)=a+bz.Also,¥nˆ-1-1n-1(b/a)-nX(z)=log(a+bz)=log(a)+log(1+b/az)=log(a)+å(-1)z.Therefore,nn=1ìlog(a),ifn=0,ïïnxˆ[n]=í(-1)n-1(b/a),forn>0,ïnïî0,elsewhere.NaNgNbNdˆ-1-13.126(a)X(z)=log(K)+ålog(1-akz)+ålog(1-gkz)-ålog(1-bkz)-ålog(1-dkz).k=1k=1k=1k=1Na¥nNg¥nNb¥nNd¥nagbdXˆ(z)=log(K)-ååkz-n-ååkzn+ååkz-n+ååkzn.nnnnk=1n=1k=1n=1k=1n=1k=1n=1ìlog(K),n=0,ïNbnNnïbaaïåk-åk,n>0,ïnnThus,xˆ[n]=ín=1n=1ïNg-nN-nïgddïåk-åk,n<0.ïnnîn=1n=197
nr(b)ˆx[n]0,andisthusanti-causal.kk(d)Ifg=d=0thenxˆ[n]=0foralln<0andisthusacausalsequence.kk3.127IfX(z)hasnopolesandzerosontheunitcirclethenfromProblem3.95,g=d=0andkkxˆ[n]=0foralln<0.ˆdXˆ(z)1dX(z)dX(z)dXˆ(z)X(z)=log(X(z))therefore=.Thus,z=zX(z).dzX(z)dzdzdz¥Takingtheinversez-transformwegetnx[n]=åkxˆ[k]x[n-k],n¹0.k=-¥nkSincex[n]=0andxˆ[n]=0forn<0,thusx[n]=åxˆ[k]x[n-k],n¹0.nk=0n-1n-1kx[n]æköxˆ[k]x[n-k]Or,x[n]=åxˆ[k]x[n-k]+xˆ[n]x[0].Hence,xˆ[n]=-åç÷,n¹0.nx[0]ènøx[0]k=0k=0Forn=0,xˆ[0]=Xˆ(z)=X(z)=log(x[0]).Thus,z=¥z=¥ìïïïï0,n<0,xˆ[n]=ílog(x[0]),n=0,ïn-1ïx[n]æköˆx[k]x[n-k]ï-åç÷,n>0.ïîx[0]ènøx[0]k=03.128ThisproblemiseasytosolveusingthemethoddiscussedinSection4.13.2.v[n]x[n]h[n]g[n]y[n]n1n1h[n]=0.6m[n].Thus,H(z)=.g[n]=0.8m[n].Thus,G(z)=.-1-11-0.6z1-0.8z-1FromEq.(4.212)wegetFvv(z)=H(z)H(z)Fxx(z),(A)-1-1-1andFyy(z)=G(z)G(z)Fvv(z)=G(z)G(z)H(z)H(z)Fxx(z).(B)-1-11z1.5625-1.5625Now,H(z)H(z)===+.-1-1-2-1-1(1-0.6z)(1-0.6z)-0.6+1.36z-0.6z1-0.6z1-1.6667zThus,usingEq.(A)weget98
æ1ö-1221Fvv(z)=H(z)H(z)sx=1.5625sxç-1--1÷,0.6X)%Errorconditiondisp("error");end%clearalltemp=ceil(log2(M));%FindlengthofcircularconvolutionN=2^temp;%zeropaddingtheshortersequenceif(N>M)fori=M+1:Nh(i)=0;endendm=ceil((-N/(N-M+1)));while(m*(N-M+1)<=X)if(((N+m*(N-M+1))<=X)&((m*(N-M+1))>0))forn=1:Nx1(n)=x(n+m*(N-M+1));endendif(((m*(N-M+1))<=0)&((N+m*(N-M+1))>=0))%underflowadjustmentforn=1:Nx1(n)=0;endforn=m*(N-M+1):N+m*(N-M+1)if(n>0)x1(n-m*(N-M+1))=x(n);endendend109
if((N+m*(N-M+1))>X)%overflowadjustmentforn=1:Nx1(n)=0;endforn=1:(X-m*(N-M+1))x1(n)=x(m*(N-M+1)+n);endendw1=circonv(h,x1);%circularconvolutionusingDFTfori=1:M-1y1(i)=0;endfori=M:Ny1(i)=w1(i);endforj=M:Nif((j+m*(N-M+1))<(X+M))if((j+m*(N-M+1))>0)yO(j+m*(N-M+1))=y1(j);endendendm=m+1;enddisp("NumberofFloatingPointOperations")flops%disp("ConvolutionusingOverlapSave:");y=real(yO);functiony=circonv(x1,x2)L1=length(x1);L2=length(x2);ifL1~=L2,error("Sequencesofunequallengths"),endX1=fft(x1);X2=fft(x2);X_RES=X1.*X2;y=ifft(X_RES);TheMATLABprogramforperformingconvolutionusingtheoverlap-savemethodish=[111]/3;R=50;d=rand(1,R)-0.5;m=0:1:R-1;s=2*m.*(0.9.^m);x=s+d;%x=[xxxxxxx];y=overlapsave(x,h);k=0:R-1;plot(k,x,"r-",k,y(1:R),"b--");xlabel("Timeindexn");ylabel("Amplitude");legend("r-","s[n]","b--","y[n]");110
8s[n]6y[n]42001020304050TimeindexnM3.16(a)UsingtheM-filerootswedeterminethezerosandthepolesofG(z)whichare1givenbyz=-3.5616,z=-0.4500+j0.7730,z=-0.4500-j0.7730,z=0.5616,and1234p=-1.0000+j1.7321,p=-1.0000-j1.7321,p=0.6000+j0.3742,p=0.6000-j0.3742.1234NextusingtheM-fileconvwedeterminethequadraticfactorscorrespondingtothecomplexconjugatezerosandpolesresultinginthefollowingfactoredformofG(z):1-1-1-1-24(1+3.5616z)(1-0.5616z)(1+0.9z+0.8z)G(z)=×.13(1+2z-1+4z-2)(1-1.2z-1+0.5z-2)(b)UsingtheM-filerootswedeterminethezerosandthepolesofG(z)whichare2givenbyz=-2,z=-1,z=-0.5,z=0.3,and1234p=-1.0482+j1.7021,p=-1.0482-j1.7021,p=-0.6094,p=-0.3942.1234NextusingtheM-fileconvwedeterminethequadraticfactorscorrespondingtothecomplexconjugatezerosandpolesresultinginthefollowingfactoredformofG(z):2-1-1-1-12(1+2z)(1+z)(1+0.5z)(1-0.3z)G(z)=×25(1+0.6094z-1)(1+0.3942z-1)(1+2.0963z-1+3.9957z-2)M3.17UsingProgram3_9wearriveatwegetResidues3.00002.0000Poles-1.0000-0.3333Constant[]32Hence,Y1(z)=-1--1,whichissameasthatdeterminedinProblem3.98.1+z1+1z3-1-2-3-4-54-3z+3z4z-3z+3zM3.18(a)Apartial-fractionexpansionofXa(z)=2=-1-2-3.(z+2)(z-3)1-4z-3z+18zusingProgram3_9wearriveat-2-10.18520.07410.125Xa(z)=0.2361z-0.1389z+0.1667--1+-12--1.SincetheROCis1-3z(1-3z)1+2z111
givenbyz>3,theinversez-transformisaright-sidedsequenceandisthusgivenbyxa[n]=0.2361d[n-2]-0.1389d[n-1]+0.1667d[n]nnn-0.1852(3)m[n]+0.0741n(3)m[n]-0.125(-2)m[n].æ-1+3z-2ö-34-3zAmorecompactsolutionisobtainedbywritingXa(z)asXa(z)=zç-1-2-3÷,è1-4z-3z+18zøandmakingapartial-fractionexpansionofthefunctioninsidethebrackets:æ21öæ1öæ-1ö-31-31-22zXa(z)=zç-1+-12+-1÷=zç-1+-1÷+zç-12÷è1-3z(1-3z)1+2zøè1-3z1+2zøè(1-3z)øTheinversez-trasformoftheabovefunctionisgivenbyn-3n-32n-2xa[n]=(3)m[n-3]+(-2)m[n-3]+(n-2)(3)m[n-2]3n-3n-3=[(3)(2n-3)+(-2)]m[n-3].(b)HeretheROCisz<2,hence,theinversez-transformofæ1öæ-1ö-31-22zXb(z)=zç-1+-1÷+zç-12÷isthusgivenbyè1-3z1+2zøè(1-3z)øn-3n-32n-2xb[n]=-(3)m[-n+2]-(-2)m[-n+2]-(n-2)(3)m[-n+1]3n-3n-3=[-(3)(2n-3)-(-2)]m[-n+2].(c)Inthiscase,theROCisgivenby20.3.Therefore,1+0.2z-11-0.3z-1(1+0.2z-1)(1-0.3z-1)é6-z-12ù1-0.4z-1Y(z)=H(z)X(z)=ê+úêë1+0.5z-11-0.4z-1úû(1+0.2z-1)(1-0.3z-1)é8-2.4z-1+0.4z-2ù1-0.4z-18-2.4z-1+0.4z-2=êú=,z>0.5.êë(1+0.5z-1)(1-0.4z-1)úû(1+0.2z-1)(1-0.3z-1)(1+0.5z-1)(1+0.2z-1)(1-0.3z-1)1581Apartial-fractionexpansionofY(z)yieldsY(z)=-+.Hence,1+0.5z-11+0.2z-11-0.3z-1nnntheinversez-transformofY(z)isgivenbyy[n]=15(-0.5)m[n]-8(-0.2)m[n]+(0.3)m[n].nn4.33(a)h[n]=(0.8)m[n]andx[n]=(0.5)m[n].Theirz-transformsaregivenby11H(z)=,z>0.8andX(z)=,z>0.5.Thez-transformY(z)oftheoutput1-0.8z-11-0.5z-1125
1y[n]isthereforegivenbyY(z)=H(z)X(z)=,z>0.5.Itspartial-fraction(1-0.8z-1)(1-0.5z-1)18/35/3expansionisgivenbyY(z)==-.Hence,theinverse(1-0.8z-1)(1-0.5z-1)1-0.8z-11-0.5z-18n5nz-transformofY(z)isgivenbyy[n]=(0.8)m[n]-(0.5)m[n].33(b)Thez-transformY(z)oftheoutputy[n]isthereforegivenby-110.5zY(z)=H(z)X(z)=,z>0.5.Now,theinversez-transformofis(1-0.5z-1)2(1-0.5z-1)2n0.5givenbyn(0.5)m[n].Thus,theinversez-transformofisgivenby-12(1-0.5z)n+1nn(n+1)(0.5)m[n+1].Hence,y[n]=(n+1)(0.5)m[n+1]=(n+1)(0.5)m[n].nn4.34y[n]=4(0.75)m[n]andx[n]=3(0.25)m[n].Theirz-transformsaregivenby43Y(z)=,z>0.75andX(z)=,z>0.25.Thus,thetransferfunctionH(z)1-0.75z-11-0.25z-1Y(z)4æ1-0.25z-1ö48/9isgivenbyH(z)==ç÷=+,z>0.75.Hence,theimpulseX(z)3çè1-0.75z-1÷ø91-0.75z-148nresponseh[n]isgivenbyh[n]=d[n]+(0.75)m[n].99114.35(a)H(z)==,z>0.8.1-0.8z-1+0.15z-2(1-0.5z-1)(1-0.3z-1)2.51.5(b)Apartial-fractionexpansionofH(z)yieldsH(z)=-.Hence,1-0.5z-11-0.3z-1nnh[n]=2.5(0.5)m[n]-1.5(0.3)m[n].(c)thestepresponses[n]isgivenbytheinversez-transformofH(z)1H(z)=,z>1.Apartial-fractionexpansionofyields1-z-1(1-0.5z-1)(1-0.3z-1)(1-z-1)1-z-1H(z)2.85712.50.6429=-+.Hence,thestepresponseisgivenby1-z-11-z-11-0.5z-11-0.3z-1nns[n]=2.8571m[n]-2.5(0.5)m[n]+0.6429(0.3)m[n].4.36Y(z)=[H(z)F(z)-H(-z)F(-z)]X(z).Sincetheoutputisadelayedreplicaoftheinput,0000wemusthaveH(z)F(z)-H(-z)F(-z)=z–r.ButH(z)=1+az-1.,hence00000-1-1–r-1(1+az)F(z)-(1-az)F(-z)=z.LetF(z)=a+az.Thisimpiles,00001–1–r2(aa+a)z=z.01Thesolutionistherefore,r=1and2(aa+a)=1.Onepossiblesolutionisthusa=1/2,010-1a=1/2,anda=1/4.HenceF(z)=0.25(1+z).10126
2-122-124.37H(z)F(z)=(E(z)+zE(z))(R(z)+zR(z))00010122-12222-222=E(z)R(z)+z((E(z)R(z)+E(z)R(z))+zE(z)R(z).Thus,000110112-122-12H(-z)F(-z)=(E(z)-zE(z))(R(z)-zR(z))00010122-12222-222=E(z)R(z)-z(E(z)R(z)+E(z)R(z))+zE(z)R(z).00011011-12222Asaresult,T(z)=H(z)F(z)-H(-z)F(-z)=2z(E(z)R(z)+E(z)R(z)).00000110HencetheconditiontobesatisfiedbyE0(z),E1(z),R0(z),R1(z)fortheoutputtobea2222-rdelayedreplicaforinputisE(z)R(z)+E(z)R(z)=0.5z.(A)0110(b)InProblem4.36,E0(z)=1,E1(z)=0.5,R0(z)=0.5,R1(z)=0.25.Thus,2222E(z)R(z)+E(z)R(z)=0.5.0110HencetheconditionofEq.(A)issatisfiedwithr=1.22–222–2(c)E(z)=E(z)=z.LetR(z)=R(z)=0.25z.r=4inthiscase.Hence0101–2–11–2–1H(z)=z(1+z)andF(z)=z(1–z).44.38h[n]=h[N-n].N–1N–1N–1–n–n–(N–k)–N–1H(z)=åh[n]z=åh[N–n]z=åh[k]z=zH(z).n=0n=0k=0Soifz=z0isarootthensoisz=1/z0.IfG(z)=1/H(z)thenG(z)willhavepolesbothinsideandoutsidetheunitcircle,andwillhencebeunstable.24.39D(z)=(0.5z+1)(z+z+0.6)=0.5(z+2)(z+0.5-j0.5916)(z+0.5+j0.5916).SinceoneoftherootsofD(z)isoutsidetheunitcircleatz=–2,H(z)isunstable.Toarriveatastable,transferjwjwfunctionG(z)suchthatG(e)=H(e),wemultiplyH(z)withanallpassfunction0.5z+1A(z)=.Hencez+0.5æ3z3+2z2+5öæ0.5z+1ö3z3+2z2+5G(z)=H(z)A(z)=çç2÷÷çè÷ø=2.è(0.5z+1)(z+z+0.6)øz+0.5(z+0.5)(z+z+0.6)23(z+1.4545(z-0.7878z+1.1459)Now,H(z)=.Thus,thereare14othertransferfunctionswith(0.5z+1)(z2+z+0.6)jwthesamemagnitudeasH(e).4.40D(z)=(z+3.1238)(z+0.5762)(z-0.2-j0.5568)(z-0.2+j0.5568).SinceoneoftherootsofD(z)isoutsidetheunitcircleatz=–3.1238,H(z)isunstable.Toarriveatastable,transferjwjwfunctionG(z)suchthatG(e)=H(e),wemultiplyH(z)withanallpassfunction127
z+3.1238A(z)=.Hence3.1238z+1æ(z2+2z-3)(z2-3z+5)öæz+3.1238öG(z)=H(z)A(z)=ç÷çç÷÷çè(z+3.1238)(z+0.5762)(z2-0.4z+0.35)÷øè3.1238z+1ø22(z+2z-3)(z-3z+5)=.(3.1238z+1)(z+0.5762)(z2-0.4z+0.35)2(z+3)(z-1)(z-3z+5)NowH(z)=.Hencethereare??othertransfer(z+3.1238)(z+0.5762)(z2-0.4z+0.35)jwfunctionswiththesamemagnitudeasH(e).4.41Thetransferfunctionofthesimplestnotchfilterisgivenbyjwo-1-jwo-1-1-2H(z)=(1-ez)(1-ez)=1-2coswoz+z.Inthesteady-state,theoutputforanjwoinputx[n]=coswonisgivenbyy[n]=H(e)cos(won+q(wo)),(seeEq.(4.18)).-2(a)ComparingH1(z)=1+zwithH(z)asgivenaboveweconcludecoswo=0orwo=p/2.jwo-j2wo-jpjwoHereH1(e)=1-e=1-e=1-1=0.Hence,y[n]=H1(e)cos(won+q(wo))=0.3-1-2(b)ComparingH2(z)=1-z+zwithH(z)asgivenaboveweconcludecoswo=3/4.2jw3-jw-j2wæ3ö-jwHerenowH2(e)=1-e+e=ç2coswo-÷e=0.Hencey[n]=0.2è2ø-1-2(c)ComparingH3(z)=1+2z+zwithH(z)asgivenaboveweconcludecoswo=-1/2.jw-jw-j2w-jwHerenowH2(e)=1+2e+e=(2coswo+2)e=0.Hencey[n]=0.Y0(z)2Y1(z)24.42FromthefigureH(z)==G(z)G(z),H(z)==G(z)G(z),0X(z)LL1X(z)HLY2(z)2Y3(z)2H(z)==G(z)G(z),andH(z)==G(z)G(z),2X(z)LH3X(z)HH128
jwj(p-w)jwì1,0£w2.Fromthetablegivenaboveitcanbekk¥¥2222seenthatåhi[m]£åh7[m],andåhi[m]=åh7[m],i=1,2,...,7.m=0m=0m=0m=04.76Amaximum-phaseFIRtransferfunctionhasallzerosoutsidetheunitcircle,andhence,theproductoftherootsisnecessarilygreaterthan1.ThisimpliesthatonlythoseFIRtransferfunctionswhichhavethecoefficientofthehighestpowerinz–1(z–6inthepresentcase)greaterthan1canhavemaximumphase.ThusonlyH1(z)andH3(z)canbemaximum-phasetransferfunctions.Also,maximum-phasetransferfunctionswillhaveminimumpartial-energy(asindicatedinthesolutionofProblem4.65).Hence,H1(z)isamaximum-phasetransferfunctionsinceithasthesmallestconstanttermincomparisonwiththatofH3(z).Likewise,aminimum-phaseFIRtransferfunctionischaracterizedby:(1)largestconstantterm,and(2)thevalueofthecoefficientofthehighestpowerofz–1beinglessthan1.Inthepresentproblem,itcanbeseenthatH2(z)satisfiesthesetwoconditionsand,ishence,aminimum-phasetransferfunction.Totalno.oflength-7sequenceshavingthesamemagnituderesponseis27.Thus,thereexist123othersequenceswiththesamemagnituderesponsesasthosegivenhere.4.77(a)Type1:{h[n]}={abcdcba},(b)Type2:{h[n]}={abcddcba},140
(c)Type3:{h[n]}={abcd0-d-c-b-a},(d)Type4:{h[n]}={abcd-d-c-b-a}.-1-2-3-4-5-6-7-84.78(a)Type1:H(z)=-1-2z-4z+5z+6z+5z-4z-2z-z.FromthezeroplotobtainedusingtheM-filezplaneitcanbeseenthatcomplex-conjugatezeropairsinsideandoutsidetheunitcircleappearinmirror-imagesymmetry,complexconjugatezero-pairontheunitcircleappearsinglyandthezeroatz=1isoforder2,verifyingtheobservationsofSection4.4.4oftext.-1-2-3-4-5-6-7-8-9(b)Type2:H(z)=-1-2z-4z+5z+6z+6z+5z-4z-2z-z.FromthezeroplotobtainedusingtheM-filezplaneitcanbeseenthatcomplex-conjugatezeropairinsideandoutsidetheunitcircleappearinmirror-imagesymmetry,complexconjugatezero-pairontheunitcircleappearsingly,thereisazeroatz=–1,andthezerosontherealaxisexhibitmirror-imagesymmetry,verifyingtheobservationsofSection4.4.4oftext.-1-2-3-4-6-7-8-9-10(c)Type3:H(z)=-1-2z-4z+5z+6z-6z-5z+4z+2z+z.FromthezeroplotobtainedusingtheM-filezplaneitcanbeseenthatcomplex-conjugatezeropairinsideandoutsidetheunitcircleappearinmirror-imagesymmetry,complexconjugatezero-pairontheunitcircleappearsingly,thereisazeroatz=–1andatz=1,verifyingtheobservationsofSection4.4.4oftext.-1-2-3-4-5-6-7-8-9(d)Type4:H(z)=-1-2z-4z+5z+6z-6z-5z+4z+2z+z.FromthezeroplotobtainedusingtheM-filezplaneitcanbeseenthatcomplex-conjugatezeropairinsideandoutsidetheunitcircleappearinmirror-imagesymmetry,complexconjugatezero-pairontheunitcircleappearsingly,andthereisazeroatz=1,verifyingtheobservationsofSection4.4.4oftext.4.79H1(z)isofType1andhence,ithasasymmetricimpulseresponseofoddlength2n+1.Leta-1betheconstanttermofH1(z).Then,thecoefficientofthehighestpowerofzofH1(z)isalsoa.H2(z)isofType2andhence,ithasasymmetricimpulseresponseofevenlength2m.Letb-1betheconstanttermofH2(z).Then,thecoefficientofthehighestpowerofzofH2(z)isalsob.H3(z)isofType3andhence,ithasananti-symmetricimpulseresponseofoddlength2r+1.-1LetgbetheconstanttermofH3(z).Then,thecoefficientofthehighestpowerofzofH3(z)is–g.141
H4(z)isofType4andhence,ithasananti-symmetricimpulseresponseofevenlength2k.-1LetdbetheconstanttermofH4(z).Then,thecoefficientofthehighestpowerofzofH4(z)is–d.(a)ThelengthofH1(z)H1(z)is(2n+1)+(2n+1)–1=4n+1whichisodd.Theconstantterm2-12ofH1(z)H1(z)isaandthecoefficientofthehighestpowerofzofH1(z)H1(z)isalsoa.Hence,H1(z)H1(z)isofType1.(b)ThelengthofH1(z)H2(z)is(2n+1)+(2m)–1=2(n+m)whichiseven.Theconstant-1termofH1(z)H2(z)isabandthecoefficientofthehighestpowerofzofH1(z)H2(z)isalsoab.Hence,H1(z)H2(z)isofType2.(c)ThelengthofH1(z)H3(z)is(2n+1)+(2r+1)–1=2(n+r)+1whichisodd.Theconstant-1termofH1(z)H3(z)isagandthecoefficientofthehighestpowerofzofH1(z)H3(z)isalso–ag.Hence,H1(z)H3(z)isofType3.(d)ThelengthofH1(z)H4(z)is(2n+1)+(2k)–1=2(n+k)whichiseven.Theconstant-1termofH1(z)H4(z)isadandthecoefficientofthehighestpowerofzofH1(z)H4(z)isalso–ad.Hence,H1(z)H4(z)isofType4.(e)ThelengthofH2(z)H2(z)is(2m)+(2m)–1=4m–1whichisodd.Theconstant2-1termofH2(z)H2(z)isbandthecoefficientofthehighestpowerofzofH2(z)H2(z)is2alsob.Hence,H2(z)H2(z)isofType1.(f)ThelengthofH3(z)H3(z)is(2r+1)+(2r+1)–1=4r+1whichisodd.Theconstantterm2-1ofH3(z)H3(z)isgandthecoefficientofthehighestpowerofzofH3(z)H3(z)isalso2g.Hence,H3(z)H3(z)isofType1.(g)ThelengthofH4(z)H4(z)is(2k)+(2k)–1=4k–1whichisodd.Theconstanttermof2-12H4(z)H4(z)isdandthecoefficientofthehighestpowerofzofH4(z)H4(z)isalsod.Hence,H4(z)H4(z)isofType1.(h)ThelengthofH2(z)H3(z)is(2m)+(2r+1)–1=2(m+r)whichiseven.Theconstant-1termofH2(z)H3(z)isbgandthecoefficientofthehighestpowerofzofH2(z)H3(z)is–bg.Hence,H2(z)H3(z)isofType4.(i)ThelengthofH3(z)H4(z)is(2r+1)+(2k)–1=2(r+k)whichiseven.Theconstant-1termofH3(z)H4(z)isgdandthecoefficientofthehighestpowerofzofH3(z)H4(z)isalsogd.Hence,H3(z)H4(z)isofType2.142
N/2(((4.80(a)G(w)=H(w)+d.H(w)=åa(n)cos(wn)wherea[0]=h[N/2].Hencen=0ìh[n],"nexceptn=N/2,g[n]=íThusm=N/2anda=d.îh[N/2]+d,n=N/2.((jwjw(b)SinceG(w)isrealandpositivehenceitcanbeexpressedasG(w)=F(e)F*(e).AsH(z)isalinearphasefiltersoisG(z).ThereforeG(z)willhaverootsatz=zandatz=1/z.ooThismeansthatG(z)willhaverootsinsidetheunitcirclewithreciprocalrootsoutsidetheunitcircle.HenceF(z)canbeobtainedbyassigningtoitalltherootsthatareinsidetheunitcircle.-1ThenF(z)isautomaticallyassignedthereciprocalrootsoutsidetheunitcircle.((c)No,H(w)cannotbeexpressedasthesquaremagnitudeofaminimum-phaseFIRfilter(becauseH(w)takesonnegativevaluestoo.K¥22-1n4.81åh[n]=0.95åh[n].SinceH(z)=1/(1+az),henceh[n]=(-a)m[n]..Thusn=0n=02K1-a0.95log(0.05)=.SolvingthisequationforKwegetK=0.5.1-a21-a2log(a)-1-24.82(a)F(z)=1+2z+3z.F1(z)hasrootsatz=–1±j2.SinceH(z)isalinearphaseFIR1transferfunction,itsrootsmustexistinreciprocalpairs.HenceifH(z)hasrootsat112z=–1±j2,thenitshouldalsohaverootsatz==-±j.ThereforeH(z)-1±j23312-1-2shouldatleasthaveanotherfactorwithrootsatz=-±j.HenceF(z)=3+2z+z,332whichisthemirrorimagepolynomialtoF1(z)andH(z)=F(z)F(z)12-1-2-1-2-1-2-3-4=(1+2z+3z)(3+2z+z)=3+8z+14z+8z+3z.-1-2-3(b)F(z)=3+5z-4z-2z.Itsmirror-imageolynomialisgivenby1-1-2-3F(z)=–2–4z+5z+3z.Therefore,H(z)=F(z)F(z)212-1-2-3-1-2-3=(3+5z–4z–2z)(–2–4z+5z+3z)-1-2-3-4-5-6=–6–22z+3z+54z+3z–22z–6z.*1-d*z(1-dz*)1-d1z2(1)14.83A(z)=.A(z)=.Thus,1z-d1(z-d)z*-d*11(1)****2(z-d1)(z-d1)-(1-d1z)(1-d1z)1-A1(z)=(z-d**1)(z-d1)143
22**22**22z+d-dz-zd-1-dz+dz+dz(z-1)(1-d)1111111==.**2(z-d1)(z-d1)z-d1ìifz>1,ìifz>1,2ï>0,2ï<1,Hence,1-A(z)í=0,ifz=1,Thus,A(z)í=1,ifz=1,11ïî<0,ifz<1.ïî>1,ifz<1.ThusEq.(4.132)holdsforanyfirstorderallpassfunction.Iftheallpassisofhigherorderitcanbefactoredintoaproductoffirstorderallpassfunctions.SinceEq.(4.132)holdstrueforeachofthesefactorsindividuallyhenceitalsoholdstruefortheproduct.4.84Anm-thorderstable,realallpasstransferfunctionA(z)canbeexpressedasaproductoffirst-1-d*ziorderallpasstransferfunctionsoftheformA(z)=.Ifdiiscomplex,thenA(z)hasiz-di1-dz"ianotherfactoroftheformA(z)=.Now,iz-d*i1-d*ejw(1-d*ejw)(1-d*ejw)jwi-jwiijqjqA(e)==e.Letd=de=ae.Then,iejw-d(1-de-jw)(1-d*ejw)iiiii-jqjw2jw-jw(1-aee)A(e)=e.Therefore,i(1+a2-2acos(q-w))ì2üjw-jqjw-jqjwarg{Ai(e)}=-w+argíî(1-aee)ýþ=-w+2arg{(1-aee)}éù-1asin(q-w)=-w+2tanêú.êë1-acos(q-w)úûéù"jw-1-asin(q+w)Similarly,arg{A(e)}=-w+2tanêú.iêë1-acos(q+w)úûéùjw-1-asinwIfdi=aisreal,thenarg{A(e)}=-w+2tanêú.iêë1-acoswúûj0jp-1-1Now,forrealdi,arg{A(e)}-arg{A(e)}=-0+2tan(-0)-{–p+2tan(-0)}=p.iij0"j0jp"jpForcomplexdi,arg{A(e)}+arg{A(e)}-arg{A(e)}-arg{A(e)}iiiiéùéù-1asinq-1-asinq=-0+2tanêú-0+2tanêúêë1-acosqúûêë1-acosqúûéùéù-1-asinq-1asinq+p-2tanêú+p-2tanêú=2p.êë1+acosqúûêë1+acosqúûdjwNow,t(w)=-(arg{A(e)}).dwppjwj0jpTherefore,òt(w)dw=-òd[arg{A(e)}]=arg{A(e)}-arg{A(e)}.00144
mpjwjwSincearg{A(e)}=åarg{Ai(e)},itfollowsthatòt(w)dw=mp.0i=1-1-jwjw/2-jw/2jbd1+zjwd1+ed1e+eaej2b4.85(a)A1(z)=-1.Thus,A1(e)=-jw=jw/2-jw/2=-jb=e,1+d1z1+d1ee+d1eaejbjw/2-jw/2whereae=d1e+e=(d1+1)cos(w/2)+j(d1-1)sin(w/2).Therefore,phaseisæö-11-d1givenbyq(w)=2b=-2tançtan(w/2)÷.Forsmallvaluesofx,tan(x)»xandè1+d1ø-1tan(x)»x.Hence,theapproximateexpressionforthephaseatlowfrequenciesisgivenbyæ1-d1öwæ1-d1öq(w)»-2ç÷=-ç÷w.Therefore,theapproximateexpressionforthephasedelayè1+d1ø2è1+d1øq(w)1-d1isgivenbytph(w)=-=d»samples.w1+d11-1+z1-d0.513(b)Ford=0.5samples,d1===.Then,A1(z)=.Thus,theexactphase1+d1.531-11+z3q(w)2æ1-d1ö2delayisgivenbytph(w)=-=çtan(w/2)÷=(0.5tan(w/2)).wwè1+d1øwForasamplingrateof20kHz,thenormalizedangularfrequencyequivalentof1kHzis3101wo=3==0.05.Theexactphasedelayatwoisthus20´102022tph(wo)=(0.5tan(wo/2))=(0.5tan(0.025))=0.500078samples,whichisseentobewo0.05veryclosetothedesiredphasedelayof0.5samples.10.60.90.550.80.50.70.60.450.50.400.20.40.60.8100.050.10.150.2ww145
-jw-j2wjw-jwjwd2+d1e+ed2e+d1+e4.86A2(e)=-jw-j2w=jw-jw1+d1e+d2ee+d1+d2e(d1+d2cosw+cosw)+j(d2sinw-sinw)(d1+(d2+1)cosw)+j(d2-1)sinw==.Therefore(d1+d2cosw+cosw)+j(sinw-d2sinw)(d1+(d2+1)cosw)-j(d2-1)sinw-1æ(d2-1)sinwöq(w)2-1æ(d2-1)sinwöq(w)=2tanç÷.Now,tp(w)=-=-tanç÷.èd1+(d2+1)coswøwwèd1+(d2+1)coswø2æö-1(d2-1)wForw@0,sinw=wandcosw=1.Thentp(w)=-tanç÷.Again,forx@0,wèd1+(d2+1)ø-12(d2-1)w2(d2-1)tanx@x.Hence,tp(w)=-=-=d.wd1+(d2+1)d1+d2+1æ2-dö(2-d)(1-d)Now,substitutingd1=2ç÷andd2=wecanshoweasilythatè1+dø(2+d)(1+d)2(d2-1)-=d.d1+d2+14.87SinceG(z)isnon-minimumphasebutcausal,itwillhavesomezerosoutsidetheunitcircle.Letæ-1ö-1-11-azz=abeonesuchzero.WecanthenwriteG(z)=P(z)(1–az)=P(z)(-a*+z)çç-1÷÷,è-a*+zøæ1-az-1öNotethatç÷isastablefirstorderallpassfunction.Ifwecarryoutthisoperationforè-a*+z-1øallzerosofG(z)thatareoutsidetheunitcirclewecanwriteG(z)=H(z)A(z)whereH(z)willhaveallzerosinsidetheunitcircleandwillthusbeaminimumphasefunctionandA(z)willbeaproductoffirstorderallpassfunctions,andhenceanallpassfunction.2(z+1.4)(z+2z+4)4.88H(z)=.Inordertocorrectformagnitudedistortionwerequirethe(z+0.8)(z-0.6)jw1transferfunctionG(z)tosatisfythefollowingpropertyG(e)=.Hence,onepossiblejwH(e)1(z+0.8)(z-0.6)solutionisG(z)==.SinceG(z)haspolesoutsidetheunitcircle.dH(z)(z+1.4)(z2+2z+4)ditisnotstable.ThereforewerequireastabletransferfunctionwithmagnituderesponsesameasG(z).Usingthetechniqueofthepreviousproblemwethusget:d2(z+0.8)(z-0.6)(z+2z+4)(z+1.4)(z+0.8)(z-0.6)G(z)==isthedesiredstable(z+1.4)(z2+2z+4)(4z2+2z+1)(1.4z+1)(4z2+2z+1)(1.4z+1)jwjwsolutionsuchthatG(e)H(e)=1.4.89(a)G(z)=H(z)A(z)whereA(z)isanallpassfunction.Then,g[0]=limG(z).z®¥g[0]=limG(z)=limH(z)A(z)=limH(z)A(z)=limH(z)limA(z)z®¥z®¥z®¥z®¥z®¥146
£limH(z)becauselimA(z)<1(seeProblem4.71)z®¥z®¥£h[0](b)IflisazeroofH(z),thenl<1,sinceH(z)isaminimum-phasecausalstabletransferll-1functionwhichhasallzerosinsidetheunitcircle.WecanexpressH(z)=B(z)(1-lz).ItlfollowsthatB(z)isalsoaminimum-phasecausaltransferfunction.(l*-z-1)*-1lNowconsiderthetransferfunctionF(z)=B(z)(l-z)=H(z).Ifh[n],b[n],andl(1-lz-1)lf[n]denote,respectively,theinversez-transformsofH(z),B(z),andF(z),thenwegetìb[0],n=0h[n]=íîb[n]-lb[n-1],n³1,lìïl*b[0],n=0,andf[n]=í*lîïllb[n]-b[n-1],n³1.mmmm222*2222Considere=åh[n]-åf[n]=b[0]-llb[0]+åh[n]-åf[n].n=0n=0n=1n=12222*Nowh[n]=b[n]+lb[n-1]-lb[n-1]b*[n]-lb*[n-1]b[n],andlll2222*f[n]=lb[n]+b[n-1]-lb[n-1]b*[n]-lb*[n-1]b[n],lllmm222æ222öæ222öHence,e=b[0]-llb[0]+åçèb[n]+llb[n-1]÷ø-åçèllb[n]-b[n-1]÷øn=1n=1æ2ö2=ç1-ll÷b[m].èømmmm2222Sincell<1,e>0,i.e,åh[n]>åf[n].Hence,åh[n]³åg[n].n=0n=0n=0n=0(z+3)(z-2)(3z+1)(1-2z)(z+3)(z-2)4.90H(z)==G(z)A(z)=(z-0.25)(z+0.5)(z-0.25)(z+0.5)(3z+1)(1-2z)(3z+1)(1-2z)ThereforeG(z)=.Theinversez-transformsofthesecausaltransfer(z-0.25)(z+0.5)functionsaregivenbyh[n]={1,0.75,-6.0625,1.6093,–1.16015,L},andg[n]={-6,2.5,–0.375,0.40625,–0.1484,0.0879,L},respectively.Itcanbem2m2seenthatåg[n]isbiggerthanåh[n]forallvaluesofm.n=0n=04.91SeeExample4.13.1-22-21-221-22(a)H(z)=(1+z).Thus,H(z)=z-(1+z)=-(1-z).BS4BP441-2-2-4-41-2-2-4(b)H(z)=(1+z)(-1+6z-z).Thus,H(z)=z-(1+z)(-1+6z-z)BS16BP16147
1-2-4-6-81-24={1-4z+6z-4z+z}=(1-z).16161-22-2-4(c)H(z)=(1+z)(-3+14z-3z).Thus,BS32-41-22-2-41-2-4-6-8HBP(z)=z-(1+z)(-3+14z-3z)={3-8z+10z-8z+3z}32321-2-4-6-8={3-8z+10z-8z+3z}.324.92H(z)=A(z)+A(z),andH(z)=A(z)–A(z),whereA0(z)andA1(z)areallpass001101functionsofordersMandN,respectively,withnocommonfactors.Hence,theordersofH0(z)–M–1–N–1zD(z)zD(z)01andH1(z)areM+N.Now,wecanwriteA(z)=,andA(z)=.0D(z)1D(z)01-M-1-N-1P(z)zD0(z)D1(z)+zD0(z)D1(z)Then,H(z)==,and0D(z)D(z)D(z)01-M-1-N-1Q(z)zD0(z)D1(z)–zD0(z)D1(z)H(z)==.1D(z)D(z)D(z)01–(M+N)–1–(M+N)M–1N–1SinceP(z)isofdegreeM+NandzP(z)=z(zD(z)D(z)+zD(z)D(z))0101–N–1–M–1=zD(z)D(z)+zD(z)D(z)=P(z).HenceP(z)issymmetric.Similarlyonecan0101provethatQ(z)isanti-symmetric.11–1–14.93H(z)=[A(z)+A(z)],H(z)=[A(z)–A(z)].Thus,H(z)H(z)+H(z)H(z)0201120100111–1–11–1–1=4[A0(z)+A1(z)][A0(z)+A1(z)]+4[A0(z)–A1(z)][A0(z)–A1(z)].1–1–1–1–1=4[A0(z)A0(z)+A0(z)A1(z)+A1(z)A0(z)+A1(z)A1(z)]1–1–1–1–1+4[A0(z)A0(z)–A0(z)A1(z)–A1(z)A0(z)+A1(z)A1(z)]1–1–1jw2jw2=2[A0(z)A0(z)+A1(z)A1(z)]=1.Thus,H0(e)+H1(e)=1implyingthatH0(z)andH1(z)formapowercomplementarypair.jw21jw*jwjw*jwjw*jwjw*jw4.94H(e)={A(e)A(e)+A(e)A(e)+A(e)A(e)+A(e)A(e)}.0400100111jwjf(w)jwjf(w)SinceA0(z)andA1(z)areallpassfunctions,A(e)=e0andA(e)=e1.01jw21j(f(w)-f(w))-j(f(w)-f(w))ThereforeH0(e)={2+e01+e01}£1asmaximumvaluesof4j(f(w)-f(w))-j(f(w)-f(w))e01ande01are1.H0(z)isstablesinceA0(z)andA1(z)arestabletransferfunctions.Hence,H0(z)isBR.148
M-1M-1M-11-11-14.95H(z)=åAk(z).Thus,H(z)H(z)=2ååAk(z)Ar(z).Hence,MMk=0r=0k=0M-1M-1jw21j(f(w)-f(w))H(e)=ååekr£1.AgainH(z)isstablesince{Ai(z)}arestable2Mr=0k=0transferfunctions.Hence,H(z)isBR.æ-2ö-1-21-a1-z1-a1-2bz+z4.96H(z)=ç÷andH(z)=.BP2çè1-b(1+a)z-1+az-2÷øBS21-b(1+a)z-1+az-2-2-2-1-2-1-21-a-z+az+1-2bz+z+a-2abz+azH(z)+H(z)==1.BSBP-1-22(1-b(1+a)z+azHenceH(z)andH(z)areallpasscomplementary.BPBS-1-14.97H(z)H(z)+H(-z)H(-z)=K.Ontheunitcircle,thisreducesto22jw-jwjw-jwjwjwH(e)H(e)+H(-e)H(-e)=K,orequivalently,H(e)+H(-e)=K,asH(z)isa22jwj(p+w)real-coefficienttransferfunction.Now,H(-e)=H(e).Hence,forw=p/2,thepower-symmetricconditionreducesto2222jp/2j(p+p/2)jp/2j(2p-p/2)H(e)+H(e)=H(e)+H(e)=K.SinceH(z)isareal-coefficient222jwjp/2j(2p-p/2)transferfunction,H(e)isanevenfunctionofw,andthus,H(e)=H(e).2jp/2jp/2Asaresult,2H(e)=K,fromwhichweobtain10log2+20logH(e)=10logK,101010jp/2or20logH(e)=G(w)=10logK-3.10w=p/2102-124.98H(z)=A(z)+A(z).Therefore,01-1-12-12-2-2H(z)H(z)+H(-z)H(-z)=[A(z)+zA(z)][A(z)+zA(z)]01012-1222+[A0((-z))-zA1((-z))][A0((-z))-zA1((-z))]2-2-12-22-22-2=A(z)A(z)+zA(z)A(z)+zA(z)A(z)+A(z)A(z)001001112-2-12-22-22-2+A(z)A(z)-zA(z)A(z)-zA(z)A(z)+A(z)A(z)=4,as001001112-22-2A(z)A(z)=A(z)A(z)=1.00111-121-227-3-45-54.99(a)Ha(z)=-z+z-z-5z-z.Thus,2222-153-1-3-5Ha(z)Ha(z)=-1.25z-28z-72,75z+325-72,75z-28z-1.25zand-153-1-3-5Ha(-z)Ha(-z)=1.25z+28z+72,75z+325+72,75z+28z+1.25z.Hence,-1-1Ha(z)Ha(z)+Ha(-z)Ha(-z)=650.149
-1-2-3-4-5(b)Hb(z)=1+3z+14z+22z-12z+4z.Thus,-153-1-3-5Hb(z)Hb(z)=4z+42z+41z+850+41z+42z+4zand-153-1-3-5Hb(-z)Hb(-z)=-4z-42z-41z+850-41z-42z-4z.Hence,-1-1Hb(z)Hb(z)+Hb(-z)Hb(-z)=1700.-12-12222-124.100H(z)H(z)=a(1+bz)(1+bz)=abz+a(1+b)+abz.Thus,c=aband22-1-1-1-1d=a(1+b).Now,H(z)H(z)+H(-z)H(-z)=cz+d+cz-cz+d-cz=2d.Therefore,22212d=2a(1+b)=1.Thisconditionissatisfiedbya=1/2(1+b).Forb=1,thena=.211Othersolutionsincludeb=–1anda=,b=2anda=.210-1-1SinceH(z)isafirst-orderFIRcausalFIRtransferfunction,G(z)=-zH(-z)isalsoafirst-orderFIRcausalFIRtransferfunction.Now,-1-1-1-1-1H(z)H(z)+G(z)G(z)=H(z)H(z)+[-zH(-z)][-zH(-z)]-1-1=H(z)H(z)+H(-z)H(-z)=1.HenceH(z)andG(z)arepower-complementary.-1-1222-1-24.101H(z)H(z)=(cz+d+cz)[d2z+d1(1+d2)z+(1+d1+d2)+d1(1+d2)z+d2z].-1-12222Thus,H(z)H(z)+H(-z)H(-z)=2[cd1(1+d2)z+2cd1(1+d2)+dd2z+d(1+d1+d2)-2-2+dd2z+cd1(1+d2)z]=1.Hence,werequire22dd2+cd1(1+d2)=0,and2cd1(1+d2)+d(1+d1+d2)=1.Solvingthesetwoequationswed21arriveatc=22andd=-22.Ford1=d2=1,wegetd2(1+d2)(2d2-1-d1-d2)2d2-1-d1-d21c=-andd=1.2-3-1SinceH(z)isathird-orderFIRcausalFIRtransferfunction,G(z)=-zH(-z)isalsoathird-orderFIRcausalFIRtransferfunction.Now,-1-1-1-3-13H(z)H(z)+G(z)G(z)=H(z)H(z)+[-zH(-z)][-zH(-z)]-1-1=H(z)H(z)+H(-z)H(-z)=1.HenceH(z)andG(z)arepower-complementary.-1-2-3-4-50.1+0.5z+0.45z+0.45z+0.5z+0.1z12-14.102H(z)=-2-4=[A(z)+z],where1+0.9z+0.2z2-1-20.2+0.9z+zA(z)=isastableallpassfunction.Thus,-1-21+0.9z+0.2z-1-112-1-212-1-2H(z)H(z)+H(-z)H(-z)=[A(z)+z][A(z)+z]+[A(z)-z][A(z)-z]=1.441-14.103(a)H(z)=(1+az).11+a150
22jw1221+a+2acoswH1(e)=2{(1+acosw)+(asinw)}=2.Thus,(1+a)(1+a)2jwdH(e)1–2asinwjw=<0,fora>0.ThemaximumvalueofH(e)=1atw=0,andthedw(1+a)212jwdH(e)1minimumvalueisatw=p.Ontheotherhand,ifa<0,then>0,Inthiscasethedwjw22maximumvalueofH(e)=(1–a)/(1+a)>1atw=p,andtheminimumvalueisatw=10.Hence,H1(z)isBRonlyfora>0.221-1jw1+b-2bcosw(b)H(z)=(1-bz).H(e)=.Thus,21+b2(1+b)22jwdH(e)22bsinwjw=>0,forb>0.ThemaximumvalueofH(e)=1atw=p,andthedw(1+b)222jwdH(e)2minimumvalueisatw=0.Ontheotherhand,ifb<0,then<0,Inthiscasethedwjw22maximumvalueofH(e)=(1–b)/(1+b)>1atw=0,andtheminimumvalueisatw=2p.Hence,H2(z)isBRonlyforb>0.-1-1(1+az)(1–bz)(c)H3(z)=.FromtheresultsofParts(a)and(b)itfollowsthatH3(z)isBR(1+a)(1+b)onlyfora>0andb>0.-1-1-1æ-1öæ-1öæ-1ö(1+0.4z)(1+0.5z)(1+0.6z)1+0.4z1+0.5z1+0.6z(d)H(z)==ç÷ç÷ç÷.Since43.36çè1.4÷øçè1.5÷øçè1.6÷øeachindividualfactorsontheright-handsideisBR,H4(z)isBR.–1æ–1ö2+2z11+3z14.104(a)H1(z)=–1=çç1+–1÷÷=(A0(z)+A1(z)),whereA0(z)=1and3+z2è3+zø2–11+3zA1(z)=–1arestableallpassfunctions.InviewofProblem4.80,H1(z)isBR.3+z–1æ–1ö1–z12+4z1(b)H2(z)=–1=çç1––1÷÷=(A0(z)–A1(z)),whereA0(z)=1and4+2z2è4+2zø2–12+4zA1(z)=–1arestableallpassfunctions.InviewofProblem4.79,H1(z)isBR.4+2z151
–2æ–1–2ö–1–21–z12+2z+4z2+2z+4z(c)H(z)==ç1–÷,whereA(z)=isa34+2z–1+2z–22ç4+2z–1+2z–2÷14+2z–1+2z–2èøstableallpassfunction.Hence,H3(z)isBR.–1–2éæ–1öùéæ–1öù(d)H(z)=3+6z+3z=ê1ç1+1+3z÷úê1ç1+1+2z÷úwhichisseentobeaproduct46+5z–1+z–2ê2çè3+z–1÷øúê2çè2+z–1÷øúëûëûoftwoBRfunctions.Hence,H4(z)isBR.–1–2æ–1–2ö3+2z+3z12+2z+4z1(e)H(z)==ç1+÷=(1+A(z))where54+2z–1+2z–22çè4+2z–1+2z–2÷ø21–1–22+2z+4zA1(z)=–1–2isastableallpassfuncion.Hence,H5(z)isBR.4+2z+2z–1–2–3éæ–1öùéæ–1öùæ–1ö(f)H(z)=3+9z+9z+3z=ê1ç1+1+3z÷úê1ç1+1+2z÷úç1+z÷whichisseen612+10z–1+2z–2ê2çè3+z–1÷øúê2çè2+z–1÷øúçè2÷øëûëûtobeaproductofthreeBRfunctions.Hence,H6(z)isBR.jwjwjwjf(w)4.105SinceA1(z)andA2(z)areLBR,aaA1(e)=1andA(e)=1.Thus,A(e)=e1,21æöandA(ejw)=ejf2(w).Now,Aç1÷=A(e–jf2(w))(e-jf2(w))=1.Hence,21çjw÷1.Thus,A1èA2(e)øæö1Aç÷isLBR.1çèA(z)÷ø2æG(z)+aöæjw)+aöæjf(w)+aö4.106F(z)=zç÷.Thus,F(ejw)=ejwçG(e÷=ejwçe÷sinceG(z)isLBR.è1+aG(z)øçè1+aG(ejw)÷øçè1+aejf(w)÷ø2jf(w)2222jwe+a(cosf(w)+a)+(sinf(w))1+2acosf(w)+aF(e)====1.jf(w)2221+ae(1+acosf(w))+(asinf(w))1+2acosf(w)+aLetz=lbeapoleofF(z).Thisimplies,G(l)=–1/aorG(l)=1/a.Ifa<1,thenG(l)>1,whichissatisfiedbytheLBRG(z)ifl<1.Hence,F(z)isLBR.TheorderofF(z)issameasthatofG(z).G(z)canberealizedintheformofatwo-pairconstrainedbythetransferfunctionF(z).152
–1–a+zF(z)C+DF(z)Tothisend,weexpressG(z)intermsofF(z)arrivingatG(z)==,1–az–1F(z)A+BF(z)whereA,B,C,andD,arethechainparametersofthetwo-pair.Comparingthetwoexpressions–1–1ontheright-handsidewegetA=1,B=–az,C=–a,andD=z.Thecorresponding2–1–1transferparametersaregivenbyt=–a,t=1,t=(1–a)z,andt=az.11211222æ1öjwjf(w)4.107LetF(z)=Gç÷.NowA(z)beingLBR,A(e)=e.Thus,èA(z)øjwæ1ö-jf(w)-jf(w)F(e)=Gçç÷÷=G(e).SinceG(z)isaBRfunction,G(e)£1.Hence,èA(ejw)øæ1öjwF(e)=Gçç÷÷£1.èA(ejw)øLetz=xbeapoleofF(z).Hence,F(z)willbeaBRfunctionif|x|<1.Letz=lbeapoleofG(z).Thenthispoleismappedtothelocationz=xofF(z)bytherelation111=l,orA(x)=.Hence,A(x)=>1becauseofEq.(4.129).Thisimplies,A(z)z=xllæ1öl<1.ThusGç÷isaBRfunction.èA(z)ø-1-1-1-1-12+2z1-z2+2z1-z3+z4.108(a)H(z)=,G(z)=.Now,H(z)+G(z)=+==1.-1-1-1-1-13+z3+z3+z3+z3+z-1-1-1-12+2z2+2z1-z1+zNext,H(z)H(z)+G(z)G(z)=+=3+z-13+z3+z-13+z4+4z-1+4z+4+1+1-z-1-z22jwjw=1.Thus,H(e)+G(e)=1-1(3+z)(3+z)HenceH(z)andG(z)arebothallpass-complementaryandpowercomplementary.Asaresult,theyaredoublycomplementary.-2-1-2-1-2-1+z3+2z+3z2+2z+4z(b)H(z)=,G(z)=.NoteH(z)+G(z)=-1-2-1-2-1-24+2z+2z4+2z+2z4+2z+2z-1-1implyingthatH(z)andG(z)areallpasscomplementary.Next,H(z)H(z)+G(z)G(z)-22-1-22-1+z-1+z3+2z+3z3+2z+3z=+-1-22-1-224+2z+2z4+2z+2z4+2z+2z4+2z+2z-222-1-2-11+1-z-z+9+6z+9z+6z+4+6z+9z+6z+9==1.Hence,H(z)-1-22(4+2z+2z)(4+2z+2z)andG(z)arealsopowercomplementary.Asaresult,theyaredoubly-complementary.153
–1–2æ–1–2ö2(1+z+z)11+2z+3z4.109(a)H(z)==ç1+÷.Itspower-complementarytransfera3+2z–1+z–22ç3+2z–1+z–2÷èøæ–1–2ö–211+2z+3z1–zfunctionthereforeisgivenbyG(z)=ç1–÷=.a2ç3+2z–1+z–2÷3+2z–1+z–2èø-1-2-3-1-2-33(1.5+6.5z+6.5z+1.5z)3(1.5+6.5z+6.5z+1.5z)(b)H(z)==b18+21z-1+8z-2+z-3(2+z-1)(3+z-1)(3+z-1)1é(1+2z-1)(1+3z-1)1+3z-1ù=ê+ú.Itspower-complementarytransfer2êë(2+z-1)(3+z-1)3+z-1úûfunctionthereforeisgivenby1é(1+2z-1)(1+3z-1)1+3z-1ù-1.5-3.5z-1+3.5z-2+1.5z-3G(z)=ê-ú=.b2êë(2+z-1)(3+z-1)3+z-1úû18+21z-1+8z-2+z-3jw2jw21ìjwjw2jwjw2ü4.110G(e)+G(e)=íA(e)+A(e)+A(e)-A(e)ý014î0101þ1jwjw*jw*jwjwjw*jw*jw={(A(e)+A(e))(A(e)+A(e))+(A(e)-A(e))(A(e)-A(e))}4010101011jw*jwjw*jwjw*jwjw*jw={A(e)A(e)+A(e)A(e)+A(e)A(e)+A(e)A(e)400110110jw*jwjw*jwjw*jwjw*jw+A(e)A(e)+A(e)A(e)-A(e)A(e)-A(e)A(e)}001101101ìjw2jw2ü=í2A(e)+2A(e)ý=1.4î01þ4.111LettheoutputofthepredictorinFigureP4.11(a)bedenotedbyE(z).ThenanalyzingthisfigurewegetE(z)=P(z)[U(z)+E(z)]andU(z)=X(z)-E(z).FromthefirstequationweP(z)haveE(z)=U(z)whichwhensubstitutedinthesecondequationyields1-P(z)U(z)H(z)==1-P(z).X(z)AnalyzingFigureP4.11(b)wearriveatY(z)=V(z)+P(z)Y(z)whichyieldsY(z)1G(z)==,whichisseentobetheinverseofH(z).V(z)1-P(z)-1-11-1-2ForP(z)=h1z,H(z)=1-h1zandG(z)=-1.Similarly,forP(z)=h1z+h2z,1-h1z-1-21H(z)=1-h1z-h2zandG(z)=-1-2.1-h1z-h2z5/31/124.112Thez-transformofh1[n]isgivenbyH1(z)=-1+-1-1.UsingtheM-file1-0.5z1+0.2z-1-2-1-10.75+0.5917z+0.1z0.75(1+0.5436z)(1+0.2453z)residuezwearriveatH1(z)=-1-2=-1-1.1-0.3z-0.1z(1-0.5z)(1+0.2z)154
SincethezerosofthenumeratorofH1(z)areinsidetheunitcircle,theinversesystemiscausalandstable.Hence,thetransferfunctionoftheinversesystemisgivenby-1-2-1-21-0.3z-0.1z1.3333-0.4z-0.1333zH(z)==Apartial-fractionexpansionof20.75+0.5917z-1+0.1z-21+0.7889z-1+0.1333z-22.94740.6141H2(z)usingtheM-fileresiduezyieldsH2(z)=-1--1-1whoseinverse1+0.5437z1+0.2452znnz-transformisgivenbyH(z)=2.9474(-0.5437)m[n]-0.6141(-0.2452)m[n]-d[n].21B4.113X=AY+BX,Y=CY+DX.Fromthefirstequation,Y=X–X.1221222A1A2Substitutingthisinthesecondequationwegetæ1BöCAD–BCY1=CçèAX1–AX2÷ø+DX2=AX1+AX2,ComparingthelasttwoequationswithCAD–BC1BEq.(4.146)wearriveatt=,t=,t=,t=–.11A12A21A22ANext,Y=tX+tX,Y=tX+tX.Fromthesecondequationweget12211222211222t221X=–X+Y.Substitutingthisexpressioninthefirstequationweget,1t2t22121æt221öt11t12t21–t11t22Y=tç–X+Y÷+tX=Y+X.Comparingthelasttwo111çèt2t2÷ø122t2t2212121211t22t11t12t21–t11t22equationswithEq.(4.149)wearriveatA=,B=–,C=,D=.tttt21212121AD-BC14.114FromEq.(4.176a)wenotet=andt=.Hence,t=timply12A21A1221AD-BC=1.é"ùé2-1ùé"ùé"ùé2-1ùé"ùé"ùé"ùYk(1-k)zXYk(1-k)zXXY4.115ê1"ú=ê11-1úê"1ú,ê1ú=ê22-1úê1ú,whereê1ú=ê1"ú.êYúê1-kzúêXúêY"úê1-kzúêX"úêX"úêYúë2ûë1ûë2ûë2ûë2ûë2ûë2ûë2ûék(1-k2)z-1ùThus,thetransfermatricesofthetwotwo-pairsaregivenbyt=ê11-1ú,and1êë1-kzúû1ék(1-k2)z-1ùt=ê22ú.ThecorrespondingchainmatricesareobtainedusingEq.(4.76b)and2ê1-kz-1úë2ûé1kz–1ùé1kz–1ùaregivenbyG=ê1–1ú,andG=ê2–1ú.Therefore,thechainmatrixoftheG-1êëkzúû2êëkzúû12é1kz–1ùé1kz–1ùé1+kkz-1kz-1+kz-2ùcascadeisgivenbyGG=ê1–1úê2–1ú=ê12-12-11-2ú.12êëkzúûêëkzúûêëk+kzkkz+zúû121212HenceusingEq.(4.176a)wearriveatthetransfermatrixoftheG-cascadeas155
ék+kz-1z-2(1-k2)(1-k2)ùê1211úê1+kkz-11+kkz-1út=ê12-112-1ú.ê1z(k+kz)úê-21úêë1+kkz-11+kkz-1úû1212é"ùé–1ùé"ùé"ùé–1ùé"ùé"ùé"ùX1kzYX1kzYYX4.116ê1"ú=ê1–1úê2"ú,ê1ú=ê2–1úê2ú,whereê2"ú=ê1ú.Therefore,êYúêkzúêXúêY"úêkzúêX"úêXúêY"úë1ûë1ûë2ûë1ûë2ûë2ûë2ûë1ûé1kz–1ùé1kz–1ùthechainmatricesofthetwotwo-pairsaregivenbyG=ê1–1ú,andG=ê2–1ú.1êëkzúû2êëkzúû12ThecorrespondingtransfermatricesareobtainedusingEq.(4.151a)andaregivenbyék(1-k2)z-1ùék(1-k2)z-1ùt=ê11-1ú,andt=ê22-1ú.Thetransfermatrixofthet-cascadeis1êë1-kzúû2êë1-kzúû12ék(1-k2)z-1ùék(1-k2)z-1ùthereforegivenbyt=tt=ê22-1úê11-1ú21êë1-kzúûêë1-kzúû21ékk+z-1(1-k2)z-1k(1-k2)-z-2k(1-k2)ù=ê1222112ú.êk-kz-1z-1(1-k2)+kk2z-2úë1211ûUsingEq.(4.151b)wethusarriveatthechainmatrixofthet-cascade:é1-(kkz-1+1-k2)z-1ùê121úêk-kz-1k-kz-1úG=ê1-12212ú.êkk+z(1-k)z-2ú122êúêëk-kz-1k-kz-1úû1212-1-14.117(a)AnalyzingFigureP4.13(a)weobtainY=X-kzXandY=kY+zX21m21m22-1-12-1=km(X1-kmzX2)+zX2=kmX1+(1-km)zX2.Hence,thetransferparametersaregivenék(1-k2)z-1ùbyt=êmm-1ú.UsingEq.(4.176b)wearriveatthechainparametersgivenbyêë1-kmzúûé1kz-1ùG=êmú.êkz-1úëmû-1(b)AnalyzingFigureP4.13(a)weobtainV1=km(X1-zX2),-1-1-1Y=V+X=(1+k)X-kzX,andY=V+zX=kX+(1-k)zX.Hence,the111m1m2212m1m2é1+k-kz-1ùtransferparametersaregivenbyt=êmm-1ú.UsingEq.(4.176b)wearriveattheêëkm(1-km)zúûé(1-k)z-1ùê1-múêkkúchainparametersgivenbyG=mm.êúê1-km1úê-úkkëmmû156
C+DG(z)–1-14.118FortheconstrainedtwopairH(z)=.Hence,C=k,D=z,A=1,B=kz.A+BG(z)mmSubstitutingthesevaluesofthechainparametersinEq.(4.151a)wegetC-12-1t==k,t=z(1-k),t21=1,t=-kz.11Am12m22m4.119FromtheresultsofProblem4.117,Part(a),weobservethatthechainmatrixofthei-thlatticeé1kz-1ùtwo-pairisgivenbyG=êi-1ú,i=1,2,3.Thus,thechainmatrixofthecascadeofthreeiêëkzúûié1kz-1ùé1kz-1ùé1kz-1ùlatticetwo-pairsisgivenbyG=ê1-1úê2-1úê3-1úcascadeêëkzúûêëkzúûêëkzúû123é1kz-1ùé1+kkz-1kz-1+kz-2ù=ê1úê2332úêkz-1úêk+kz-1kkz-1+z-2úë1ûë2323ûé1+kkz-1+kz-1(k+kz-1)kz-1+kz-2+kz-1(kk+z-2)ù=ê2312332123ú.FromEq.(4.181a)êk(1+kkz-1)+z-1(k+kz-1)k(kz-1+kz-2)+z-1(kk+z-2)úë1232313223ûC+DweobtainA(z)=3A+B1+kkz-1+kz-1(k+kz-1)+kz-1+kz-2+kz-1(kk+z-2)2312332123=k(1+kkz-1)+z-1(k+kz-1)+k(kz-1+kz-2)+z-1(kk+z-2)1232313223k+(kk+kk+k)z-1+(kk+k+kkk)z-2+kz-31231231321231=whichisseentobeanallpassk+(kk+k+kkk)z-1+(kk+kk+k)z-2+z-3113212323123function.-1-2-1-14.120LetD(z)=1+dz+dz=(1-lz)(1-lz).Thus,d=llandd=–(l+l).1212212112Forstability,l<1,i=1,2.Asaresult,d=ll<1.i212*Case1:Complexpoles:d2>0.Inthiscase,l=l.Now,21-d±d2-4d1122l,l=.Hence,landlwillbecomplex,ifd<4d.Inthiscase,1221212d1j22122l1=–2+24d2-d1.Thus,l1=4(d1+4d2-d1)=d2<1.Consequently,ifthepolesarecomplexandd2<1,thentheyareinsidetheunitcircle.Case2:Realpoles.Inthiscaseweget–1–2+d.Againitisnotpossibleto2121satisfytheinequalityontherighthandsidewithaplussigninfrontofthesquarerootasit22wouldimplythend1>2.Therefore,-d-4d>–2+d,ord-4d<2-d,or12112122d-4d<4+d-4d,orequivalently,d<1+d.(15)121112CombiningEqs.(14)and(15)wegetd<1+d.124.121(a)d1=0.92and1+d2=1.1995.Sinced1<1+d2andd2<1,bothrootsareinsidetheunitcircle.(b)d=0.2and1+d=–0.43.Sinced>1andd>1+d,allrootsarenotinsidethe12212unitcircle.(c)d1=1.4562and1+d2=1.81.Sinced1<1+d2andd2<1,bothrootsareinsidetheunitcircle.(d)d1=2.1843and1+d2=1.81.Sinced1<1+d2andd2<1,bothrootsareinsidetheunitcircle.11–11–2–3–z–z+z124214.122(a)A(z)=.Note,k=<1.UsingEq.(4.177)wearriveat31–11–21–33121–z–z+z2412–1–2–0.2098–0.4825z+zA(z)=.Here,k=0.2098<1.Continuingthisprocess,weget,2–1–221–0.4825z–0.2098z–1–0.6106+zA(z)=.Finally,k=0.6106<1.Sincek<1,fori=3,2,1,Ha(z)isstable.1–11i1–0.6106z11–113–2–3–+z+z+z–1–236611+2.5z+z(b)A(z)=.Note,k=<1.A(z)=.Since313–11–21–33321+2.5z–1+z–21+z+z–z663k=1,Hb(z)isunstable.215–118.5–25–3–4+z+z+z+z36181831(c)A(z)=.Note,k=<1.45–118.5–25–31–44361+z+z+z+z3181836–1–2–30.2317+z+1.6602z+zA(z)=.Thus,k=0.2317<1.3–1–2–331+1.6602z+z+0.2317z–1–2–10.6503+1.5096z+z0.9147+zA(z)=.Here,k=0.6503<1.Finally,A(z)=.2–1–221–11+1.5096z+0.6503z1+0.9147zThus,k=0.9147<1.Sincek<1,fori=4,3,2,1,Hc(z)isstable.1i158
15–15–25–35–4–5+z+z+z+z+z32164221(d)A(z)=.Thisimplies,k=<1.55–15–25–35–41–55321+z+z+z+z+z2241632–1–2–3–40.2346+1.173z+2.4633z+2.4927z+zA(z)=.Thus,k=0.2346<1.4–1–2–3–441+2.4927z+2.4633z+1.173z+0.2346z–1–2–30.6225+1.9952z+2.3466z+zA(z)=.Implyingk=0.6225<1.3–1–2–331+2.3466z+1.9952z+0.6225z–1–2–10.8726+1.8034z+z0.9630+zA(z)=.Hence,k=0.8726<1.Finally,A(z)=.2–1–221–11+1.8034z+0.8726z1+0.9630zThus,k=0.9630<1.Sincek<1,fori=5,4,3,2,1,Hd(z)isstable.1i11–11–22–35–4–5+z+z+z+z+z632331(e)A(z)=.Thisimplies,k=<1.55–12–21–31–41–5561+z+z+z+z+z63266–1–2–3–40.2+0.4z+0.6z+0.8z+zA(z)=.Thus,k=0.2<1.4–1–2–3–441+0.8z+0.6z+0.4z+0.2z–1–2–30.25+0.5z+0.75z+zA(z)=.Hence,k=0.25<1.3–1–2–331+0.75z+0.5z+0.25z–1–2–10.3333+0.6667z+z0.5+zA(z)=.Here,k=0.3333<1.Finally,A(z)=.Asa2–1–221–11+0.6667z+0.3333z1+0.5zresult,k=0.5<1.Sincek<1,fori=5,4,3,2,1,He(z)isstable.1i12–13–24–3–4+z+z+z+z555514.123(a)A(z)=.Thus,k=<1..44–13–22–31–4451+z+z+z+z5555–1–2–30.25+0.5z+0.75z+zA(z)=.Thus,k=0.25<1.Repeatingtheprocess,we3–1–2–331+0.75z+0.5z+0.25z–1–20.3333+0.6667z+zarriveatA(z)=.Thus,k=0.3333<1.Finally,weget2–1–221+0.6667z+0.3333z–10.5+zA(z)=.Thus,k=0.5<1.Sincek<1,fori=5,4,3,2,1,Da(z)hasall1–11i1+0.5zrootsinsidetheunitcircle.159
–1–2–3–1–20.4+0.3z+0.2z+z0.2619+0.0952z+z(b)A(z)=.Thus,k=0.4<1.A(z)=.3–1–2–332–1–21+0.2z+0.3z+0.4z1+0.0952z+0.2619z–10.0755+zThisimplies,k=0.2619<1.Next,A(z)=.Hence,k=0.0755<1.Since21–111+0.0755zk<1,fori=3,2,1,Db(z)hasallrootsinsidetheunitcircle.i1+sz-14.124z=.Hence,s=.Thus,thek-throotinthes-domainisgivenby1-sz+12(z-1)(z*+1)z-1+z-z*kkkkks==wherezkisthek-throotinthez-domain.Hence,k22z+1z+1kk2z-1kRe{s}=.SinceD(z)isaminimumphasepolynomial,z<1.Therefore,k2kz+1kRe{s}<0.HenceB(s)isastrictlyHurwitzpolynomial.k-14.125H(z)=1-az.Hence,usingEq.(4.212)weget-1-12Fyy(z)=H(z)H(z)Fyy(z)=(1-az)(1-az)sx.Therefore,fromEq.(4.214)wehave22jwjw2-jwjw222Pyy(w)=H(e)Pxx(w)=H(e)sx=(1-ae)(1-ae)sx=(1+a-2acosw)sx.-1-12-1Now,H(z)H(z)=(1-az)(1-az)=-az+(1+a)-az.Hence,22fyy[n]=(-ad[n+1]+(1+a)d[n]-ad[n-1])sx.22Asaresult,averagepower=fyy[0]=(1+a)sx.Notethattheaveragepowerincreaseswithincreasinga.1jw2114.126H(z)=.H(e)==.Hence,romEq.1-0.5z-1(1-0.5e-jw)(1-0.5ejw)1.25-cosw2jw2Pxx(w)sx(4.214)wehavePyy(w)=H(e)Pxx(w)=-jwjw=.Now,(1-0.5e)(1-0.5e)1.25-cosw-1-1-111z2zH(z)H(z)=×=-=-1-0.5z-11-0.5z(1-0.5z-1)(0.5-z-1)(1-0.5z-1)(1-2z-1)24/34/34sxnn=-1--1.Therefore,jyy[n]=((0.5)m[n]+(2)m[-n-1]).1-0.5z1-2z32jwjw2A(e)14.127(a)P(w)=A(e)P(w)==.(16)yyxx1+dcos(w)1+dcos(w)(b)No,Theanswerdoesnotdependuponthechoiceofa.HoweverEq.(16)holdsifthefilterisstableforwhichwerequirea<1.160
4.128(a)f[l]=E{x[n+l]y[n]}=E{x[n+l]x[n]}*h[n]=f[n]*h[n].Takingthediscrete-xyxxjwjwtimeFouriertransformofbothsideswegetP(w)=P(w)H(e).SinceH(e)inxyxxgeneralisnotreal,P(w)ingeneralisnotreal.xy(b)f[l]=E{x[n+l]u[n]}=E{x[n+l]h[-n]*y[n]}=f[n]*h[n]*h[–n].Takingthexuxx2jwdiscrete-timeFouriertransformofbothsideswegetP(w)=P(w)H(e).Thus,P(w)xuxxxuisarealfunctionofw.jwdH(e)M4.1FromTable3.2,theDTFTof{nh[n]}isj.Hence,thegroupdelayt(w)usingEq.dw(4.203)canbecomputedatasetofNdiscretefrequencypointswk=2pk/N,k=0,1,...,N–1,asfollows:{t(wk)}=Re(DFT{nh[n]}/DFT{h[n]}),whereallDFTsareN-pointsinlengthwithNgreaterthanorequaltolengthof{h[n]}.M4.2WemodifyProgram4_2usingthefollowingstatements:b=[-4.87889.5631-4.8788];x1=cos(0.2*n);x2=cos(0.5*n);21.510.50-0.5-1-1.5020406080TimeindexnM4.3WemodifyProgram4_2usingthefollowingstatements:b=[-13.486645.228-63.808945.228-13.4866];zi=[0000];x1=cos(0.2*n);x2=cos(0.5*n);x3=cos(0.8*n);y=filter(b,1,x1+x2+x3,zi);21.510.50-0.5-1-1.5020406080Timeindexn161
M4.4M4.5(a)num1=[00.752210.75];num2=[0-0.752-21-0.75];den=[303.501];w=0:pi/255:pi;h1=freqz(num1,den,w);h2=freqz(num2,den,w);plot(w/pi,abs(h1).*abs(h1)+abs(h2).*abs(h2));(b)ReplacethefirstthreelinesintheaboveMATLABprogramwiththefollowing:num1=[11.53.752.752.753.751.51];num2=[1-1.53.75-2.752.75-3.751.5-1];den=[606.504.7501];M4.6ThemagnituderesponseofH(z)isasshownbelowfromwhichweobservethatH(z)isalowpassfilter.BymultiplyingoutthefactorsofH(z)weget–1–2–30.05634–0.000935z+0.000935z+0.05634zH(z)=,Thecorrespondingdifference–1–2–31–2.1291z+1.783386z+0.543463zequationrepresentationisthereforegivenbyy[n]=0.05634x[n]–0.000935x[n–1]+0.000935x[n–2]–0.05634x[n–3]–2.1291y[n–1]+1.783386y[n–2]+0.543463y[n–3].M4.7ThemagnituderesponseofH(z)isasshownbelowfromwhichweobservethatH(z)isahighpassfilter.162
BymultiplyingthefactorsofH(z)weget-1-2-3-41-4z+6z-4z+zH(z)=1-3.0538z-1+3.8227z-2-2.2837z-3+0.5472z-4Thecorrespondongdifferenceequationisgivenbyy[n]-3.0538y[n-1]+3.8227y[n-2]-2.2837y[n-3]+0.5472y[n-4]=x[n]-4x[n-1]+6x[n-2]-4x[n-3]+x[n-4].1M4.8FromEq.(4.66),weobtainM=–=4.7599.WechooseM=5.Acascade2log(cos(0.12p)21–15of5first-orderlowpassFIRfilterhasatransferfunctiongivenbyG(z)=(1+z),whose32gainresponseisplottedbelow:M4.9ForacascadeofMsectionsofthefirst-orderhighpassFIRfilterofEq.(4.67),the3-dB–1–1/2M1cutofffrequencywcisgivenbywc=2sin(2).Hence,M=–.2log(sin(w/2)2cSubstitutingthevalueofwwethenobtainM=4.76.ChooseM=5.Acascadeof5first-c1–15orderhighpassFIRfilterhasatransferfunctiongivenbyG(z)=(1–z),whosegain32responseisplottedbelow:163
M4.10FromEq.(4.72b),weobtaina=0.32492.Hence,fromEq.(4.70)weget–1–10.33754(1+z)0.66246(1–z)H(z)=.Likewise,fromEq.(4.73)wegetH(z)=.LP–1HP–11–0.32492z1–0.32492zThemagnituderesponsesofHLP(z)andHHP(z)areshowbelow:ThemagnituderesponseofHLP(z)+HHP(z)isshownbelowontheleft-handside,whilethe22jwjwgainresponseofH(e)+H(e)isshownbelowontheright-handside:LPHPM4.11FromEq.(4.76)wefirstobtainb=0.309017.FromEq.(4.77)wearriveattwopossiblevaluesofa:0.6128and1.63185leadingtotwoposiblesolutions:–2–2"0.1935(1–z)"–0.3159(1–z)H(z)=,andH(z)=.ItcanbeBP–1–2BP–1–21–0.4984z+0.6128z1–0.813287z+1.63185z"seenthatH(z)isunstable.FromEq.(4.79),thebandstoptransferfunctioncorrespondingtoBP164
–1–2""0.8064(1–0.6180z+z)H(z)isgivenbyH(z)=.AplotofthemagnituderesponsesBPBS–1–21–0.498383z+0.6128z""ofH(z)andH(z)aregivenbelow:BPBSThemagnituderesponseofHBP(z)+HBS(z)isshownbelowontheleft-handside,whilethe22jwjwgainresponseofH(e)+H(e)isshownbelowontheright-handside:BPBSM4.12FromEq.(4.76),wefirstobtainb=0.309017.FromEq.(4.77)wearriveattwopossiblevaluesofa:1.376381and0.72654253leadingtotwoposiblesolutions:–2–2"0.1935996(1–z)"–0.3159258(1–z)H(z)=,andH(z)=.BP–1–2BP–1–21+0.94798z+0.6128z1+1.5469636z+1.63185168z""ItcanbeseenthatH(z)isunstable.AplotofthegainresponseofH(z)isshownbelow:BPBPM4.13FromEq.(4.76),wefirstobtainb=0.309016.FromEq.(4.77)wearriveattwopossiblevaluesofa:0.72654253and1.376382leadingtotwoposiblesolutions:165
–1–2–1–2"0.86327126(1+0.618033z+z)"1.188191(1+0.618033z+z)H(z)=,andH(z)=.BS–1–2BS–1–21–0.533531z+0.72654253z1–0.7343424z+1.376382z""ItcanbeseenthatH(z)isunstable.AplotofthegainresponseofH(z)isshownbelow:BSBSM4.14(a)Usingthefollowingprogramwearriveatthetwoplotsshownbelow:b1=[22];b2=[1-1];den=[31];w=0:pi/255:pi;h1=freqz(b1,den,w);h2=freqz(b2,den,w);sum=abs(h1).*abs(h1)+abs(h2).*abs(h2);subplot(2,1,1);plot(w/pi,abs(h1+h2));gridaxis([0101.2]);xlabel("Normalizedfrequency");ylabel("Magnitude");title("Illustrationofallpasscomplementaryproperty");subplot(2,1,2);plot(w/pi,20*log10(sum));gridaxis([01-1010]);xlabel("Normalizedfrequency");ylabel("Gain,dB");title("Illustrationofpowercomplementaryproperty");(b)Forthispart,replacethefirstlineintheaboveprogramwiththefollowing:b1=[–101];b2=[323];den=[422];166
M4.15(a)(b)(c)(d)167
(e)(f)M4.16(a)(b)(c)(d)168
(e)M4.17(a)Thestabilitytestparametersare0.0833-0.2098-0.6106stable=1(b)Thestabilitytestparametersare–0.33331.00002.0000stable=0(c)Thestabilitytestparametersare0.02780.23170.65030.9147stable=1(d)Thestabilitytestparametersare0.03120.23460.62250.87260.9630stable=1(e)Thestabilitytestparametersare0.16670.02860.37660.32080.5277stable=1M4.18(a)Thestabilitytestparametersare0.20000.25000.33330.5000stable=1(b)Thestabilitytestparametersare0.40000.26190.0755stable=1kækö–1k–2M4.19H(z)=–+ç1+÷z–z.4è2ø4kkækkökk21–112–21–32–4M4.20H(z)=–+z+ç1–+÷z+z–z.44è22ø44169
170
Chapter5(2e)¥5.1p(t)=åd(t-nT).Sincep(t)isperiodicfunctionoftimetwithaperiodT,itcanben=-¥¥T/2j(2pnt/T)-j(2pnt/T)1representedasaFourierseries:p(t)=åcne,wherecn=òd(t)edt=T.n=-¥-T/2¥¥1j(2pnt/T)Hencep(t)=åd(t-nT)=åe.Tn=-¥n=-¥5.2Sincethesignalxa(t)isbeingsampledat1.5kHzrate,therewillbemultiplecopiesofthespectrumatfrequenciesgivenbyFi±1500k,whereFiisthefrequencyofthei-thsinusoidalcomponentinxa(t).Hence,F1=250Hz,F1m=250,1250,1750,....,HzF2=450Hz,F2m=450,1050,1950....,HzF3=1000Hz,F3m=1000,500,2500....,HzF4=2750Hz,F4m=2750,1250,250,....,HzF5=4050Hz,F5m=34050,1050,450,....,HzSoafterfilteringbyalowpassfilterwithacutoffat750Hz,thefrequenciesofthesinusoidalcomponentspresentinya(t)are250,450,500Hz.5.3OnepossiblesetofvaluesareF1=450Hz,F2=625Hz,F3=950HzandF4=7550Hz.AnotherpossiblesetofvaluesareF1=450Hz,F2=625Hz,F3=950Hz,F4=7375Hz.Hencethesolutionisnotunique.n5.4t=nT=.Therefore,4000æ400pnöæ1200pnöæ4400pnöæ5200pnöx[n]=3cosç÷+5sinç÷+6cosç÷+2sinç÷è4000øè4000øè4000øè4000øæpnöæ3pnöæ11pnöæ13pnö=3cosç÷+5sinç÷+6cosç÷+2sinç÷è10øè10øè10øè10øæpnöæ3pnöæ(20-9)pnöæ(20-7)pnö=3cosç÷+5sinç÷+6cosç÷+2sinç÷è10øè10øè10øè10øæpnöæ3pnöæ9pnöæ7pnö=3cosç÷+5sinç÷+6cosç÷-2sinç÷.è10øè10øè10øè10ø5.5Boththechannelsaresampledat48.2kHz.Thereforethereareatotalof2´48200=96400samples/sec.Eachsampleisquantizedusing15bits.Hencethetotalbitrateofthetwochannelsaftersamplinganddigitization=15´96400=1.446Mb/s.æ2pWnösinçc÷sin(Wt)sin(WnT)2pçèWT÷øcc5.6h(t)=.Thus,h(nT)=,SinceT=,henceh(nT)=.r(Wt/2)r(WnT/2)WrpnTTTsin(pn)IfW=W/2,then,h(nT)==d[n].cTrpn5.7Aftersamplingthespectrumofthesignalisasindicatedbelow:171
2ppWmppT==.Asaresult,w==.Henceafterthelowpassfilteringthespectrum2WWc3W3mmmofthesignalwillbeasshownbelow:5.8(a)W2=200p,W1=160p.Thus,DW=W2-W1=40p.NoteDWisanintegermultipleofW2.Hence,wechoosethesamplingangularfrequencyas2´200pWT=2DW=2(W2-W1)=80p=,whichissatisfiedforM=5.ThesamplingMfrequencyisthereforeFT=40Hz.Xp(jW)M=5W-160p-80p-40p040p80p120p160p200p-120p(b)W2=160p,W1=120p.Thus,DW=W2-W1=40p.NoteDWisanintegermultipleofW2.Hence,wechoosethesamplingangularfrequencyas2´160pWT=2DW=2(W2-W1)=80p=,whichissatisfiedforM=4.ThesamplingMfrequencyisthereforeFT=40Hz.Xp(jW)M=4W-160p-80p-40p040p80p120p160p200p-120p172
(c)W2=150p,W1=110p.Thus,DW=W2-W1=40p.NoteDWisnotanintegermultipleofW2.Hence,weextendthebandwidthtotheleftbyassumingthelowestfrequencytobeW0andchoosethesamplingangularfrequencysatisfyingtheequation2´150pWT=2DW=2(W2-W0)=,whichissatisfiedforW0=100pandM=3.TheMsamplingfrequencyisthereforeFT=50Hz.Xp(jW)M=3W-50p050p-ap/20-as/205.9ap=-20log10(1-dp)andas=-20log10ds.Therefore,dp=1-10andds=10.(a)ap=0.15,as=43.,Hence,dp=0.0171andds=0.0071(b)ap=0.04,as=57.Hence,dp=0.0046andds=0.0014(c)ap=0.23,as=39.Hence,dp=0.0261andds=0.01122aa2a5.10H(s)=.Thus,H(jW)=,andhence,i.e.H(jW)=.1s+a1jW+a1a2+W22H(jW)isamonotonicallydecreasingfunctionwithH(j0)=1andH(j¥)=0.Letthe11122a13-dBcutofffrequencybegivenbyW.Then,H(jW)==,andhence,W=a..c1ca2+W22cc2sjW2W5.11H(s)=.Thus,H(jW)=,andhence,H(jW)=.2s+a2jW+a2a2+W22H(jW)isamonotonicallyincreasingfunctionwithH(j0)=0andH(j¥)=1.The3-222W21cdBcutofffrequencyisgivenby=,andhence,W=a..a2+W22cca1æs-aös1æs-aö5.12H1(s)==çè1-÷ø,andH2(s)==çè1+÷ø.Thus,A1(s)=1andA2(s)=s+a2s+as+a2s+as-a.SinceA(jW)=1andA(jW)=1"WhenceA1(s)andA2(s)arebothallpass12s+afunctions.22bsjbW2bW5.13H1(s)=22.Thus,H1(jW)=22,henceH1(jW)=b2W2+(W2-W2)2.s+bs+WjbW+W-W0o0NowatW=0,H1(j0)=0andatW=¥,H1(j¥)=0andatW=Wo,H1(jWo)=1.Hence173
22bWc1H1(s)hasabandpassresponse.The3-dBfrequenciesaregivenby22222=.bW+(W-W)2c02222242224Thus,(W-W)=bWorW-(b+2W)W+W=0.HenceifWandWaretherootsocccoco124ofthisequation,thensoare–W,–W,andtheproductoftherootsisW.Thisimplies12o2222222WW=W.AlsoW+W=b+2W.Hence(W-W)=bwhichgivesthedesiredresult12o12o21W-W=b.212222222s+WW-W2(W-W)ooo5.14H(s)=.Thus,H(jW)=,hence,H(jW)=.222222222222s+bs+WW-W+jbW(W-W)+bWoooNow,H(j0)=1,H(j¥)=1andH(jW)=1.Hence,H2(s)hasabandstopresponse.Asin222o2theearlierproblemonecanshowthatWW=WandalsoW-W=b.12o21æ22öæ22ö1çs-bs+Wo÷11çs-bs+Wo÷5.15H(s)=1-={A(s)-A(s)}andH(s)=1+=12çs2+bs+W2÷21222çs2+bs+W2÷èoøèoø221s-bs+Wo{A(s)+A(s)}.Thus,A1(s)=1andA(s)=.Now2122s2+bs+W2o222222(W-W)+bWoA(jW)==1,andishenceA2(s)isanallpassfunction.A1(s)isseen222222(W-W)+bWotobeanallpassfunction.221dk(1/H(jW))W2N–ka5.16H(jW)=.=2N(2N–1)L(2N–k+1).Thereforea1+(W/W)2NdWkW2Ncck2k2d(1/H(jW))d(H(jW))aa=0fork=1,2,...,2N–1.or,equivalently,=0forkkdWdWW=0W=0k=1,2,...,2N–1.æ1öæ1ö5.1710log10ç2÷=–0.5,,whichyieldse=0.3493.10log10çè2÷ø=–30,whichyieldsè1+eøA221Ws81A–1999A=1000.Now,===3.8095238and===90.486576.Then,kWp2.1k1e0.3493log10(1/k1)90.4866fromEq.(5.33)wegetN===3.3684.HencechooseN=4astheorder.log10(1/k)3.8095p(N+2l-1)j5.18Thepolesaregivenbypl=Wce2N,l=1,2,K,N.Here,N=5andWc=1.Hencej6p/10j8p/10j10p/10p1=e=-0.3090+j0.9511,p2=e=-0.8090+j0.5878,p3=e=-1,j12p/10*j6p/10*p4=e=-0.8090-j0.5878=p2,andp5=e=-0.3090-j0.9511=p1.174
5.19FromEq.(5.39)TN(x)=2xTN-1(x)-TN-2(x)whereTN(x)isdefinedinEq.(5.38).Case1:x£1.MakinguseofEq.(5.38)inEq.(5.39)weget-1-1TN(x)=2xcos((N-1)×cos(x))-cos((N-2)×cos(x))-1-1-1-1=2xcos(Ncosx-cosx)-cos(Ncosx-2cosx)-1-1-1-1=2x[cos(Ncosx)cos(cosx)+sin(Ncosx)sin(cosx)]-1-1-1-1-[cos(Ncosx)cos(2cosx)+sin(Ncosx)sin(2cosx)]-1-1-1-1=2xcos(Ncosx)×cos(cosx)-cos(Ncosx)×cos(2cosx)2-1-12-1=2xcos(Ncosx)-cos(Ncosx)×[2cos(cosx)-1]-122-1=cos(Ncosx)[2x-2x+1]=cos(Ncosx).Case2:x>1.MakinguseofEq.(5.38)inEq.(5.39)weget-1-1TN(x)=2xcosh((N-1)×cosh(x))-cohs((N-2)×cosh(x)).Usingthetrigonometricidentitiescosh(A-B)=cosh(A)cosh(B)-sinh(A)sinh(B),sinh(2A)=2sinh(A)cosh(A),and2cosh(2A)=2cosh(A)-1,andfollowingasimilaralgebraasinCase1,wecanshow-1-1-1TN(x)=2xcosh((N-1)×cosh(x))-cohs((N-2)×cosh(x))=cosh(Ncoshx).æ1öæ1ö5.2010log10ç2÷=–0.5,,whichyieldse=0.3493.10log10ç2÷=–60,whichyieldsA2=è1+eøèAø21Ws81A–19991000.Now,===3.8095238and===90.486576..Then,kWp2.1k1e0.3493-1-1cosh(1/k1)cosh(90.486576)5.1983fromEq.(5.41)wegetN====2.5824.Wethuscosh-1(1/k)cosh-1(3.8095238)2.013chooseN=3astheorder.115.21FromProblem5.18solution,weobserve=3.8095238ork=0.2625,and=90.4865769kk1ork1=0.011051362.SubstitutingthevalueofkinEq.(5.52a)wegetk"=0.964932.Then,fromEq.(5.52b)wearriveatr=0.017534.SubstitutingthevalueofinEq.(5.52c)wegetor=0.017534.FinallyfromEq.(5.51)wearriveatN=2.9139.WechooseN=3.225.22FromEq.(5.53)B(s)=(2N–1)B(s)+sB(s),whereB1(s)=s+1andB2(s)=s+3s+NN–1N–2222323.Thus,B(s)=5B(s)+sB(s)=5(s+3s+3)+s(s+1)=s+6s+15s+15,and32123222432B(s)=7B(s)+sB(s)=7(s+6s+15s+15)+s(s+3s+3)=s+10s+45s+105s+105.4322432232Thus,B5(s)=9B4(s)+sB3(s)=9(s+10s+45s+105s+105)+s(s+6s+15s+15)5432=s+15s+105s+420s+945s+945,and175
25432B6(s)=11B5(s)+sB4(s)=11(s+15s+105s+420s+945s+945,)243265432+s(s+10s+45s+105s+105)=s+21s+210s+1260s+4725s+10395s+10395.5.23Weusethelowpass-to-highpasstransformationgiveninEq.(5.59)wherenowWp=2p(0.2)=0.4pandWˆp=2p(2)=4p.Therefore,fromEq.(5.59)wegetthedesiredWpWˆp1.6p215.791367transformationass®==.Therefore,sss24.524.52sHHP(s)=HLP(s)s®15.791367=2=2.sæ15.791367öæ15.791367ö4.52s+47.3741s+2.49367ç÷+3ç÷+4.52èsøèsø5.24Weusethelowpass-to-bandpasstransformationgiveninEq.(5.61)wherenowWp=2p(0.16)=0.32p,Wˆo=2p(3)=6p,andWˆp2-Wˆp1=2p(0.5)=p.Substitutingtheseæs2+36p2ös2+36p2valuesinEq.(5.59)wegetthedesiredtransformations®0.32pç÷=.èpsø3.125séæ22ö2ù0.056ês+36p+17.95úêçè3.125s÷øú22ëûThereforeHBP(s)=HLP(s)s®s+36p=23.125sæs2+36p2öæs2+36p2öç÷+1.06ç÷+1.13è3.125søè3.125sø420.056(s+49.61s+70695.62)=.432s+3.3125s+721.64667s+1176.95s+126242.1825.25Wˆ6,Wˆ6,aˆaˆp=2p(5)´10s=2p(0.5)´10p=0.3dBands=45dB.FromFigure5.14weæ1ö20.03observe10log10ç2÷=-0.3,hence,e=10-1=0.0715193,ore=0.26743.Fromè1+eøæ1ö24.5Figure5.14wealsonotethat10log10ç2÷=-45,andhenceA=10=31622.7766.UsingèAøethesevaluesinEq.(5.30)wegetk1==0,001503898.2A-1Todevelopthebandedgesofthelowpassprototype,wesetWp=1andobtainusingEq.(5.59)Wˆp5Wp1Ws===10.Next,usingEq.(5.29)weobtaink===0.1.SubstitutingtheWˆs0.5Ws10log10(1/k1)valuesofkandk1inEq.(5.33)wegetN==2.82278.WechooseN=3asthelog10(1/k)orderofthelowpassfilterwhichisalsotheorderofthehighpassfilter.ToverifyusingMATLABerusethestatement[N,Wn]=buttord(1,10,0.3,45,"s")whichyieldsN=3andWn=1.77828878.176
5.26Fˆ3,Fˆ3,Fˆ3,Fˆ3,ap1=20´10p2=45´10s1=10´10s2=60´10p=0.5dBandas=40dB.WeobserveFˆFˆ6=9´108,andFˆFˆ6=6´108.Sincep1p2=20´45´10s1s2=10´60´10FˆFˆFˆFˆFˆ3inwhichcases1s2¹p1p2,weadjustthethestopbandedgeonthelefttos1=15´10FˆFˆFˆFˆ2=9´108.Theangularcenterfrequencyofthedesiredbandpassfilteriss1s2=p1p2=Fo3thereforeWo=2pFo=2p´30´10.ThepassbandbandwidthisBWˆWˆ3.w=p2-p1=2p´25´10TodeterminethebanedgesoftheprototypelowpassfilterwesetWp=1andthenusingEq.Wˆ2-Wˆ2302-152W1os1p(5.62)weobtainWs=Wp==1.8.Thus,k===0.5555555556.Wˆs1Bw15´25Ws1.8æ1ö20.05Now,10log10ç2÷=-0.5,andhence,e=10-1=0.1220184543,ore=0.34931140019.è1+eøæ1ö24Inaddition10log10ç2÷=-40orA=10=10000.UsingthesevaluesinEq.(5.30)wegetèAøek1==0.00349328867069.FromEq.(5.52a)weget2A-12k"=1-k=0.831479419283,andthenfromEq.(5.52b)weget1-k"ro==0.02305223718137.SubstitutingthevalueofroinEq.(5.52c)wethenget2(1+k")r=0.02305225.Finally,substitutingthevaluesofrandk1inEq.(5.51)wearriveat2log10(4/k1)N==3.7795.WechooseN=4astheorderfortheprototypelowpassfilter.log10(1/r)Theorderofthedesiredbandpassfilteristherefore8.Usingthestatement[N,Wn]=ellipord(1,1.8,0.5,40,"s")wegetN=4andWn=1.5.27Fˆ6,Fˆ6,Fˆ6,andFˆ6,Weobservep1=10´10p2=70´10s1=20´10s2=45´10FˆFˆ12,andFˆFˆ12.SinceFˆFˆFˆFˆp1p2=700´10s1s2=900´10p1p2¹s1s2.weadjusttherightstopbandedgetoFˆ6,inwhichcaseFˆFˆFˆFˆFˆ2=700´1012.Thes2=35´10p1p2=s1s2=ostopbandbandwidthisBWˆWˆ6.w=s2-s1=2p´15´10177
æ1ö20.05Now,10log10ç2÷=-0.5,andhence,e=10-1=0.1220184543,ore=0.34931140019.è1+eøæ1ö23Inaddition10log10ç2÷=-30orA=10=1000.UsingthesevaluesinEq.(5.30)wegetèAøek1==0.01105172361656.2A-1TodeterminethebanedgesoftheprototypelowpassfilterwesetWs=1andthenusingEq.WˆWs1Bwp(5.65)weobtainWp=WsWˆ22=0.3157894.Therefore,k==0.3157894.o-Wˆs1Ws-1cosh(1/k1)Substitutingthevaluesofkandk1inEq.(5.41)wegetN=-1=2.856.Wechoosecosh(1/k)N=3astheorderfortheprototypelowpassfilter.Theorderofthedesiredbandstopfilteristherefore6.Usingthestatement[N,Wn]=cheb1ord(0.3157894,1,0.5,30,"s")wegetN=3andWn=0.3157894.5.28FromEq.(5.59),thedifferenceindBintheattenuationlevelsatWandWisgivenbypoæöW20Nlogço÷.HenceforW=2W,attenuationdifferenceindBisequalto20Nlog2=10çW÷op10èpø6.0206N.Likewise,forW=3W,attenuationdifferenceindBisequalto20Nlog3=op109.5424N.Finally,forW=4W,attenuationdifferenceindBisequalto20Nlog4=op1012.0412N.5.29TheequivalentrepresentationoftheD/AconverterofFigure5.39reducestothecircuitshownbelowifthel-thisONandtheremainingbitsareOFF,i.e.a=1,anda=0,k¹l.lkV0,lN-1ll-1l-20G22222L+aVlR–YinIntheabovecircuit,YinisthetotalconductanceseenbytheloadconductanceGLwhichisN-1iNgivenbyYin=å2=2-1.Theabovecircuitcanberedrawnasindicatedbelow:i=0V0,ll-1l-1G2Y-2Lin+aVlR–178
l-12UsingthevoltagedividerrelationwethengetV=aV.Usingthesuperposition0,lY+GlRiLtheorem,thegeneralexpressionfortheoutputvoltageV0isthusgivenbyNl-1NæRöV=å2aV=å2l-1açL÷V.0Y+GlRlç1+(2N-1)R÷Rl=1iLl=1èLø5.30TheequivalentrepresentationoftheD/AconverterofFigure5.40reducestothecircuitshownbelowiftheN-thbitisONandtheremainingbitsareOFF,i.e.aN=1,andak=0,k¹N.V0,NGGG22L+aVNR–whichsimplifiestotheoneshownbelowV0,NGG+GL22+aNVR–G2RLUsingthevoltage-dividerrelationwethengetV=aV=aV.0,NGGNR2(R+R)NR+G+L2L2TheequivalentrepresentationoftheD/AconverterofFigure5.40reducestothecircuitshownbelowifthe(N–1)-thbitisONandtheremainingbitsareOFF,i.e.aN–1=1,andak=0,k¹N.GV0,N-1GGGGL222+aVN-1R–Theabovecircuitsimplifiestotheoneshownbelow:GV0,N-1GGG+G222L+aVN-1R–ItsTheveninequivalentcircuitisindicatedbelow:V0,N-1GG+G22L+aN-1V2R–fromwhichwereadilyobtain179
G2aN-1RLaN-1V=V=V.0,N-1G+G2R2(R+R)2RLLFollowingthesameprocedurewecanshowthatifthel-thbitisONandtheremainingbitsareOFF,i.e.a=1,andak=0,k¹l,thenlRaLlV=V.0,l2(R+R)2N-lRLHence,ingeneralwehaveNRaLlV0=åN-lVR.2(R+R)2l=1L5.31Fromtheinput-outputrelationofthefirst-orderholdwegettheexpressionfortheimpulsed(nT)-d(nT-T)responseashf(t)=d(nT)+(t-nT),nT£t<(n+1)T.Intherange0£tK>3.1-K6.4-1-1Fromtheabovefigure,wegetW=KX+zW,W=(z-a)W,1321-1-1-1W=aW-bzW=(a-bz)W,andY=zW+bW.Substitutingthethirdequationin311121-1-1-1-2thefirstwegetW=KX+z(a-bz)W,or[1-az+bz]W=KX.Next,substituting111-1-1thesecondequationinthelastonewegetY=[z(z-a)+b]W.Fromthelasttwoequations1æ-1-2öYb-az+zwefinallyarriveatH(z)==Kç÷.Xç1-az-1+bz-2÷èø(a)Sincethestructureemploys4unitdelaystoimplementasecond-ordertransferfunction,itisnoncanonic.æ-1-2öæ2ö(b)and(c)WenextformH(z)H(z-1)=K2çb-az+z÷çb-az+z÷ç-1-2÷ç2÷è1-az+bzøè1-az+bzø189
æ-1-2öæ-2-1ö=K2çb-az+z÷çbz-az+1÷=K2.Therefore,H(ejw)=K,forallç-1-2÷ç-2-1÷è1-az+bzøèz-az+bzøjwvaluesofw.HenceH(e)=1ifK=1.(d)NoteH(z)isanallpasstransferfunctionwithaconstantmagnitudeatallvaluesofw.6.5W(z)X(z)k2-k1z-1-k2U(z)z-1a1a2Y(z)-1-1Analyzingthefigureweget(1):W(z)=X(z)-k1zU(z)+k2zW(z),-1-1-1-1(2):U(z)=-k2W(z)+zW(z)=(-k2+z)W(z),and(3):Y(z)=a1zU(z)+a2zW(z).-1-1-1SubstitutingEq.(2)inEq.(1)wegetW(z)=X(z)-k1z(-k2+z)W(z)+k2zW(z),or-1-21[1-(1+k1)k2z+k1z]W(z)=X(z),or(4):W(z)=-1-2X(z).1-(1+k1)k2z+k1z-1-k2+zSubstitutingEq.(4)inEq.(2)weget(5):U(z)=X(z).Finally,-1-21-(1+k1)k2z+k1z-1-2(a2-a1k2)z+a1zsubstitutingEqs.(4)and(5)inEq.(3)wegetY(z)=X(z),or-1-11-(1+k1)k2z+k1z-1-2(a2-a1k2)z+a1zH(z)=.Forstabilitywemusthavek<1,and-1-111-(1+k1)k2z+k1zk(1+k)<1+k,ork<1.21126.6S0(z)S4(z)a4X(z)Y(z)a3a1–1z–1–1S2(z)–1S3(z)–1S5(z)z–1–1za2S1(z)-1-1-1AnalysisyieldsS(z)=X(z)-S(z),S(z)=zS(z)+zS(z),S(z)=aS(z)-zS(z),011252302190
-1-1-1-1S(z)=azS(z)-zS(z),S(z)=S(z)+zS(z),S(z)=aS(z)-zS(z),and33534035145Y(z)=aS(z).EliminatingS0(z),S1(z),S2(z),S3(z),S4(z)andS5(z)fromtheseequationswe44getaftersomealgebraY(z)a(1+z-1)34H(z)==.X(z)1+(3+a+a)z-1+(3+2a+2a-aa)z-2+(1+a+a-aa-aaa)z-313131213121236.7–1X(z)W(z)z–1W(z)13b2R1(z)a1R2(z)a2a3a0b1b3z–1W2(z)z–1Y(z)–1-1-1AnalysisyieldsY(z)=aX(z)+bzR(z),W(z)=X(z)-bzR(z),011122-1-1-1R(z)=W(z)+abzR(z),W(z)=bzR(z)-bzW(z),1111121133-1-1-1R(z)=W(z)+abzR(z),W(z)=bzR(z)+abzW(z).22222322333-1FromthethirdequationwegetW(z)=(1-abz)R(z).Fromthesixthequationweget1111bz-1R(z)W(z)222W(z)=.FromthefifthequationwegetR(z)=.31-abz-121-abz-13322-1-1RewritingthefourthequationwegetW(z)-bzW(z)=bzR(z),inwhichwesubstitute23311theexpressionsforW3(z)andW1(z)resultinginìïbbz-1üï-123-1R(z)í(1-abz)+ý=bzR(z),or2îï22(1-abz-1)þï1133bz-1(1-abz-1)133R(z)=R(z).2bbz-1+(1-abz-1)(1-abz-1)1232233-1-1CombiningW(z)=X(z)-bzR(z),W(z)=(1-abz)R(z).andmakinguseofthe1221111expressionforR2(z)wearriveatbbz-2+(1-abz-1)(1-abz-1)232233R(z)=X(z).1bbz-2(1-abz-1)+(1-abz-1)(1-abz-1)(1-abz-1)+bbz-2(1-abz-1)23111122331233-1SubstitutingtheaboveinY(z)=aX(z)+bzR(z),wefinallyget011Y(z)bbbz-2+bz-1(1-abz-1)(1-abz-1)12312233H(z)==a+.X(z)0(1-abz-1)(1-abz-1)(1-abz-1)112233-2-1-2-1+bbz(1-abz)+bbz(1-abz)23111233191
6.8a0X(z)Y(z)Wb1W(z)b3a10(z)2–1–1zz–1–1zz–1b0W1(z)b2W3(z)a2a3-1-1-1AnalysisyieldsW(z)=X(z)+bzW(z),W(z)=bzW(z)+bzW(z),01110022-1-1-1-1W(z)=bzW(z)+bzW(z),W(z)=bzW(z)-bzW(z).2113332233bz-12FromtheseequationswegetW(z)=W(z),and31+bz-123æ1+bz-1-bbz-2öæbz-1(1+bz-1)öç323÷W(z)=bz-1W(z),Or,W(z)=ç13÷W(z),çè1+bz-1÷ø2112çè1+bz-1-bbz-2÷ø13323æbz-1(1+bz-1-bbz-2)öInaddition,W(z)=ç0323÷W(z).1çè1+bz-1-(bb+bb)z-2-bbbz-3÷ø032312123æbbz-2(1+bz-1-bbz-2)öNow,W(z)ç1-01323÷=X(z),Hence,0çè1+bz-1-b(b+b)z-2-bbbz-3÷ø3213123æ1+bz-1-b(b+b)z-2-bbbz-3öW(z)=ç3213123÷X(z),0çè1+bz-1-(bb+bb+bb)z-2-bb(b+b)z-3+bbbbz-4÷ø312230113200123æbz-1(1+bz-1-bbz-2)öW(z)=ç0323÷X(z),1çè1+bz-1-(bb+bb+bb)z-2-bb(b+b)z-3+bbbbz-4÷ø312230113200123æbbz-2(1+bz-1)öW(z)=ç103÷X(z),2çè1+bz-1-(bb+bb+bb)z-2-bb(b+b)z-3+bbbbz-4÷ø312230113200123æbbbz-3öW(z)=ç012÷X(z).3çè1+bz-1-(bb+bb+bb)z-2-bb(b+b)z-3+bbbbz-4÷ø312230113200123-1-1-1-1Finally,Y(z)=abzW(z)+abzW(z)+abzW(z)+abzW(z).Substitutingthe300011222133expressionsforW0(z),W1(z),W2(z)andW3(z)intheaboveweget-1-2-3abz+(abb+abb)z+(abbb+abbb-abbb-abbb)z303300100013212032103023Y(z)+(-a+a-a+a)bbbbz-332010123H(z)==X(z)1+bz-1-(bb+bb+bb)z-2-bb(b+b)z-3+bbbbz-4312230113200123192
6.9W(z)1U(z)-k11X(z)1-z-1-1Y(z)1-zk1k21Analysisyields(1):W(z)=X(z)+k1Y(z),(2):U(z)=-1W(z)+k2Y(z),and1-z-k1(3):Y(z)=U(z).SubstitutingEq.(2)inEq.(3)weget-11-z-k1æ1ök1k1k2(4):Y(z)=-1çè-1W(z)+k2Y(z)÷ø=--12W(z)--1Y(z).Substituting1-z1-z(1-z)1-zk1k1k2Eq.(1)inEq.(4)wethengetY(z)=--12[X(z)+k1Y(z)]--1Y(z)(1-z)1-zkkék-1ù111+k2-k2z=-X(z)-êúY(z),or-12-1-1(1-z)1-zêë1-zúûék-1)ùk1(k1+k2-k2z1ê1+úY(z)=-X(z).Hence,-12-12êë(1-z)úû(1-z)Y(z)k1H(z)==-.X(z)1+k-1+z-2[1(k1+k2)]-(2+k1k2)z6.10(a)Adirectformrealizationof-1-2-3-4-5H(z)=1-3.5z+4.9z-3.43z+1.2005z-0.16807zanditstransposedstructureaareshownbelow:x[n]z-1z-1z-1-1-1zz–3.54.9–3.431.2005–0.16807y[n]z-1z-1z-1z-1z-1y[n]–0.168071.2005–3.434.9–3.5x[n](b)Acascaderealizationof-1-1-1-1-1H(z)=(1-0.7z)(1-0.7z)(1-0.7z)(1-0.7z)(1-0.7z)isshownbelow:–0.7–0.7–0.7–0.7–0.7x[n]y[n]z-1z-1z-1z-1z-1-1-1-2-1-2(c)AcascaderealizationofH(z)=(1-0.7z)(1-1.4z+0.49z)(1-1.4z+0.49z)isshownbelow:193
–0.70.490.49x[n]z-1z-1z-1z-1z-1y[n]–1.4–1.4-1-2-3-1-2(d)AcascaderealizationofH(z)=(1-2.1z+1.47z-0.343z)(1-1.4z+0.49z)isshownbelow:–0.3430.49x[n]z-1z-1z-1z-1z-1y[n]–2.10.98–1.4-3-6-1-3-6-2-36.11(a)H(z)=(h[0]+h[3]z+h[6]z)+z(h[1]+h[4]z+h[7]z)+z(h[2]+h[5]z).-3-6-3-6-3Hence,E(z)=h[0]+h[3]z+h[6]z,E(z)=h[1]+h[4]z+h[7]z,E(z)=h[2]+h[5]z.012(b)FigureissameasinFigure6.9withh[8]=0.-2-4-6-1-2-4-66.12(a)H(z)=(h[0]+h[2]z+h[4]z+h[6]z)+z(h[1]+h[3]z+h[5]z+h[7]z).-2-4-6-2-4-6Hence,E(z)=h[0]+h[2]z+h[4]z+h[6]z,E(z)=h[1]+h[3]z+h[5]z+h[7]z.01(b)h[1]h[3]h[5]h[7]X(z)z-2z-2z-2z-1Y(z)h[4]h[6]h[2]h[0]-4-1-4-2-4-3-46.13(a)H(z)=(h[0]+h[4]z)+z(h[1]+h[5]z)+z(h[2]+h[6]z)+z(h[3]+h[7]z).-4-4Hence,E(z)=h[0]+h[4]z,E(z)=h[1]+h[5]z,01-4-4E(z)=h[2]+h[6]z,E(z)=h[3]+h[7]z.23194
(b)h[3]h[7]z-4h[6]h[2]h[5]z-1z-1z-1h[1]h[4]h[0]6.14x[n]z-1z-1z-1z-1-1-1-1-1-1z-1z-1-1zzh[0]h[1]h[2]h[3]y[n]6.15x[n]z-1z-1-1-1zzz-1-1-1-1-1-1z-1z-1z-1-1zh[0]h[1]h[2]h[3]h[4]y[n]6.16ConsiderfirstthecaseofN=4.-1-1-1-1-1-1-1-2H(z)=b0+b1z(1++b2z(1+b3z(1+b4z)))=b0+b1z(1++b2z(1+b3z+b3b4z))-1-1-2-3=b0+b1z(1+b2z+b2b3z+b2b3b4z)-1-2-3-4-1-2-3-4=b+bz+bbz+bbbz+bbbbz=h[0]+h[1]z+h[2]z+h[3]z+h[4]z.01121231234-1Comparinglikepowersofzonbothsides,weobtainh[0]=b,h[1]=b,h[2]=bb,0112h[2]h[3]=bbb,h[4]=bbbb.Solvingtheseequationswegetb=h[0],b=h[1],b=,1231234012h[1]h[3]h[4]b=,andb=.Inthegeneralcase,wehave3h[2]4h[3]h[k]b=h[0],b=h[1],andb=,2£k£N.01kh[k-1]AnestedrealizationofH(z)forN=7isshownbelow:195
b1b2b3b4b5b6b7x[n]y[n]z-1z-1z-1z-1z-1z-1z-1b0-N/26.17G(z)=z-H(z).AcanonicrealizationofbothG(z)andH(z)isasindicatedonnextpageforN=8.z-1z-1z-1z-1G(z)z-1-1-1-1-1zzzh[0]h[1]h[2]h[3]h[4]H(z)6.18Withoutanylossofgenerality,assumeM=5whichmeansN=11.InthiscasethetransferfunctionisgivenbyH(z)=-5-12-23-34-45-5z[h[5]+h[4](z+z)+h[3](z+z)+h[2](z+z)+h[1](z+z)+h[0](z+z)].Now,therecursionrelationfortheChebyshevpolynomialisgivenbyTr(x)=2xTr-1(x)-Tr-2(x),forr³2withT0(x)=1andT1(x)=x.Hence,223T2(x)=2xT1(x)-T0(x)=2x-1,T3(x)=2xT2(x)-T1(x)=2x(2x-1)-x=4x-3x,3242T4(x)=2xT3(x)-T2(x)=2x(4x-3x)-(2x-1)=8x-8x+1,3242T4(x)=2xT3(x)-T2(x)=2x(4x-3x)-(2x-1)=8x-8x+1,Wecanthusrewritetheexpressioninsidethesquarebracketsgivenaboveasæz+z-1öæz+z-1öæz+z-1öh[5]+2h[4]T1ç÷+2h[3]T2ç÷+2h[2]T3ç÷è2øè2øè2øæz+z-1öæz+z-1ö+2h[1]T4ç÷+h[0]T5ç÷è2øè2øæ-1öéæ-1ö2ùéæ-1ö3æ-1öùz+zz+zz+zz+z=h[5]+2h[4]ç÷+2h[3]ê2ç÷-1ú+2h[2]ê4ç÷-3ç÷úè2øêè2øúêè2øè2øúëûëûéæ-1ö4æ-1ö2ùéæ-1ö5æ-1ö3æ-1öù+2h[1]ê8z+z-8z+z+1ú+2h[0]ê16z+z-20z+z+5z+zúêçè2÷øçè2÷øúêçè2÷øçè2÷øçè2÷øúëûëû196
5æ-1önz+z=åa[n]ç÷,wherea[0]=h[5]-2h[3]+2h[1],a[1]=2h[4]-6h[2]+10h[0],è2øn=0a[2]=4h[3]-16h[1],a[3]=8h[2]-40h[1],a[4]=16h[1],anda[5]=32h[0].é5æ-1önùæ-2öArealizationofH(z)=z-5êa[n]z+zú=a[0]z-5+a[1]z-41+zåç÷ç÷ên=0è2øúè2øëûæ-2ö2æ-2ö3æ-2ö4æ-2ö5-31+z-21+z-11+z1+z+a[2]zç÷+a[3]zç÷+a[4]zç÷+a[5]ç÷è2øè2øè2øè2øisthusasshownbelow:P(z)P1(z)P2(z)P3(z)6.19ConsiderH(z)==××.AssumeallzerosofP(z)andD(z)arecomplex.D(z)D1(z)D2(z)D3(z)Notethatthenumeratorofthefirststagecanbeoneofthe3factors,P1(z),P2(z),andP3(z),thenumeratorofthesecondstagecanbeoneoftheremaining2factors,andthenumeratorofthethirdstageistheremainingfactor.Likewise,thedenominatorofthefirststagecanbeoneofthe3factors,D1(z),D2(z),andD3(z),thedenominatorofthesecondstagecanbeoneoftheremaining2factors,andthedenominatorofthethirdstageistheremainingfactor.Hence,2thereare(3!)=36differenttypesofcascaderealizations.IfthezerosofP(z)andD(z)areallreal,thenP(z)has6realzerosandD(z)has6realzeros.In2thiscasethenthereare(6!)=518400differenttypesofcascaderealizations.KPi(z)æKö6.20H(z)=Õ.Herethenumeratorofthefirststagecanbechoseninçè1÷øways,theD(z)i=1iæK-1önumeratorofthesecondstagecanbechoseninç÷ways,anduntilthethereisonlyè1øpossiblechoiceforthenumeratoroftheK-thstage.Likewise,thedenominatorofthefirstæKöstagecanbechoseninç÷ways,thedenominatorofthesecondstagecanbechoseninè1øæK-1öç÷ways,anduntilthethereisonlypossiblechoiceforthedenominatoroftheK-thstage.è1øHencethetotalnumberofpossiblecascaderealizationsareequaltoæKö2æK-1ö2æK-2ö2æ2ö2æ1ö22ç÷ç÷ç÷Lç÷ç÷=(K!)è1øè1øè1øè1øè1ø-1-23+4z-2z6.21AcanonicdirectformIIrealizationofH(z)=isshownonnextpage:1+3z-1+5z-2+4z-4197
34–2x[n]z–1z–1z–1z–1z–1y[n]–3–5–4AcanonicdirectformIIrealizationofH(z)isasindicatedbelow:t34–2x[n]z–1z–1z–1z–1z–1y[n]–4–5–3æ0.3-0.5z-1öæ2+3.1z-1ö6.22(a)AcascaderealizationofH(z)=ç÷ç÷isshownbelow:1çè1+2.1z-1-3z-2÷øçè1+0.67z-1÷ø0.3–0.523.1–1–1–1x[n]zzzy[n]–2.13–0.67æ2+3.1z-1öæ0.3-0.5z-1öAcascaderealizationofH(z)=ç÷ç÷isshownbelow:1çè1+2.1z-1-3z-2÷øçè1+0.67z-1÷ø23.10.3–0.5x[n]–1–1–1y[n]zzz–2.13–0.67æ1öç4.1z(z-)÷æ2ö3z+0.4(b)AcascaderealizationofH(z)=ç÷ç÷2ç(z+0.3)(z-0.5)÷çèz2-z-0.1÷øç÷èøæ4.1-1.3667z-1öæ1+0.4z-2ö=ç÷ç÷isshownbelow:çè1-0.2z-1-0.15z-2÷øçè1-z-1+0.1z-2÷ø4.1–4.1/30.4x[n]–1z–1–1–1y[n]zzz0.20.15–0.1198
æ1+0.4z-2öæ4.1-1.3667z-1öAcascaderealizationofH(z)=ç÷ç÷isshownbelow:2çè1-0.2z-1-0.15z-2÷øçè1-z-1+0.1z-2÷øy[n]4.1–4.1/30.4x[n]–1–1–1–1zzzz0.20.15–0.1æ3-2.1z-1öæ2.7+4.2z-1-5z-2ö(c)AcascaderealizationofH(z)=ç÷ç÷isshownbelow:3çè1+0.52z-1÷øçè1+z-1-0.34z-2÷ø32.74.2–2.1–5x[n]z–1z–1z–1y[n]–0.52–10.34æ2.7+4.2z-1-5z-2öæ3-2.1z-1öAcascaderealizationofH(z)=ç÷ç÷isshownbelow:3çè1+0.52z-1÷øçè1+z-1-0.34z-2÷øy[n]2.74.23–2.1–5x[n]–1–1–1–1zzzz–0.52–10.34-16.23(a)Apartial-fractionexpansionofH(z)inzobtainedusingtheM-fileresiduezgives1-1-20.6-0.07z-1.55z0.44540.15710.3117H(z)==-+whose11+2.77z-1-1.593z-2-2.01z-31+3.0755z-11-0.9755z-11+0.67z-1realizationyieldstheParallelFormIstructureshownbelow:0.4454–1z–3.0755–0.1571x[n]y[n]–1z0.97550.3117–1z–0.67199
Apartial-fractionexpansionofH(z)inzobtainedusingtheM-fileresiduegives1320.6z-0.07z-1.55z-1.36990.1533-2088H(z)==0.6+++1z3+2.77z2-1.593z-2.01z+3.0755z-0.9755z+0.67-1-1-1-1.3699z0.1533z-2088z=0.6+++,whoserealizationyieldstheParallelFormII1+3.0755z-11-0.9755z-11+0.67z-1structureshownbelow:0.6–1z–1.3699–3.0755x[n]z–1y[n]–0.15330.9755–1z–0.2088–0.67-1(b)Apartial-fractionexpansionofH(z)inzobtainedusingtheM-fileresiduezgives24324.1z-1.3667z+1.64z-0.5467zH2(z)=432z-1.2z+0.15z+0.13z-0.0157.5709-3.70143.2458-3.0153=+++whoserealizationyieldstheParallel-1-1-1-11-0.8873z1-0.5z1+0.3z1-0.1127zFormIstructureshownbelow:7.5709-1z0.8873–3.7014x[n]z-1y[n]0.53.2458z-1–0.3–3.0153-1z0.1127200
Apartial-fractionexpansionofH(z)inzobtainedusingtheM-fileresiduegives26.7176-1.8507-0.9737-0.3398H(z)=4.1++++1z-0.8873z-0.5z+0.3z-0.1127-1-1-1-16.7176z-1.8507z-0.9737z-0.3398z=4.1++++whoserealizationyieldsthe1-0.8873z-11-0.5z-11+0.3z-11-0.1127z-1ParallelFormIIstructureshownbelow:4.16.7176-1z0.8873–1.8507x[n]z-1y[n]0.5–0.9737-1z–0.3–0.3398-1z0.1127-1(c)Apartial-fractionexpansionofH(z)inzobtainedusingtheM-fileresiduezgives3-1-2-38.1+6.93z-23.82z+10.5z-24.243477.044914.6876H3(z)=-1-2-3=-59.3891+-1+-1+-11+1.52z+0.18z-0.1768z1+1.2681z1+0.52z1-0.2681zwhoserealizationyieldstheParallelFormIstructureshownbelow:–59.3891–24.2434–1.2681z-177.0449x[n]y[n]-1–0.52z14.68760.2681z-1201
Apartial-fractionexpansionofH(z)inzobtainedusingtheM-fileresiduegives3-1-1-130.7434-40.06333.93830.7434z-40.0633z3.938zH(z)=8.1+++=8.1+++3z+1.2681z+0.52z-0.26811+1.2681z-11+0.52z-11-0.2681z-1whoserealizationyieldstheParallelFormIIstructureshownbelow:8.130.7434-1z–1.2681–40.0633x[n]z-1y[n]–0.523.938-1z0.26816.24Acascaderealizationbasedonthefactorizationæz-1öæ0.44+0.362z-1+0.02z-2öH(z)=ç÷ç÷isindicatedbelow:çè1+0.8z-1+0.5z-2÷øçè1-0.4z-1÷ø0.440.3620.02x[n]–1–1–1–1y[n]zzzz–0.8–0.50.4-1-1-1-22+0.1z3+0.2z16+0.7z+0.02z6.25(a)H(z)=××=.-1-1-1-1-2-31+0.4z1-0.3z1-0.2z1-0.1z-0.14z+0.024z(b)y[n]=6x[n]+0.7x[n-1]+0.02x[n-2]+0.1y[n-1]+0.14y[n-2]-0.024y[n-3].(c)AcascaderealizationofH(z)isshownbelow:230.10.2x[n]-1-1y[n]zz-1–0.40.30.2z(d)AparallelformIrealizationofH(z)isobtainedbymakingapartial-fractionexpansionin1.666711-6.6667usingM-FileresiduezH(z)=+,1+0.4z-11-0.3z-11-0.2z-1202
whoserealizationisshownbelow:1.6667-1z–0.411x[n]y[n]-1z0.3–6.6667-10.2znnn(e)h[n]=1.6667(-0.4)m[n]+11(0.3)m[n]-6.6667(0.2)m[n].-1-110.3z1-0.3z16.26(a)Y(z)=-=.X(z)=.Thus,1-0.4z-11-0.4z-11-0.4z-11-0.2z-1-1-1-1-2Y(z)(1-0.3z)(1-0.2z)1-0.5z+0.06zH(z)===.X(z)1-0.4z-11-0.4z-1(b)y[n]=x[n]-0.5x[n-1]+0.06x[n-2]+0.4y[n-1].(c)–0.50.06x[n]z-1z-1y[n]0.4-1(d)Apartial-fractionexpansionofH(z)inzobtainedusingM-fileresiduezisgiven-10.125byH(z)=0.875z-0.15+whoserealizationyieldstheParallelFormIstructureas1-0.4z-1indicatedbelow:–0.150.125x[n]-1y[n]z0.40.875-1zn(e)Theinversez-transformofH(z)yieldsh[n]=0.875d[n-1]-0.15d[n]+0.125(0.4)m[n].203
-1-110.4z1-0.4z(f)X(z)=-=.Therefore1-0.3z-11-0.3z-11-0.3z-1æ(1-0.3z-1)(1-0.2z-1)öæ1-0.4z-1öY(z)=H(z)X(z)=ç÷ç÷=1-0.2z-1,whoseinverseçè1-0.4z-1÷øçè1-0.3z-1÷øz-transformyieldsy[n]=d[n]-0.2d[n-1].6.27FigureP6.11canbeseentobeaParallelFormIIstructure.Apartial-fractionexpansionof23z+18.5z+17.5H(z)=inzobtainedusingtheM-fileresidueisgivenby(z+0.5)(z+2)56H(z)=3++.Comparingthecoefficientsoftheexpansionwiththecorrespondingz+2z+0.5multipliercoefficientsinFigureP6.11weconcludethatthemultipliercoefficient2shouldbereplacedwith6andthemultipliercoefficient0.5shouldbereplacedwith–0.6.6.28FigureP6.11canbeseentobeaParallelFormIstructure.Apartial-fractionexpansionof-13z(5z-1)3.75-1.5z-1H(z)==inzobtainedusingtheM-fileresiduezis(z+0.5)(4z+1)1+0.75z-1+0.125z-213.59.5givenbyH(z)=-.Comparingthecoefficientsoftheexpansionwiththe1+0.5z-11+0.25z-1correspondingmultipliercoefficientsinFigureP6.11weconcludethatthemultipliercoefficientA=13.5andthemultipliercoefficientB=–0.25.Y(z)1+a+b6.29ThedifferenceequationcorrespondingtothetransferfunctionH(z)==X(z)1+az-1+bz-2isgivenbyy[n]+ay[n-1]+by[n-2]=(1+a+b)x[n],whichcanberewrittenintheformy[n]=x[n]+a(x[n]-y[n-1])+b(x[n]-y[n-2]).ArealizationofH(z)basedonthisequationisthusasshownbelow:6.30(a)Thedifferenceequationcorrespondingtothetransferfunction-1-2Y(z)(1+a1+a2)(1+2z+z)H(z)==isgivenbyX(z)1-az-1+az-212y[n]+ay[n-1]-ay[n-2]=(1+a+a)(x[n]+2x[n-1]+x[n-2]),whichcanberewrittenas1212y[n]=(x[n]+2x[n-1]+x[n-2])+a(y[n-1]-x[n]-2x[n-1]-x[n-2])1-a(y[n-2]-x[n]-2x[n-1]-x[n-2]).Denotingw[n]=x[n]+2x[n-1]+x[n-2],2thedifferenceequationrepresentationbecomesy[n]=w[n]+a(y[n-1]-w[n])-a(y[n-2]-w[n]).ArealizationofH(z)basedonthelasttwo12204
equationsisasindicatedbelowwherethefirststagerealizesw[n]whilethesecondstagerealizesy[n].Aninterchangeofthetwostagesleadstoanequivalentrealizationshownbelow:Finally,bydelaysharingtheabovestructurereducestoacanonicrealizationasshownbelow:(b)Thedifferenceequationcorrespondingtothetransferfunction-2Y(z)(1-a2)(1-z)H(z)==isgivenbyX(z)1-az-1+az-212y[n]-ay[n-1]+ay[n-2]=(1-a)(x[n]-x[n-2])whichcanberewrittenas122y[n]=ay[n-1]-ay[n-2]+x[n]-ax[n]-x[n-2]-ax[n-2]1222=ay[n-1]-a(x[n]-x[n-2]+y[n-2])+(x[n]-x[n-2]).Denotingw[n]=x[n]-x[n-2],we12canrewritethelastequationasy[n]=ay[n-1]-a(w[n]+y[n-2])+w[n].Arealizationof12H(z)basedonthelasttwoequationsisasshownbelow:Aninterchangeofthetwostagesleadstoanequivalentrealizationshownbelow:205
Finally,bydelaysharingtheabovestructurereducestoacanonicrealizationasshownbelow:YC+DH(z)1N-16.31(a)FromthestructureofFigureP.6/14itfollowsthatH(z)==,fromNXA+BH(z)1N-1C-AH(z)NwhichwegetH(z)=.SubstitutingtheexpressionforHN(z)wethenarriveN-1BH(z)-DNæNöæNöCç1+ådz-i÷-Açåpz-i÷çi÷çi÷èi=1øèi=0øatH(z)=N-1æNöæNöBçåpz-i÷-Dç1+ådz-i÷çi÷çi÷èi=0øèi=1ø(C-Ap)+(Cd-Ap)z-1+L++(Cd-Ap)z-N+1++(Cd-Ap)z-N011N-1N-1NN=.(Bp-D)+(Bp-Dd)z-1+L+(Bp-Dd)z-N+1+(Bp-Dd)z-N011N-1N-1NNSubstitutingthevaluesA=1,B=dNz–1,C=p0,andD=pNz–1,wegetHN–1(z)(p-p)+(pd-p)z-1+L+(pd-p)z-N+1+(pd-p)z-N000110N-1N-10NN=(dp-p)z-1+(dp-pd)z-2+L+(dp-pd)z-N+(dp-pd)z-N-1N0NN1N1NN-1NN-1NNNN(pd-p)+(pd-p)z-1+L+(pd-p)z-N+2+(pd-p)z-N+10110220N-1N-10NN=(pd-p)+(pd-pd)z-1+L+(pd-pd)z-N+10NN1NN1N-1NNN-1p"+p"z-1+L+p"z-N+2+p"z-N+101N-2N-1=where1+d"z-1+L+d"z-N+11N-1pd-ppd-pdp"=kk+1k+1,kNNkk=0,1,K,N-1,andd"=,k=1,2,K,N-1.kpd-pkpd-p0NN0NNC(b)Fromthechainparameters,weobtainforthefirststaget==p,11A0AD-BC-11B-1t==(p-pd)z,t==1,andt=-=-dz.The12AN0N21A22ANcorrespondinginput-outputrelationsarethengivenby206
-1-1-1Y=pX+(p-pd)z=p(X-dzX)+pzX,101N0N01N2N2-1Y=X-dzX.Substitutingthesecondequationintothefirstwerewriteitas21N2-1Y=pY+pzX.Arealizationofthetwo-pairbasedonthelasttwoequationsis102N2thereforeasindicatedbelow:Exceptforthefirststage,allotherstagesrequire2multipliers.HencethetotalnumberofmultipliersneededtoimplementanN-thordertransferfunctionHN(z)is2N+1.Thetotalnumberoftwo-inputaddersrequiredis2NwhiletheoverallrealizationiscanonicrequiringNdelays.-1-2-30.44z+0.362z+0.02z6.32FromH3(z)=-1-2-3,usingEq.(6.135)wearriveat1+0.4z+0.18z-0.2z-1-2-122+18.1z+z1.0593+zH(z)=.RepeatingtheprocedureweobtainH(z)=.2-1-21-11+4.8z+8.8z1+0.7746zFromH3(z),H2(z)andH1(z)wethenarriveatthecascadedlatticerealizationofH3(z)asshownbelow:0.2–3.81.05930.4422–0.7746z–1z–1z–10.02-1-20.6-0.07z-1.55z6.33(a)FromH(z)=(Notethechangeinthelabellingofthe31+2.77z-1-1.593z-2-2.01z-3-1-2-1.4362-0.4927z+ztransferfunction)usingEq.(6.135)wearriveatH(z)=.21-0.1167z-1-2.5833z-2-10.2436+zRepeatingtheprocedureweobtainH(z)=.FromH3(z),H2(z)andH1(z)we11+0.5127z-1thenarriveatthecascadedlatticerealizationofH3(z)asshownbelow:x[n]2.012.5833–0.51270.6–1.43620.2436y[n]–1–1–1zzz207
-1-2-34.1-1.3667z+1.64z-0.5467z(b)FromH(z)=(Notethechangeinthelabelling41-1.2z-1+0.15z-2+0.13z-3-0.015z-4ofthetransferfunction)usingEq.(6.135)wearriveat-1-2-357.7772+16.6667z-17.5561z+zH(z)=.Repeatingtheprocedureweobtain31-0.3333z-1+0.4z-2-0.1333z-3-1-2-14.1284-4.6735z+z-2.8997+zH(z)=,andH(z)=.FromH(z),H3(z),H2(z)21+0.2170z-1-0.2230z-221-0.4296z-14andH1(z)wethenarriveatthecascadedlatticerealizationofH3(z)asshownbelow:x[n]0.0150.13330.2230.42960.657.77724.1284–2.8997–1–1–1–1zzzzy[n]-1-2-38.1+6.93z-23.82z+10.5z(c)FromH(z)=usingEq.(6.135)wearriveat31+1.52z-1+0.18z-2-0.1768z-3-1-2-1-0.4511-2.1185z+z2.7827+zH(z)=.RepeatingtheprocedureweobtainH(z)=.21+1.4403z-1-3.3873z-221+10.866z-1FromH3(z),H2(z)andH1(z)wethenarriveatthecascadedlatticerealizationofH3(z)asshownbelow:x[n]0.17683.3873–10.8668.1–0.45112.7827y[n]–1–1–1zzz6.34WhenHN(z)isanallpasstransferfunctionoftheform–1–Nd+dz+L+zNN-1H(z)=A(z)=,thenfromEq.(6.135a),thenumeratorcoefficientsNN–1–N1+dz+L+dz1Npd-pdd-d0k+1k+1Nk+1N–k–1ofHN–1(z)aregivenbyp"==,andfromEq.(6.135b)thekpd-pd2-10NNNdenominatorcoefficientsofHN–1(z)aregivenbypd-ddd-dN-k-1NN-k-1k+1NN–k–1d"===p",implyingHN–1(z)isanallpassN-k-1pd-pd2-1k0NNNtransferfunctionoforderN–1.SinceherepN=1andp0=dN,thelatticestructureofProblem6.31thenreducestothelatticestructureemployedintheGray-Markelrealizationprocedure.208
6.35(a)ConsidertherealizationofType1Ballpasstransferfunction.Fromitstransferparameters-1-1-1giveninEq.(6.62b)wearriveatY=zX+(1+z)X=z(X+X)+X,and112122-1-1-1Y=(1-z)X-zX=X-z(X+X).Arealizationofthetwo-pairbasedonthesetwo212112equationsisasshownbelowwhichleadstothestructureofFigure6.36(b).(b)FromthetransferparametersofType1AtallpassgiveninEq.(6.62c)weobtain-1-2-1-1-1-1Y=zX+X,andY=(1-z)X-zX=X-z(zX+X)=X-zY.A11221211211realizationofthetwo-pairbasedonthesetwoequationsisasshownbelowwhichleadstothestructureofFigure6.36(c).(c)FromthetransferparametersofType1BtallpassgiveninEq.(6.62d)weobtain-1-1-1Y=zX+(1-z)X=z(X-X)+X,and112122-1-1-1Y=(1+z)X-zX=X+z(X-X).Arealizationofthetwo-pairbasedonthesetwo212112equationsisasshownbelowwhichleadstothestructureofFigure6.36(d).6.36(a)AcascadeconnectionofthreeType1Afirst-orderallpassnetworksisashownbelowwhichisseentorequire6delays:Simpleblock-diagrammanipulationsresultsinthestructureshownbelow:Bydelay-sharingbetweenadjacentallpasssectionswearriveatthefollowingequivalentrealizationrequiringnow4delays.(b)AcascadeconnectionofthreeType1Atfirst-orderallpassnetworksisashownbelowwhichisseentorequire6delays:209
Bydelay-sharingbetweenadjacentallpasssectionswearriveatthefollowingequivalentrealizationrequiringnow4delays.6.37AnalyzingthestructuctureofFigureP6.14weobtain–1–1zW(z)aX(z)Y(z)U(z)–1-1-1(1):W(z)=X(z)-zU(z),(2)U(z)=aW(z)+zU(z),and(3):Y(z)=-W(z)+U(z).aFromEq.(2)weobtain(4):U(z)=W(z).SubstitutingEq.(4)inEq.(3)weget-11-zæ1-a-z-1ö(5):Y(z)=-ç-1÷W(z).SubstitutingEq.(4)inEq.(1)wegetè1-zøæ1-z-1+az-1ö(6):X(z)=ç-1÷W(z).FromEqs.(5)and(6)wefinallygetè1-zø-1Y(z)-(1-a)+zH(z)==.X(z)1-(1-a)z-1-1-2dd+dz+z1216.38WerealizeA(z)=intheformofaconstrainedthree-pairasindicated2-1-21+dz+ddz112below:210
Y2X1étùê11t12t13úêt21t22t23údêú1ët31t32t33ûY1X2X3Y3–d2éYùétttùéXùê1úê111213úê1úFromtheabovefigure,wehaveêY2ú=êt21t22t23úêX2ú,andX2=d1Y2,X3=-d2Y3.êëY3úûêët31t32t33úûêëX3úûY1N(z)Fromtheseequations,aftersomealgebrawegetA(z)==,where2XD(z)1D(z)=1-dt+dt+dd(tt-tt),and1222331223322233N(z)=t-d(tt-tt)+d(tt-tt)11111221221211331331+dd{t(tt-tt)+t(tt-tt)+t(tt-tt)}.12211233133231221312231123322233ComparingtrhedenominatorofthedesiredallpasstransferfunctionwithD(z)weget-1-2t=z,t=0,tt=z.Next,comparingtrhenumeratorofthedesiredallpasstransfer22332332-1-1-2functionwithN(z)wegett=z,tt=z(z-1),tt=0,and1112211331t(tt-tt)+t(tt-tt)=1.Substitutingtheappropriatetransferparameters32112321133122131223-4fromthepreviousequationsintothelastequationwesimplifyittottt+ttt=z-1.132132311223Sincett=0,eithert=0,ort=0.(Bothcannotbesimultaneouslyequaltozero,asthis13311331-4willviolatetheconditionttt+ttt=z-1.132132311223-4Considerthecaset=0.Thentheequationabovereducestottt=z-1.Fromthis13311223-2equationandtt=z.itfollowsthat2332-2-4-1-1-2t=z,t=1,tt=z-1=(z-1)(z+1)(z+1).32233112Therearefourpossiblerealizablesetsofvaluesoft21andt31satisfyingthelastequationand-1-2tt=z(z-1).Theseleadtofourdifferentrealizabletransfermatricesforthethree-pair:1221éz-2z-2-10ùéz-2z-1+10ùê-1-1úê-1-1-1úType2A:êzz1ú,Type2B:êz(z-1)z1ú,êz-2+1z-20úê-2-1-2úëûë(z+1)(z-1)z0ûéz-2z-1-10ùéz-210ùê-1-1-1úê-1-2-1úType2C:êz(z+1)z1ú,Type2D:êz(z-1)z1ú.ê(z-2+1)(z-1+1)z-20úêz-4-1z-20úëûëûArealizationofeachType2allpassstructuresisobtainedbyimplementingitsrespectivetransfermatrix,andthenconstrainingtheY2andX2variablesthroughthemultiplierd1and211
constrainingtheY3andX3variablesthroughthemultiplier–d2resultinginthefourstructuresshowninFigure6.38oftext.Itcanbeeasilyshownthattheallpassstructuresobtainedforthecaset=0arepreciselythe31transposeofthestructuresofFigure6.38.6.39AcascadeconnectionoftwoType2Dsecond-orderallpassnetworksisashownbelowwhichisseentorequire8delays:Bydelay-sharingbetweenadjacentallpasssectionswearriveatthefollowingequivalentrealizationrequiringnow6delays.TheminimumnumberofmultipliersneededtoimplementacascadeofMType2Dsecond-orderallpasssectionsisthus4+2(M–1)=2(M+1).-1-2d+dz+z216.40WerealizeA(z)=intheformofaconstrainedthree-pairasindicatedin2-1-21+dz+dz12thefigureinthesolutionofProblem6.46.ComparingthenumeratorandthedenominatoroftheType3allpasstransferfunctionwithN(z)andD(z)giveninthesolutionofProblem6.46-2-1-2-2-1-2wearriveatt=z,t=z,t=z,tt=z,tt=z(z-1),11223323322332-4-3-2-1-4tt=z-1,ttt+ttt=z(z-1)+z(z-1).Tosolvethelastfour1331132132132132-2equations,wepreselectt23andt32satiasfyingtt=z,andthendeterminerealizablevalues2332fort12,t21,t13,andt31,212
Choice#1:t23=z–1,t32=z–2.Thisleadstofourpossiblerealizablesetsofvaluesoft12,t21,t13,andt31,satisfyingtheconstraintequationsgivenearlierandresultinginthetransfermatricesgivenbelow:é-2-2-2ùé-2-1-1ùzz-1z-1zz+1z+1ê-1-1-1úê-1-1-1-1úType3A:êzzzú,Type3B:êz(z–1)zzú,êz-2+1z-2z-2úê-1-2-2-2úëûë(z-1)(z+1)zzûé-2-1-1ùé-2ùzz-1z-1z11ê-1-1-1-1úê-1-2-1-1úType3C:êz(z+1)zzú,Type3D:êz(z-1)zzú.ê(z-1+1)(z-2+1)z-2z-2úêz-4-1z-2z-2úëûëûArealizationofeachType3allpassstructuresisobtainedbyimplementingitsrespectivetransfermatrix,andthenconstrainingtheY2andX2variablesthroughthemultiplierd1andconstrainingtheY3andX3variablesthroughthemultiplier–d2.RealizationsofTypes3A,3Cand3DallpassareshowninFigure6.38oftext.TherealizationofType3Ballpassisshownbelow:Choice#2:t23=1,t32=z–3.Thisleadstofourpossiblerealizablesetsofvaluesoft12,t21,t13,andt31,satisfyingtheconstraintequationsgivenearlierandresultinginthetransfermatricesgivenbelow:é-2-1-2-2ùé-2-1-1-1ùzz(z-1)z-1zz(z+1)z+1êúêú-1-1-1Type3E:ê1z1ú,Type3F:êz-1z1ú,ê-2-3-2úê-1-2-3-2úêëz+1zzúûêë(z-1)(z+1)zzúûé-2-1-1-1ùé-2-1-1-1ùzz(z-1)z-1zz(z-1)z-1êúêú-1-1-2-1Type3G:êz+1z1ú,Type3H:êz-1z1ú.ê-1-2-3-2úê-4-3-2úêë(z+1)(z+1)zzúûêëz-1zzúûRealizationofTypes3HisshowninFigure6.38oftext.TherealizationsofTypes3E,3Fand3Gallpassareshownbelow:Choice#3:t23=z–2,t32=z–1.ThestructuresinthiscasearethetransposeoftheTypes3A,3B,3Cand3DallpassnetworksofChoice#1givenabove.Choice#4:t23=z–3,t32=1.ThestructuresinthiscasearethetransposeoftheTypes3E,3F,3Gand3HallpassnetworksofChoice#2givenabove.6.41AcascadeconnectionoftwoType3Hsecond-orderallpassnetworksisashownbelowwhichisseentorequire8delays:213
Bydelay-sharingbetweenadjacentallpasssectionswearriveatthefollowingequivalentrealizationrequiringnow7delays.TheminimumnumberofmultipliersneededtoimplementacascadeofMType3Hsecond-orderallpasssectionsisthus4+3(M–1)=3M+1.6.42FromthetransferparametersgiveninEq.(6.52d)wearriveattheinput-outputrelationsofthe-12-1two-pairas(1):Y=kX+zX,and(2)Y=(1-k)X-kzX.Athree-multiplier1m122m1m2realizationbasedonthesetwoequationsisshownbelow:1-k2mX1Y2-kmkmY1–1X2z6.43Eq.(2)inthesolutiongivenabovecanberewrittenas2-1-1Y=X-kX-kzX=X-k(kX+zX).SubstitutingEq.(1)ofthesolution21m1m21mm122-1givenaboveinthisequationwethenget(3):Y=X-kX-kzX=X-kY.21m1m21m1ArealizationbasedonEq.(3)andEq.(1)inthesolutiongivenaboveresultsinthelatticestructureshownbelow:214
X1Y2-kmkmY1–1X2z-1AnalyzingtheabovestructureweobtainY1=kmX1+zX2,and-12-1Y2=X1-kmY1=X1-km(kmX1+zX2)=(1-km)X1-kmzX2.Hence,thetransferparametersofthelatticetwo-pairaregivenby-12-1t11=km,t12=z,t21=1-km,t22=-kmz.ThecorrespondingchainparametersareobtainedusingEq.(4.176b)andaregivenby-1-11kmzkmzA=,B=,C=,D=.1-k2222m1-km1-km1-kmX1W1-k2-k1k2k1Y1-1z-1S1zHence,theinput-outputrelationoftheall-polecascadedlatticestructuregivenabovecanbeéX1(z)ù1é1k2z-1ùé1k1z-1ùéW1(z)ùexpressedas:êY1(z)ú=22ê-1úê-1úêS1(z)ú,fromwhichweëû(1-k2)(1-k1)êëk2zúûêëk1zúûëû22W1(z)(1-k2)(1-k1)obtainHr(z)==.X1(z)1+k1(1+k2)z-1+k2z-2X1W1-k2-k1k2k1Y1z-1-1S1zLikewise,fromSection6.8.1,wearriveattheinput-outputrelationoftheall-polecascadedéX1(z)ùé1k2z-1ùé1k1z-1ùéW1(z)ùlatticestructuregivenaboveas:êY1(z)ú=ê-1úê-1úêS1(z)ú,fromwhichweëûêëk2zúûêëk1zúûëûW1(z)1obtainHs(z)==.X1(z)1+k1(1+k2)z-1+k2z-2Nowforasecond-orderall-poletransferfunctioncanalsobeexpressedintermsofitspolesas1H(z)=.ComparingthedenominatorofH(z)withthatofHs(z)1-2rcosw-12-2oz+rz215
2andHr(z)weobservek2=randk1(1+k2)=-2rcoswo.Asr@1,2rcoswok1=-=-coswo.1+r2jwr1Atthetrueresonancepeak,wehaveH(e)=.Likewise,atw=wr,(1-r2)sinwojwr12jwrHs(e)=.Asr®1,k2=r®1,andhence,thegainHs(e)2(1-k2)1-k1becomesexcessivelylarge.Inthecaseoftheall-polestructurerealizedusingthereverselattice2two-pairs,asr®1,k2=r®1,2222(1-k1)(1-k2)=(1-k1)(1-k2)(1+k2)@2(1-k1)(1-k2).Hence,atw=wr,2jwr2(1-k1)(1-k2)2Hr(e)@=21-k1=2sinwo.Thisindicatesthatthegainatthe2(1-k2)1-k1peakresonanceisapproximatelyindependentofthepoleradiusaslongasthepoleangleisconstantandtheradiusiscloseto1.-1-2-1-22+z+2z0.125-0.75z+z6.44(a)H(z)=.Choose,A(z)=.Note1-1-22-1-21-0.75z+0.125z1-0.75z+0.125zk=A(¥)=d=0.125<1.UsingEq.(6.71)wenextdeterminethecoefficientsofA1(z)222-10.66667+zarrivingatA(z)=.Here,k=A(¥)=d"=-0.66667,Hence,1-11111-0.66667zk=0.66667<1.Therefore,A2(z)andhenceH1(z)isstable.1Todeterminethefeed-forwardcoefficientsweusea=p=2,a=p-ad=2.5,122111a=p-ad-ad"=3.41667.FinalrealizationofH1(z)isthusasshownbelow:301221X1–0.1250.666670.125–0.66667–1–1zz22.53.41667Yo-1-2-1-21+2z+3z0.25-z+z(b)H(z)=.Thus,A(z)=.Notek=A(¥)=d=0.25<1.2-1-22-1-22221-z+0.25z1-z+0.25z-1–0.8+zUsingEq.(6.71)wenextdeterminethecoefficientsofA1(z)arrivingatA(z)=.1-11-0.8zHere,k=A(¥)=-0.8.Thus,k=0.8<1.Therefore,A2(z)andhenceH2(z)isstable.111216
Todeterminethefeed-forwardcoefficientsweusea=p=3,a=p-ad=5,122111a=p-ad-ak=4.25.FinalrealizationofH2(z)isthusasshownbelow:301221X1–0.250.80.25–0.8–1–1zz354.25Yo-1-2-3-1-2-32+5z+8z+3z0.25+0.5z+0.75z+z(c)H(z)=.Thisimplies,A(z)=.3-1-2-33-1-2-31+0.75z+0.5z+0.25z1+0.75z+0.5z+0.25zNotek=A(¥)=d=0.25<1.UsingEq.(6.71)wenextdeterminethecoefficientsofA2(z)33312-1-2+z+z33arrivingatA(z)=.Notek=A(¥)=d"=1/3<1.UsingEq.(6.71)we22-11-22221+z+z33-10.5+znextdeterminethecoefficientsofA1(z)arrivingatA(z)=.Thus,1-11+0.5zk=A(¥)=d"=0.5<1.Sincek<1fori=3,2,1,A3(z)andhenceH3(z)isstable.111iTodeterminethefeed-forwardcoefficientswemakeuseofEq.(6.98)andobtaina=p=3,1311a=p-ad=5.75,a=p-ad-ad"=-.a=p-ad-ad"-ad"=-.22113112213401322312FinalrealizationofH3(z)isthusasshownbelow:X1–0.25–1/3–0.50.251/30.5–1–1–1zzz35.75–1/3–1/2Yo-1-2-1-2-31+1.6z+0.6z0.25-0.25z-z+z(d)H(z)=.ThisimpliesA(z)=.Note4-1-2-33-1-2-31-z-0.25z+0.25z1-z-0.25z+0.25zk=A(¥)=d=0.25<1.UsingEq.(6.71)wenextdeterminethecoefficientsofA2(z)333-1-1+zarrivingatA(z)=.Notek=A(¥)=d"=0.UsingEq.(6.71)wenextdetermine2-12221-z-1-1+zthecoefficientsofA1(z)arrivingatA(z)=.k=A(¥)=d"=-1.Since1-11111-zk=1,A3(z)andhenceH4(z)isunstable.Feed-forwardcoefficientsarenextdeterminedusing1217
Eq.(6.98)andaregivenbya=0,a=0.6,a=2.2,a=3.2.FinalrealizationofH4(z)is1234thusasshownbelow:X1–0.25–1–1–1–1zzz0.62.23.2Yo-1-2-33+1.5z+z+0.5z(e)H(z)=.Hence,5-1-2-3-41-1.8333z+1.5z-0.5833z+0.0833z-1-2-3-40.0833-0.5833z+1.5z-1.8333z+zA(z)=.Notek=A(¥)=d=0.0833<1.4-1-2-3-44441-1.8333z+1.5z-0.5833z+0.0833zUsingEq.(6.71)wenextdeterminethecoefficientsofA3(z)arrivingat-1-2-30.433595+1.38466z-1.7972z+zA(z)=.Thus,k=A(¥)=d"=0.433595<1.3-1-2-33331-1.7972z+1.38466z-0.433595z-1-20.74558-1.4739z+zContinuingthisprocessweobtainA(z)=.Thus,2-1-21-1.4739z+0.74558z-1-0.84436+zk=A(¥)=d"=0.74558<1.FinallywearriveatA(z)=.Thisimplies2221-11-0.84436zk=A(¥)=d"""=-0.84436.Sincek<1fori=4,3,2,1,A4(z)andhenceH5(z)isstable.111iFeed-forwardcoefficientsarenextdeterminedusingEq.(6.98)andaregivenbya=0,a=0.5,a=1.89859,a=3.606,a=4.846.12345-1-20.6-0.07z-1.55z6.45(a)H(z)=.Hence11+2.77z-1-1.593z-2-2.01z-3-1-2-3-2.01-1.593z+2.77z+zA(z)=.Notek=A(¥)=-2.01.UsingEq.(6.47)we31+2.77z-1-1.593z-2-2.01z-333-1-2-1.3074+0.1421z+zthenarriveatA(z)=.Notek=A(¥)=-1.3074.UsingEq.21+0.1421z-1-1.3074z-222-1-2.0434+z(6.47)againwenextarriveatA(z)=.Notek=A(¥)=-2.0434.Since11-2.0434z-111k>1,1£i£3,A(z)andhence,H(z)isanunstabletransferfunction.NextusingEq.i31(6.79)wearriveatthefeed-forwardmultipliers:a=0,a=-1.55,a=0.1503,and123a=0.4306.4NotethatifProgram6_4isusedtorealizeH(z),thefollowingerror1messagewillappear:???Errorusing==>tf2latcTheIIRsystemisunstable218
x[n]2.011.30742.0434–1.3074–2.0434-1-1-1zzz–1.550.15030.4306y[n]-1-3-44.1-1.3667z+1.64z-0.5467z(b)H(z)=.Hence,21-1.2z-1+0.15z-2+0.13z-3-0.015z-4-1-2-3-4-0.015+0.13z+0.15z-1.2z+zA(z)=.NoteA(¥)=k=-0.015.UsingEq.41-1.2z-1+0.15z-2+0.13z-3-0.015z-444-1-2-30.112+0.1523z-1.1983z+z(6.47)wethenarriveatA(z)=.Note31-1.1983z-1+0.1523z-2+0.112z-3A(¥)=k=0.112.UsingEq.(6.47)againwenextarriveat33-1-20.2902-1.2308z+zA(z)=.NoteA(¥)=k=0.2902.UsingEq.(6.47)we21-1.2308z-1+0.2902z-222-1-0.9540+zfinallyarriveatA(z)=.NoteA(¥)=k=-0.9540.Sincek<1,11-0.9540z-111i1£k£4,A(z)andhence,H(z)isastabletransferfunction.i42Thefeed-forwardmultipliers{ai}aredeterminedusingthefollowingequations:"a=p=-0.5467,a=p-ad=0.9840,a=p-ad-ad=1.2611,142311321221""""""a4=p1-a1d3-a2d2-a2d3=0.1067,anda5=p0-a1d4-a2d3-a2d2-a3d1=3.7175,Thelatticeandfeed-forwardmultiplierscanalsobeobtainedusingProgram6_4yieldingLatticeparametersare-0.95400.29020.1120-0.0150Feedforwardmultipliersare3.71750.10671.26110.9840-0.5467x[n]0.015–0.112–0.29020.954–0.0150.1120.2902–0.954-1-1-1-1zzzz–0.54670.9841.26110.10673.7175y[n]219
-1-2-38.1+6.93z-23.82z+10.5z(c)H(z)=.Hence,31+1.52z-1+0.18z-2-0.1768z-3-1-2-3-0.1768+0.18z+1.52z+zA(z)=.NoteA(¥)=k=-0.1768.UsingEq.(6.47)31+1.52z-1+0.18z-2-0.1768z-333-1-20.4632+1.6019z+zwethenarriveatA(z)=.NoteA(¥)=k=0.4632.UsingEq.21+1.6019z-1+0.4632z-222-11.0948+z(6.47)againwenextarriveatA(z)=.NoteA(¥)=k=1.0948.Since11+1.0948z-111k>1,A(z),andhence,H(z)areunstabletransferfunctions.NextusingEq.(6.79)we133arriveatthefeed-forwardmultipliers:a=10.5,a=-39.78,a=68.7636,and123a=-46.8999.4NotethatifProgram6_4isusedtorealizeH(z),thefollowingerrormessagewillappear:3???Errorusing==>tf2latcTheIIRsystemisunstablex[n]0.1768–0.4632–1.0948–0.17680.46321.0948-1-1-1zzz10.5–39.7868.7636–46.8999y[n]-1-1-20.5634(1+z)(1-1.10166z+z)6.52H(z)=.(1-0.683z-1)(1-1.4461z-1+0.7957z-2)-1-2-30.05634(1-0.10166z-0.10166z+z)(a)Directcanonicform-H(z)=.1-2.1291z-1+1.78339z-2-0.54346z-3X(z)Y(z)z–1–0.101662.1291–1.7833863z–1–0.10166–0.5434631z–1Hardwarerequirements:#ofmultipliers=5,#oftwo-inputadders=6,#ofdelays=3.(b)CascadeForm220
0.05634X(z)Y(z)z–1z–1–1.01660.6831.4461–1–0.7957zHardwarerequirements:#ofmultipliers=5,#oftwo-inputadders=6,#ofdelays=3.(c)Gray-MarkelForm-0.05634X1-d3-d2"-d1"d3d"d1"2–1–1–1zzzaa1a23a4Yo""d=-0.5434631,d=0.8881135,d=-0.8714813.321"a=p=1,a=p-ad=2.02744,a=p-ad-ad=1.45224,132211311221""a=p-ad-ad-ad=1.00702.40132231Hardwarerequirements:#ofmultipliers=9,#oftwo-inputadders=6,#ofdelays=3.(d)CascadedLatticeStructure-p"+p"z-1+p"z-21.3136-1.2213z-1+z-2012UsingEqn.(6.152)weobtainH(z)==.21+d"z-1+d"z-21-1.4152z-1+1.1197z-212p"+p"z-1–1.3546+z-101andH(z)==.Thefinalstructureisasshownbelow:11+d"z-11-0.1015z-11X1–d3-d2"-d1"pp"00"p0Y–1–1–1ozzzp3""wherep=0.5634,d=-0.54346,p=0.5634,p=1.3136,d=1.1197,03302""p=–1.3546,andd=–0.1015.01N/2N/2*uuii6.47(a)ApartialfractionexpansionofG(z)isoftheformG(z)=d+å+å*.Ifwei=1z-lii=1z-liN/2duidefineH(z)=+å,thenwecanwriteG(z)=H(z)+H*(z),whereH*(z)representsthe2z-li=1itransferfunctionobtainedfromH(z)byconjugatingitscoefficients.221
(b)Inthiscase,thepartialfractionexpansionofG(z)isoftheformNrNc/2Nc/2*ruuiiiG(z)=d+å+å+å*,whereNrandNcarethenumberofrealpolesi=1z-xii=1z-lii=1z-lix"sandcomplexpolesl"s,respectively,withresiduesr"sandu"s.WecanthusdecomposeiiiiNN/2rr/2cudiiG(z)asG(z)=H(z)+H*(z),whereH(z)=+å+å.2z-xz-li=1ii=1i(c)AnimplementationofrealcoefficientG(z)isthussimplyaparallelconnectionoftwocomplexfilterscharacterizedbytransferfunctionsH(z)andH*(z)asindicatedinthefigurebelow:y[n]x[n]H(z)H(z)*=0However,forarealvaluedinputx[n],theoutputofH(z)isthecomplexconjugateofH*(z).Asaresult,twotimestherealpartoftheoutputofH(z)isthedesiredreal-valuedsequencey[n]indicatingthatsinglecomplexfilterH(z)issufficienttorealizeG(z)asindicatedbelow:2y[n]x[n]H(z)Y(z)A+jB6.48FromH(z)==,wearriveatthedifferenceequationrepresentationX(z)1+(a+b)z-1y[n]+jy[n]=–(a+jb)(y[n-1]+jy[n-1])+Ax[n]+jBx[n],whichisequivalenttoasetreimreimoftwodifferenceequationsinvolvingallrealvariablesandrealmultipliercoefficients:y[n]=–ay[n-1]+by[n-1]+Ax[n],andy[n]=–by[n-1]–ay[n-1]+Bx[n].rereimimreimArealizationofH(z)basedonthelasttwoequationsisshownbelow:Ax[n]yre[n]–1z–a–bByim[n]–1z–abTodeterminethetransferfunctionYre(z)/X(z),wetakethez-transformsofthelasttwo-1-1differenceequationsandarriveat(1+az)Y(z)–bzY(z)=AX(z),andreim222
-1-1bzY(z)+(1+az)Y(z)=BX(z).SolvingthesetwoequationswegetreimY(z)A+(Aa+Bb)z-1re=,whichisseentobeasecond-ordertransferfunction.X(z)1+2az-1+(a2+b2)z-26.49Anm-thordercomplexallpassfunctionisgivenby**-1*-(m-1)*-ma+az+L+az+azmm-11mA(z)=.m-1-2-(m-1)-m1+az+az+L+az+az12m-1mTogeneratean(m–1)-thorderallpassweusetherecursioné*ùP(z)A(z)-kA(z)=m-1=zêmmú.m-1D(z)ê1-kA(z)úm-1ëmmûSubstitutingtheexpressionforAm(z)intheaboveweobtainaftersomealgebra**-1*-2*-(m-1)-mP(z)=z[a+az+az+L+az+zm-1mm-1m-21*-1-2-(m-1)-m-a(1+az+az+L+az+az)]m12m-1m****-1**-(m-2)2-(m-1)=(a-aa)+(a-aa)z+L+(a-aa)z+(1-a)z,m-1m1m-2m21mm-1m-1-2-(m-1)-mD(z)=1+az+az+L++az+azm-112m-1m**-1*-2*-(m-1)-m-a(a+az+az+L++az+z)mmm-1m-212*-1*-2*-(m-1)=(1-a)+(a-aa)z+(a-aa)z+L+(a-aa)z.m1mm-12mm-2m-1m1Hence,Am–1(z)isacomplexallpassfunctionoforderm–1givenby**-1*-(m-2)-(m-1)b+bz+L+bz+zm-1m-21A(z)=,m-1-1-2-(m-2)-(m-1)1+bz+bz+L+bz+bz12m-2m-1*a-aakmm-kwhereb=,k=1,2,...,m–1.k21-amTodeveloparealizationofAm(z)weexpressAm(z)intermsofAm–1(z):*-1Yk-zA(z)1mm-1A(z)==,mX1+kz-1A(z)1mm-1andcompareitwithEq.(6.73)resultinginthefollowingexpressionsforthetransfer*-1*-1parametersofthetwo-pair:t=k,t=-kz,andtt=(1-kk)z.Asinthe11m22m1221mmcaseoftherealizationofarealallpassfunction,therearemanypossiblechoicesfort12andt21.*-1Wechooset=(1-kk)z,t=1.Thecorrespondinginput-outputrelationsofthetwo-12mm21**-1*-1-1-1pairY=kX+(1-kk)zX=k(X-kzX)+zX,andY=X-kzX.1m1mm2m1m2221m2ArealizationofAm(z)basedontheabovetwo-pairrelationsisindicatedbelow:223
X1Y2–kmAm(z)®Am–1(z)k*mY1–1X2zBycontinuingthisprocess,wearriveatacascadedlatticerealizationofacomplexallpasstransferfunction.-1æ-1ö2+2z11+3z16.50(a)H(z)==ç1+÷=(A(z)+A(z)),whereA(z)=1and13+z-12çè3+z-1÷ø2010-11+3zA(z)=.1-13+z-1æ-1ö1-z12+4z1(b)H(z)==ç1-÷=(A(z)-A(z)),whereA(z)=1and24+2z-12çè4+2z-1÷ø2010-12+4zA(z)=.1-14+2z-2æ-1-2ö1-z12+2z+4z1(c)H(z)==ç1-÷=(A(z)-A(z)),whereA(z)=134+2z-1+2z-22çè4+2z-1+2z-2÷ø2010-1-22+2z+4zandA(z)=.1-1-24+2z+2z-1-2-3æ-1-2-3öéæ-1öæ-1öù(d)H(z)=3+9z+9z+3z=1ç3+9z+9z+3z÷=1êz-1ç1+3z÷+ç1+2z÷ú412+10z-1+2z-22çè(3+z-1)(2+z-1)÷ø2êçè3+z-1÷øçè2+z-1÷øúëûæ-1öæ-1ö=1(A(z)+A(z)),whereA(z)=z-1ç1+3z÷andA(z)=ç1+2z÷.2010ç3+z-1÷1ç2+z-1÷èøèø6.51(a)Fromtheequationgivenwegety[2l]=h[0]x[2l]+h[1]x[2l-1]+h[2]x[2l-2]+h[3]x[2l-3]+h[4]x[2l-4]+h[5]x[2l-5],andy[2l+1]=h[0]x[2l+1]+h[1]x[2l]+h[2]x[2l-1]+h[3]x[2l-2]+h[4]x[2l-3]+h[5]x[2l-4].Rewritingtheabovetwoequationsinamatrixformwearriveatéy[2l]ùéh[0]0ùéx[2l]ùéh[2]h[1]ùéx[2l-2]ù=+ëêy[2l+1]ûúëêh[1]h[0]ûúëêx[2l+1]ûúëêh[3]h[3]ûúëêx[2l-1]ûúéh[4]h[3]ùéx[2l-4]ùé0h[5]ùéx[2l-6]ù++,ëêh[5]h[4]ûúëêx[2l-3]ûúëê00ûúëêx[2l-5]ûúwhichcanbealternatelyexpressedasY=HX+HX+HX+HX,l0l1l-12l-23l-3224
éy[2l]ùéx[2l]ùéh[0]0ùéh[2]h[1]ùwhereY=,X=,H=,H=,lëêy[2l+1]ûúlëêx[2l+1]ûú0ëêh[1]h[0]ûú1ëêh[3]h[3]ûúéh[4]h[3]ùé0h[5]ùH=,andH=.2ëêh[5]h[4]ûú3ëê00ûú(b)Herey[3l]=h[0]x[3l]+h[1]x[3l-1]+h[2]x[3l-2]+h[3]x[3l-3]+h[4]x[3l-4]+h[5]x[3l-5],y[3l+1]=h[0]x[3l+1]+h[1]x[3l]+h[2]x[3l-1]+h[3]x[3l-2]+h[4]x[3l-3]+h[5]x[3l-4],andy[3l+2]=h[0]x[3l+2]+h[1]x[3l+1]+h[2]x[3l]+h[3]x[3l-1]+h[4]x[3l-2]+h[5]x[3l-3].Rewritingtheabovetwoequationsinamatrixformwearriveatéy[3l]ùéh[0]00ùéx[3l]ùéh[3]h[2]h[1]ùéx[3l-3]ùêy[3l+1]ú=êh[1]h[0]0úêx[3l+1]ú+êh[4]h[3]h[2]úêx[3l-2]úêúêúêúêúêúëy[3l+2]ûëh[2]h[1]h[0]ûëx[3l+2]ûëh[5]h[4]h[3]ûëx[3l-1]ûé0h[2]h[1]ùéx[3l-6]ù+ê00h[2]úêx[3l-5]ú,êúêúë000ûëx[3l-4]ûwhichcanbealternatelyexpressedasY=HX+HX+HX,wherel0l1l-12l-2éy[3l]ùéx[3l]ùéh[0]00ùéh[3]h[2]h[1]ùY=êy[3l+1]ú,X=êx[3l+1]ú,H=êh[1]h[0]0ú,H=êh[4]h[3]h[2]ú,andlêúlêú0êú1êúëy[3l+2]ûëx[3l+2]ûëh[2]h[1]h[0]ûëh[5]h[4]h[3]ûé0h[2]h[1]ùH=ê00h[2]ú.2êúë000û(c)FollowingaproceduresimilartothatoutlinedinParts(a)and(b)above,wecanshwthathereY=HX+HX+HX,wherel0l1l-12l-2éy[4l]ùéx[4l]ùéh[0]000ùêy[4l+1]úêx[4l+1]úêh[1]h[0]00úYl=êy[4l+2]ú,Xl=êx[4l+2]ú,H0=êh[2]h[1]h[0]0ú,êúêúêúëy[4l+3]ûëx[4l+3]ûëh[3]h[2]h[1]h[0]ûéh[4]h[3]h[2]h[1]ùé000h[5]ùêh[5]h[4]h[3]h[2]úê0000úH1=ê0h[5]h[4]h[3]ú,andH2=ê0000ú.êúêúë00h[5]h[4]ûë0000û6.52(a)dy[2l]+dy[2l-1]+dy[2l-2]+dy[2l-3]+dy[2l-4]01234=px[2l]+px[2l-1]+px[2l-2]+px[2l-3]+px[2l-4],01234dy[2l+1]+dy[2l]+dy[2l-1]+dy[2l-2]+dy[2l-3]01234=px[2l+1]+px[2l]+px[2l-1]+px[2l-2]+px[2l-3],01234Rewritingtheabovetwoequationsinamatrixformwearriveatéd00ùéy[2l]ùéd2d1ùéy[2l-2]ùéd4d3ùéy[2l-4]ùêëddúûëêy[2l+1]ûú+êëddúûëêy[2l-1]ûú+êë0dúûëêy[2l-3]ûú10324ép00ùéx[2l]ùép2p1ùéx[2l-2]ùép4p3ùéx[2l-4]ù=êëppúûëêx[2l+1]ûú+êëppúûëêx[2l-1]ûú+êë0púûëêx[2l-3]ûú,10324225
whichcanbealternatelyexpressedasDY+DY+DY=PX+PX+PX,0l1l-12l-20l1l-12l-1éy[2l]ùéx[2l]ùéd00ùéd2d1ùéd4d3ùwhereYl=ëêy[2l+1]ûú,Xl=ëêx[2l+1]ûú,D0=êëddúû,D1=êëddúû,D2=êë0dúû,10324ép0ùéppùéppùP=ê0ú,P=ê21ú,andP=ê43ú.0ëp1p0û1ëp3p2û2ë0p4ûéd000ùéy[3l]ùéd3d2d1ùéy[3l-3]ùé00d4ùéy[3l-6]ù(b)êdd0úêy[3l+1]ú+êdddúêy[3l-2]ú+ê000úêy[3l-5]úê10úêúê432úêúêúêúêëd2d1d0úûëy[3l+2]ûêë0d4d3úûëy[3l-1]ûë000ûëy[3l-4]ûép00ùéx[3l]ùépppùéx[3l-3]ùé00pùéx[3l-6]ùê0úê321úê4ú=êp1p00úêx[3l+1]ú+êp4p3p2úêx[3l-2]ú+ê000úêx[3l-5]ú,êúêúêúêëp2p1p0úûëx[3l+2]ûêë0p4p3úûëx[3l-1]ûë000ûëx[3l-4]ûwhichcanbealternatelyexpressedasDY+DY+DY=PX+PX+PX,0l1l-12l-20l1l-12l-1éy[3l]ùéx[3l]ùéd000ùéd3d2d1ùwhereY=êy[3l+1]ú,X=êx[3l+1]ú,D=êdd0ú,D=êdddú,lêúlêú0ê10ú1ê432úëy[3l+2]ûëx[3l+2]ûêëd2d1d0úûêë0d4d3úûé00dùép00ùépppùé00pùê4úê0úê321úê4úD2=ê000ú,P0=êp1p00ú,P1=êp4p3p2ú,andP2=ê000ú.ë000ûêëp2p1p0úûêë0p4p3úûë000ûéd000ùéddddù0éy[4l]ù4321éy[4l-4]ùêdd00úêy[4l+1]úê0dddúêy[4l-3]ú(c)ê10úêú+ê432úêúêd2d1d00úêy[4l+2]úê00d4d3úêy[4l-2]úêëddddúûëy[4l+3]ûêë000dúûëy[4l-1]û32104ép000ùéppppù0éx[4l]ù4321éx[4l-4]ùêpp00úêx[4l+1]úê0pppúêx[4l-3]ú=ê10úêú+ê432úêú,êp2p1p00úêx[4l+2]úê00p4p3úêx[4l-2]úêëppppúûëx[4l+3]ûêë000púûëx[4l-1]û32104whichcanbealternatelyexpressedasDY+DY=PX+PX,where0l1l-10l1l-1éy[4l]ùéx[4l]ùéd0000ùéd4d3d2d1ùêy[4l+1]úêx[4l+1]úêdd00úê0dddúY=êú,X=êú,D=ê10ú,D=ê432ú,ly[4l+2]lx[4l+2]0êd2d1d00ú1ê00d4d3úêúêúëy[4l+3]ûëx[4l+3]ûêëddddúûêë000dúû32104ép000ùéppppù04321êpp00úê0pppúP=ê10ú,andP=ê432ú.0êp2p1p00ú1ê00p4p3úêëppppúûêë000púû321046.53Wefirstrewritethesecond-orderblock-differenceequationDY+DY+DY=PX+PX+PX,0l1l-12l-20l1l-12l-1astwoseparateequations:W=PX+PX+PX,andl0l1l-12l-1-1-1-1Y=–DDY–DDY+DW.l01l-102l-20l226
AcascaderealizationoftheIIRblockdigitalfilterbasedontheabovetwoequationsisthusasshownbelow:WlXlPD–1Yl00DDPD11DDPD22Byinterchangingthelocationsofthetwoblocksectionsintheabovestructurewegetanequivalentrealizationasindicatedbelow:XlD–1PY00lDDDP11DDDP22Finally,bydelay-sharingtheabovestructurereducestoacanonicrealizationasshownbelow:X–1PYlDl00DDP11DDP226.54Bysettingasinq=±binEq.(6.138),thestate-spacedescriptionofthesine-cosinegeneratorécosq±1ùés1[n+1]ùê2úés1[n]ùreducestoêës[n+1]úû=êmbcosqúêës[n]úû,whichleadstothethree-multiplierstructure2ê2ú2ëaûshownbelow:227
cosqcosqs2[n+1]–1±1s1[n+1]z–1zs1[n]s2[n]mb2/a26.55Bysettinga=±bsinqinEq.(6.138),thestate-spacedescriptionofthesine-cosinegeneratorés[n+1]ùé2ùés[n]ùreducestoê1ú=êcosq±sinqúê1ú,whichleadstothethree-multiplierstructureës2[n+1]ûëm1cosqûës2[n]ûshownbelow:cosqcosq2±sinqss2[n+1]–11[n+1]z–1s[n]zs[n]12m122b1+cosqa-sinqcosq-1b6.56Let=-.Thensinq===cosq-1,and,-sinq=cosq+1.asinqbcosq+1cosq+1aés1[n+1]ùécosqcosq-1ùés1[n]ùSubstitutingthesevaluesinEq.(6.138)wearriveatêës[n+1]úû=ëêcosq+1cosqûúêës[n]úû,22Theseequationscanbealternatelyrewrittenass[n+1]=cosq(s[n]+s[n])-s[n],ands[n+1]=cosq(s[n]+s[n])+s[n].Arealization11222121basedonthelasttwoequationsresultsinasingle-multiplierstructureasindicatedbelow:s2[n+1]s2[n]–1s1[n+1]–1z–1sz1[n]cosqéa(1-Ccosq)ùés[n+1]ùéa(C-cosq)ùés[n+1]ùêCúés[n]ù6.57ê1ú=ê0bsinqúê1ú+êbsinqúê1ú.ës2[n+1]ûêúës2[n+1]ûêbúës2[n]ûêë00úûê–sinqcosqúëaûIfC=0,choosea=bsinq.Thenés1[n+1]ùé0–cosqùés1[n+1]ùé01ùés1[n]ùêës2[n+1]úû=ëê00ûúêës2[n+1]úû+ëê-1cosqûúêës2[n]úû,whichcanberealizedwithtwomultipliersasshownbelow:228
–cosqs2[n+1]s2[n]s1[n+1]s1[n]–1–1zzcosq–1Theabovestructurecanbemodifiedtoyieldasinglemultiplierrealizationasindicatedonnextpage:s2[n+1]–1s2[n]s1[n+1]–1s1[n]zzcosq–1z–1éa(1-cosq)ùés[n+1]ùéa(1-cosq)ùés[n+1]ùê1úés[n]ù6.58IfC=1,thenê1ú=ê0bsinqúê1ú+êbsinqúê1ú.ës2[n+1]ûêúës2[n+1]ûêbúës2[n]ûêë00úûê–sinqcosqúëaûChoosea=bsinq.Thisleadstoés1[n+1]ùé01-cosqùés1[n+1]ùé11-cosqùés1[n]ùêës2[n+1]úû=ëê00ûúêës2[n+1]úû+ëê–1cosqûúêës2[n]úû.Atwo-multiplierrealizationoftheaboveequationisshownbelow:1–cosqs2[n+1]s2[n]s1[n+1]–1s1[n]z–1zcosq–1Toarriveatanone-multiplierrealizationweobservethatthetwoequationsdescribingthesine-cosinegeneratoraregivenbys[n+1]=(1-cosq)s[n+1]+s[n]+(1-cosq)s[n],and1212s[n+1]=–s[n]+cosqs[n].Substitutingthesecondequationinthefirstequationwearriveat212analternatedescriptionintheforms[n+1]=–cosqs[n+1]+s[n],122s[n+1]=–s[n]+cosqs[n].212Arealizationofaboveisidenticaltothesingle-multiplierstructureofProblem6.64,6.59FromFigureP6.17(a),theoutput-inputrelationofthechannelisgivenby229
éY(z)ùé1H(z)ùéX(z)ù1121êëY(z)úû=êëH(z)1úûêëX(z)úû.2212Likewise,theoutput-inputrelationofthechannelseparationcircuitofFigureP6.17(b)isgivenéV(z)ùé1–G(z)ùéY(z)ùbyê1ú=ê12úê1ú.Hence,theoverallsystemischaracterizedbyëV2(z)ûë–G21(z)1ûëY2(z)ûéV(z)ùé1–G(z)ùé1H(z)ùéX(z)ù112121êëV(z)úû=êë–G(z)1úûêëH(z)1úûêëX(z)úû=221212é1–H(z)G(z)H(z)-G(z)ùéX(z)ùê21121212úê1ú.ëH21(z)-G21(z)1–H12(z)G21(z)ûëX2(z)ûThecross-talkiseliminatedifV1(z)isafunctionofeitherX1(z)orX2(z),andsimilarly,ifV2(z)isafunctionofeitherX1(z)orX2(z),FromtheaboveequationitfollowsthatifH12(z)=G12(z),andH21(z)=G21(z),thenV(z)=(1–H(z)G(z))X(z),and121121-1-1V(z)=(1–H(z)G(z))X(z).Alternately,ifG(z)=H(z),andG(z)=H(z),then21221212212112æH(z)H(z)-1öæH(z)H(z)-1öV(z)=ç1221÷X(z),andV(z)=ç1221÷X(z).1çèH(z)÷ø22çèH(z)÷ø12112-1-1-1-2M6.1(a)H(z)=(1-0.3261z)(1-3.0666z)(1+4.748z+7.4388z)1-1-2-1-2´(1-1.0935z+z)(1+0.6383z+0.1344z).-1-1-1-1-2(b)H(z)=(1+3.5585z)(1+0.281z)(1-z)(1-1.4078z+2.0338z)2-1-2-1-2´(1+0.2604z+z)(1-0.6922z+0.4917z)-1-2-1-21.5(1+3z+4z)(1+0.6667z+0.3333z)M6.2(a)G(z)=.(1-z-1+0.5z-2)(1-z-1+0.3333z-2)-1-21.5(1+3z+4z)(b)G(z)=G(z)G(z)whereG(z)=,andaba1-z-1+0.5z-2-1-21+0.6667z+0.3333zG(z)=.Alternately,wecanwriteG(z)=G(z)G(z)whereb1-z-1+0.3333z-2cd-1-2-1-21.5(1+0.6667z+0.3333z)1+3z+4zG(z)=,andG(z)=.c1-z-1+0.5z-2d1-z-1+0.3333z-2(c)ParallelformIisobtainedusingtheM-fileresiduezresultinginthepartialfraction-1expansioninzofG(z)givenby-35.25-j71.25-35.25+j71.2530+j147.2230-j147.22G(z)=12++++1-(0.5-j0.5)z-11-(0.5+j0.5)z-11-(0.5-j0.2887)z-11-(0.5+j0.2887)z-1-1-1-70.5-36z60+55z=12++.1-z-1+0.5z-21-z-1+0.3333z-2230
ParallelformIIisobtainedusingtheM-fileresidueresultinginthepartialfractionexpansioninzofG(z)givenby-1-1-1-1(-53.25+j18)z(-53.25-j18)z(57.50-j64.9519)z(57.50+j64.9519)zG(z)=1.5++++1-(0.5+j0.5)z-11-(0.5-j0.5)z-11-(0.5+j0.2887)z-11-(0.5-j0.2887)z-1-1-2-1-2-106.5z+35.25z115z-20z=1.5++.1-z-1+0.5z-21-z-1+0.333z-2-1-2-1-22(1-0.6667z+0.3333z)(1+0.5z+0.25z)M6.3(a)H(z)=.(1-0.5z-1+0.5z-2)(1+z-1+0.3333z-2)-1-22(1-0.6667z+0.3333z)(b)H(z)=H(z)H(z)whereH(z)=,andaba1-0.5z-1+0.5z-2-1-21+0.5z+0.25zH(z)=.Alternately,wecanwriteH(z)=H(z)H(z)whereb1+z-1+0.3333z-2cd-1-2-1-22(1-0.6667z+0.3333z)1+0.5z+0.25zH(z)=,andH(z)=.c1+z-1+0.3333z-2d1-0.5z-1+0.5z-2(c)ParallelformIisobtainedusingtheM-fileresiduezresultinginthepartialfraction-10.1622-j0.2860.1622+j0.286expansioninzofH(z)givenbyH(z)=1++1-(0.25-j0.6614)z-11-(0.25+j0.6614)z-1-1-10.3378-j1.20930.3378+j1.20930.3243-0.4595z0.6757-0.3604z++=1++.1+(0.5+j0.2887)z-11+(0.5-j0.2887)z-11-0.5z-1+0.5z-21+z-1+0.3333z-2ParallelformIIisobtainedusingtheM-fileresidueresultinginthepartialfraction-1-1(-0.5135+j0.1022)z(-0.5135-j0.1022)zexpansioninzofH(z)givenbyH(z)=2++1-(0.25+j0.6614)z-11-(0.25-j0.6614)z-1-1-1(-0.1532-j1.2795)z(-0.1532+j1.2795)z++1+(0.5-j0.2887)z-11+(0.5+j0.2887)z-1-1-2-1-2-1.027z+0.1216z-0.3063z+0.5856z=2++.1-0.5z-1+0.5z-21+z-1+0.3333z-2M6.4UsingProgram6_4weobtain:Latticeparametersare-0.83640.7980-0.51430.1667Feedforwardmultipliersare63.6358170.1598144.114357.000012.0000M6.5UsingProgram6_4weobtain:231
Latticeparametersare0.34810.18010.25710.1667Feedforwardmultipliersare12.7136-3.82232.5810-2.00004.0000M6.6(a)UsingProgram6_6weobtainthefollowingerrormessage:???Errorusing==>tf2latcTheFIRsystemhasazeroontheunitcircle(b)UsingProgram6_6weobtainthefollowingerrormessage:???Errorusing==>tf2latcTheFIRsystemhasazeroontheunitcircleM6.7(a)UsingrootswefirstdeterminethepolesofthedenominatorofthelowpasstransferfunctionG(z)whicharethenpairedusingthepoleinterlacingpropertyresultingintheparallelallpassdecompositiongivenby1é0.1302-0.3486z-1+z-2-0.0868+0.6216z-1-0.6367z-2+z-3ùG(z)=ê+ú.2êë1-0.3486z-1+0.1302z-21-0.6367z-1+0.6216z-2-0.0868z-3úû(b)Hence,thepower-complementaryhighpasstransferfunctionH(z)isgivenby1é0.1302-0.3486z-1+z-2-0.0868+0.6216z-1-0.6367z-2+z-3ùH(z)=ê-ú2êë1-0.3486z-1+0.1302z-21-0.6367z-1+0.6216z-2-0.0868z-3úû-1-2-3-4-50.1085(1-4.9928z+9.9891z-9.9891z+4.9928z+z)=.1-0.9853z-1+0.9738z-2-0.3864z-3+0.1112z-4-0.0113z-5(c)jw2jw2|H(e)|+|G(e)|10.8jw2|H(ejw)|2|G(e)|0.60.40.2000.20.40.60.81w/p232
M6.8(a)UsingrootswefirstdeterminethepolesofthedenominatorofthehighpasstransferfunctionG(z)whicharethenpairedusingthepoleinterlacingpropertyresultingintheparallelallpassdecompositiongivenby1é0.5776+0.6976z-1+z-20.3974+1.1635z-1+1.0052z-2+z-3ùG(z)=ê-ú.2êë1+0.6976z-1+0.5776z-21+1.0052z-1+1.1635z-2+0.3974z-3úû(b)Hence,thepower-complementarylowpasstransferfunctionH(z)isgivenby1é0.5776+0.6976z-1+z-20.3974+1.1635z-1+1.0052z-2+z-3ùH(z)=ê+ú2êë1+0.6976z-1+0.5776z-21+1.0052z-1+1.1635z-2+0.3974z-3úû-1-2-3-4-50.4875(1+1.3594z+2.2098z+2.2098z+1.3594z+z)=.1+1.7028z-1+2.4423z-2+1.7896z-3+0.9492z-4+0.2295z-5(c)|G(ejw)|2+|H(ejw)|210.8jw2|G(e)|0.60.40.2jw2|H(e)|000.20.40.60.81w/pM6.9(a)UsingrootswefirstdeterminethepolesofthedenominatorofthebandpasstransferfunctionG(z)whicharethenpairedusingthepoleinterlacingpropertyresultingintheparallelallpassdecompositiongivenby1é0.5874-0.5154z-1+z-20.6159+0.7531z-1+z-2ùG(z)=ê-ú2êë1-0.5154z-1+0.5874z-21+0.7531z-1+0.6159z-2úû(b)Hence,thepower-complementarybandstoptransferfunctionH(z)isgivenby1é0.5874-0.5154z-1+z-20.6159+0.7531z-1+z-2ùH(z)=ê+ú2êë1-0.5154z-1+0.5874z-21+0.7531z-1+0.6159z-2úûæ1+0.3013z-1+1.6183z-2+0.3013z-3+z-4ö=1.2033ç÷.çè1+0.2377z-1+0.8152z-2+0.1294z-3+0.3618z-4÷ø233
(c)jw2jw2|G(e)|+|H(e)|10.8jw2jw2|G(e)||H(e)|0.60.40.2000.20.40.60.81w/pM6.10TheMATLABprogramforthesimulationofthesine-cosinegeneratorofProblem6.56isgivenbelow:s10=0.1;s20=0.1;a=0.9;y1=zeros(1,50);y2=y1;forn=1:50;y1(n)=a*(s10+s20)-s20;y2(n)=a*(s10+s20)+s10;s10=y1(n);s20=y2(n);endk=1:1:50;stem(k-1,y1/abs(y1(7)));axis([050-1.11.1]);xlabel("Timeindexn");ylabel("Amplitude");pausestem(k-1,y2/y2(3));axis([050-1.11.1]);xlabel("Timeindexn");ylabel("Amplitude");Theplotsgeneratedbytheaboveprogramforinitialconditionss1[–1]=s2[–1]=0.1areshownbelow:Theoutputsarezeroforzeroinitialconditions.Non-zeroinitialconditionsofequalvaluesappeartohavenoeffectsontheoutputs.However,unequalinitialconditionshaveeffectsontheamplitudesandphasesofthetwooutputsequences.M6.11TheMATLABprogramforthesimulationofthesine-cosinegeneratorofProblem6.57isgivenbelow:234
s10=.1;s20=1;a=0.9;y1=zeros(1,50);y2=y1;forn=1:50;y1(n)=-s20+a*s10;y2(n)=-a*y1(n)+s10;s10=y1(n);s20=y2(n);endk=1:1:50;stem(k-1,y1/y1(11));axis([050-1.11.1]);xlabel("Timeindexn");ylabel("Amplitude");pausestem(k-1,y2/y2(14));axis([050-1.11.1]);xlabel("Timeindexn");ylabel("Amplitude");Theplotsgeneratedbytheaboveprogramforinitialconditionss1[–1]=s2[–1]=0.1areshownbelow:Theoutputsarezeroforzeroinitialconditions.Non-zeroinitialconditionsofequalvaluesappeartohavenoeffectsontheoutputs.However,unequalinitialconditionshaveeffectsontheamplitudesandphasesofthetwooutputsequences.M6.12Sincethesinglemultipliersine-cosinegeneratorofProblem6.58isidenticaltothesingle-multiplierstructureofProblem6.64,thesimulationprogramgivenaboveinthesolutionofProblemM6.12alsoholdshere.235
Chapter7(2e)-a/10-a/107.1d=1-10pandd=10s.ps-0.15/20(a)a=0.15anda=41.Henced=1-10=0.017121127andpsp-41/20d=10=0.0089125.s-0.035/20(b)a=0.23anda=73.Hence,d=1-10=0.0261322146andpsp-73/20d=10=0.000223872.s7.2a=-20log(1-d)anda=-20log(d).p10ps10s(a)d=0.01andd=0.01.Hence,a=-20log(1-0.01)=0.0873dBandpsp10a=-20log(0.01)=40dB.s10(b)d=0.035andd=0.023.Hence,a=-20log(1-0.035)=0.3094537dBandpsp10a=-20log(0.023)=32.76544dB.s1022jw2jwjw2jwjw7.3G(z)=H(z)orequivalently,G(e)=H(e).G(e)=H(e)=H(e).LetdandpjwddenotethepassbandandstopbandripplesofH(e),respectively.Also,letd=2dsp,2p,jwandddenotethepassbandandstopbandripplesofG(e),respectively.Thens,222Md=1-(1-d),andd=(d).ForacascadeofMsections,d=1-(1-d),andp,2ps,2sp,MpMd=(d).s,Ms7.4jwHLP(e)jwH(e)HP1+dp1–dp1+dp1–dpddssww–p0p–p0p–ws–wpwpws–(p–wp)–(p–ws)p-wsp-wpTherefore,thepassbandedgeandthestopbandedgeofthehighpassfilteraregivenbyw=p-w,andw=p-w,respectively.p,HPps,HPs7.5236
jwH)jwLP(eG(e)1+dp1+dp1–dp1–dpdsdsww–p0p–p0wop–ws–wpwpwswo-wswo-wpwo+wpwo+wsNotethatG(z)isacomplexbandpassfilterwithapassbandintherange0£w£p.Itspassbandedgesareatw=w±w,andstopbandedgesatw=w±w.Arealp,BPops,BPoscoefficientbandpasstransferfunctioncanbegeneratedaccordingtojw–jwG(z)=H(eoz)+H(eoz)whichwillhaveapassbandintherange0£w£pandBPLPLPanotherpassbandintherange–p£w£0.Howeverbecauseoftheoverlapofthetwospectraasimpleformulaforthebandedgescannotbederived.¥¥7.6(a)hp(t)=ha(t)×p(t)wherep(t)=åd(t-nT).Thus,hp(t)=åha(nT)d(t-nT).n=-¥n=-¥¥-stWealsohave,g[n]=ha(nT).Now,Ha(s)=òha(t)edtand-¥¥¥¥¥-st-st-snTHp(s)=òhp(t)edt=åòha(nT)d(t-nT)edt=åha(nT)e.-¥n=-¥-¥n=-¥¥¥-n-nComparingtheaboveexpressionwithG(z)=åg[n]z=åha(nT)z,weconcluden=-¥n=-¥thatG(z)=Hp(s)1.s=lnzT1¥-j(2pkt/T)WecanalsoshowthataFourierseriesexpansionofp(t)isgivenbyp(t)=åe.Tk=-¥æ1¥ö1¥-j(2pkt/T)-j(2pkt/T)Therefore,hp(t)=çåe÷ha(t)=åha(t)e.Hence,èTk=-¥øTk=-¥1¥2pktæöHp(s)=åHaès+jø.Asaresult,wehaveTTk=-¥1¥2pktæö(1):G(z)=åHaès+jø.TTs=1lnzk=-¥TsT(b)Thetransformationfroms-planetoz-planeisgivenbyz=e.Ifweexpresss=sjwsoTjWoTo+jwo,thenwecanwritez=re=ee.Therefore,237
ì<1,forso<1,ïz=í=1,forso=1,Orinotherwords,apointintheleft-halfs-planeismappedontoaïî>1,forso>1.pointinsidetheunitcircleinthez-plane,apointintheright-halfs-planeismappedontoapointoutsidetheunitcircleinthez-plane,andapointonthejw-axisinthes-planeismappedontoapointontheunitcircleinthez-plane.Asaresult,themappinghasthedesirablepropertiesenumeratedinSection7.1.3.2pk(c)However,allpointsinthes-planedefinedbys=so+jwo±j,k=0,,1,2,K,areT2pkj(Wo±)Tmappedontoasinglepointinthez-planeasz=esoTeT=esoTejWoT.ThemappingisillustratedinthefigurebelowjWImz3pTpTsRez-11p–T3p–Ts-planez-planeNotethatthestripofwidth2p/Tinthes-planeforvaluesofsintherange-p/T£W£p/Tismappedintotheentirez-plane,andsoaretheadjacentstripsofwidth2p/T.Themappingismany-to-onewithinfinitenumberofsuchstripsofwidth2p/T.ItfollowsfromtheabovefigureandalsofromEq.(1)thatifthefrequencyresponsepjw1wHa(jW)=0forW³,thenG(e)=Ha(j),forw£p,andthereisnoaliasing.TTTjwjWT(d)Forz=e=e.Orequivalently,w=WT.A-at7.7H(s)=.Thenh(t)=Aem(t)wherem(t)istheunitstepfunction.Hence,as+aa¥-anT-anT-nA-aTg[n]=ha(nT)=Aem[n].Thus,G(z)=Aåez=1-e-aTz-1,providede<1.n=0AA7.8FromProblem7.7werecallthatifH(s)=thenG(z)=.Wecanexpressas+a1-e-aTz-1l1æ1ö1æ1öH(s)==çç÷÷-çç÷÷.Hence,al2+(s+b)22jès+b-jlø2jès+b+jlø238
1æ11ö1æ-e-bTe-jlTz-1+e-bTejlTz-1öG(z)=çç-÷÷=ç÷2jè1-e-(b-jl)Tz-11-e-(b+jl)Tz-1ø2jçè(1-e-(b-jl)Tz-1)(1-e-(b+jl)Tz-1)÷øæ-1-bTjlT-jlTö-1-bT=1çze(e-e)÷=zesin(lT)2jç1-2z-1e-bTcos(lT)+e-2bTz-2÷1-2z-1e-bTcos(lT)+e-2bTz-2èø-bTzesin(lT)=.2-bT-2bTz-2zecos(lT)+es+b1æ1ö1æ1ö7.9H(s)==çç÷÷+çç÷÷.Henceal2+(s+b)22ès+b+jlø2ès+b-jlø1æ11ö1æ1-e-bTejlTz-1+1-e-bTe-jlTz-1öG(z)=çç+÷÷=ç÷2è1-e-(b+jl)Tz-11-e-(b-jl)Tz-1ø2çè1-2z-1e-bTcos(lT)+e-2bTz-2÷ø-1-bT2-bT1-zecos(lT)z-zecos(lT)==.-1-bT-2bT-22-bT-2bT1-2zecos(lT)+ezz-2zecos(lT)+estsnT7.10Assumeha(t)iscausal.Now,ha(t)=òHa(s)eds.Henceg[n]=ha(nT)==òHa(s)eds.Therefore,¥¥¥-nsnT-n-nsnTHa(s)G(z)=åg[n]z=åòHa(s)ezds=òHa(s)åzeds=òsT-1ds.1-ezn=0n=0n=0éH(s)ùaHenceG(z)=åResiduesêsT-1ú.ë1-ezûallpolesofH(s)aA7.11Ha(s)=.Thetransferfunctionhasapoleats=-a.Nows+aéAùAAG(z)=Residueêú==.ats=–aêë(s+a)(1-esTz-1)úû1-esTz-11-e-aTz-1s=–a7.12(a)Applyingpartial-fractionexpansionwecanexpress16(s+2)é-1/80.0625-j0.18750.0625+j0.1875ùHs)==16ê++úa(s+3)(s2+2s+5)êës+3s+1-j2s+1+j2úûé117ùê-s+ú888-22s+14-22(s+1)6´2=16ê+ú=+=+.ês+3s2+2s+5ús+3(s+1)2+22s+3(s+1)2+22(s+1)2+22êëúûUsingtheresultsofProblems7.7,7.8and7.9wethusarriveat2(z2-ze-2Tcos(2T))-2T26zesin(2T)G(z)=-++.ForT=0.2,1-e-3Tz-1z2-2ze-2Tcos(2T)+e-4Tz2-2ze-2Tcos(2T)+e-4T239
2(z2-ze-0.4cos(0.4))-0.4T26zesin(0.4)wethengetG(z)=-++a1-e-0.6z-1z2-2ze-0.4cos(0.4)+e-0.8z2-2ze-0.4cos(0.4)+e-0.8222(z-0.6174z)1.5662z=-++1-0.5488z-1z2-1.2348z+0.4493z2-1.2348z+0.4493-1-122-1.2348z1.5662z=-++1-0.5488z-11-1.2348z-1+0.4493z-21-1.2348z-1+0.4493z-2-122+0.3314z=-+1-0.5488z-11-1.2348z-1+0.4493z-2(b)Applyingpartial-fractionexpansionwecanexpress31312-j+j4s+10s+812222H(s)==++b(s2+2s+3)(s+1)s+1s+1-j2s+1+j213s+513(s+1)2(2)=+=++.s+1s2+2s+3s+1(s+1)2+(2)2(s+1)2+(2)2UsingtheresultsofProblems7.7,7.8and7.9wethusarriveat2-T2(ze-Tsin(2T))13(z-zecos(2T)G(z)=++b1-e-Tz-1z2-2ze-Tcos(2T)+e-2Tz2-2ze-Tcos(2T)+e-2T2-0.2-0.213(z-zecos(0.22))2(zesin(0.22))=++1-e-0.2z-1z2-2ze-0.2cos(0.22)+e-0.4z2-2ze-0.2cos(0.22)+e-0.4-0.2-1-0.2-113(1-ecos(0.22)z)2(esin(0.22))z=++1-e-0.2z-11-2e-0.2cos(0.22)z-1+e-0.4z-21-2e-0.2cos(0.22)z-1+e-0.4z-2-1-113-2.3585z0.32314z=++1-0.81873z-11-1.5724z-1+0.67032z-21-1.5724z-1+0.67032z-2-113-2.03545z=+.1-0.81873z-11-1.5724z-1+0.67032z-2(c)Applyingpartial-fractionexpansionwecanexpress323s+7s+10s+7-1.8s+0.64.8s+5.2H(s)==+c(s2+s+1)(s2+2s+3)s2+s+1s2+2s+3-1.8(s+0.5)3(3/2)4.8(s+1)0.2828(2)=+++(s+0.5)2+(3/2)2(s+0.5)2+(3/2)2(s+1)2+(2)2(s+1)2+(2)2UsingtheresultsofProblems7.7,7.8and7.9wethusarriveat-1.8(z2-ze-0.5Tcos(0.866T))-0.5T3zesin(0.866T)G(z)=+cz2-2ze-0.5Tcos(0.866T)+e-Tz2-2ze-0.5Tcos(0.866T)+e-T4.8(z2-ze-Tcos(2T))-T0.2828zesin(2T)++z2-2ze-Tcos(2T)+e-2Tz2-2ze-Tcos(2T)+e-2T240
2-1.8z+4.6811z0.2955z=+z2-9.36226z+0.8187z2-9.36226z+0.818724.8z+3.77376z0.071414z++z2-1.5724z+0.67032z2-1.5724z+0.6703222-1.8z+4.9766z4.8z+3.845174z=+.z2-9.36226z+0.8187z2-1.5724z+0.670327.13(a)ComparingG(z)withEq.(7.148)wecanwritea2323G(z)=+=+.Hence,a=3andb=4.a1-e-0.9z-11-e-1.2z-11-e-aTz-11-e-bTz-123Therefore,H(s)=+as+3s+4.(b)ComparingwithEq.(7.152)weobservebT=0.6andlT=0.9.ForT=0.3weget1s+3b=1/3andl=3.Thus,H(s)=.a12(s+)+93æ1+sö2æ1+sö5ç÷+4ç÷-1è1-søè1-sø12s7.14(a)Ha(s)=Ga(z)z=1+s=2=2.æ1+söæ1+sö7s+16s+121-s8ç÷+4ç÷è1-søè1-søéæ1+sö3æ1+sö2æ1+söù8êç÷+3ç÷+3ç÷+1úêëè1-søè1-søè1-søúû7s3+21s2+21s+15(b)Hb(s)=Gb(z)z=1+s=é2ù=(2s+4)(4s2+8s+16).1-séæ1+söùêæ1+söæ1+söúê3ç÷+1ú7ç÷+6ç÷+3ëè1-søûêëè1-søè1-søúû3-37.15Fortheimpulseinvariancedesignw=WT=2p´0.5´10´0.5´10=0.5p.FortheppæWTö-1çp÷-1-1bilineartransformationmethoddesignw=2tan=2tan(pFT)=2tan(0.25p)=pç2÷pèø0.4238447331p.wp0.3p7.16Fortheimpulseinvariancedesign2pF==orf=1.5kHz.ForthebilinearpT10-4p4transformationmethoddesignf=10tan(0.15p)/p=1.62186kHz.p7.17ThepassbandandthestopbandedgesoftheanaloglowpassfilterareassumedtoW=0.25ppandW=0.55p.Therequirementstobesatisfiedbytheanaloglowpassfilterarethuss20logH(j0.25p)³-0.5dBand20logH(j0.55p)£-15dB.10a10a241
222Froma=20log(1+e)=0.5weobtaine=0.1220184543.Froma=10log(A)=15p10s102weobtainA=31.6227766.FromEq.(5.??),theinversediscriminationratioisgivenby21A-1==15.841979andfromEq.(5.??)theinversetransitionratioisgivenbyke11Ws==2.2.SubstitutingthesevaluesinEq.(5.??)weobtainkWplog(1/k)log(15.841979)10110N===3.503885.WechooseN=4.log(1/k)log(2.2)10102NæWöçp÷22FromEq.(5.??)wehave=e.SubstitutingthevaluesofW,N,andewegetçW÷pècøW=1.3007568(W)=1.021612.cpUsingthestatement[z,p,k]=buttap(4)wegetthepolesofthe4-thorderButterworthanalogfilterwitha3-dBcutoffat1rad/sasp=-0.3827+j0.9239,p=-0.3827-j0.9239,12p=-0.9239+j0.3827,andp=-0.9239-j0.3827.Therefore3411H(s)==.an(s-p)(s-p)(s-p)(s-p)(s2+0.7654s+1)(s2+1.8478s+1)1234NextweexpandH(s)inapartial-fractionexpansionusingtheM-fileresidueandarriveatan-0.9238729s-0.70713230.9238729s+1.7071323H(s)=+.WenextdenormalizeH(s)toans2+0.7654s+1s2+1.8478s+1anmovethe3-dBcutofffrequencytoW=1.021612usingtheM-filelp2lpresultingincæsöHa(s)=Hançè÷ø1.021612-0.943847s-0.7380390.943847s+1.78174665=+s2+0.781947948s+1.0437074244s2+1.887749436s+1.0437074244-0.943847s-0.7380390.943847s+1.78174665=+(s+0.390974)2+(0.9438467)2(s+0.94387471)2+(0.39090656)2MakinguseoftheM-filebilinearwefinallyarriveat22-0.943847z+0.68178386z0.943847z-0.25640047zG(z)=+CHECKz2-1.363567724z+0.4575139z2-0.77823439z+0.1514122p7.18FornoaliasingT£.FigurebelowshowsthemagnituderesponsesofthedigitalfiltersWcH1(z)andH2(z).242
jwjwH1(e)H2(e)21ww–p0p–p0p(a)ThemagnituderesponsesofthedigitalfiltersG1(z)andG2(z)areshownbelow:jwjwG)G(e)1(e23/21ww–p0p–p0p(b)AscanbeseenfromtheaboveG1(z)isamulti-bandfilter,whereas,G2(z)isahighpassfilter.RAìüìïRüïk-1Ha(s)-1Ak7.19a)Ha(s)=å.Henceha(t)=Líý=Líåý.k=0s-akîïsþïïîk=0s(s-ak)ïþìRæöüRA-1ïAkç11÷ïkatThush(t)=Líå-ý=å(ek-1)m(t).aïaçès-as÷øïaîk=0kkþk=0kRAkanTHenceg[n]=h(nT)=å(ek-1)m(nT).Asaresult,maak=0k¥RAæöRAæ-1aTö-nk11kz(ek-1)Gm(z)=ågm[n]z=åçaT-1--1÷=åççaT-1-1÷÷.n=-¥k=0akè1-ekz1-zøk=0akè(1-ekz)(1-z)øNowthetransferfunctionG(z),whichisthez-transformoftheimpulseresponseg[n],is-1relatedtothez-transformofthestepresponseg[n]byG(z)=(1-z)G(z).HencemmRAæ-1aTökz(ek-1)G(z)=åççaT-1÷÷.k=0akè1-ekzøHa(jw)1(b)Now,»0ifw³.Considerthedigitaltransferfunctionobtainedbyjw2TH(s)asamplingF(s)=.Nowthisdigitaltransferfunctionwouldcorrespondtothez-stransformofthesampledstepresponseofHa(s).Thus¥jw1w2pk1wHa(jw/T)Gm(e)=åF(jT+jT)=F(jT)=..TTjwk=-¥243
-1jwT-jwTjwT-jwTHa(jw)SinceG(z)=(1-z)G(z),henceG(e)=(1-e)G(e)=(1-e).mmjwT-jwTjwTSincewT<<1,therefore1-e»jwT.Thus,G(e)@H(jw).a1-117.20Themappingisgivenbys=(1-z)orequivalently,byz=.ForT1-sT121s=so+jWo,z=.Therefore,z=.Hence,z<1,1-soT-jWoT(1-soT)2+(WoT)2forso<0.Asaresult,astableHa(s)resultsinastableH(z)afterthetransformation.21However,forso=0,z=whichisequalto1onlyforWo=0.Hence,onlythe1+(W2oT)pointWo=0onthejW-axisinthes-planeismappedontothepointz=1ontheunitcircle.Consequently,thismappingisnotusefulforthedesignofdigitalfiltersbyanalogfiltertransformation.7.21Ha(s)iscausalandstableandHa(s)£1"s,Now,G(z)=Ha(s)2æç1-z-1ö÷.Thus,G(z)s=Tç1+z-1÷èøiscausalandstable.Now,jwæ2öG(e)=Ha(s)2æç1-e-jwö÷=Ha(s)2=HaçèjTtan(w/2)÷ø.Therefore,s=s=jtan(w/2)Tç1+e-jw÷Tèøjwæ2öG(e)=Haçjtan(w/2)÷£1forallvaluesofw.Hence,G(z)isaBRfunction.èTøjBWBW7.22H(jW)=.Thus,H(jW)=.Itcanbeseenthata(W2-W2)+jBWa(W2-W2)2+B2W2ooBWoH(j0)=0,H(j¥)=0andH(jW)==1.HenceH(s)isananalogbandpassaaa022aBWotransferfunction.ApplyingbilineartransformationtoH(s)wegetaæz-1öBç÷2èz+1øB(z-1)G(z)=Ha(s)s=z-1=2=222z+1æz-1ö+Bæz-1ö2(z-1)+B(z-1)+(z+1)ç÷ç÷+Woèz+1øèz+1ø2B(z-1)=(1+B+W2)z2-2(1-W2)z++(1-B+W2)ooo-2B1-z=×.FromEqs.(7.36)wehave1+B+W2æ2(1-W2)öæ1-B+W2öo1-ço÷z-1+ço÷z-2ç1+B+W2÷ç1+B+W2÷èoøèoø244
1-B+W21-W21-B+W22(1+W2)ooooa=andb=.Now1+a=1+=and1+B+W21+W21+B+W21+B+W2oooo1-B+W22B1-a1-z-2o1-a=1-=.Hence,G(z)=×whichisthe1+B+W21+B+W221-b(1+a)z-1+az-2oosameasthatgiveninEq.(4.113).-1-21+a1-2bz+z7.23G(z)=×.Forb=cosw0thenumeratorofG(z)becomes21-b(1+a)z-1+az-21-2cosw-1-2jw0-1-jw0-1±jw00z+z=(1-ez)(1-ez)whichhasrootsatz=e.TheNjw0-N-jw0-NnumeratorofG(z)isthengivenby(1-ez)(1-ez)whoserootsareobtainedN±jw0j(2pn±w0)/Nbysolvingtheequationz=e,andaregivenbyz=e,0£n£N-1.NHenceG(z)hasNcomplexconjugatezeropairslocatedontheunitcircleatanglesof2pn±w0radians,0£n£N-1.Nw0=p/2,thereare2Nequallyspacedzerosontheunitcirclestartingatw=p/2N.1N(z)7.24(a)H(z)=[1+A4(z)]=where2D(z)æa1-b1(1+a1)z-1+z-2öæa2-b2(1+a2)z-1+z-2öA4(z)=ç-1-2÷ç-1-2÷.Therefore,è1-b1(1+a1)z+a1zøè1-b2(1+a2)z+a2zø1-1-2-1-2N(z)=[(a1-b1(1+a1)z+z)(a2-b2(1+a2)z+z)2-1-2-1-2+(1-b1(1+a1)z+a1z)(1-b2(1+a2)z+a2z)]1+a1a2(1+a1)(1+a2)(b1+b2)-12[a1+a2+b1b2(1+a1)(1+a2)]-2={1-z+z21+a1a21+a1a2(1+a1)(1+a2)(b1+b2)-3-4-z+z}whichisseentobeamirror-image1+a1a2-1-2-3-4polynomial.WecanexpressN(z)=a(1+b1z+b2z+b1z+z),where(1+a1)(1+a2)(b1+b2)2[a1+a2+b1b2(1+a1)(1+a2)](1):b1=-,(2):b2=,and1+a1a21+a1a21+a1a2(b)(3):a=.2jw(c)forz=e,wecanwritejw-jw-j2w-j3w-j4w-j2wN(e)=a(1+b1e+b2e+b1e+e)=ae(b2+2b1cosw+2cos2w).245
jwiNowN(e)=0,fori=1,2.Fori=1weget(4):b2+2b1cosw1+2cos2w1=0,andfori=2weget(5):b2+2b1cosw2+2cos2w2=0.SolvingEqs.(4)and(5)weget(6):b1=-2(cosw1+cosw2),and(7):b2=2(2cosw1cosw2+1).(1+a1)(1+a2)(b1+b2)FromEqs.(1)and(6)wehave(8):=2(cosw1+cosw2),and1+a1a2fromEqs.(2)and(7)wehave2[a1+a2+b1b2(1+a1)(1+a2)](9):=2(2cosw1cosw2+1).Substituting1+a1a21-tan(B1/2)1-tan(B2/2)a1=anda2=,andafterrearrangementweget1+tan(B1/2)1+tan(B2/2)éæB1öæB2öùD(10):b1+b2=(cosw1+cosw2)ê1+tanèøtanèøú=q1,andë22ûéæB1öæB2öùæB1öæB2öD(11):b1b2=ê1+tanèøtanèøúcosw1cosw2+tanèøtanèø=q2.ë22û22Theabovetwononlinearequationscanbesolvedresultinginq21±q1-4q2q2b1=andb2=.2b1(d)Forthedoublenotchfilterwiththefollowingspecifications:w1=0.3p,w2=0.5p,B1=0.1p,andB2=0.15p,wegetthefollowingvaluesfortheparametersofthenotchfiltertransferfunction:a1=0.7265,a2=0.6128,b1=0.5397,andb2=0.0705.10.80.60.40.2000.20.40.60.81W7.25Azero(pole)ofHLP(z)isgivenbythefactor(z-zk).Afterapplyingthelowpass-to-ˆz-alowpasstransformation,thisfactorbecomes-z,andhencethenewlocationofthe1-aˆzkzero(pole)isgivenbytherootsoftheequation246
a+zzˆ-a-z+azzˆ=(1+az)zˆ-(a+z)=0orzˆ=k.Forzk=-1,kkkkk1+azka-1zˆ==-1.k1-a2-b+aˆz-ˆz2ab7.26Thelowpass-to-bandpasstransformationisgivenbyz®wherea=and1-azˆ+bˆz2b+1b-1b=.Azero(pole)ofHLP(z)isgivenbythefactor(z-zk).Afterapplyingtheb+12-b+azˆ-ˆzlowpass-to-bandpasstransformation,thisfactorbecomes-z,andhencethenew1-azˆ+bzˆ2klocationofthezero(pole)ofthebandpasstransferfunctionisgivenbytherootsofthea(1+z)b+z22kkequation(1+bz)z-a(1+z)z+(b+z)=0,orz-z+=0,whosesolutionkkk1+bz1+bzkk2a(1+z)éa(1+z)ùæb+zöisgivenbyzˆ=k±êkú-çk÷.Forz=-1,zˆ=±1.k2(1+bz)êë2(1+bz)úûçè1+bz÷økkkkkæw-wˆösinçcc÷çè2÷øsin(-0.075p)7.27Forw=0.42pandwˆ=0.57pwehavea===-0.233474.Thus,ccæw+wˆösin(0.495p)sinçcc÷çè2÷øæzˆ-1ö2-a0.223ç1+÷ç-1÷è1-azˆøH(zˆ)=G(z)-1ˆz-1-a=LPLPz=-1æzˆ-1öæˆz-1ö21-aˆz-a-a1-0.2952ç÷+0.187ç÷çè1-aˆz-1÷øçè1-azˆ-1÷ø2-120.223(1-a)(1+zˆ)=(1+0.2952a+0.187a2)+[-2a-0.2952(1+a2)-0.374a]zˆ-1+(a2+0.2952a+0.187)zˆ-2-120.33929(1+ˆz)=0.94127+0.24298zˆ-1+0.17259ˆz-2247
0H(z)-10LP-20G(z)LP-30-4000.20.40.60.81w/pæw+wˆöcosçcc÷çè2÷øcos(0.515p)7.28Forw=0.42pandwˆ=0.61pwehavea=-=-=-0.0492852.ccæw+wˆöcos(-0.95p)cosçcc÷çè2÷øæzˆ-1ö2+a0.223ç1-÷ç-1÷è1+azˆøH(zˆ)=G(z)-1zˆ-1+a=HPLPz=--1æzˆ-1öæzˆ-1ö21+azˆ+a+a1+0.2952ç÷+0.187ç÷çè1+aˆz-1÷øçè1+azˆ-1÷ø2-120.223(1-a)(1-zˆ)=(1+0.2952a+0.187a2)+[2a+0.2952(1+a2)+0.374a]zˆ-1+(a2+0.2952a+0.187)zˆ-2-120.20156(1-zˆ)=.1.015+0.41292zˆ-1+0.20398zˆ-20-10H(z)G(z)HPLP-20-30-4000.20.40.60.81w/p7.29Sincethepassbandedgefrequenciesarenotspecified,weassumethedesired3-dBbandwidthofthebandpassfilterHBP(z)iswˆc2-wˆc1=wc,wherewcisthe3-dBcutofffrequencyofGLP(z).248
Moreover,thedesiredcenterfrequencywˆcisrelatedtothepassbandedgefrequenciesthroughwˆwˆwˆwˆ2=wˆc1wˆc2.Hence,c=c1c2orc222222(wˆc2+wˆc1)=(wˆc2-wˆc1)+4wˆc2wˆc1=wc+4wˆcorwˆc2+wˆc1=wc+4wˆc.FromEq.(7.48)thelowpass-to-bandpasstransformationisgivenbyæw22öc+4wˆcæwˆc2+wˆc1öcosç÷-1-1-2cosç÷ç2÷-1-1z-aaz-zè2øèøz®-z=,wherea==.1-az-11-az-1æwˆc2-wˆc1öæwcöcosç÷cosè2øè2øForwc=0.42pandwˆc=0.45p,a=0.013564Then,HBP(z)=GLP(z)-1-2-1dz-zz®1-dz-1-220.223(1-z)=1+(-2a-0.2952a)z-1+[a2+0.2952(1+a2)+0.187a2]z-2+(-0.2952a-0.374a)z-3+0.187z-4-220.1494(1-z)=.1-0.036718z-1+0.70738z-2-0.018832z-3+0.3407z-40H(z)BP-10G(z)-20LP-30-4000.20.40.60.81w/pæw-wˆösinçpp÷ç2÷èøsin(0.05p)7.30wp=0.6p,andwˆp=0.5p.Thus,a===0.15838444.æw+wˆösin(0.55p)sinçpp÷ç2÷èø-1N(z)Therefore,H(z)=G(z)-1z-a=whereHPHPz®-1D(z)1-az3-13-13N(z)=0.0916(1+a)(1-z)=0.14238(1-z)and23D(z)=(1-0.7601a+0.7021a-0.2088a)232-1+[-3a+0.7601(1+2a)-0.7021(2a+a)+0.6264a]z249
232-2+[3a-0.7601(2a+a)+0.7021(1+2a)-0.6264a]z32-3+(-a+0.7601a-0.7021a+0.2088)z-1-2-3=0.896395+0.113602498z+0.469574z+0.1126927z.50-5H(z)G(z)-10HPHP-15-20-2500.20.40.60.81w/p-20.136728736(1-z)7.31HBP(z)=.Thelowpass-to-lowpasstransformationis1-0.53353098z-1+0.726542528z-2æwo-wˆoö-1sinç÷-1z-aè2øgivenbyz®wherea=wherewo=0.4pandwˆo=0.5p.1-az-1æwo+wˆoösinç÷è2øThus,a=–0.15838.ææ-1ö2öz-a0.136728736çç1-ç-1÷÷÷èè1-azøøz-1-aGLP(z)=HLP(z)-1=z=21-az-1æz-1-aöæz-1-aö1-0.5335098ç-1÷+0.726542528ç-1÷è1-azøè1-azø2-120.136728736(1-a)(1+zˆ)=(1+0.533531a+0.72654253a2)+[-2a-0.533531(1+a2)-1.4531a]zˆ-1+(a2+0.533531a+0.72654253)ˆz-2-20.1333(1-zˆ)=.0.93372+2.7299´10-9´zˆ-1+0.66713zˆ-2250
50-5H(z)G(z)BPBP-10-15-20-2500.20.40.60.81w/pæ60ö7.32ThenotchfilterdesignedinExample7.8hasnotchfrequencyatwo=2pç÷=0.3panditsè400ø-1-20.940809-1.105987z+0.94089ztransferfunctionisgivenbyGBS(z)=.Thedesired1-1.105987z-1+0.881618z-2æ100önotchfrequencyofthetransformedfilteriswˆo=2pç÷=0.5p.Thelowpass-to-lowpassè400øæwo-wˆoö-1sinç÷-1z-aè2øtransformationtobeusedisgivenbyz®wherea==1-az-1æwo+wˆoösinç÷è2ø–0.32492.Thedesiredtransferfunctionisthusgivenby2æz-1-aöæz-1-aö0.940809-1.105987ç-1÷+0.94089ç-1÷-1è1-azøè1-azøHBS(z)=GBS(z)-1z-a=z=21-az-1æz-1-aöæz-1-aö1-1.105987ç-1÷+0.881618ç-1÷è1-azøè1-azø(0.940809+1.105987a+0.940809a2)+[-3.7632a-1.105987(1+a2)]z-1+(0.940809a2+1.105987a+0.940809)z-2=(1+1.105987a+0.940809a2)+[-2a-1.105987(1+a2)-1.7632a]z-1+(a2+1.105987a+0.881618)z-2-7-1-20.68078+3.4367´10z+0.68078z=.0.73997+3.4367´10-7z-1+0.62783z-2251
50-5G(z)H(z)-10BSBS-15-20-2500.20.40.60.81w/p-130.0916(1-z)7.33G(z)=.Nowwp=0.6p,andwˆp=0.5p.Thus,HP1+0.7601z-1+0.7021z-2+0.2088z-3æw+wˆöcosçpp÷ç2÷èøcos(0.55p)a=-=-=0.15838444.Therefore,æw-wˆöcos(0.05p)cosçpp÷ç2÷èøz-1-aN(z)3-13HHP(z)=GHP(z)-1=,whereN(z)=0.0916(1+a)(1+z),andz®--1D(z)1-az23D(z)=(1-0.7601a+0.7021a-0.2088a)232-1+[-3a+0.7601(1+2a)-0.7021(2a+a)+0.6264a]z232-2+[3a-0.7601(2a+a)+0.7021(1+2a)-0.6264a]z32-3+(-a+0.7601a-0.7021a+0.2088)z5H(z)G(z)0LPHP-5-10-15-20-2500.20.40.60.81w/pjw7.34TheidealL-banddigitalfilterHML(z)withanidealfrequencyresponsegivenbyHML(e)=Akforwk-1£w£wk,k=1,2,K,L,canbeconsideredassumofLidealbandpassfilterskk0Lwithcutofffrequenciesatw=wandw=w,wherew=0andw=p.Nowfromc1k-1c2kc1c2Eq.(7.90)theimpulseresponseofabandpassfilterisgivenbyhBP[n]=sin(wc2n)sin(wc1n)kæsin(wkn)sin(wk-1n)ö-.Therefore,hBP[n]=Akç-÷.Hence,pnpnèpnpnø252
LLkæsin(wkn)sin(wk-1n)öhML[n]=åhBP[n]=åAkç-÷èpnpnøk=1k=1L-1L-1æsin(w1n)sin(0n)ösin(wkn)æsin(wkn)sin(wk-1n)ö=A1ç-÷+åAk-åAkç-÷èpnpnøpnèpnpnøk=2k=2æsin(wn)sin(wn)öLL-1+ALç-÷èpnpnøL-1L-1sin(wn)sin(wn)sin(wn)sin(wn)1kk-1L-1=A1+åAk-åAk-ALpnpnpnpnk=2k=2L-1Lsin(wn)sin(wn)kk-1=åAk-åAk.pnpnk=1k=2sin(wn)LSincewL=p,sin(wLn)=0.WeaddatermALtothefirstsumintheabovepnexpressionandchangetheindexrangeofthesecondsum,resultinginLL-1sin(wn)sin(wn)kkhML[n]=åAk-åAk+1.pnpnk=1k=1sin(wn)LFinally,sinceAL+1=0,wecanaddatermAL+1tothesecondsum.ThisleadstopnLLLsin(wn)sin(wn)sin(wn)kkkhML[n]=åAkpn-åAk+1pn=å(Ak-Ak+1)pn.k=1k=1k=1jwìj,-p0.SincehDIF[n]=–hDIF[–n],thetruncatedimpulseîïnresponseisaType3linear-phaseFIRfilter.ThemagnituderesponsesoftheabovedifferentiatorforseveralvaluesofMaregivenbelow:ìwï1-c,forn=M,ïpïïsin(wc(n-m))7.38N=2M+1.hˆ[n]=í–,ifn¹M,0£nlk,l¹ibM+21iDefineci=M+2.Itcanbeshownbyinductionthatci=Õ.Therefore,n=1coswi-coswnÕbrn¹ir=1r¹iM+2åbiD(wi)cD(w)+cD(w)+L+cD(w)i=11122M+2M+2e==.M+2iM+1(-1)c1c2cM+2(-1)åbi-+L+P(w)P(w)P(w)P(w)i=1i12M+27.64ItfollowsfromEq.(7.67)thattheimpulseresponseofanidealHilberttransformerisanantisymmetricsequence.Ifwetruncateittoafinitenumberoftermsbetween-M£n£Mtheimpulseresponseisoflength(2M+1)whichisodd.HencetheFIRHilberttransformerobtainedbytruncationandsatisfyingEq.(7.143)cannotbesatisfiedbyaType4FIRfilter.273
N-1N-1(-1n(-n(æ-a+zöP(z)7.65(a)X(z)=åx[n]z.X(z)=X(z)-a+z(-1=åx[n]ç(-1÷=(,n=0z-1=n=0è1-azøD(z)(-11-azN-1N-1((-1(-1N-1-n(-1nwhereP(z)=åp[n]z=åx[n](1-az)(-a+z),andn=0n=0N-1((-1(-1N-1D(z)=åd[n]z=(1-az).n=0((((P(z)P[k](((b)X[k]=X(z)(j2pk/N=(=(,whereP[k]=P(z)(j2pk/Nisthez=eD(z)(j2pk/ND[k]z=ez=e((N-pointDFTofthesequencep[n]andD[k]=D(z)(j2pk/NistheN-pointDFTofthez=esequenced[n].TT(c)LetP=[p[0]p[1]Kp[N-1]]andX=[x[0]x[1]Kx[N-1]].Withoutanylossofgenerality,assumeN=4inwhichcase3((-n23P(z)=åp[n]z=(x[0]-ax[1]+ax[2]-ax[3])n=222(-1+(-3ax[0]+(1+2a)x[1]-a(2+a)x[2]+3ax[3])z222(-2+(3ax[0]-a(2+a)x[1]+(1+2a)x[2]-3ax[3])z32(-3(-1+(-ax[0]ax[1]-ax[2]+ax[3])z.Equatinglikepowersofzwecanwriteép[0]ùé1-aa2-a3ùéx[0]ùêp[1]úê222úêx[1]úêúê-3a1+2a-a(2+a)3aúêúP=Q×Xor=.Itcanbeseenthattheêp[2]úê3a2-a(2+a2)1+2a2-3aúêx[2]úêúêúêúëp[3]ûêë-a3a2-a1úûëx[3]ûelementsqr,s,0£r,s£3,ofthe4´4matrixQcanbedeterminedasfollows:s(i)Thefirstrowisgivenbyq0,s=(-a),3r3!r(ii)Thefirstcolumnisgivenbyqr,0=Cr(-a)=(-a),andr!(3-r)!(iii)theremainingelementscanbeobtainedusingtherecurrencerelationqr,s=qr-1,s-1-aqr,s-1+aqr-1.s.Inthegeneralcase,weonlychangethecomputationoftheelementsofthefirstcolumnusingN-1r(N-1)!rtherelationqr,0=Cr(-a)=(-a),r!(N-1-r)!274
M7.1UsingtheM-filebuttapwefirstdesigna4-thorderanalogBesselfilterwhosetransfer1functionisgivenbyH(z)=.Apartial-fractionexpansions4+3,1239s3+4.3916s2+3.2011s+11.0393s+1.84701.0393s+2.3615ofH(z)usingtheM-fileresidueyieldsH(z)=-+s2+1.3144s+1.1211s2+1.8095s+8.9199wherewehaveusedtheM-fileresidueagaintocombinethecomplexconjugatepole-pairs.INCOMPLETEM7.2Givenspecifications:F=4kHz,F=20kHz,F=80kHz,a=0.5dBanda=45dB.psTpsUsingEqs.(7.7)and(7.8)weobtainthenormalizedbandedgesasw=0.1p=0.31416andpw=0.5p=1.5708.LetT=2.FromEq.(7.26),thebandedgesoftheanalogprototypearesW=tan(w/2)=0.15838andW=tan(w/2)=1FromEq.(5.29)theinversetransitionratioppss1Wsis==6.31375.kWpæ1ö2æ1ö20log10çç2÷÷=-0.5whichyieldse=0.1220184.Similarly,20log10çè÷ø=-45whichè1+eøA2yieldsA=31622.7766.FromEq.(5.5.30theinversediscriminationratioisgivenby21A–131621.7766===509.073363.Hence,fromEq.(5.33),theorderofthelowpasske20.122018451log(1/k)log(509.073363)10110ButterworthfilterisgivenbyN===3.38226.WechooseN=4log(1/k)log(6.3137515)1010astheorder.Nextwedeterminethe3-dBcutofffrequencyWbymeetingexactlythec82stopbandedgespecifications.FromEq.(5.??a),wehave(Ws/Wc)=A-1=31621.7766orWsW==0.273843W=0.273843.c(A2-1)1/8sUsingbuttapwedeterminethenormalizedanalogButterworthtransferfunctionof4-thorderwitha3-dBcutofffrequencyatW=1whichisc1Han(s)=22.WedenormalizeHan(s)tomoveWcto(s+0.76536686s+1)(s+1.847759s+1)æsö0.273843leadingtoHa(s)=Hançè÷ø0.2738431=éæsö2æsöùéæsö2æsöùêç÷+0.76536686ç÷+1úêç÷+1.847759ç÷+1úêëè0.273843øè0.273843øúûêëè0.273843øè0.273843øúû0.005625=.(s2+0.2096s+0.075)(s2+0.5059958678s+0.075)275
ApplyingbilineartransformationtoHa(s)usingtheM-filebilinearwefinallyarriveat-140.0027696(1+z)G(z)=.(1-1.440137z-1+0.67367274z-2)(s2-1.1701485365z-1+0.3599z-2)Plotsofthegainandphaseresponsesofthistransferfunctionareshownbelow.00-10-2-20-30-4-40-6-50-60-800.20.40.60.8100.20.40.60.81w/pw/pM7.3ThemodifiedProgramP7_3isgivenbelow:formatshorteFp=input("PassbandedgefrequencyinHz=");Fs=input("StopbandedgefrequencyinHz=");FT=input("SamplingfrequencyinHz=");Rp=input("PassbandrippleindB=");Rs=input("StopbandminimumattenuationindB=");Wp=2*Fp/FT;Ws=2*Fs/FT;[N,Wn]=buttord(Wp,Ws,Rp,Rs)[b,a]=butter(N,Wn);disp("Numeratorpolynomial");disp(b)disp("Denominatorpolynomial");disp(a)[h,w]=freqz(b,a,512);plot(w/pi,20*log10(abs(h)));grid;axis([01-605]);xlabel("omega/pi");ylabel("Phase,radians")Pauseplot(w/pi,unwrap(angle(h)));grid;axis([01-81]);xlabel("omega/pi");ylabel("Phase,radians")ThenumeratorandthedenominatorcoefficientsofthelowpasstransferfunctionobtainedaregivenbyNumeratorpolynomial2.7690e-031.1076e-021.6614e-021.1076e-022.7690e-03Denominatorpolynomial1.0000e+00-2.6103e+002.7188e+00-1.3066e+002.4246e-01Plotsofthegainandphaseresponsesbyrunningthisprogramareshownbelow.276
00-10-2-20-30-4-40-6-50-8-6000.20.40.60.8100.20.40.60.81w/pw/pM7.4Givenspecifications:w=0.1p=0.31416w=0.5p=1.5708.psj0.1pj0.5p20logG(e)³–0.5,and20logG(e)£–45.1010ImpulseInvarianceMethod:LetT=1.Assumenoaliasing.ThenthespecificationsofHa(s)issameasthatofG(z),i.e.W=0.1p,W=0.5p,20logH(j0.1p)³–0.5,and20logH(j0.5p)£–45.Now,ps10a10aæ1ö2æ1ö20log10çç2÷÷=-0.5whichyieldse=0.12202.Similarly,20log10çè÷ø=-45è1+eøA2whichyieldsA=31622.7766.FromEq.(5.30)theinversediscriminationratioisgivenby21A–131621.7766===509.073363.FromEq.(5.29)theinversetransitionratioiske20.12201845110.5pgivenby==5.Hence,fromEq.(5.33),theorderofthelowpassButterworthfilterisk0.1plog(1/k)log(509.073363)10110givenbyN===3.8725.WechooseN=4astheorder.Nextlog(1/k)log(5)1010wedeterminethe3-dBcutofffrequencyWbymeetingexactlythestopbandedgec82specifications.FromEq.(5.32b),wehave(Ws/Wc)=A-1=31621.7766orWsW==0.273843W=0.273843´0.5p=0.43015.c(A2-1)1/8sUsingthestatement[B,A]=butter(4,0.43105,"s")wedeterminetheanalogButterworthtransferfunctionH(s)of4-thorderwitha3-dBcutofffrequencyataW=0.43105.H(s)isthentransformedintoadigitaltransferfunctionusingthestatementca[num,den]=impinvar(B,A,1);whichyields-1-2-40.0043063z+0.012898z+0.0024532zG(z)=.Aplotofitsgainresponseisshown1-2.8879z-1+3.2407z-2-1.6573z-3+0.3242z-4below.Itcanbeseenthatthefiltermeetsthegivenspecifications277
PassbandDetails0.50-100-20-30-0.5-40-50-1-6000.050.100.20.40.60.81w/pw/pM7.5Givenspecifications:w=0.1p=0.31416w=0.5p=1.5708.psj0.1pj0.5p20logG(e)³–0.5,and20logG(e)£–45.1010ImpulseInvarianceMethod:LetT=1.Assumenoaliasing.ThenthespecificationsofHa(s)issameasthatofG(z),i.e.W=0.1p,W=0.5p,20logH(j0.1p)³–0.5,and20logH(j0.5p)£–45.Now,ps10a10aæ1ö2æ1ö20log10çç2÷÷=-0.5whichyieldse=0.12202.Similarly,20log10çè÷ø=-45è1+eøA2whichyieldsA=31622.7766.FromEq.(5.30)theinversediscriminationratioisgivenby21A–131621.7766===509.073363.FromEq.(5.29)theinversetransitionratioiske20.12201845110.5pgivenby==5.Hence,fromEq.(5.41),theorderofthelowpassType1Chebyshevk0.1pcosh-1(1/k)cosh-1(509.073363)1filterisgivenbyN===3.0211.HencewechooseN=4.cosh-1(1/k)cosh-1(5)TheMATLABcodefragmentsusedare:[z,p,k]=cheb1ap(4,0.5);[B,A]=zp2tf(z,p,k);[BT,AT]=lp2lp(B,A,0.1*pi);[num,den]=impinvar(BT,AT,1);278
ImpulseInvarianceMethodImpulseInvarianceMethod00-10-2-20-4-30-6-40-50-800.10.20.30.400.51w/pw/pBilinearTransformationMethod:LetT=2.FromEq.(7.26),thebandedgesoftheanalogprototypeareW=tan(w/2)=0.15838andW=tan(w/2)=1FromEq.(5.29)theppss1Wsinversetransitionratio==6.31375.kWpæ1ö2æ1ö20log10çç2÷÷=-0.5whichyieldse=0.1220184.Similarly,20log10çè÷ø=-45whichè1+eøA2yieldsA=31622.7766.FromEq.(5.5.30theinversediscriminationratioisgivenby21A–131621.7766===509.073363.Hence,fromEq.(5.41),theorderofthelowpasske20.122018451-1-1cosh(1/k1)cosh(509.073363)Type1ChebyshevfilterisgivenbyN===2.7379.cosh-1(1/k)cosh-1(6.31375)HencewechooseN=3.TheMATLABcodefragmentsusedare[z,p,k]=cheb1ap(3,0.5);[B,A]=zp2tf(z,p,k);[BT,AT]=lp2lp(B,A,0.15838);[num,den]=bilinear(BT,AT,0.5);BilinearTransformationMethodBilinearTransformationMethod00-1-10-20-2-30-3-40-4-50-500.10.20.30.400.51w/pw/pNote:Bothdesignsmeetthespecifications.However,thebilineartransformationmethodmeetswithafilteroflowerorder.279
M7.6TheonlychangetoProgram7_2isgivenbythestatement[b,a]=cheby1(N,Rp,Wn);00-1-10-2-20-3-30-40-4-50-500.10.20.30.40.500.20.40.60.81w/pw/pM7.7ThecodefragmentsusedareWp=input("Passbandedgefrequency=");Ws=input("Stopbandedgefrequency=");Rp=input("PassbandrippleindB=");Rs=input("StopbandminimumattenuationindB=");[N,Wn]=cheb1ord(0.1*pi,0.5*pi,0.5,45,"s");[B,A]=cheby1(N,0.5,Wn,"s");[num,den]=impinvar(B,A,1);00-10-2-20-4-30-6-40-50-800.10.20.30.400.51w/pw/pM7.8ImpulseInvarianceMethod:FromExerciseM7.5wehavetheinverseselectivityparameter1W0.5p1A2–1s===5,andtheinversediscriminationratiogivenby==509.073363.kW0.1pke2p12log10(4/k1)21-k"FromEq.(5.51)wehaveN@wherek"=1-k,r=,andlog(1/r)o2(1+k")105913r=r+2(r)+15(r)+150(r),whichyieldsN=2.5519.WechooseN=3.ooooThecodefragmentsusedare:[z,p,k]=ellipap(3,0.5,45);[B,A]=zp2tf(z,p,k);[BT,AT]=lp2lp(B,A,0.1*pi);[num,den]=impinvar(BT,AT,1);280
ImpulseInvarianceMethodImpulseInvarianceMethod00-2-10-20-4-30-6-40-50-800.10.20.30.400.51w/pw/pBilinearTransformationMethod:FromExerciseM7.5,wehaveW=tan(w/2)=0.15838pp1WsandW=tan(w/2)=1,theinversetransitionratio==6.31375,andtheinversesskWp21A–131621.7766discriminationparameter===509.073363.Usingtheformulaofke20.122018451Eq.(5.51)wegetN=2.3641.WechooseN=3.Thecodefragmentsusedare:[N,Wn]=ellipord(0.1*pi,0.5*pi,0.5,45,"s");[z,p,k]=ellipap(3,0.5,45);[B,A]=zp2tf(z,p,k);[BT,AT]=lp2lp(B,A,0.15838);[num,den]=bilinear(BT,AT,0.5);BilinearTransformationMethodBilinearTransformationMethod00-1-10-2-20-3-30-40-4-50-500.10.20.30.400.51w/pw/pNotethatthefilterdesignedusingtheimpulseinvariancemethoddoesnotmeetthespecificationsduetoaliasing.However,iftheorderNisincreasedto4from3,itwillmeetthespecifications.M7.9NomodificationstoProgramP7_1arenecessary.UsingthisprogramwegetthesameresultsasindicatedinthesecondpartofthesolutiontoProblemM7.8M7.10Digitalhighpassfilterspecifications:F=325kHz,F=225kHz,F=1MHz,a=psTp0.5dB,anda=50dB.s281
2pFp2pFs(a)UsingEqs.(7.7)and(7.8)wehavew==2.042andw==1.4137.NextpFsFTTæwpöæwöusingEq.(7.26),wegetWˆ=tanç÷=1.6319andWˆ=tançs÷=0.85408.pçè2÷øsè2øAnaloghighpassfilterspecifications:Wˆ=1.6319radians,Wˆ=0.85408.a=0.5dB,andapsps=50dB.(b)FortheprototypeanaloglowpassfilterwechooseW=1.FromEq.(5.60)wethengetpWˆpW==1.9106.sWˆsAnaloglowpassfilterspecifications:W=1radians,W=1.9106radians,a=0.5dB,andpspa=50dB.sCodefragmentsusedare:[N,Wn]=ellipord(1,1.9106,0.5,50,"s");[B,A]=ellip(N,0.5,50,Wn,"s");[BT,AT]=lp2hp(B,A,1.6319);[num,den]=bilinear(BT,AT,0.5);PassbandDetails0.200-10-20-0.2-30-0.4-40-0.6-50-60-0.800.20.40.60.810.60.70.80.91w/pw/p(c)Analoglowpasstransferfunctioncoefficients:Numerator00.0202938.8818e-160.155801.1102e-150.25555Denominator11.15942.01591.41000.888800.25555Analoghighpasstransferfunctioncoefficients:Numerator17.2050e-151.623601.5710e-140.563177.2908e-15282
Denominator15.675714.69434.28232.17545.289Digitalhighpasstransferfunctioncoefficients:Numerator0.023939-0.0370660.059191-0.0591910.037066-0.023939Denominator12.40793.31022.63461.25350.28079M7.11Digitalbandpassfilterspecifications:F=560Hz,F=780Hz,F=375Hz,p1p2s1F=1000Hz,F=2500Hz,a=1.2dB,anda=25dB.s2Tps2pFp1(a)UsingEqs.(7.7)and(7.8)wehavew==0.448p=1.4074,p1FT2pFp22pFs1w==0.624p=1.9604,w==0.3p=0.94248,andp2Fs1FTT2pFs2ˆæwp1öw==0.8p=2.5133.NextusingEq.(7.26),wegetW=tanç÷=0.84866,s2Fp1çè2÷øTˆæwp2öˆæws1öˆæws2öW=tanç÷=1.4915,W=tanç÷=0.50953,,andW=tanç÷=3.0777.Thep2çè2÷øs1è2øs2è2øpassbandwidthisB=Wˆ-Wˆ=0.64287.NowWˆ2=WˆWˆ=1.2658andwp2p1op2p1WˆWˆ=1.5682¹WˆWˆ.Henceweadjustthelowerstopbandedgetos2s1p2p1WˆWˆˆp2p1ˆW==0.41128.NoteW=1.1251.s1Wˆos2Analogbandpassfilterspecifications:Wˆ=0.84866radians,Wˆ=1.4915radians,p1p2Wˆ=0.41128radians,Wˆ=3.0777radians,a=1.2dB,anda=25dB.s1s2ps(b)FortheprototypeanaloglowpassfilterwechooseW=1.FromEq.(5.62)wethengetpWˆ2-Wˆ21.2658-0.16915os1W===4.1477.sWˆ×B0.41477´0.64287s1wAnaloglowpassfilterspecifications:W=1radians,W=4.1477radians,a=1.2.dB,pspanda=25dB.sCodefragmentsusedare:[N,Wn]=cheb2ord(1,4.1477,1.2,25,"s");[B,A]=cheby2(N,25,Wn,"s");[BT,AT]=lp2bp(B,A,1.1251,0.64287);[num,den]=bilinear(BT,AT,0.5);283
PassbandDetails00-10-0.5-20-30-1-40-1.5-50-60-200.20.40.60.810.40.450.50.550.60.65w/pw/p(c)Analoglowpasstransferfunctioncoefficients:Numerator0.056234-2.2204e-161.8260Denominator11.85651.8260Analogbandpasstransferfunctioncoefficients:Numerator0.056234-2.2204e-160.89703-8.8818e-160.090108Denominator11.19353.28641.51081.6024Digitalbandpasstransferfunctioncoefficients:Numerator0.121420.015768-0.106600.0157680.12142Denominator10.354251.05220.206550.37058M7.12Digitalbandstopfilterspecifications:F=500Hz,F=2125Hz,F=1050Hz,p1p2s1F=1400Hz,F=5000Hz,a=2dB,anda=40dB.s2Tps2pFp1(a)UsingEqs.(7.7)and(7.8)wehavew==0.2p=0.62832,p1FT2pFp22pFs1w==0.85p=2.6704,w==0.42p=1.3195,andp2Fs1FTT284
2pFs2ˆæwp1öw==0.56p=1.7593.NextusingEq.(7.26),wegetW=tanç÷=0.32492,s2Fp1çè2÷øTˆæwp2öˆæws1öˆæws2öW=tanç÷=4.1653,W=tanç÷=0.77568,,andW=tanç÷=1.2088.Thep2çè2÷øs1è2øs2è2østopbandwidthisB=Wˆ-Wˆ=0.43311.NowWˆ2=WˆWˆ=0.93764andws2s1os2s1WˆWˆ=1.3534¹WˆWˆ.Henceweadjustthelowerpassbandedgetop2p1s2s1WˆWˆWˆ=s2s1=0.22511.NoteWˆ=0.96832.p1Wˆop2Analogbandstopfilterspecifications:Wˆ=0.22511radians,Wˆ=4.1653radians,p1p2Wˆ=0.41128radians,Wˆ=1.2088radians,a=2dB,anda=40dB.s1s2ps(b)FortheprototypeanaloglowpassfilterwechooseW=1.FromEq.(5.65)wethengetsWˆ×Bp1w0.22511´0.43311W===0.16913.pWˆ2-Wˆ20.93764-0.10557op1Analoglowpassfilterspecifications:W=1radians,W=0.16913radians,a=2dB,andaspps=40dB.Codefragmentsusedare:[N,Wn]=buttord(0.16913,1,2,40,"s");[B,A]=butter(N,Wn,"s");[BT,AT]=lp2bs(B,A,0.96832,0.43311);[num,den]=bilinear(BT,AT,0.5);50-5-10-15-20-25-3000.20.40.60.81w/p(c)Analoglowpasstransferfunctioncoefficients:Numerator0000.010001Denominator285
10.430890.0928350.010001Analogbandstoptransferfunctioncoefficients:NumeratorColumns1through61-1.5044e-162.8129-2.8211e-162.6375-1.3226e-16Column70.82435DenominatorColumns1through614.020610.89515.66410.2163.5348Column70.82435Digitalbandstoptransferfunctioncoefficients:NumeratorColumns1through60.15762-0.0304340.47481-0.0609100.47481-0.030434Column70.15762DenominatorColumns1through610-0.094374-0.0641472-0.0172320.33514-0.010173Column7-0.0061388M7.13TheimpulseresponsecoefficientsofthetruncatedFIRhighpassfilterwithcutofffrequencyat0.4pcanbegeneratedusingthefollowingMATLABstatements:n=-M:M;num=-0.4*sinc(0.4*n);num(M+1)=0.6;ThemagnituderesponsesofthetruncatedFIRhighpassfilterfortwovaluesofMareshownbelow:286
M7.14TheimpulseresponsecoefficientsofthetruncatedFIRbandpassfilterwithcutofffrequenciesat0.7pand0.3pcanbegeneratedusingthefollowingMATLABstatements:n=-M:M;num=0.7*sinc(0.7*n)-0.3*sinc(0.3*n);ThemagnituderesponsesofthetruncatedFIRbandpassfilterfortwovaluesofMareshownbelow:M7.15TheimpulseresponsecoefficientsofthetruncatedHilberttransformercanbegeneratedusingthefollowingMATLABstatements:n=1:M;c=2*sin(pi*n/2).*sin(pi*n/2);b=c./(pi*n);num=[-fliplr(b)0b];ThemagnituderesponsesofthetruncatedHilberttransformerfortwovaluesofMareshownbelow:287
M7.16FornotchfilterdesignusingtheHammingwindow,thefollowingMATLABprogramcanbeused:fc=input("Normalizednotchangularfrequency=");N=input("Desirednotchfilterorder=");%mustbeanevenintegerM=N/2;n=-M:1:M;t=fc*n;lp=fc*sinc(t);b=2*[lp(1:M)(lp(M+1)-0.5)lp((M+2):N+1)];bw=b.*hamming(N+1)";[h2,w]=freqz(bw,1,512);plot(w/pi,abs(h2));axis([0101.2]);xlabel("omega/pi");ylabel("Amplitude");title(["omega="num2str(fc),",Filterorder=",num2str(N)])Foranotchfilteroflength41withanotchfrequencyat60-Hzandoperatingata400-Hzsamplingrate,thenormalizedangularnotchfrequencyisgivenbywo=0.3p.Themagnituderesponseofthedesignedfilterisshownbelow:w=0.3,Filterorder=401.210.80.60.40.2000.20.40.60.81w/p32M7.17ThedesiredfunctionD(x)=2.2x-3x+0.5isdefinedfor-3£x£3.5.Wewishto2approximateD(x)byaquadraticfunctionax+ax+abyminimizingthepeakvalueof210288
2theabsoluteerrorD(x)-a2x-a1x-a0.Sincetherearefourunknownsa0,a1,a2ande,weneedfourextremalpointsonxintherange-3£x£3.5,whichwearbitrarilychooseasx1=–3,x2=–2,x3=1andx4=3.5.Wethensolvethefourlinearequations2la+ax+ax-(-1)e=D(x),l=1,2,3,4,whichleadto01l2llé1-391ùéa0ùé-85.9ùê1-24-1úêêa1úúê-29.1úêú=êú.ê1111úêa2úê-0.3úë13.51.225-1ûêëeúûë-58.075ûItssolutionyieldsa=-1.6214,a=18.229,a=-1.5857,ande=-15.321.Figure(a)below01232showstheplotoftheerrorE1(x)=2.2x-1.4143x-18.229x+2.1214alongwiththevaluesoftheerroratthechosenextremalpoints.ThenextsetofextremalpointsarethosepointswhereE1(x)assumesitsmaximumabsolutevalues.Theseextremalpointsaregivenbyx1=–3,x2=–1.454,x3=1.89andx4=3.5.Thenewvaluesoftheunknownsareobtainedbysolvingé1-391ùéa0ùé-85.9ùê1-1.4542.1141-1úêêa1úúê-12.605úêú=êúê11.893.57211úêa2úê4.6365úë13.51.225-1ûêëeúûë5.8075ûwhosesolutionyieldsa=-7.0706,a=-17.021,,a=-1.3458,ande=-18.857.Figure(b)01232belowshowstheplotoftheerrorE2(x)=2.2x-1.6542x-17.021x+4.3685alongwiththevaluesoftheerroratthechosenextremalpoints.Thistimethealgorithmhasconvergedaseisalsothemaximumvalueoftheabsoluteerror.2020101000-10-10-20-20-202-202xx(a)(b)M7.18FromEqs.(7.7)and(7.8),thenormalizedbandedgesaregivenbyw=0.2pandpw=0.4p.Therefore,w=0.3pandDw=0.2p.sc(a)UsingEq.(7.77)andTable7.2,theestimatedorderoftheFIRfilterfordesigningusingtheHammingwindowisgivenbyN=2MwhereM=3.32p/Dw=16.6.WethereforechooseM=17orequivalently,N=34.TheMATLABprogramusedtogeneratethewindowedfiltercoefficientsaren=-17:17;num=0.4*sinc(0.4*n);wh=hamming(35);289
b=num.*wh";ImpulseResponseCoefficientsFilterDesignedUsingHammingWindow0.400.3-200.20.1-400-60-0.1010203000.20.40.60.81Timeindexnw/p(b)FordesigningtheFIRfilterusingtheHannwindow,weobtainfromEq.(7.77)andTable7.2,M=3.11p/Dw=15.55.WechooseM=16,andhenceN=32.ImpulseResponseCoefficientsFilterDesignedUsingHannWindow0.400.3-200.20.1-400-60-0.1010203000.20.40.60.81Timeindexnw/p(c)FordesigningtheFIRfilterusingtheBlackmanwindow,weobtainfromEq.(7.77)andTable7.2,M=5.56p/Dw=27.8.WechooseM=28,andhenceN=56.ImpulseResponseCoefficientsFilterDesignedUsingBlackmanWindow0.400.3-200.2-400.1-600-80-0.10102030405000.20.40.60.81Timeindexnw/pThefilterdesignedusingtheHannwindowmeetsthespecificationswithasmallerlength.M7.19FromEqs.(7.7)and(7.8),thenormalizedbandedgesaregivenbyw=0.2pandpw=0.4p.Therefore,w=0.3pandDw=0.2p.sc290
Substitutinga=40inEq.(7.85)weobtainb=3.3953.Next,substitutingthevalueofsa=40andDw=0.2pinEq.(7.86)weobtainN=22.289.Wechoosethenexthigherevensintegervalueof24asthefilterorderN,andhenceM=12.Thecodefragmentsusedforthedesignaregivenbelow:n=-N/2:N/2;num=0.4*sinc(0.4*n);wh=kaiser(N+1,3.3953);b=num.*wh";ImpulseResponseCoefficients0.400.3-10-200.2-300.1-400-50-0.1-6000.20.40.60.810510152025Timeindexnw/pw+wpsM7.20w=0.3p,w=0.5p,anda=40dB.Thus,w==0.4p,andpssc2Dw=w-w=0.2p.FromTable7.2weobservethatHannwindowisappropriateforthefilterspdesignasitmeetstheminimumstopbandattenuationrequirementwithafilteroflowestorder.3.11p3.11pFromEq.(7.77)andTable7.2,wegetM===15.55.WechooseM=16Dw0.2pimplyingafilteroforderN=32.Codefragmentsusedinthedesignaregivenbelow:n=-16:1:16;lp=0.4*sinc(0.4*n);wh=hanning(33);b=lp.*wh";ImpulseResponseCoefficientsLowpassFilterDesignedUsingHannWindow0.400.3-100.2-20-300.1-400-50-0.1-60010203000.20.40.60.81Timeindexnw/p291
M7.21w=0.3p,w=0.5p,anda=40dB.Thus,w=0.4p,andDw=0.2p.UsingEq.(7.82)pssc2.065´40-16.4weestimatethefilterorderN=2MwhichisgivenbyN==45.859.Hencewe2.285(0.2p)chooseN=46,i.e.M=23.Codefragmentsusedforthedesignare:n=-23:1:23;lp=0.4*sinc(0.4*n);wh=chebwin(47,40);b=lp.*wh";FilterDesignedUsingDolph-ChebyshevWindow0-10-20-30-40-50-6000.20.40.60.81w/pM7.22Codefragmentsusedforthedesignare:n=-16:1:16;b=fir1(32,0.4,hanning(33));ImpulseResponseCoefficientsFilterDesignedUsingfir1withHanningWindow0.400.3-100.2-20-300.1-400-50-0.1-60010203000.20.40.60.81Timeindexnw/pM7.23N=29;fork=1:N+1w=2*pi*(k-1)/30;if(w>=0.5*pi&w<=1.5*pi)H(k)=1;elseH(k)=0;endif(w<=pi)phase(k)=i*exp(-i*w*N/2);elsephase(k)=-i*exp(i*(2*pi-w)*N/2);endendH=H.*phase;292
f=ifft(H);[FF,w]=freqz(f,1,512);k=0:N;subplot(211)stem(k,real(f));xlabel("Timeindexn");ylabel("Amplitude")subplot(212)plot(w/pi,20*log10(abs(FF)));xlabel("omega/pi");ylabel("Gain,dB");axis([01-505]);gridon0.50-10-200-30-40-0.5-50010203000.20.40.60.81Timeindexnw/pM7.24%Length=41andbandpass,henceType3filterN=40;L=N+1;fork=1:Lw=2*pi*(k-1)/L;if(w>=0.3*pi&w<=0.5*pi)H(k)=i*exp(-i*w*N/2);elseif(w>=1.5*pi&w<=1.7*pi)H(k)=-i*exp(i*(2*pi-w)*N/2);elseH(k)=0;endendf=ifft(H);[FF,w]=freqz(f,1,512);k=0:N;subplot(211);stem(k,real(f));xlabel("Timeindexn");ylabel("h[n]");subplot(212);plot(w/pi,20*log10(abs(FF)));ylabel(Gain,dB");xlabel("omega/pi");axis([01-505]);grid;293
0.200.1-10-200-30-0.1-40-0.2-5001020304000.20.40.60.81Timeindexnw/pM7.25N=36;L=N+1;fork=0:36if(k<=5|k>=32)H(k+1)=exp(-i*2*pi*k/L*18);elseif(k==6|k==31)H(k+1)=0.5*exp(-i*2*pi*k/L*18);endendf=ifft(H);[FF,w]=freqz(f,1,512);k=0:N;subplot(211);stem(k,real(f));axis([036-0.10.4]);xlabel("Timeindexn");ylabel(h[n]);subplot(212);plot(w/pi,20*log10(abs(FF)));xlabel("omega/pi");ylabel("Gain,dB");axis([01-705]);grid;0.400.3-200.20.1-400-60-0.1010203000.20.40.60.81Timeindexnw/pM7.26N=36;L=N+1;fork=0:36if(k<=5|k>=32)H(k+1)=exp(-i*2*pi*k/L*18);elseif(k==6|k==31)H(k+1)=2/3*exp(-i*2*pi*k/L*18);elseif(k==7|k==30)H(k+1)=1/3*exp(-i*2*pi*k/L*18);endendf=ifft(H);[FF,w]=freqz(f,1,512);k=0:N;294
subplot(211);stem(k,real(f));xlabel("Timeindexn");ylabel("Amplitude");subplot(212);plot(w/pi,20*log10(abs(FF)));xlabel("omega/pi");ylabel(Gain,dB");axis([01-705]);grid;0.400.3-200.20.1-400-60-0.1010203000.20.40.60.81Timeindexnw/pM7.27w=0.3pandDw=0.2p.c(a)HammingWindow:FromExerciseM7.18(a)wehaveM=17.Codefragmentusedforthedesignare:b=fir1(2*M,0.3);ImpulseResponseCoefficientsFilterDesignedUsingHammingWindow0.300.2-200.1-400-60-0.101020304000.20.40.60.81Timeindexnw/p(b)HannWindow:FromExerciseM7.18(b)wehaveM=16.Codefragmentusedforthedesignare:b=fir1(2*M,0.3,hanning(2*M+1));295
ImpulseResponseCoefficientsFilterDesignedUsingHannWindow0.400.3-200.20.1-400-60-0.1010203000.20.40.60.81Timeindexnw/p(c)BlackmanWindow:FromExerciseM7.18(c)wehaveM=28.Codefragmentusedforthedesignare:b=fir1(2*M,0.3,blackman(2*M+1));FilterDesignedUsingBlackmanWindowImpulseResponseCoefficients0.400.3-200.2-400.1-600-80-0.10102030405000.20.40.60.81Timeindexnw/p(d)KaiserWindow:w=0.2p,w=0.4p,a=40dB.Thus,pss-a/2-2d=10s=10=0.01.Codefragmentsusedforthedesignare:s[N,Wn,beta,type]=kaiserord([0.20.4],[10],[0.010.01]);b=fir1(N,0.3,kaiser(N+1,beta));ImpulseResponseCoefficientsFilterDesignedUsingKaiserWindow0.400.3-200.20.1-400-60-0.10510152000.20.40.60.81Timeindexnw/p296
M7.28w=0.6p,w=0.45p,a=0.2dBanda=45.Thus,Dw=0.15pandpsps0.6p+0.45pw==0.525p.c2(a)HammingWindow:FromTable7.2andEq.(7.77)wehave3.32p3.32pM===22.133,WechooseM=23,i.e.filterorderN=2M=46.Dw0.15pCodefragmentusedis:b=fir1(2*M,0.525,"high");ImpulseResponseCoefficientsFilterDesignedUsingHammimgWindow0.600.4-200.20-40-0.2-60-0.401020304000.20.40.60.81Timeindexnw/p3.11p3.11p(b)HannWindow:FromTable7.2andEq.(7.77)wehaveM===20.733.Dw0.15pWechooseM=21,i.e.filterorderN=2M=42.Codefragmentusedis:b=fir1(2*M,0.525,"high",hanning(2*M+1));FilterDesignedUsingHannWindowImpulseResponseCoefficients0.600.4-200.20-40-0.2-60-0.401020304000.20.40.60.81Timeindexnw/p(c)BlackmanWindow:FromTable7.2andEq.(7.77)wehave5.56p5.56pM===37.067.WechooseM=38,i.e.filterorderN=2M=76.Dw0.15pCodefragmentusedis:297
b=fir1(2*M,0.525,"high",blackman(2*M+1));ImpulseResponseCoefficientsFilterDesignedUsingBlackmanWindow0.600.4-200.2-400-60-0.2-80-0.4020406000.20.40.60.81Timeindexnw/p(d)KaiserWindow:w=0.6p,w=0.45p,a=45dB.Thus,w=0.525pandpssc-a/20-2.25d=10s=10=0.0056234.Codefragmentsusedforthedesignare:sds=0.0056234;[N,Wn,beta,type]=kaiserord([0.450.6],[10],[dsds]);N=N+1;%Forhighpassfilter,ordermustbeevenb=fir1(N,0.525,"high",kaiser(N+1,beta));FilterDesignedUsingKaiserWindowImpulseResponseCoefficients0.600.4-200.2-400-60-0.2-80-0.4010203000.20.40.60.81Timeindexnw/pM7.29w=0.55p,w=0.7p,w=0.45p,w=0.8p,a=0.15anda=40.Thus,p1p2s1s2psDw=w-w=0.1pandDw=w-w=0.1p=Dw.1p1s12s2p21(a)HammingWindow:FromTable7.2andEq.(7.77)wehave3.32p3.32pM===33.2WechooseM=34,i.e.filterorderN=2M=68.Dw0.1p1Codefragmentusedis:b=fir1(2*M,[0.50.75]);298
ImpulseResponseCoefficientsFilterDesignedUsingHammingWindow0.300.2-200.10-40-0.1-60-0.2020406000.20.40.60.81Timeindexnw/p3.11p3.11p(b)HannWindow:FromTable7.2andEq.(7.77)wehaveM===31.1.Dw0.1pWechooseM=32,i.e.filterorderN=2M=64.Codefragmentusedis:b=fir1(2*M,[0.50.75],hanning(2*M+1));ImpulseResponseCoefficientsFilterDesignedUsingHannWindow0.300.2-200.10-40-0.1-60-0.2020406000.20.40.60.81Timeindexnw/p(c)BlackmanWindow:FromTable7.2andEq.(7.77)wehave5.56p5.56pM===55.6.WechooseM=56,i.e.filterorderN=2M=12.Dw0.1p1w+ww+wp1s10.55p+0.45pp2s20.7p+0.8pw===0.5pandw===0.75p.c122c222Codefragmentusedis:b=fir1(2*M,[0.550.7],blackman(2*M+1));299
FilterDesignedUsingBlackmanWindowImpulseResponseCoefficients0.20-200.1-400-60-0.1-8002040608010000.20.40.60.81Timeindexnw/p(d)KaiserWindow:w=0.55p,w=0.7p,w=0.45p,w=0.8p,a=0.15andp1p2s1s2p-a/20-0.075-a/20-2a=40.Thus,d=10p=10=0.98288andd=10s=10=0.01.spsCodefragmentsusedforthedesignare:[N,Wn,beta,type]=kaiserord([0.550.7],[10],[0.982880.01]);b=fir1(N,[0.50.75],kaiser(N+1,beta));FilterDesignedUsingKaiserWindowImpulseResponseCoefficients0.300.2-200.10-40-0.1-60-0.2010203000.20.40.60.81Timeindexnw/p2p´10M7.30FromEq.(7.7),thenormalizedcrossoverfrequencyw==0.25pThedelay-c80complementaryFIRlowpassandhighpassfiltercoefficientsoflength27arethengeneratedusingtheMATLABstatements:d1=fir1(26,0.7854);d2=-d1;d2(14)=1-d1(14);Thegainresponsesofthetwofiltersareasindicatedbelow:300
0H(z)H(z)-20HPLP-40-6000.20.40.60.81w/pM7.31ThefiltercoefficientsoftheFIRlowpassandthehighpass,andtheirdelay-complementaryFIRbandpassfilteraregeneratedusingtheMATLABprogram:c=hanning(31);d1=fir1(30,0.15873,c);d2=fir1(30,0.40816,"high",c);d3=-d1-d2;d3(16)=1-d1(16)-d2(16);ThegainresponsesofthethreeFIRfiltersareasgivenbelow:20H(z)H(z)H(z)LPBPHP0-20-40-6000.20.40.60.81w/pM7.32ThefiltercoefficientsaregeneratedusingtheMATLABprogram:fpts=[00.40.420.70.7211];mval=[0.50.50.30.31.01.0];b=fir2(80,fpts,mval);ThegainresponseofthemultibandFIRfilterisasgivenbelow:301
1.210.80.60.40.200.20.40.60.81w/pM7.33Thefilterwasalsodesignedbyusingremezordtoestimatethefilterorderandthenusingremeztodeterminethefiltercoefficients.TothisendtheMATLABprogramusedisgivenbelow:Rp=0.1;Rs=40;FT=20;f=[24];m=[10];dev=[(10^(Rp/20)-1)/(10^(Rp/20)+1)10^(-Rs/20)];[N,fo,mo,wo]=remezord(f,m,dev,FT);b=remez(N,fo,mo,wo);Thefilterobtainedusingtheaboveprogramisoflength20,butitsgainresponsedidnotmeetthespecifications.Thespecificationsweremetwhenthefilterorderwasincreasedto22.0-20-40-6000.20.40.60.81w/pM7.34Thefilterwasalsodesignedbyusingremezordtoestimatethefilterorderandthenusingremeztodeterminethefiltercoefficients.TothisendtheMATLABprogramusedisgivenbelow:Rp=0.2;Rs=45;f=[0.450.65];m=[10];dev=[(10^(Rp/20)-1)/(10^(Rp/20)+1)10^(-Rs/20)];[N,fo,mo,wo]=remezord(f,m,dev);b=remez(N,fo,mo,wo);Thefilterobtainedusingtheaboveprogramisoflength27,butitsgainresponsedidnotmeetthespecifications.Thespecificationsweremetwhenthefilterorderwasincreasedto30.302
0-20-40-6000.20.40.60.81w/pM7.35ThecodefragmentsusedtodesigntheFIRbandpassfilterare:Rp=0.15;Rs=40;f=[0.450.550.70.8];m=[010];dev=[10^(-Rs/20)(10^(Rp/20)-1)/(10^(Rp/20)+1)10^(-Rs/20)];[N,fo,mo,wo]=remezord(f,m,dev);b=remez(N,fo,mo,wo);Thefilterobtainedusingtheaboveprogramisoflength40,butitsgainresponsedidnotmeetthespecifications.Thespecificationsweremetwhenthefilterorderwasincreasedto41.0-20-40-6000.20.40.60.81w/pM7.36Thecodefragmentusedtodesignalength-32differentiatorisgivenbelow.b=remez(31,[01],[0pi],"differentiator");Themagnituderesponseofthedifferentiatordesignedisshownbelow:303
32.521.510.5000.20.40.60.81w/pM7.37Thecodefragmentsusedtodesignthe26-thorderHilberttransformerare:f=[0.010.0780.080.920.941];m=[001100];wt=[1601];b=remez(28,f,m,wt,"hilbert");Notethatthepassbandandthestopbandshavebeenweightedtoreducetherippleinthepassband.ThemagnituderesponseoftheHilberttransformerdesignedisshownbelow:10.80.60.40.2000.20.40.60.81w/pM7.38Thecodefragmentusedforthedesignisb=firls(31,[00.9],[00.9*pi],"differentiator");32.521.510.5000.20.40.60.81w/p304
ww2p-wsssM7.39Fromthegivenspecifications=0.2p.Wealsorequire£,fornooverlaptoMMMjMw2poccurinH(e)(seeFigureP7.3(b)).Hence0.2p£-0.2pwhichimpliesM£5.MWechooseM=4.HencespecificationsforH(z)arew=0.6p,w=0.8p,d=0.001andpspd=0.001.SubstitutingthesevaluesinEq.(7.15),wearriveattheestimatedvalueofthesFIRfilterlengthasN=33.SpecificationsforF(z)arew=0.15p,w=0.3p,d=0.001andd=0.001.Hence,frompspsEq.(7.15),theestimatedlengthofF(z)isN=43.331Thereforethetotalnumberofmultiplicationsrequiredperoutputsampleis(+43)´=2230.(Thedivisionby2isduetothefactthatH(z)andF(z)arelinearphasefilters.)Ontheotherhandforadirectsinglestageimplementation,wenotethatthespecificationsofG(z)are:wp=0.15p,ws=0.2p,dp=0.002,ds=0.001.Hence,thefilterlengthofG(z)fromEq.(7.15)is121.Thereforethetotalnumberofmultiplicationsrequiredperoutputsampleis121/2=61.20M0F(z)H(z)0G(z)-20-20-40-40-60-6000.20.40.60.8100.20.40.60.81w/pw/pM7.40Fromthespecifiedstopbandedgews=0.14p,wegettheestimatedorderNpftheRRS2pprefilterH(z)asN@=14.2857.WechooseN=15.NextusingthefollowingtwowsMATLABfunctionswedesigntheequalizerF(z)andtheoverallcascadedfilterH(z)F(z).%Plottingfunction%plotstheresultofusinganequalizeroflengthInpNfunction[N]=plotfunc(InpN);%CreatingfiltersWfilt=ones(1,InpN);Efilt=remezfunc(InpN,Wfilt);%Plotrunningsumfilterresponse%figure(1)Wfilt=Wfilt/sum(Wfilt);[hh,w]=freqz(Wfilt,1,512);plot(w/pi,20*log10(abs(hh)));axis([01-505]);grid;xlabel("omega/pi");ylabel("Gain,dB");title("PrefilterH(z)");305
%Plotequalizerfilterresponse%figure(2)pause[hw,w]=freqz(Efilt,1,512);plot(w/pi,20*log10(abs(hw)));axis([01-505]);grid;xlabel("omega/pi");ylabel("Gain,dB");title("EqualizerF(z)");%Plotcascadedfilterresponse%figure(3)pauseCfilt=conv(Wfilt,Efilt);[hc,w]=freqz(Cfilt,1,512);plot(w/pi,20*log10(abs(hc)));axis([01-805]);grid;title("CascadedfilterH(z)F(z)");ylabel("G(omega),dB");xlabel("omega/pi");%Plotfiltercoefficients%figure(4)pause%subplot(2,1,1);n1=0:length(Wfilt)-1;stem(n1,Wfilt);xlabel("Timeindexn");ylabel("Amplitude");title("Prefiltercoefficients");pause%subplot(2,1,2);n2=0:length(Efilt)-1;stem(n2,Efilt);xlabel("Timeindexn");ylabel("Amplitude");title("Equalizercoefficients");%Remezfunctionusing1/P(z)asdesiredamplitude%andP(z)asweightingfunction[N]=remezfunc(Nin,Wfilt);%Nin:numberoftuplesintheremezequalizerfilter%Wfilt:theprefiltera=[0:0.001:0.999];%Theaccuracyofthecomputationw=a.*pi;wp=0.042*pi;%Thepassbandedgews=0.14*pi;%Thestopbandedgei=1;n=1;fort=1:(length(a)/2)ifw(2*t)wssto(n)=w(2*t-1);sto(n+1)=w(2*t);n=n+2;end;end;w=cat(2,pas,sto);bi=length(w)/2;%bigivesthenumberofsubintervalsfort1=1:bi306
bw(t1)=(w(2*t1)+w(2*t1-1))/2;W(t1)=Weight(bw(t1),Wfilt,ws);end;W=W/max(W);fort2=1:length(w)G(t2)=Hdr(w(t2),Wfilt,wp);end;G=G/max(G);N=remez(Nin,w/pi,G,W);%Weightingfunctionfunction[Wout]=Weight(w,Wfilt,ws);K=22.8;L=length(Wfilt);Wtemp=0;Wsum=0;fork=1:LWtemp=Wfilt(k)*exp((k-1)*i*w);Wsum=Wsum+Wtemp;end;Wout=abs(Wsum);ifw>wsWout=K*max(Wout);end;%Desiredfunctionfunction[Wout]=Hdr(w,Wfilt,ws);ifw<=wsL=length(Wfilt);Wtemp=0;Wsum=0;fork=1:LWtemp=Wfilt(k)*exp(i*(k-1)*w);Wsum=Wsum+Wtemp;end;Wsum=abs(Wsum);Wout=1/Wsum;elseWout=0;end;PrefilterH(z)EqualizerF(z)00-10-10-20-20-30-30-40-40-50-5000.20.40.60.8100.20.40.60.81w/pw/p307
CascadedfilterH(z)F(z)0-20-40-60-8000.20.40.60.81w/pPrefiltercoefficientsEqualizercoefficients0.080.10.080.060.060.040.040.020.0200051015051015TimeindexnTimeindexn308
Chapter8(2e)8.1a0x[n]y[n]bw[n]11z–1a1–1bw[n]2w[n]3z–15a2–1bw[n]3w[n]4z–12a3Analysisyieldsw[n]=abw[n-1]+x[n]-w[n],11113w[n]=bw[n-1],234w[n]=bw[n-1],325w[n]=aw[n]+w[n],4323w[n]=aw[n]-w[n]+bw[n-1],523211y[n]=ax[n]+bw[n-1].011Inmatrixformtheabovesetofequationsisgivenby:éw1[n]ùé00-1000ùéw1[n]ùéa1b100000ùéw1[n-1]ùéx[n]ùêw[n]úê000000úêw[n]úêê000b00úúêw[n-1]úê0úê2úê000000úê2ú3ê2úêúêw[n]úêw[n]úê0000b0úêw[n-1]ú0ê3ú=ê0a1000úê3ú+ê2úê3ú+ê0úêw4[n]úêê3úúêw4[n]úê000000úêw4[n-1]úê0úêw5[n]úê0-1a2000úêw5[n]úêb100000úêw5[n-1]úêúêy[n]úë000000ûêy[n]úêb00000úêy[n-1]úêëa0x[n]úûëûëûë1ûëûHeretheFmatrixisgivenbyé00-1000ùê000000úê000000úF=êê0a1000úú3ê0-1a000úê2úë000000ûSincetheFmatrixcontainsnonzeroentriesabovethemaindiagonal,theabovesetofequationsarenotcomputable.8.2AcomputablesetofequationsofthestructureofFigureP8.1isgivenbyw[n]=bw[n-1],234w[n]=bw[n-1],325309
w[n]=abw[n-1]-w[n]+x[n],11113w[n]=aw[n]+w[n],4323w[n]=aw[n]-w[n]+bw[n-1],523211y[n]=ax[n]+bw[n-1].011Inmatrixformtheabovesetofequationsisgivenby:éw2[n]ùé000000ùéw2[n]ùé000b300ùéw1[n-1]ùé0ùêw[n]úê000000úêw[n]úêê0000b0úúêw[n-1]úê0úê3úê0-10000úê3ú2ê2úêúêw[n]úêw[n]úê00ab000úêw[n-1]úx[n]ê1ú=êa10000úê1ú+ê11úê3ú+ê0úêw4[n]úêê3úúêw4[n]úê000000úêw4[n-1]úê0úêw5[n]úê-1a20000úêw5[n]úê00b1000úêw5[n-1]úêúêy[n]úë000000ûêy[n]úê00b000úêy[n-1]úêëa0x[n]úûëûëûë1ûëûHeretheFmatrixisgivenbyé000000ùê000000úê0-10000úF=êêa10000úú3ê-1a0000úê2úë000000ûSincetheFmatrixdoesnotcontainnonzeroentriesabovethemaindiagonal,thenewsetofequationsarecomputable.8.3a0x[n]y[n]wa11[n]w2[n]b1–1–1za2w3[n]b2w4[n]–1z–1w5[n]a3w[n]b36–1zAnalysisyieldsw[n]=x[n]-aw[n]+w[n],1232w[n]=abw[n-1],2111w[n]=aw[n]-aw[n]+w[n],311354w[n]=abw[n-1],4223w[n]=aw[n]+w[n],5236w[n]=abw[n-1],6335310
y[n]=ax[n]+aw[n].011Inmatrixforméw1[n]ùé01-a20000ùéw1[n]ùêw[n]úê0000000úêw[n]úê2úêúê2úêw3[n]úêa1001-a300úêw3[n]úêw[n]ú=ê0000000úêw[n]úê4úê00a0001úê4úêw5[n]úê2úêw5[n]úêw[n]úê0000000úêw[n]úê6úêa000000úê6úëy[n]ûë1ûëy[n]ûé0000000ùéw1[n-1]ùéx[n]ùêab000000úêw2[n-1]úê0úê11úêúêúê0000000úêw3[n-1]úê0ú+ê00a2b20000úêw[n-1]ú+ê0úê0000000úê4úê0úêúêw5[n-1]úê0úê0000a3b300úêw6[n-1]úêúêë0000000úûêy[n-1]úëa0x[n]ûëûé01-a0000ù2ê0000000úêúêa1001-a300úHeretheFmatrixisgivenbyF=ê0000000úê00a0001úê2úê0000000úêëa1000000úûSincetheFmatrixcontainsnonzeroentriesabovethemaindiagonal,theabovesetofequationsarenotcomputable.8.4Thesignal-flowgraphrepresentationofthestructureofFigureP8.1isshownbelow:a01-1b-1-1-11w1[n]1z1b2zw3[n]1b3zv[n]w2[n]x[n]1w5[n]w4[n]y[n]a1a2a3-1Thereducedsignal-flowgraphobtainedbyremovingthebranchesgoingoutoftheinputnodeandthedelaybranchesisasindicatedbelow:311
1-1v1w3[n]1w1[n]1[n]ww5[n]w4[n]2[n]y[n]a1a2a3-1Fromtheabovesignal-flowgraphwearriveatitsprecedencegraphshownbelow:y[n]1a1v[n]w[n]111-11w3[n]w4[n]a3a2ww[n]2[n]5-1NN12Intheaboveprecedencegraph,thesetN1containsnodeswithonlyoutgoingbranchesandthefinalsetN2containsnodeswithonlyincomingbranches.Asaresult,thestructureofFigureP8.1hasnodelay-freeloops.AvalidcomputationalalgorithmbycomputingthenodevariablesinsetN1firstinanyorderfollowedbycomputingthenodevariablesinsetN2inanyorder.Forexample,onevalidcomputationalalgorithmisgivenbyv[n]=bw[n-1],111w[n]=bw[n-1],325w[n]=bw[n-1],234w[n]=av[n]-w[n]+x[n],1113w[n]=aw[n]+w[n],4323w[n]=aw[n]-w[n]+bv[n],523211y[n]=ax[n]+v[n].018.5Thereducedsignal-flowgraphobtainedbyremovingthebranchesgoingoutoftheinputnodeandthedelaybranchesfromthesignal-flowgraphrepresentationofthestructureofFigureP8.2isasindicatedbelow:312
a1a2w1[n]w3[n]w5[n]w6[n]y[n]w2[n]w4[n]1–a2–a3a1Theonlynodewithoutgoingbranchisw6[n]andhenceitistheonlymemberofthesetN1.SinceitisnotpossibletofindasetofnodesN2withincomingbranchesfromN1andallotherbranchesbeingoutgoing,thestructureofFigureP8.2hasdelay-freeloopsandisthereforenotrealizable.8.6(a)w3[n]w2[n]w1[n]x[n]-k3-k2-k1k3k2k1y[n]z-1z-1z-1s3[n]s2[n]AnalysisofFigureP8.3yieldsw[n]=w[n]-kw[n-1],1211w[n]=w[n]-ks[n-1],2322w[n]=x[n]-ks[n-1],333s[n]=kw[n]+w[n-1],2111s[n]=kw[n]+s[n-1],3222y[n]=kw[n]+s[n-1].333Inmatrixformwehaveéw1[n]ùé0ùé010000ùéw1[n]ùé-k100000ùéw1[n-1]ùêw[n]úê0úê001000úêw[n]úê000-k00úêw[n-1]úê2úêúê000000úê2úê2úê2úêw3[n]ú=êx[n]ú+êúêw3[n]ú+ê0000-k30úêw3[n-1]úês[n]úê0úêk100000úês[n]úê100000úês[n-1]úê2úê0úê0k0000úê2úêúê2úês3[n]úê0úê2úês3[n]úê000100úês3[n-1]úêëy[n]úûëûë10440444k23404404430ûêëy[n]úûë000010ûêëy[n-1]úû1444442444443FGAsthediagonalelementsoftheF-matrixareallzeros,therearenodelay-freeloops.However,thesetofequationsasorderedisnotcomputableastherenon-zeroelementsabovethediagonalofF.(b)Thereducedsignalflow-graphrepresentationofthestructureofFigureP8.3isshownbelow:313
k1w2[n]1s3[n]y[n]w3[n]1w1[n]s2[n]k2k3Fromtheaboveflow-graphweobservethatthesetcomposedofnodeswithoutgoingbranchesisN={w[n]}.Thesetcomposedofnodeswithoutgoingbranchesandincomingbranches13fromNisN={w[n]}.Thesetcomposedofnodeswithoutgoingbranchesandincoming122branchesfromNandNisN={w[n]}.Finally,setcomposedofnodeswithonly1231incomingbranchesfromN,NandNisN={s[n],s[n],y[n]}.Therefore,one123423possibleorderedsetofequationsthatiscomputableisgivenbyéw3[n]ùé0ùé000000ùéw3[n]ùé0000-k30ùéw3[n-1]ùêw[n]úê0úê100000úêw[n]úê000-k00úêw[n-1]úê2úêúê010000úê2úê2úê2úêw1[n]ú=êx[n]ú+êúêw1[n]ú+ê00-k3000úêw1[n-1]úês[n]úê0úê00k1000úês[n]úê001000úês[n-1]úê2úê0úê0k0000úê2úêúê2úês3[n]úê0úê2úês3[n]úê000100úês3[n-1]úêëy[n]úûëûë1k434044420404404430ûêëy[n]úûë000010ûêëy[n-1]úû144444424444443FGNotethatallelementsonthediagonalandabovedoagonalarezeros.p+pz-1+pz-20128.7H(z)=and{h[n]}={2.1,1.1,-3.2,-4.1,-28.3,L},n=0,1,K1-3z-1-5z-2épùé2.100ùé1ùé2.1ùê0úFromEq.(8.15)wehaveêp1ú=ê1.12.10úê-3ú=ê-5.2ú.Hence,êúêúêúêëp2úûë-3.21.12.1ûë-5ûë-17û-1-2P(z)=2.1-5.2z-17z.p+pz-1+pz-20128.8H(z)=and{h[n]}={3,-2,-6,12,-15},0£n£4.Theequation1+dz-1+dz-212ép0ùé300ùêpúê-230úé1ùê1úê-6-23úêúcorrespondingtoEq.(8.12)isêp2ú=êúêd1ú.Therefore,fromEQ.(8.17)weê0úê12-6-2úêëd2úûêë0úûë-1512-6û314
éd1ùé-6-2ù-1é12ùé1.7ùhaveêú=-=,andfromEq.(8.18)wehaveëd2ûëê12-6ûúëê-15ûúëê0.9ûúép0ùé300ùé1ùé3ù-1-2êpú=ê-230úê1.7ú=ê3.1ú.Hence,H(z)=3+3.1z-6.7z.ê1úêúêúêú1+1.7z-1+0.9z-2êëp2úûë-6-23ûë0.9ûë-6.7ûp+pz-1+pz-2+pz-301238.9H(z)=and1+dz-1+dz-2+dz-3123{h[n]}={2,-5,6,-2,-9,18,-7-31,65,-30,L},n³0.Theequationépù0é2000ùêpúê-5200úê1úé1ùêpúê6-520úêdúê2úêúê1úcorrespondingtoEq.(8.17)isgivenbyêp3ú=ê-26-52úêdú.Therefore,fromEq.0ê-9-26-5ú2êêúúê18-9-26úêëd3úû0ê-718-9-2úêë0úûëûédùé-26-5ù-1é-9ùé2ùê1ú(8.17)wehaveêd2ú=-ê-9-26úê18ú=ê3úandfromEq.(8.18)wehaveêúêúêúêëd3úûë18-9-2ûë-7ûë1ûép0ùé2000ùé1ùé2ùêêp1úúê-5200úê2úê-1ú2-z-1+2z-2-3z-3êpú=ê6-520úê3ú=ê2ú.Hence,H(z)=1+2z-1+3z-2+z-3.2êúêúêúêpúë-26-52ûë1ûë-3ûë3ûp+pz-1+pz-2+pz-301238.10G(z)=withg[n]={3,7,13,-3},0£n£3.Therefore,from2-6z-1+8z-2+10z-3ép0ùé3000ùé2ùé6ùêêp1úúê7300úê-6úê-4ú6-4z-1+8z-2+2z-3Eq.(8.18)wehaveêpú=ê13730úê8ú=ê8ú.Hence,G(z)=2-6z-1+8z-2+10z-3.2êúêúêúêpúë-31373ûë10ûë2ûë3ûp+pz-1+pz-2+pz-3+pz-4012348.11H(z)=with1+2z-1+2z-2+3z-3+3z-4{h[n]}={2,0,-5,-10,-10,55,-45,40,-125,140,-15}0£n£10TheequationcorrespondingtoEq.(8.18)isgivenbyépùê0úé20000ùé1ùé2ùêp1úê02000úê2úê4úêp2ú=ê-50200úê2ú=ê-1ú.êpúêê-10-5020úúêê3úúêê-14úúê3ú-10-10-5023-34êëp4úûëûëûëû-1-2-3-42+4z-z-14z-34zTherefore,H(z)=.1+2z-1+2z-2+3z-3+3z-4315
N-1nk8.12Thek-thsampleofanN-pointDFTisgivenbyX[k]=åx[n]WN.Thusthecomputationofk=0X[k]requiresNcomplexmultiplicationsandN–1complexadditions.Noweachcomplexmultiplication,inturn,requires4realmultiplications,and2realadditions.Likewise,eachcomplexadditionrequires2realadditions.Asaresult,theNcomplexmultiplicationsneededtocomputeX[k]requireatotalof4Nrealmultiplicationsandatotalof2Nrealadditions.Similarly,theN–1complexadditionsneededinthecomputationofX[k]requireatotalof2N–2realadditions.Hence,eachsampleoftheN-pointDFTinvolves4Nrealmultiplicationsand4N–2realadditions.ThecomputationofallNDFTsamplesthusrequires4N2realmultiplicationsand(4N–2)Nrealadditions.8.13Letthetwocomplexnumbersbea=a+jbandb=c+jd.Then,ab=(a+jb)(c+jd)=(ac–bd)+j(ad+bc)whichrequires4realmultiplicationsand2realadditions.Considertheproducts(a+b)(c+d),acandbdwhichrequire3realmultiplicationsand2realadditions.Theimaginarypartofabcanbeformedfrom(a+b)(c+d)-ac–bd=ad+bcwhichnowrequires2realadditions.Likewise,therealpartofabcanbeformedbyformingac–bdrequiringanadditionalrealaddition.Hence,thecomplexmultplicationabcanbecomputedusing3realmultplicationsand5realadditions.kFT8.14Thecenterfrequencyofbink:f(k)=whereN=#ofbins,andFisthesamplingcNTêúfrequency.Invertingwehavek(f)=êfNú.Therefore,theabsolutedifferencefromoneoftheêëFTúûgivenfourtones(150Hz,375Hz,620Hz,and850Hz)tothecenterofitsbinisgivenbyFTêfNúdist(N,f)=f-êú.ItfollowsfromthisequationthatthedistancegoestozeroifNêëFTúûFTêfNúfNêú=forisaninteger.NêëFTúûFTfNiThetotaldistanceisreducedtozeroifisanintegerfori=1,K,4.TheminimumNforFTwhichthisistrueis500.However,thetotaldistancecanbesmall,butnonzero,forsignificantlysmallervaluesofN.TheMATLABgeneratedplotgivenbelowshowsaniceminimumatN=21.Totalabsolutedifferenceplot80060040020005101520253035Numberofbins(N)316
8.15x[n]y[n]y[–1]=0–1z–kWN–N/21X(z)1+zH(z)=.Hence,Y(z)==.k-k-1-k-1-k-11-Wz1-Wz1-WzNNN–N/21+z–N/2-1-1-2-2-N/2-N/2Fork=1,Y(z)==(1+z)(1+Wz+Wz+L+Wz+L-1-1NNN1-WzN-1-2-(N-2)/2-N/2-(N-1)Hence,y[n]={1,W,W,L,W,1+W,L,1+W,L}NNNNN–N/21+zFork=N/2,Y(z)=.Hence,-N/2-11-WzNy[n]={1,-1,1,-1,K-1,2,0,2,0,K0}8.16x[0]X[0]x[1]W2X[4]NW0–1Nx[2]X[2]W0–1Nx[3]X[6]W0–12–1NWNx[4]X[1]W0–1Nx[5]X[5]W0–1W1–1NNx[6]X[3]W0–1W2–1NNx[7]X[7]W0–1W2–13–1NNWN8.17317
x[0]X[0]x[1]X[1]W0N–1x[2]X[2]W0Nx[3]X[3]W0W1NNx[4]–1X[4]W0–1Nx[5]–1–1X[5]W0–1W2NNx[6]0–1X[6]WN–1W2–1Nx[7]X[7]W0–1W2–1W3–1NNNN-1nk8.18X[k]=åx[n]WNn=0NNN-1-1-1r1r1r1nkr1nkr1+knkr1+(r1-1)k=åx[nr1]WN+åx[nr1+1]WN+L+åx[nr1+r1-1]WNn=0n=0n=0N-1r1-1r1nkr1ki=ååx1[nr414+2i]4W4N3×WNi=0n=0(N/r)-pointDFT1Thus,ifthe(N/r1)-pointDFThasbeencalculated,weneedatthefirststageanadditional(r1–1)multiplicationstocomputeonesampletheN-pointDFTX[k]andasaresult,additional(r1–1)NmultiplicationsarerequiredtocomputeallNsamplesoftheN-pointDFT.Decomposingitfurther,itfollowsthenthatadditional(r2–1)Nmultiplicationsareneededatthesecondstage,andsoon.Therefore,Totalnumberofmultiply(add)operations=(r1–1)N+(r2–1)N+...+(rn–1)Nænö=çèåi=1ri-n÷øN.3-13-238.19X(z)=X(z)+zX(z)+zX(z).Thus,theN-pointDFTcanbeexpressedas012k2kX[k]=X[]+WX[]+WX[].Hence,thestructural0N/3N1N/3N2N/3interpretationofthefirststageoftheradix-3DFTisasindicatedbelow:318
X0[N]xN0[n]-point3x[n]33X[k]DFTzNX1[N]Wkx1[n]-point3N33DFTzNX2[N]W2kxN2[n]-point333DFT8nk0×k3k6k8.20X[k]=åx[n]W9=(x[0]W9+x[3]W9+x[6]W9)n=0k4k7k2k5k8k+(x[1]W9+x[4]W9+x[7]W9)+(x[2]W9+x[5]W9+x[8]W9)0×kk2k0×kk2kk=(x[0]W3+x[3]W3+x[6]W3)+(x[1]W3+x[4]W3+x[7]W3)W90×kk2k2kk2k+(x[2]W3+x[5]W3+x[8]W3)W9=G0[3]+G1[3]W9+G2[3]W9,0×kk2k0×kk2kwhereG[]=x[0]W+x[3]W+x[6]W,G[]=x[1]W+x[4]W+x[7]W,03333133330×kk2kandG[]=x[2]W+x[5]W+x[8]W,arethree3-pointDFTs.Aflow-graph23333representationofthisDITmixed-radixDFTcomputationschemeisshownbelow:G0[0]x[0]X[0]3-pointG0[1]x[3]X[1]DFTG0[2]x[6]X[2]G1[0]x[1]X[3]3-pointG1[1]x[4]X[4]DFTG1[2]x[7]X[5]G2[0]x[2]X[6]3-pointG[1]x[5]2X[7]DFTG[2]2x[8]X[8]wherethetwiddlefactorsforcomputingtheDFTsamplesareindicatedbelowforatypicalDFTsample:G[k]00×kkW3W3G[k]X[k]12kW3G[k]2319
Intheabovediagram,the3-pointDFTcomputationiscarriedoutasindicatedbelow:x[l]0Gl[0]W30W31Wx[l+3]3G[1]l2W32W3x[l+6]1Gl[2]W314nk0×k3k6k9k12k8.21X[k]=åx[n]W15=(x[0]W15+x[3]W15+x[6]W15+x[9]W15+x[12]W15)n=0k4k7k10k13k+(x[1]W15+x[4]W15+x[7]W15+x[10]W15+x[13]W15)2k5k8k11k14k+(x[2]W15+x[5]W15+x[8]W15+x[11]W15+x[14]W15)k2k=G[]+G[]W+G[]W,where05151525150×kk2k3k4kG[]=x[0]W+x[3]W+x[6]W+x[9]W+x[12]W,05555550×kk2k3k4kG[]=x[1]W+x[4]W+x[7]W+x[10]W+x[13]W,and15555550×kk2k3k4kG[]=x[2]W+x[5]W+x[8]W+x[11]W+x[14]W.2555555Aflow-graphrepresentationofthisDITmixed-radixDFTcomputationschemeisshownbelow:320
G0[0]x[0]X[0]G[1]x[3]0X[1]5-pointG[2]x[6]0X[2]DFTG0[3]x[9]X[3]G0[4]x[12]X[4]x[1]G1[0]X[5]G1[1]x[4]X[6]5-pointG[2]1x[7]X[7]DFTG[3]1x[10]X[8]G1[4]x[13]X[9]G2[0]x[2]X[10]G2[1]x[5]X[11]5-pointG2[2]x[8]X[12]DFTG2[3]x[11]X[13]G[4]2x[14]X[14]8.22X[0]x[0]1/21/21/2X[1]x[4]1/21/2–1W–0/2NX[2]x[2]1/2–1W–0/21/2NX[3]x[6]1/2–1–2–1–0WN/2WN/2X[4]x[1]–1W–0/21/21/2NX[5]x[5]–1–11/2–1–0WN/2WN/2X[6]x[3]–1–1W–0/21/2NX[7]x[7]–1–3–1–2–1–0W/2W/2W/2NNN8.231/NXre[k]xre[n]DFTXim[k]xim[n]1/N321
N-1nkNow,bydefinition,q[n]=Im{X[n]}+jRe{X[n]}.ItsN-pointDFTisQ[k]=åq[n]WN.n=0N-1ææ2pmköæ2pmkööThus,Re{Q[k]}=åçIm{X[m]}cosç÷+Re{X[m]}sinç÷÷,(18)èèNøèNøøm=0N-1ææ2pmköæ2pmkööIm{Q[k]}=åç–Im{X[m]}sinç÷+Re{X[m]}cosç÷÷,(19)èèNøèNøøm=0N-11-mkFromthedefinitionoftheinverseDFTweobservex[k]=åX[m]WN.Hence,Nm=0N-11ææ2pmköæ2pmkööRe{x[k]}=åçRe{X[m]}cosç÷-Im{X[m]}sinç÷÷,(20)NèèNøèNøøm=0N-11ææ2pmköæ2pmkööIm{x[k]}=åçIm{X[m]}cosç÷+Re{X[m]}sinç÷÷.12`(21)NèèNøèNøøm=01ComparingEqs.(19)and(20),wegetRe{x[n]}=Im{Q[k]},Nk=n1andcomparingEqs.(18)and(21)wegetIm{x[n]}=Re{Q[k]}.Nk=nX[0],ifn=0,8.24r[n]=X[<–n>]=Therefore,N{X[N-n],ifn¹0.N-1N-1N-1nknknkR[k]=år[n]WN=r[0]+år[n]WN=X[0]+åX[N-n]WNn=0n=1n=1N-1N-1N-1(N-n)k-nk-nk=X[0]+åX[n]WN=X[0]+åX[n]WN=åX[n]WN=Nx[k].n=1n=1n=01Thus,x[n]=×R[k].Nk=n8.25Lety[n]denotetheresultofconvolvingalength-Lsequencex[n]withalength-Nsequenceh[n].Thelengthofy[n]isthenL+N–1.HereL=8andN=5,hencelengthofy[n]is12.Method#1:Directlinearconvolution-Foralength-Lsequencex[n]andalength-Nh[n],æNöæ5ö#ofrealmult.=2çån÷+N(L-N-1)=2çån÷+5(8-5-1)=40.ç÷ç÷èn=1øèn=1øMethod#2:Linearconvolutionviacircularconvolution-Sincey[n]isoflength12,togetthecorrectresultweneedtopadbothsequenceswithzerostoincreasetheirlengthsto12beforecarryingoutthecircularconvolution.#ofrealmult.=12´12=144.322
Method#3:Linearconvolutionviaradix-2FFT-Theprocessinvolvescomputingthe16-pointFFTG[k]ofthelength-16complexsequenceg[n]=x[n]+jh[n]wherex[n]andeeeh[n]arelength-16sequencesobtainedbyzero-paddingx[n]andh[n],respectively.Thenerecoveringthe16-pointDFTs,X[k]andH[k],ofx[n]andh[n],respectively,fromG[k].eeeeFinally,theIDFToftheproductY[k]=X[k]×H[k]yieldsy[n].eeNow,thefirststageofthe16-pointradix-2FFTrequires0complexmultiplications,thesecondstagerequires0complexmultiplications,thethirdstagerequires4complexmultiplications,andthelaststagerequires6complexmultiplicationsresultinginatotalof10complexmultiplications.#ofcomplexmult.toimplementG[k]=10#ofcomplexmult.torecoverX[k]andH[k]fromG[k]=0ee#ofcomplexmult.toformY[k]=X[k]×H[k]=16ee#ofcomplexmult.toformtheIDFTofY[k]=10Hence,thetotalnumberofcomplexmult.=36Adirectimplementationofacomplexmultiplicationrequires4realmultiplicationsresultinginatotalof4´36=144realmultiplicationsforMethod#3.However,ifacomplexmultiplycanbeimplementedusing3realmultiplies(seeProblem8.13),inwhichcaseMethod#3requiresatotalof3´36=108realmultiplications.8.26Method#1:Total#ofrealmultiplicationsrequiredæNöæ6ö=2çån÷+N(L-N-1)=2çån÷+6(8-6-1)=48.ç÷ç÷èn=1øèn=1øMethod#2:Total#ofrealmultiplicationsrequired=132=169.Method#3:Linearconvolutionviaradix-2FFT-Theprocessinvolvescomputingthe16-pointFFTG[k]ofthelength-16complexsequenceg[n]=x[n]+jh[n]wherex[n]andeeeh[n]arelength-16sequencesobtainedbyzero-paddingx[n]andh[n],respectively.Thenerecoveringthe16-pointDFTs,X[k]andH[k],ofx[n]andh[n],respectively,fromG[k].eeeeFinally,theIDFToftheproductY[k]=X[k]×H[k]yieldsy[n].eeNow,thefirststageofthe16-pointradix-2FFTrequires0complexmultiplications,thesecondstagerequires0complexmultiplications,thethirdstagerequires4complexmultiplications,andthelaststagerequires6complexmultiplicationsresultinginatotalof10complexmultiplications.#ofcomplexmult.toimplementG[k]=10#ofcomplexmult.torecoverX[k]andH[k]fromG[k]=0ee#ofcomplexmult.toformY[k]=X[k]×H[k]=16ee#ofcomplexmult.toformtheIDFTofY[k]=10323
Hence,thetotalnumberofcomplexmult.=36Adirectimplementationofacomplexmultiplicationrequires4realmultiplicationsresultinginatotalof4´36=144realmultiplicationsforMethod#3.However,ifacomplexmultiplycanbeimplementedusing3realmultiplies(seeProblem8.13),inwhichcaseMethod#3requiresatotalof3´36=108realmultiplications.8.27(a)Sincetheimpulseresponseofthefilterisoflength34,thetransformlengthNshouldbegreaterthan34.IfLdenotesthenumberofinputsamplesusedforconvolution,thenL=N–33.SoforeveryLsamplesoftheinputsequence,anN-pointDFTiscomputedandmultipliedwithanN-pointDFToftheimpulseresponsesequenceh[n](whichneedstobecomputedonlyonce),andfinallyanN-pointinverseoftheproductsequenceisevaluated.Hence,thetotalnumberRMofcomplexmultiplicationsrequired(assumingNisapower-of-2)isgivenbyRé1024ùNM=(Nlog2N+N)+2log2NêêN-33úúItshouldbenotedthatindevelopingtheaboveexpression,multiplicationsduetotwiddlefactorsofvalues±1and±jhavenotbeenexcluded.ThevaluesofRMfordifferentvaluesofNareasfollows:ForN=64,RM=15,424ForN=128,RM=11,712forN=256,RM=12,544forN=512,RM=17,664Hence,N=128istheappropriatechoiceforthetransformlengthrequiring14,848complexmultiplicationsorequivalently,11,712´3=35,136realmultiplications.SincethefirststageoftheFFTcalculationprocessrequiresonlymultiplicationsby±1,thetotalnumberofcomplexmultiplicationsforN=128isactuallyRé1024ùNNM=(Nlog2N+N)+2log2N-2=11,648êêN-33úúorequivalently,11,648´3=34,944realmultiplications.(b)Fordirectconvolution,#ofrealmultiplications=æNöæ34ö2çån÷+N(L-N-1)=2çån÷+34(1024-34-1)=34,816.ç÷ç÷èn=1øèn=1ø8.28Fromtheflow-graphofthe8-pointsplit-radixFFTalgorithmgivenbelowitcanbeseenthatthetotalnumberofcomplexmultiplicationsrequiredis2.Ontheotherhand,thetotalnumberofcomplexmultiplicationsrequiredforastandardDIFFFTalgorithmisalso2.324
x[0]X[0]x[1]X[4]–1x[2]X[2]–1x[3]X[6]–1–j–1x[4]X[1]–1x[5]X[5]–1W1–18x[6]X[3]–1–j–1x[7]X[7]–1–j–1W3–188.29Ifmultiplicationsby±j,±1areignored,theflow-graphshownbelowrequires8complex=24realmultiplications.Aradix-2DIF16-pointFFTalgorithm,ontheotherhand,requires10complexmultiplications=30realmultiplications.x[0]X[0]x[1]X[8]–1x[2]X[4]–1–jx[3]X[12]–1–1x[4]X[2]–1x[5]X[10]–1W1–18x[6]X[6]–1–j–1x[7]X[14]–1–j–1W3–18x[8]X[1]–10W16x[9]X[9]–1W1–116x[10]X[5]–1W2–116x[11]X[13]–1W3–1–116x[12]X[3]–1–j–10W16x[13]X[11]–1–j–1W3–116x[14]X[7]–1–j–1W6–116x[15]X[15]–1–j–1W9–1–j–116325
8.30(a)FromEq.(8.97a),x[n]=x[n+Nn]where0£n£N-1,and0£n£N-1.Now,1121122N-1nkusingEq.(8.97b)wecanrewriteX[k]=åx[n]WN,0£k£N-1,asn=0N1N2–1N1-1N2–1X[Nk+k]=x[n]Wn(N2k1+k2)=x[n+Nn]W(n1+N1n2)(N2k1+k2)212åNåå112Nn=0n=0n=012N1-1N2–1=x[n+Nn]Wn1N2k1WN1n2N2k1WN1n2k2Wn1k2.åå112NNNNn=0n=012Since,Wn1N2k1=Wn1k1,WN1N2n1k1=1,andWN1n2k2=Wn2k2,wegetNN1NNN2N1-1éæN2–1öùX[k]=X[Nk+k]=êçx[n+Nn]Wn2k2÷Wn1k2úWn1k1.(22)212åêçå112N2÷NúN1n=0êçèn=0÷øú1ë2û(b)ForN1=2andN2=N/2,theaboveDFTcomputationschemeleadstoéæNöù1êç–1÷ú2X[k]=X[Nk+k]=êçx[n+2n]Wn2k2÷Wn1k2úWn1k1212åêçå12N/2÷Nú2n=0êçn=0÷ú1êç2÷úëèøûNN–1–122=x[2n]Wn2k2+Wk1Wk2x[2n+1]Wn2k2å2N/2N1Nå2N/2n=0n=022NN–1–122n2k2kn2k2=åx[2n2]WN/2+WNåx[2n2+1]WN/2n=0n=022whichisseentobethefirststageintheDITDFTcomputation.Ontheotherhand,forN1=N/2andN2=2,theaboveDFTcomputationschemeleadstoN–1éæöù21X[k]=X[2k+k]=êçx[n+Nn]Wn2k2÷Wn1k2úWn1k112åêçå1222÷NúN/2n=0êçèn=0÷øú1ë2ûN–12æNk2ön1k2n1k1=åçèx[n1]+x[n1+2](-1)÷øWNWN/2n=01N–12ìæNönkünk=íx[n]+(-1)k2x[n+]12ýW11åîçè112÷øWNþN/2n=01whichrepresentsthefirststageoftheDIFFFTalgorithm.326
(c)IntheDFTcomputationschemeofEq.(22),wefirstcomputeatotalofN2N1-pointn1k2DFTs,multiplyalltheN1N2=NcomputedDFTcoefficientsbythetwiddlefactorsW,andNfinallycalculateatotalofN2N1-pointDFTs.IfR(N)denotesthetotalnumberofmultiplicationsneededtocomputeanN-pointDFT,thenthetotalnumberofmultplicationsrequiredintheDFTcomputationschemeofEq.(22)isgivenby(i)N×R(N1)forthefirststep,2(ii)N2N1=Nformultiplicationsbythetwiddlefactors,and(iii)N×R(N2)forthelaststep.111Therefore,R(N)=N2×R(N1)+N+N1×R(N2)=N(R(N1)+R(N2)+1).NN12(d)ForN=2n,chooseN=2,i=1,2,...n.NowfromFigure8.24fora2-pointDFTiænöNR(Ni)=2.Hence,R(N)=Nçè÷ø=log2N.228.28(a)é0ùé0ù1000W00010W00000ê8úê8úê01000W100úê010W20000úê8úê8úê001000W20úê10W000000úê8úê8úê0001000W3úê010W20000úV=ê48ú,V=ê80ú,8ê1000W000ú4ê000010W0úê8úê8úê01000W500úê0000010W2úê8úê8úê6úê0ú001000W0000010W0ê8úê8ú72êë0001000W8úûêë0000010W8úûé0ù1W000000ê8úê1W4000000úê8úéùê001W00000ú10000000ê8úê00001000úê001W40000úê00100000úV=ê8ú,ê00000010ú2ê00001W000úE=êú.ê8úê01000000úê00001W400úê00000100úê8úê00010000úê0úêú0000001W8ë00000001ûêú4êë0000001W8úûAscanbeseenfromtheabove,multiplicationbyeachmatrixVk,k=1,2,3,requiresatmost8complexmultiplications.(b)Thetransposeofthematricesgiveninpart(a)areasfollows:327
é10001000ùé10100000ùê01000100úê01010000úê00100010úêW00W000000úêúê88úê0000104001úê22úTêW8000W8000úTê0W80W80000úV8=ê15ú,V4=ê00001010ú,ê0W8000W800úê00000101úê26úê00úê00W8000W80úê0000W80W80úê37úê22úêë000W8000W8úûêë00000W80W8úûé11000000ùêW0W4000000úé10000000ùê88úê00001000úê00110000úêúê00W0W40000úê00100000úVT=ê88ú,ET=E=ê00000010ú.2ê00001100úê01000000úê0000W0W400úê00000100úê88úê00010000úê00000011úêúê000000W0W4úë00000001ûë88ûTTTTItiseasytoshowthattheflow-graphrepresentationofD=EVVVispreciselythe8-8248pointDIFFFTalgorithjmofFigure8.28.N–1N–12N–12ln2ln2lnN8.29X[2l]=åx[n]WN=åx[n]WN+åx[n]WN,l=0,1,K,–1.2n=0n=0n=N/2NReplacingnbyn+intheright-mostsumweget2NNN–1–1–12222lnN2lnNlìNülnNX[2l]=åx[n]WN+åx[n+2]WNWN=åíîx[n]+x[n+2]ýþWN/2,0£l£2-1.n=0n=0n=0NN3N–1–1–1424N–1(4l+1)n(4l+1)n(4l+1)n(4l+1)nX[4l+1]=åx[n]WN+åx[n]WN+åx[n]WN+åx[n]WN,n=0NN3Nn=n=n=424NNNwhere0£l£–1.Replacingnbyn+inthesecondsum,nbyn+inthethirdsum,4423Nandnbyn+inthefourthsum,weget4NN–1–1444lnnN4lnnlNN/4X[4l+1]=åx[n]WNWN+åx[n+4]WNWNWNWNn=0n=0NN–1–144N4lnn2lNN/23N4lnn3lN3N/4+åx[n+2]WNWNWNWN+åx[n+4]WNWNWNWNn=0n=0lN2lN3lNN/4N/23N/4Now,W=W=W=1,W=-j,W=-1,andW=+j.Therefore,NNNNNN328
N–14ìæNöæN3NöüNnlnX[4l+1]=åíçèx[n]-x[n+]÷ø-jçèx[n+]-x[n+]÷øýWNWN/4,0£l£-1.î244þ4n=0NN–1–144(4l+3)nN(4l+3)n(4l+3)N/4Similarly,X[4l+3]=åx[n]WN+åx[n+]WNWN4n=0n=0NN–1–144N(4l+3)n(4l+3)N/23N(4l+3)n(4l+3)3N/4+åx[n+]WNWN+åx[n+]WNWN24n=0n=0NN–1–1444ln3nN4ln3nlN3N/4=åx[n]WNWN+åx[n+4]WNWNWNWNn=0n=0NN–1–144N4ln3n2lN6N/43N4ln3n3lN9N/4=åx[n+2]WNWNWNWN+åx[n+4]WNWNWNWNn=0n=0N–14ìæNöæN3NöüN3nln=åíçèx[n]-x[n+]÷ø+jçèx[n+]-x[n+]÷øýWNWN/4,0£l£-1.î244þ4n=0Thebutterflyhereisasshownbelowwhichisseentorequiretwocomplexmultiplications.x[n]X[4l]Nx[n+]X[4l+2]4nN–1WNx[n+]X[4l+1]2–j3n3NWNx[n+]X[4l+3]4–1j8.30Fromtheflow-graphofthe8-pointsplit-radixFFTalgorithmgivenbelowitcanbeseenthatthetotalnumberofcomplexmultiplicationsrequiredis2.Ontheotherhand,thetotalnumberofcomplexmultiplicationsrequiredforastandardDIFFFTalgorithmisalso2.329
x[0]X[0]x[1]X[4]–1x[2]X[2]–1x[3]X[6]–1–j–1x[4]X[1]–1x[5]X[5]–1W1–18x[6]X[3]–1–j–1x[7]X[7]–1–j–1W3–188.31Ifmultiplicationsby±j,±1areignored,theflow-graphshownbelowrequires8complex=24realmultiplications.Aradix-2DIF16-pointFFTalgorithm,ontheotherhand,requires10complexmultiplications=30realmultiplications.x[0]X[0]x[1]X[8]–1x[2]X[4]–1–jx[3]X[12]–1–1x[4]X[2]–1x[5]X[10]–1W1–18x[6]X[6]–1–j–1x[7]X[14]–1–j–1W3–18x[8]X[1]–10W16x[9]X[9]–1W1–116x[10]X[5]–1W2–116x[11]X[13]–1W3–1–116x[12]X[3]–1–j–10W16x[13]X[11]–1–j–1W3–116x[14]X[7]–1–j–1W6–116x[15]X[15]–1–j–1W9–1–j–116330
8.32(a)FromEq.(8.97a),x[n]=x[n+Nn]where0£n£N-1,and0£n£N-1.Now,1121122N-1nkusingEq.(8.97b)wecanrewriteX[k]=åx[n]WN,0£k£N-1,asn=0N1N2–1N1-1N2–1X[Nk+k]=x[n]Wn(N2k1+k2)=x[n+Nn]W(n1+N1n2)(N2k1+k2)212åNåå112Nn=0n=0n=012N1-1N2–1=x[n+Nn]Wn1N2k1WN1n2N2k1WN1n2k2Wn1k2.åå112NNNNn=0n=012Since,Wn1N2k1=Wn1k1,WN1N2n1k1=1,andWN1n2k2=Wn2k2,wegetNN1NNN2N1-1éæN2–1öùX[k]=X[Nk+k]=êçx[n+Nn]Wn2k2÷Wn1k2úWn1k1.(22)212åêçå112N2÷NúN1n=0êçèn=0÷øú1ë2û(b)ForN1=2andN2=N/2,theaboveDFTcomputationschemeleadstoéæNöù1êç–1÷ú2X[k]=X[Nk+k]=êçx[n+2n]Wn2k2÷Wn1k2úWn1k1212åêçå12N/2÷Nú2n=0êçn=0÷ú1êç2÷úëèøûNN–1–122=x[2n]Wn2k2+Wk1Wk2x[2n+1]Wn2k2å2N/2N1Nå2N/2n=0n=022NN–1–122n2k2kn2k2=åx[2n2]WN/2+WNåx[2n2+1]WN/2n=0n=022whichisseentobethefirststageintheDITDFTcomputation.Ontheotherhand,forN1=N/2andN2=2,theaboveDFTcomputationschemeleadstoN–1éæöù21X[k]=X[2k+k]=êçx[n+Nn]Wn2k2÷Wn1k2úWn1k112åêçå1222÷NúN/2n=0êçèn=0÷øú1ë2ûN–12æNk2ön1k2n1k1=åçèx[n1]+x[n1+2](-1)÷øWNWN/2n=01N–12ìæNönkünk=íx[n]+(-1)k2x[n+]12ýW11åîçè112÷øWNþN/2n=01whichrepresentsthefirststageoftheDIFFFTalgorithm.331
(c)IntheDFTcomputationschemeofEq.(22),wefirstcomputeatotalofN2N1-pointn1k2DFTs,multiplyalltheN1N2=NcomputedDFTcoefficientsbythetwiddlefactorsW,andNfinallycalculateatotalofN2N1-pointDFTs.IfR(N)denotesthetotalnumberofmultiplicationsneededtocomputeanN-pointDFT,thenthetotalnumberofmultplicationsrequiredintheDFTcomputationschemeofEq.(22)isgivenby(i)N×R(N1)forthefirststep,2(ii)N2N1=Nformultiplicationsbythetwiddlefactors,and(iii)N×R(N2)forthelaststep.111Therefore,R(N)=N2×R(N1)+N+N1×R(N2)=N(R(N1)+R(N2)+1).NN12(d)ForN=2n,chooseN=2,i=1,2,...n.NowfromFigure8.24fora2-pointDFTiænöNR(Ni)=2.Hence,R(N)=Nçè÷ø=log2N.228.33(a)N=12.ChooseN1=4andN2=3.Thus,ì0£n1£3ì0£k1£3n=n1+4n2,í,andk=3k1+k2,í.î0£n2£2î0£k2£2nkn10123k21012320x[0]x[1]x[2]x[3]0X[0]X[3]X[6]X[9]1x[4]x[5]x[6]x[7]1X[1]X[4]X[7]X[10]2x[8]x[9]x[10]x[11]2X[2]X[5]X[8]X[11](b)N=15.ChooseN1=3andN2=5.Thus,ì0£n1£2ì0£k1£2n=n1+3n2,í,andk=5k1+k2,í.î0£n2£4î0£k2£4n1kn012k2101220x[0]x[1]x[2]0X[0]X[5]X[10]1x[3]x[4]x[5]1X[1]X[6]X[11]2x[6]x[7]x[8]2X[2]X[7]X[12]3x[9]x[10]x[11]3X[3]X[8]X[13]4x[12]x[13]x[14]4X[4]X[9]X[14](c)N=21.ChooseN1=7,andN2=3.Thus,ì0£n1£6ì0£k1£6n=n1+7n2,í,andk=3k1+k2,í.î0£n2£2î0£k2£2332
n1n201234560x[0]x[1]x[2]x[3]x[4]x[5]x[6]1x[7]x[8]x[9]x[10]x[11]x[12]x[13]2x[14]x[15]x[16]x[17]x[18]x[19]x[20]k1k012345620X[0]X[3]X[6]X[9]X[12]X[15]X[18]1X[1]X[4]X[7]X[10]X[13]X[16]X[19]2X[2]X[5]X[8]X[11]X[14]X[17]X[20](d)N=35.ChooseN1=7,andN2=5.Thus,ì0£n1£6ì0£k1£6n=n1+7n2,í,andk=5k1+k2,í.î0£n2£4î0£k2£4n1n20123456x[0]x[1]x[2]x[3]x[4]x[5]x[6]01x[7]x[8]x[9]x[10]x[11]x[12]x[13]2x[14]x[15]x[16]x[17]x[18]x[19]x[20]3x[21]x[22]x[23]x[24]x[25]x[26]x[27]4x[28]x[29]x[30]x[31]x[32]x[33]x[34]k1k012345620X[0]X[5]X[10]X[15]X[20]X[25]X[30]1X[1]X[6]X[11]X[16]X[21]X[26]X[31]2X[2]X[7]X[12]X[17]X[22]X[27]X[32]3X[3]X[8]X[13]X[18]X[23]X[28]X[33]4X[4]X[9]X[14]X[19]X[24]X[29]X[34]8.34(a)n=,k=,whereN=N1N2.12N12NN1-1N2-1(An1+Bn2)(Ck1+Dk2)X[k]=X[N]=ååx[N]WNn=0n=012N1-1N2-1=x[]WACn1k1WADn1k2WBDn2k2WBCn2k1.åå12NNNNNn=0n=012TocompletelyeliminatethetwiddlefactorswerequireWACn1k1WADn1k2WBDn2k2WBCn2k1=Wn1k1Wn2k2.NNNNN1N2ToachievethisweneedtochoosetheconstantsA,B,C,andD,suchthat=0,=0,=N,and=N.(23)NNN2N1333
N1-1N2-1n1k1n2k2Then,wecanwriteX[N]==ååx[N]WN1WN2.n=0n=012(b)WeshallshowthatthefollowingchoiceoftheconstantssatisfytheconstraintsofEq.(23):-1-1A=N,B=N,C=N,andD=N,2122N111N2-1-1whereisthemultiplicativeinverseofN1evaluatedmoduloN2,i.e.if=1N21N2a,then=1.Hence,NamustbeexpressibleasNb+1,wherebisanyinteger.1N212-1-1Likewise,isthemultiplicativeinverseofN2evaluatedmoduloN1,andif2N12N1=g,thenNg=Nd+1,wheredisanyinteger.Now,fromEq.(23),21-1=>===N.Similarly,N222N1N21N212N2-1=>===N.Next,weN111N2N12N121N1-1-1observethat=>=>==0,andN211N2N1N2NN-1-1=>=>==0.N122N1N2N1NNN1-1N2-1Hence,X[k]=X[]=x[]WN2n1k1WN1n2k212Nåå12NNNn=0n=012N1-1N2-1n1k1n2k2=ååx[N]WN1WN2n=0n=012N2-1æN1-1öçn1k1÷n2k2=åçåx[N]WN1÷WN2.n=0çèn=0÷ø218.35(a)N=12.ChooseN1=4andN2=3.-1-1A=3,B=4,C=3<3>=9,D=4<4>=4.43ì0£n£3,ì0£k£3,n=<3n+4n>,í1k=<9k+4k>í11212î0£n2£2,1212î0£k2£2.nn1kk120123201230x[0]x[3]x[6]x[9]0X[0]X[9]X[6]X[3]1x[4]x[7]x[10]x[1]1X[4]X[1]X[10]X[7]2x[8]x[11]x[2]x[5]2X[8]X[5]X[2]X[11](b)N=15.ChooseN1=3andN2=5.-1-1A=5,B=3,C=5<5>=10,D=3<3>=6.35ì0£n£2,ì0£k£2,n=<5n+3n>,í1k=<10k+6k>,í11215î0£n2£4,1215î0£k2£4.334
n1kn2012k210120x[0]x[5]x[10]0X[0]X[10]X[5]1x[3]x[8]x[13]1X[6]X[1]X[11]2x[6]x[11]x[1]2X[12]X[7]X[2]3x[9]x[14]x[4]3X[3]X[13]X[8]4x[12]x[2]x[7]4X[9]X[4]X[14](c)N=21.ChooseN1=7andN2=3.-1-1A=3,B=7,C=3<3>=3´5=15,D=7<7>=7´1=7.73ì0£n£6,ì0£k£6,n=<3n+7n>,í1k=<15k+7k>,í11221î0£n2£2,1221î0£k2£2.n1n201234560x[0]x[3]x[6]x[9]x[12]x[15]x[18]1x[7]x[10]x[13]x[16]x[19]x[1]x[4]2x[14]x[17]x[20]x[2]x[5]x[8]x[11]k1k201234560X[0]X[15]X[9]X[3]X[18]X[12]X[6]1X[7]X[1]X[16]X[10]X[4]X[19]X[13]2X[14]X[8]X[2]X[17]X[11]X[5]X[20](d)N=35.ChooseN1=7andN2=5.-1-1A=5,B=7,C=5<5>=5´3=15,D=7<7>=7´3=21.75ì0£n£6,ì0£k£6,n=<5n+7n>,í1k=<15k+21k>,í11235î0£n2£4,1235î0£k2£4.n1n201234560x[0]x[5]x[10]x[15]x[20]x[25]x[30]1x[7]x[12]x[17]x[22]x[27]x[32]x[2]2x[14]x[19]x[24]x[29]x[34]x[4]x[9]3x[21]x[26]x[31]x[1]x[6]x[11]x[16]4x[28]x[33]x[3]x[8]x[13]x[18]x[23]335
k1k201234560X[0]X[15]X[30]X[10]X[25]X[5]X[20]1X[21]X[1]X[16]X[31]X[11]X[26]X[6]2X[7]X[22]X[2]X[17]X[32]X[12]X[27]3X[28]X[8]X[23]X[3]X[18]X[33]X[13]4X[14]X[29]X[9]X[24]X[4]X[19]X[34]8.36N=12.N1=4andN2=3.-1-1A=3,B=4,C=3<3>=9,D=4<4>=4.43ì0£n£3,ì0£k£3,n=<3n+4n>,í1k=<9k+4k>,í11212î0£n2£4,1212î0£k2£2.nkn210123k2101230x[0]x[3]x[6]x[9]0X[0]X[9]X[6]X[3]1x[4]x[7]x[10]x[1]1X[4]X[1]X[10]X[7]2x[8]x[11]x[2]x[5]2X[8]X[5]X[2]X[11]n=<9n+4n>k=<3k+4k>12121212nn1kk101232012320x[0]x[9]x[6]x[3]0Y[0]Y[3]Y[6]Y[9]1x[4]x[1]x[10]x[7]1Y[4]Y[7]Y[10]Y[1]2x[8]x[5]x[2]x[11]2Y[8]Y[11]Y[2]Y[5]Hence,X[2k]=Y[2k],andX[2k+1]=X[2k+1]=Y[<6+(2k+1)>],k=0,1,...,5,12-1-18.37N=10,N1=2,andN2=5.ChooseA=5,B=2,C=5<5>=5,D=2<2>=6.25n1kkn201n2101k21010x[0]x[5]0G[0,0]G[1,0]0X[0]X[5]1x[2]x[7]1G[0,1]G[1,1]1X[6]X[1]2x[4]x[9]2G[0,2]G[1,2]2X[2]X[7]3x[6]x[1]3G[0,3]G[1,3]3X[8]X[3]4x[8]x[3]4G[0,4]G[1,4]4X[4]X[9]336
G[0,0]x[0]2-pointX[0]G[1,0]x[5]DFTX[6]G[0,1]5-pointx[2]2-pointX[2]DFTG[1,1]x[7]DFTX[8]G[0,2]x[4]2-pointX[4]G[1,2]x[9]DFTX[5]G[0,3]x[6]2-pointX[1]DFTG[1,3]5-pointx[1]DFTX[7]G[0,4]x[8]2-pointX[3]G[1,4]x[3]DFTX[9]Theflow-graphofthe2-pointDFTisgiveninFigure8.21.Theflow-graphofthe5-pointDFTisshownbelow1W2W3W4W2W4W1W2W3W1W4W4W3W2W1W-1-18.38N=12,N1=4,andN2=3.ChooseA=3,B=4,C=3<3>=9,andD=4<4>=4.43n1knn120123201230x[0]x[3]x[6]x[9]0G[0,0]G[1,0]G[2,0]G[3,0]1x[4]x[7]x[10]x[1]1G[0,1]G[1,1]G[2,1]G[3,1]2x[8]x[11]x[2]x[5]2G[0,2]G[1,2]G[2,2]G[3,2]337
k1k201230X[0]X[9]X[6]X[3]1X[4]X[1]X[10]X[7]2X[8]X[5]X[2]X[11]G[0,0]x[0]X[0]G[1,0]3-pointx[3]X[4]4-pointDFTDFTG[2,0]x[6]X[8]G[3,0]x[9]X[9]G[0,1]3-pointx[4]X[1]DFTG[1,1]x[7]X[5]4-pointDFTG[2,1]x[10]X[6]G[3,1]3-pointx[1]X[10]DFTG[0,2]x[8]X[2]G[1,2]x[11]X[3]4-pointDFTG[2,2]3-pointx[2]X[7]DFTG[3,2]x[5]X[11]Theflow-graphsofthe3-pointDFTandthe4-pointDFTareshownbelow:j–1–j–11jW2–jW–j2W–14Wj8.39Notethat3072=512´6.NowanN-pointDFT,withNdivisibleby6,canbecomputedasN-1nkk2kfollows:X[k]=åx[n]WN=X0[N/6]+WN×X1[N/6]+WN×X2[N/6]n=03k4k5k+W×X[]+W×X[]+W×X[],whereN3N/6N4N/6N5N/6N-16rkXl[N/6]=åx[6r+l]WN/6,0£l£5.ForN=3072,wethusgetr=0k2kX[k]=X[]+W×X[]+W×X[]05123072151230722512338
3k4k5k+W×X[]+W×X[]+W×X[],where307235123072451230725512511rkXl[512]=åx[6r+l]W512,0£l£5.r=0x000[n]X000[512]x[n]6512-pointX[k]FFTkW1536zx001[n]512-pointX001[512]6FFTkWkW30721536zx010[n]X010[512]512-point6FFTzx011[n]512-pointX011[512]6FFTkW1536zx100[n]X100[512]512-point6FFTkW1536zx101[n]512-pointX101[512]6FFTNNowanN-pointFFTalgorithmrequireslogNcomplexmultiplicationsandNlogN222NNæNöcomplexadditions.Hence,an-pointFFTalgorithmrequireslogç÷complex6122è6øNæNömultiplicationsandlogç÷complexadditions.Inaddition,weneed5´Ncomplex62è6ømultiplicationsand5´NcomplexadditionstocomputetheN-pointDFTX[k].Hence,forNNæNö=3072,theevaluationofX[k]using6(512)-pointFFTmodulesrequireslogç÷+5´N122è6øNæNö=256´log(512)+5´3072=17,664complexmultiplicationsandlogç÷+5´N262è6ø512´log(512)+5´3072=19,968complexadditions.2Itshouldbenotedthatadirectcomputationofthe3072-pointDFTwouldrequire9,437,184complexmultiplicationsand9,434,112complexadditions.8.40(a)#ofzero-valuedsamplestobeaddedis1024–1000=24.(b)Directcomputationofa1024-pointDFTofalength-1000sequencerequires2(1000)=1,000,000complexmultiplicationsand999´1000=999,000complexadditions.339
(c)A1024-pointCooley-TukeytypeFFTalgorithmrequires512´log(1024)=5,1202complexmultiplicationsand1024´log(1024)=10,240complexadditions.2-1-2-1-18.41(a)Y(z)=H(z)X(z)ory[0]+y[1]z+y[2]z=(h[0]+h[1]z)(x[0]+x[1]z).Now,Y(z)=Y(–1)=y[0]-y[1]+y[2]=H(-1)X(–1)=(h[0]-h[1])(x[0]-x[1]),0Y(z)=Y(¥)=y[0]=H(¥)X(¥)=h[0]x[0],1Y(z)=Y(1)=y[0]+y[1]+y[2]=H(1)X(1)=(h[0]+h[1])(x[0]+x[1]).2FromEqs.(3.158)and(3.159),wecanwriteI(z)I(z)I(z)012Y(z)=Y(z)+Y(z)+Y(z),I(z)0I(z)1I(z)2001122-1-1-1-1whereI(z)=(1-zz)(1-zz)=(1-zz)(1-z),0121z1=¥-1-1-1-1-2I(z)=(1-zz)(1-zz)=(1+z)(1-z)=1-z,102-1-1-1-1I(z)=(1-zz)(1-zz)=(1+z)(1-zz).2011z1=¥I0(z)1-1-1I1(z)-2I2(z)1-1-1Therefore,=-z(1-z),=(1-z),and=z(1+z).Hence,I(z)2I(z)I(z)20011221-1-1-21-1-1Y(z)=-z(1-z)Y(z)+(1-z)Y(z)+z(1+z)Y(z)20122æ11ö-1æ11ö-2=Y(z1)+çè-2Y(z0)+2Y(z2)÷øz+çè2Y(z0)-Y(z1)+2Y(z2)÷øzæ11ö-1=h[0]x[0]+ç-(h[0]-h[1])(x[0]-x[1])+(h[0]+h[1])(x[0]+x[1])÷zè22øæ11ö-2+ç(h[0]-h[1])(x[0]-x[1])-h[0]x[0]+(h[0]+h[1])(x[0]+x[1])÷zè22ø-1-2=h[0]x[0]+(h[0]x[1]+h[1]x[0])z+h[1]x[1]z.1Ignoringthemu,tiplicationsby,computationofthecoefficientsofY(z)requirethevaluesof2Y(z0),Y(z1),andY(z2)whichcanbeevaluatedusingonly3multiplications.(b)Y(z)=H(z)X(z)or-1-2-3-4-1-2-1-2y[0]+y[1]z+y[2]z+y[3]z+y[4]z=(h[0]+h[1]z+h[2]z)(x[0]+x[1]z+x[2]z).1Now,Y(z)=Y(-)=(h[0]-2h[1]+4h[2])(x[0]-2x[1]+4x[2]),02Y(z)=Y(-1)=(h[0]-h[1]+h[2])(x[0]-x[1]+x[2]),1Y(z)=Y(¥)=h[0]x[0],2Y(z)=Y(1)=(h[0]+h[1]+h[2])(x[0]+x[1]+x[2]),31Y(z)=Y()=(h[0]+2h[1]+4h[2])(x[0]+2x[1]+4x[2]).42FromEqs.(3.158)and(3.159),wecanwrite340
I(z)I(z)I(z)I(z)I(z)01234Y(z)=Y(z)+Y(z)+Y(z)+Y(z)+Y(z),I(z)0I(z)1I(z)2I(z)3I(z)40011223344-1-1-1-1-21-1-1whereI(z)=(1-zz)(1-zz)(1-zz)(1-zz)=(1-z)(1-z)(1-zz),0123422z=¥2-1-1-1-11-2-1-1I(z)=(1-zz)(1-zz)(1-zz)(1-zz)=(1-z)(1-z)(1-zz),1023442z=¥2-1-1-1-11-2-2I(z)=(1-zz)(1-zz)(1-zz)(1-zz)=(1-z)(1-z),201344-1-1-1-11-2-1-1I(z)=(1-zz)(1-zz)(1-zz)(1-zz)=(1-z)(1+z)(1-zz),3012442z=¥2-1-1-1-1-21-1-1I(z)=(1-zz)(1-zz)(1-zz)(1-zz)=(1-z)(1+z)(1-zz).4012322z=¥2I0(z)1-11-1-2I1(z)2-1-11-2Therefore,=z(1-z)(1-z),=–z(1-z)(1-z),I(z)122I(z)340011I2(z)-21-2I3(z)2-1-11-2=(1-z)(1-z),=z(1+z)(1-z),andI(z)4I(z)342233I4(z)1-11-1-2=–z(1+z)(1-z).I(z)122441-11-2-31-42-1-21-31-4Hence,Y(z)=(z-z-z+z)Y(z)-(z-z-z+z)Y(z)1222034412-1-21-31-41-11-2-31-4+(z+z-z-z)Y(z)-(z+z-z-z)Y(z)344312224æ1221ö-1=Y(z2)+çèY(z0)-Y(z1)+Y(z3)-Y(z4)÷øz123312æ12521ö-2+çè-Y(z0)+Y(z1)-Y(z2)+Y(z3)-Y(z4)÷øz2434324æ1111ö-3+çè-Y(z0)+Y(z1)-Y(z3)+Y(z4)÷øz126612æ11111ö-4+çèY(z0)-Y(z1)+Y(z2)-Y(z3)+Y(z4)÷øz.2464624SubstitutingtheexpressionsforY(z0),Y(z1),Y(z2),Y(z3),andY(z4),intheaboveequation,wethenariveattheexpressionsforthecoefficients{y[n]}intermsofthecoefficients{h[n]}and{x[n]}.Forexample,y[0]=Y(z2)=h[0]x[0].12y[1]=(Y(z)-Y(z))+(Y(z)-Y(z))=12043311=([(h[0]-2h[1]+4h[2])(x[0]-2x[1]+4x[2])]-[(h[0]+2h[1]+4h[2])(x[0]+2x[1]+4x[2])])122+([(h[0]+h[1]+h[2])(x[0]+x[1]+x[2])]-[(h[0]-h[1]+h[2])(x[0]-x[1]+x[2])])3=h[0]x[1]+h[1]x[0],=h[0]x[1]+h[1]x[0].Inasimilarmannerwecanshow,125y[2]=-(Y(z)+Y(z))+(Y(z)+Y(z))-Y(z)=h[0]x[2]+h[1]x[1]+h[2]x[0],24043134211y[3]=(Y(z)-Y(z))+(Y(z)-Y(z))=h[1]x[2]+h[2]x[1],and1240213341
111y[4]=(Y(z)+Y(z))-(Y(z)+Y(z))+Y(z)=h[2]x[2].240461342125111Hence,ignoringthemu,tiplicationsby,,,,,and,computationofthe12344624coefficientsofY(z)requirethevaluesofY(z0),Y(z1),Y(z2),Y(z3),andY(z4)whichcanbeevaluatedusingonly5multiplications.-1-2-1-18.42Y(z)=H(z)X(z)ory[0]+y[1]z+y[2]z=(h[0]+h[1]z)(x[0]+x[1]z)-1-2h[0]x[0]+(h[0]x[1]+h[1]x[0])z+h[1]x[1]z.Hence,y[0]=h[0]x[0],y[1]=h[0]x[1]+h[1]x[0],andy[2]=h[1]x[1].Now,(h[0]+h[1])(x[0]+x[1])-h[0]x[0]-h[1]x[1]=h[0]x[1]+h[1]x[0]=y[1].Asaresult,evaluationofH(z)X(z)requiresthecomputationof3products,h[0]x[0],h[1]x[1],and(h[0]+h[1])(x[0]+x[1]).Inaddition,itrequires4additions,h[0]+h[1],x[0]+x[1],and(h[0]+h[1])(x[0]+x[1])-h[0]x[0]-h[1]x[1].8.43Letthetwolength-Nsequencessbedenotedby{h[n]}and{x[n]}.Denotethesequence2N-1generatedbythelinearconvolutionofh[n]andx[n]asy[n],i.e.y[n]=åh[l]x[n–l].l=0Computationof{y[n]}thusrequires2Nmultiplications.LetH(z)andX(z)denotethez-N-1–nN-1–ntransformsof{h[n]}and{x[n]},i.e.H(z)=åh[n]z,andX(z)=åx[n]z.Rewriten=0n=0–N/2–N/2H(z)andX(z)intheformH(z)=H(z)+zH(z),andX(z)=X(z)+zX(z),where0101(N/2)-1–n(N/2)-1N–n(N/2)-1–nH0(z)=ån=0h[n]z,H1(z)=ån=0h[n+]z,X0(z)=ån=0x[n]z,and2(N/2)-1N–nX1(z)=ån=0x[n+]z.Therefore,wecanwrite2–N/2–N/2Y(z)=(H(z)+zH(z))(X(z)+zX(z))0101–N/2–N=H(z)X(z)+z(H(z)X(z)+H(z)X(z))+zH(z)X(z)00010111–N/2–N=Y(z)+zY(z)+zY(z),012whereY(z)=H(z)X(z),Y(z)=H(z)X(z)+H(z)X(z),Y(z)=H(z)X(z).000101102112NæNöNowY0(z)andY1(z)areproductsoftwopolynomialsofdegree,andhence,requireç÷2è2ømultiplicationseach.Now,wecanwriteY(z)=(H(z)+H(z))(X(z)+X(z))-Y(z)-Y(z).1010102NSince(H(z)+H(z))(X(z)+X(z))isaproductoftwopolynomialsofdegree,andhence,010122æNöitcanbecomputedusingç÷multiplications.Asaresult,Y(z)=H(z)X(z)canbecomputedè2ø2æNö2using3ç÷multiplicationsinsteadofNmultiplications.è2øNIfNisapower-of-2,iseven,andthesameprocedurecanbeappliedtocomputeY0(z),2Y1(z),andY2(z)reducingfurtherthenumberofmultiplications.Thisprocesscanbecontinueduntil,thesequencesbeingconvolvedareoflength1each.342
LetR(N)denotethetotalnumberofmultiplicationsrequiredtocomputethelinearconvolutionoftwolength-Nsequences.Then,inthemethodoutlinedabove,wehaveR(N)=3×R(N/2)withlogNR(1)=1.AsolutionofthisequationisgivenbyR(N)=32.(B-1)(B-1)8.44ThedynamicrangeofasignedB-bitintegerhisgivenby-(2-1)£h<(2-1)3131whichforB=32isgivenby-(2-1)£h<(2-1).(a)ForE=6andM=25,thevalueofa32-bitfloatingpointnumberisgivenbysE-3132h=(-1)2(M).Hence,thevalueofthelargestnumberis»2,andthevalueofthe3232smallestnumberis»-2.Thedynamicrangeistherefore»2´2.(b)ForE=7andM=24,thevalueofa32-bitfloatingpointnumberisgivenbysE-6364h=(-1)2(M).Hence,thevalueofthelargestnumberis»2,andthevalueofthe6464smallestnumberis»-2.Thedynamicrangeistherefore»2´2.(c)ForE=8andM=23,thevalueofa32-bitfloatingpointnumberisgivenbysE-127128h=(-1)2(M).Hence,thevalueofthelargestnumberis»2,andthevalueofthe128128smallestnumberis»-2.Thedynamicrangeistherefore»2´2.Hence,thedynamicrangeinafloating-pointrepresentationismuchlargerthanthatinafixed-pointrepresentationwiththesamewordlength.8.45A32-bitfloating-pointnumberintheIEEEFormathasE=8andM=23.AlsotheexponentEiscodedinabiasedformasE–127withcertainconventionsforspecialcasessuchasE=0,255,andM=0(Seetextpages540and541).Nowapositive32-bitfloatingpointnumberhrepresentedinthe"normalized"formhaveansE-127exponentintherange0wisdesiredalongwithalowpass-to-lowpasstransformation,wecancc((imposetheconditionH(wˆ)=H(w).Thisconditionismetifb=1+aand-1w.cc350
coswcoswˆ-1-2-3-4-50.052817+0.079708z+0.1295z+0.1295z+0.079708z+0.052817zM8.1H(z)=1-1.8107z-1+2.4947z-2-1.8801z-3+0.95374z-4-0.23359z-50.60.40.20-0.20102030Timeindexn-1-2-3-40.0083632-0.033453z+0.050179z-0.033453z+0.0083632zM8.2H(z)=1+2.3741z-1+2.7057z-2+1.5917z-3+0.41032z-40.40.20-0.2-0.40102030TimeindexnM8.3NumeratorcoefficientsColumns1through72.7107e-040-1.8975e-0305.6924e-030-9.4874e-03351
Columns8through1409.4874e-030-5.6924e-0301.8975e-030Column15-2.7107e-04DenominatorcoefficientsColumns1through51.0000e+001.7451e+004.9282e+006.1195e+009.8134e+00Columns6through109.2245e+001.0432e+017.5154e+006.4091e+003.4595e+00Columns11through152.2601e+008.4696e-014.1671e-018.5581e-022.9919e-020.30.20.10-0.1-0.20102030TimeindexnM8.4ModifiedProgram8_2isgivenbelow:n=0:60;w=input("Angularfrequencyvector=");num=input("Numeratorcoefficients=");den=input("Denominatorcoefficients=");w=input("Normalizedangularfrequencyvector=");x1=cos(w(1)*pi*n);x2=cos(w(2)*pi*n);x=x1+x2;subplot(2,1,1);stem(n,x);title("Inputsequence");xlabel("Timeindexn");ylabel("Amplitude");[N,Wn]=ellipord(0.4,0.5,0.5,40);[num,den]=ellip(N,0.5,40,Wn);y=filter(num,den,x);subplot(2,1,2);stem(n,y);title("Outputsequence");xlabel("Timeindexn");ylabel("Amplitude");TheplotsgeneratedbythisprogramforthefilterofExample7.19foraninputcomposedofasumoftwosinusoidalsequencesofangularfrequencies,0.3pand0.6p,aregivenbelow:352
InputsequenceOutputsequence2110.500-1-0.5-2-102040600204060TimeindexnTimeindexnTheblockingofthehigh-frequencysignalbythelowpassfiltercanbedemonstratedbyreplacingthestatementstem(k,x);withstem(k,x2);andthestatementy=filter(num,den,x)intheaboveprogramwiththefollowing:y=filter(num,den,x2).Theplotsoftheinputhigh-frequencysignalcomponentandthecorrespondingoutputareindicatedbelow:InputsequenceOutputsequence10.30.20.50.100-0.5-0.1-1-0.202040600204060TimeindexnTimeindexnM8.5TheplotsgeneratedbyusingthemodifiedprogramofProblemM8.4andusingthedataofthisproblemareshownbelow:InputsequenceOutputsequence21.5110.500-1-0.5-2-102040600204060TimeindexnTimeindexnTheblockingofthelow-frequencysignalbythehighpassfiltercanbedemonstratedbyreplacingthestatementstem(k,x);withstem(k,x1);andthestatementy=filter(num,den,x)intheaboveprogramwiththefollowing:y=filter(num,den,x1).Theplotsoftheinputhigh-frequencysignalcomponentandthecorrespondingoutputareindicatedbelow:353
InputsequenceOutputsequence10.40.50.200-0.5-0.2-1-0.402040600204060TimeindexnTimeindexnM8.6%Thefactorsforthetransferofexample7.16are%num1=[0.25460.25460]%den1=[1.0000-0.49090]%num2=[0.29820.18010.2982]%den2=[1.0000-0.76240.5390]%num3=[0.6957-0.06600.6957]%den3=[1.0000-0.55740.8828]N=input("Thetotalnumberofsections=");fork=1:N;num(k,:)=input("Thenumerator=");den(k,:)=input("Thedenominator=");endn=0:60;w=input("Normalizedangularfrequencyvector=");x1=cos(w(1)*pi*n);x2=cos(w(2)*pi*n);x=x1+x2;subplot(2,1,1);stem(n,x);xlabel("Timeindexn");ylabel("Amplitude");title("Inputsequence")si=[00];fork=1:Ny(k,:)=filter(num(k,:),den(k,:),x,si);x=y(k,:);endsubplot(2,1,2);stem(n,x);axis([050-44]);xlabel("Timeindexn");ylabel("Amplitude");title("Outputsequence")M8.7%Thefactorsforthehighpassfilterare%num1=[0.0495-0.10060.0511]%den1=[1.00001.31010.5151]%num2=[0.1688-0.33230.1636]%den2=[1.00001.06400.7966]M8.8Toapplythefunctiondirect2tofilterasumoftwosinusoidalsequences,wereplacethestatementy=filter(num,den,x,si)intheMATLABprogramgiveninthesolutionofProblemM8.4withthestatementy=direct2(num,den,x,si).Theplotsgeneratedbythemodifiedprogramforthedatagiveninthisproblemaregivenbelow:354
M8.9TheMATLABprogramthatcanbeusedtocomputeallDFTsamplesusingthefunctiongfftandthefunctionfftisasfollows:clearN=input("DesiredDFTlength=");x=input("Inputsequence=");forj=1:NY(j)=gfft(x,N,j-1);enddisp("DFTsamplescomputedusinggfftare");disp(Y);disp("DFTsamplescomputedusingfftare");X=fft(x,N);disp(X);Resultsobtainedforthecomputationoftwoinputsequences{x[n]}oflengths8,and12,respectrively,aregivenbelow:DesiredDFTlength=8Inputsequence=[12344321]FFTvaluescomputedusinggfftareColumns1through420.0000-5.8284+2.4142i0-0.1716+0.4142iColumns5through80-0.1716-0.4142i0-5.8284-2.4142iFFTvaluesusingfftareColumns1through420.0000-5.8284-2.4142i0-0.1716-0.4142iColumns5through80-0.1716+0.4142i0-5.8284+2.4142iDesiredDFTlength=12Inputsequence=[2481213579601]FFTvaluescomputedusinggfftareColumns1through458.0000-8.3301+5.5000i-12.5000+19.9186i-1.0000-7.0000iColumns5through88.5000-7.7942i0.3301+5.5000i-8.00000.3301-5.5000iColumns9through128.5000+7.7942i-1.0000+7.0000i-12.5000355
-19.9186i-8.3301-5.5000iFFTvaluesusingfftareColumns1through458.0000-8.3301-5.5000i-12.5000-19.9186i-1.0000+7.0000iColumns5through88.5000+7.7942i0.3301-5.5000i-8.0000+0.0000i0.3301+5.5000iColumns9through128.5000-7.7942i-1.0000-7.0000i-12.5000+19.9186i-8.3301+5.5000iM8.10TheMATLABprogramthatcanbeusedtoverifytheplotsofFigure8.37isgivenbelow:[z,p,k]=ellip(5,0.5,40,0.4);a=conv([1-p(1)],[1-p(2)]);b=[1-p(5)];c=conv([1-p(3)],[1-p(4)]);w=0:pi/255:pi;alpha=0;an1=a(2)+(a(2)*a(2)-2*(1+a(3)))*alpha;an2=a(3)+(a(3)-1)*a(2)*alpha;g=b(2)-(1-b(2)*b(2))*alpha;cn1=c(2)+(c(2)*c(2)-2*(1+c(3)))*alpha;cn2=c(3)+(c(3)-1)*c(2)*alpha;a=[1an1an2];b=[1g];c=[1cn1cn2];h1=freqz(fliplr(a),a,w);h2=freqz(fliplr(b),b,w);h3=freqz(fliplr(c),c,w);ha=0.5*(h1.*h2+h3);ma=20*log10(abs(ha));alpha=0.1;an1=a(2)+(a(2)*a(2)-2*(1+a(3)))*alpha;an2=a(3)+(a(3)-1)*a(2)*alpha;g=b(2)-(1-b(2)*b(2))*alpha;cn1=c(2)+(c(2)*c(2)-2*(1+c(3)))*alpha;cn2=c(3)+(c(3)-1)*c(2)*alpha;a=[1an1an2];b=[1g];c=[1cn1cn2];h1=freqz(fliplr(a),a,w);h2=freqz(fliplr(b),b,w);h3=freqz(fliplr(c),c,w);hb=0.5*(h1.*h2+h3);mb=20*log10(abs(hb));alpha=-0.25;an1=a(2)+(a(2)*a(2)-2*(1+a(3)))*alpha;an2=a(3)+(a(3)-1)*a(2)*alpha;g=b(2)-(1-b(2)*b(2))*alpha;cn1=c(2)+(c(2)*c(2)-2*(1+c(3)))*alpha;cn2=c(3)+(c(3)-1)*c(2)*alpha;a=[1an1an2];b=[1g];c=[1cn1cn2];h1=freqz(fliplr(a),a,w);h2=freqz(fliplr(b),b,w);h3=freqz(fliplr(c),c,w);hc=0.5*(h1.*h2+h3);mc=20*log10(abs(hc));plot(w/pi,ma,"r-",w/pi,mb,"b--",w/pi,mc,"g-.");axis([01-805]);xlabel("Normalizedfrequency");ylabel("Gain,dB");legend("b--","alpha=0.1","w","","r-","alpha=0","w","","g-.","alpha=–0.25");M8.11TheMATLABprogramthatcanbeusedtoverifytheplotsofFigure8.39isgivenbelow:w=0:pi/255:pi;356
wc2=0.31*pi;f=[00.360.461];m=[1100];b1=remez(50,f,m);h1=freqz(b1,1,w);m1=20*log10(abs(h1));n=-25:-1;c=b1(1:25)./sin(0.41*pi*n);d=c.*sin(wc2*n);q=(b1(26)*wc2)/(0.4*pi);b2=[dqfliplr(d)];h2=freqz(b2,1,w);m2=20*log10(abs(h2));wc3=0.51*pi;d=c.*sin(wc3*n);q=(b1(26)*wc3)/(0.4*pi);b3=[dqfliplr(d)];h3=freqz(b3,1,w);m3=20*log10(abs(h3));plot(w/pi,m1,"r-",w/pi,m2,"b--",w/pi,m3,"g-.");axis([01-805]);xlabel("Normalizedfrequency");ylabel("Gain,dB");legend("b--","wc=0.31p","w","","r-","wc=0.41p","w","","g-.","wc=0.51p")M8.12TheMATLABprogramtoevaluateEq.(8.104)isgivenbelow:x=0:0.001:0.5;y=3.140625*x+0.0202636*x.^2-5.325196*x.^3+0.5446778*x.^4+1.800293*x.^5;x1=pi*x;z=sin(x1);plot(x,y);xlabel("Normalizedangle,radians");ylabel("Amplitude");title("Approximatesinevalues");grid;axis([00.501]);pauseplot(x,y-z);xlabel("Normalizedangle,radians");ylabel("Amplitude");title("Errorofapproximation");grid;Theplotsgeneratedbytheaboveprogramareasindicatedbelow:M8.13TheMATLABprogramtoevaluateEq.(8.110)isgivenbelow:k=1;357
forx=0:.01:1op1=0.318253*x+0.00331*x^2-0.130908*x^3+0.068524*x^4-0.009159*x^5;op2=0.999866*x-0.3302995*x^3+0.180141*x^5-0.085133*x^7+0.0208351*x^9;arctan1(k)=op1*180/pi;arctan2(k)=180*op2/pi;actual(k)=atan(x)*180/pi;k=k+1;endsubplot(211)x=0:.01:1;plot(x,arctan2);ylabel("Angle,degrees");xlabel("TangentValues");subplot(212)plot(x,actual-arctan2,"--");ylabel("TangentValues");xlabel("error,radians");Theplotsgeneratedbytheaboveprogramareasindicatedbelow:Note:Expansionforarctan(x)givenbyEq.(8.110)givestheresultinnormalizedradians,i.e.theactualvalueinradiansdividedbyp.358
Chapter9(2e)9.1Two"sComplementTruncation-Assumex>0.Therelativeerroretisgivenbybbb-i-i-iåa-i2-åa-i2-åa-i2Q(x)-xQ(M)-Mi=1i=1i=b+1et====.xMMMNowetwillbeaminimumifalla-i"sare1andwillbeamaximumifalla-i"sare0ford0b+1£i£b,Thus–£et£.Since0.5£M£1hence-2d£et£0.MMNowconsiderx<0.Here,therelativeerroretisgivenbybb-i-i-1+åa-i2+1-åa-i2Q(x)-xQ(M)-Mi=1i=1d0et===.Asbefore,–£et£.InxMMMMthiscase–10.Therelativeerroretisgivenbybbb-i-i-iåa-i2-åa-i2-åa-i2Q(x)-xQ(M)-Mi=1i=1i=b+1et====.xMMMNowetwillbeaminimumifalla-i"sare1andwillbeamaximumifalla-i"sare0ford0b+1£i£b,Thus–£et£.Since0.5£M£1hence-2d£et£0.MMNowconsiderx<0.Here,therelativeerroretisgivenbybb-b-i-b-i-(1-2)+åa-i2+(1-2)-åa-i2Q(x)-xQ(M)-Mi=1i=1et===xMMb-b-b-i(2-2)+åa-i2i=b+1=.MNowetwillbeamaximumifalla-i"sare0andwillbeaminimumifalla-i"sare1,Inthiscase,0d0.Here,bbb-i-i-i–åa-i2+åa-i2åa-i2Q(M)-Mi=1i=1i=b+1et===.Since,–1£M£–0.5,hence,MMM–2d£et<0.359
-dd-ddRounding-e=Q(M)–M.Hence£e£.Thisimplies£er£.222M2MSince–10.ë2N+1ûë2N+1ûHence0>e,G@=.4e(e2+q2)4eq24e2221+r11+(1-e)1Also,H=×=×1-r2r4-2r2cos2q+11-(1-e)2(1-e)4-2(1-e)2(1-2q2)+111»».4e(q2+e2)4eq2Nˆ4N4Thus,@q.Asaresult,witherrorfeedback,theratiogetsmultipliedbyq.NS-2b22-2b2qNq2(a)inputwithuniformdensity:££16e2Sp2e2-2b22-2b23qN3q2(b)Wide-sensestationary,Gaussiandensity,white:££16e2Sp2e2-2b2N2q(c)sinusoidwithknownfrequency:=S24e9.37Thecoupledformwitherrorfeedbackisshownbelow:-1ze1[n]l–1gv1[n]+Qy[n]-1dzz-1au[n]v2[n]+Qb–l2e2[n]-1z-1-1-1AnalysisyieldsV1(z)=l1zE1(z)+dzY(z)+gzU(z),E1(z)=Y(z)-V1(z),-1-1-1V2(z)=l2zE2(z)+bzY(z)+azU(z),andE2(z)=Y(z)-V2(z).EliminatingU1(z),V1(z),U2(z),andV2(z)fromtheseequationswearriveatthenoisetransferfunctions-1-1Y(z)(1-az)(1+l1z)G1(z)==-1-2,andE1(z)E1-(a+d)z+(ad-bg)z2(z)=0-1-1Y(z)gz(1+l2z)G2(z)==-1-2.ThetotaloutputnoiseE2(z)E1-(a+d)z+(ad-bg)z1(z)=0399
22222poweristhusgivenbyso=G12se1+G22se2.Hence,theoutputnoisepower,foralowpassfilterdesign,canbereducedbyplacingzerosofthenoisetransferfunctionsinthepassband.Foreachofthenoisetransferfunctionsgivenabove,wecanonlyplaceazeroatz=1bychoosingli=-1,i=1,2,Usingthenotationsb=-(a+d)andd=ad-bgwerewritethenoisetransferfunctionsas2z-(1+a)z+a-(1+a+b)+(a-d)gz-gG1(z)=2=1+2,andG2(z)=2.UsingTablez+bz+dz+bz+dz+bz+d2222[(1+a+b)+(a-d)](1-d)+2(1+a+b)(a-d)(1-d)9.4weobtainG12=1+22222,and(1-d)+2db-(1+d)b2222g(1-d)G22=22222.(1-d)+2db-(1+d)b9.38TheKingsburystructurewitherrorfeedbackisshownbelow:e1[n]e2[n]e3[n]-1-1-1zzzl1–l2–l3–v1[n]+u1[n]v2[n]+-k1v3[n]+y[n]z-1QQQu2[n]u3[n]k1-1k2z-1-1Analysisyields:V1(z)=k1Y(z)+zU1(z)+l1zE1(z),E1(z)=U1(z)-V1(z),-1V2(z)=k2Y(z)+U1(z)+l2zE2(z),E2(z)=U2(z)-V2(z),-1-1V3(z)=Y(z)-k1U2(z)+l3zE3(z),E3(z)=U3(z)-V3(z),andY(z)=zU1(z).EliminatingU1(z),V1(z),U2(z),V2(z),U3(z)andV3(z)fromtheseequationsweget-1-1Y(z)-k1z(1+l1z)G1(z)==-1-2,E1(z)E2(z)=E3(z)=01-[2-k1(k1+k2)]z+(1-k1k2)z-1-1-1Y(z)-k1z(1-z)(1+l2z)G2(z)==-1-2,andE2(z)E1(z)=E3(z)=01-[2-k1(k1+k2)]z+(1-k1k2)z-1-1-1Y(z)z(1-z)(1+l3z)G3(z)==-1-2.ThetotaloutputnoiseE3(z)E1(z)=E2(z)=01-[2-k1(k1+k2)]z+(1-k1k2)z2222222poweristhusgivenbyso=G12se1+G22se2+G32se3.Hence,theoutputnoisepower,foralowpassfilterdesign,canbereducedbyplacingzerosofthenoisetransferfunctionsinthepassband.Foreachofthenoisetransferfunctionsgivenabove,wecanonlyplaceazeroatz=1bychoosingli=-1,i=1,2,3,inwhichcase,usingthenotationsb=-[2-k1(k1+k2)],-1-1-k1z(1-z)-k1z+k1andd=1-k1k2.thenoisetransferfunctionsreducetoG1(z)=-1-2=2,1+bz+dzz+bz+d400
-1-12-k1z(1-z)-k1(z-2z+1)-k1A-k1(Cz+D)G2(z)=-1-2=2=z+2and1+bz+dzz(z+bz+d)z+bz+d-1-122z(1-z)z-2z+1ACz+D11G3(z)=-1-2=2=z+2whereA=d,C=1-d,and1+bz+dzz(z+bz+d)z+bz+d2æbö222(1-d)-2(1-d)bD=-çè2+÷ø.UsingTable9_4wethenobtainG12=k122222,d(1-d)+2db-(1+d)b22222(C+D)(1-d)-2CD(1-d)b222G32=A+2BC+22222,andG22=k1G32.(1-d)+2db-(1+d)b9.39Thetransferfunctionofthecoupled-formstructureshownbelowisgivenbyEq.(9.42)wherea=d=rcos(q)andb=-g=rsin(q).Analysisyieldsgs2[n+1]x[n]y[n]–1d–1zazs1[n+1]bs[n+1]=a(x[n]+s[n])+bs[n]ands[n+1]=g(x[n]+s[n])+ds[n].Rewritingthese112212és1[n+1]ùéabùés1[n]ùéaùequationsinmatrixformwegetêës[n+1]úû=êëgdúûêës[n]úû+êëgúûx[n]22éabùéagùéabùéa2+g2ab+gdùér20ùThusA=êú.Therefore,ATA=êúêú=ê22ú=ê2ú.ëgdûëbdûëgdûêëab+gdb+dúûë0rûéabùéagùéa2+b2ag+bdùér20ùLikewise,AAT=êúêú=ê22ú=ê2ú.ëgdûëbdûêëag+bdg+dúûë0rûThusAisofnormalformandhence,thestructurewillnotsupportlimitcycles.9.40Thetransferfunctionofthemodifiedcoupled-formstructureshownbelowisgivenbys[n+1]cs[n+1]c12x[n]z–1z–1y[n]dd–1s[n+1]=cds[n]-cs[n]+x[n],s[n+1]=cs[n]+cds[n],andy[n]=cs[n].1122122Inmatrixformtheseequationscanbewrittenasés1[n+1]ùécd-cùés1[n]ùé1ùés1[n]ùêës2[n+1]úû=êëccdúûêës2[n]úû+êë0úûx[n],andy[n]=[0c]êës2[n]úû+[0]x[n].Thus,401
écd-cùA=.Now,inthez-domaintheequationsdescribingthestructureareëêccdûúzS(z)=cdS(z)-cS(z)+X(z),zS(z)=cS(z)+cdS(z),andY(z)=cS(z).Fromthe1122122equationontheleftweget(z-cd)S(z)=-cS(z)+X(z)andfromtheequationontherightwe12cX(z)get(z-cd)S(z)=cS(z).EliminatingS(z)wearriveatS(z)=.Hence2112z2-2cdz+c2d2+c22Y(z)cH(z)==.X(z)z2-2cdz+c2(1+d2)22ComparingdenominatorofH(z)withthedenominatorz-2rcosqz+rofasecondorderécd-cùércosq-rsinqùtransferfunctionwegetc=rsinq,d=cotq.ThenA=êccdú=êrsinqrcosqú.ëûëûér20ùér20ùThusATA=ê2ú,andsimilarlyAAT=ê2ú.Sinceforstabilityr<1,ë0rûë0rûAisnormalformmatrix,andthusthestructuredoesnotsupportlimitcycles.9.41Ablock-diagramrepresentationofadigitalfilterstructurebasedonthestate-spacedescriptiongivenbyEqs.(9.200)and(9.201)isasshownbelow.DBs[n+1]x[n]-1zIACy[n]s[n]Thefeedbackloopunderzero-inputconditionsisthusasindicatedbelow:Qs[n+1]v[n+1]z-1IAs[n]Hence,underzero-inputconditionswehavev[n+1]=As[n],s[n+1]=Q(v[n+1]).Thequadraticfunctionf(s[n])=sT[n]Ds[n],whereDisapositive-definitediagonalmatrix,isrelatedtothepowerstoredinthedelays.Thechangesinthisquantitycanprovideinformationregardingoscillationsunderzero-inputconditions:TTDf(s[n])=f(s[n+1])-f(s[n])=-s[n]×D×s[n]+s[n+1]×D×s[n+1]TTTT=-s[n]×D×s[n]+s[n+1]×D×s[n+1]+v[n+1]×D×v[n+1]-v[n+1]×D×v[n+1]402
NTTT22=-s[n]×D×s[n]+s[n+1]×A×D×A×s[n+1]-åk=1(vk[n+1]-Q(vk[n+1]))dkkNTT22=-s[n](D-A×D×A)s[n]-åk=1(vk[n+1]-Q(vk[n+1]))dkk.TTNowifs[n](D-ADA)s[n]³0,andvk[n]arequantizedsuchthatQ(v[n+1])£v[n+1],kk(i.e.usingapassivequantizer),thenthepowerstoredinthedelayswillnotincreasewithincreasingn.Sounderpassivequantization,limitcycleswillbeeliminatedifTTTs[n](D-ADA)s[n]³0,orD-ADAispositive-definite.ForsecondorderstableIIRfilters,eigenvaluesofA=[aij]arelessthan1.Thisconditionissatisfiedifaa³0,1221or,aa<0anda-a+det(A)£1.12211122Forthegivenstructure,éaa+1ùéa-bùA=ê11ú,B=ê11ú,C=[11],andD=1.ëa2-1a2ûëa2-b2ûThetransferfunctionofthefilterisgivenbyz2-(b+b)z+(1+b-b)1212H(z)=.z2-(a+a)z+(1+a-a)1212Thefilterisstableif1+a-a<1,anda+a<1+(1+a-a),orequivalently,if121212a-a<0,anda+a<2+a-a.121212122Now,a1a2=(a1+1)(a2-1)=4[(a1+a2)-(2+a1-a2)],andsince,22(a+a)<(2+a-a),a12a21<0.1212Next,a-a+det(A)=a-a+aa-(a+1)(a-1)1122121212==-(a-a)+aa-(aa+a-a-1)=1.12121221Hence,thestructuredoesnotsupportzero-inputlimitcycles.9.42FromSection8.3.1weknowthateachcomputationofaDFTsamplerequires2N+4realmultiplications.Assumingthatquantizationnoisegeneratedfromeachmultiplierisindependent-2b222(N+2)ofthenoisegeneratedfromothermultipliers,wegets=(2N+4)s=.r062b2b229.43SNR=.Hence,anSNRof25dBimplies=102.5orN2N212.52b=2log2((10)´(512))=13.1524.Thereforeb=14bitsshouldbechosentogetanSNRof25dB.Thereforenumberofbitsrequiredforeachsample=14+1=15.9.44LetN=2n.Considerthemthstage.Theoutputsees4(2n-m)noisesourcesfromthemthstage.n-m11Eachnoisesourcehasavariancereductionbyafactorof()duetomultiplicationbyat42eachstagetilltheoutput.Hencethetotalnoisevarianceattheoutputduetothenoisesinjectedinthemthstageis4(2n-m)-2(n-m)2.(2)s0403
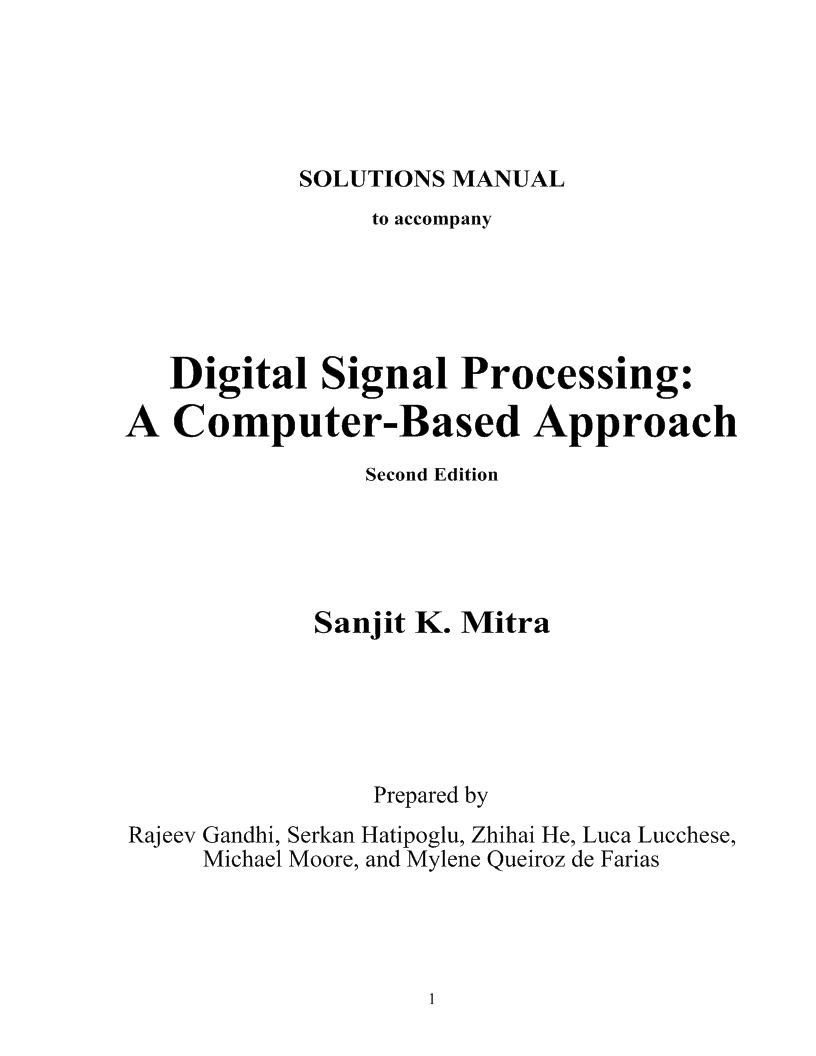
n2n-m-2(n-m)2Thereforethetotalnoisevarianceattheoutput=s=å4(2)(2)s0m=1n-2bn2-nm2-n2(2-1)2-2b-n2-2b4s02å2=42=32(1-2)»32forlargeN.122-1m=12b212.59.45SNR=.Henceb=log((10)´2´512)=8.652.2N22Hencewechooseb+1=10bitspersampletogetanSNRof25dB.M9.1%Thisisthefunctiontoobtainthepoledistribution%plotofasecondordertransferfunctionwithadenominator%oftheformZ*Z-KZ+L.Forstability00)if(k1*k1<4-2*k1*k2)c=1-k1*k2;b=-(2-k1*k2-k1*k1);p1=(-b+(sqrt(b*b-4*c)))/2;p2=(-b-(sqrt(b*b-4*c)))/2;if(imag(p1)~=0)if(real(p1)~=0)if(abs(p1)<1)plot(p1,".");endendendif(imag(p2)~=0)if(real(p2)~=0)if(abs(p2)<1)plot(p2,".");endendendendendendendendhold;Runningthisprogramusingthestatementpole_plot_kb(6)yieldsthefollowingplot.10.50-0.5-1-1-0.500.51Realpart406
M9.30-20-40-60unquantizedquantized-8000.20.40.60.81w/pM9.4N=5;wn=0.45;Rp=0.5;Rs=45;[B,A]=ellip(N,Rp,Rs,wn);zplane(B,A);z=cplxpair(roots(B));p=cplxpair(roots(A));disp("Factorsforthenumerator");const=B(1)/A(1);k=1;whilek<=length(z),if(imag(z(k))~=0)factor=[1-2*real(z(k))abs(z(k))^2]k=k+2;elsefactor=[1-z(k)]k=k+1;endenddisp("Factorsforthedenominator");k=1;whilek<=length(p),if(imag(p(k))~=0)factor=[1-2*real(p(k))abs(p(k))^2]k=k+2;elsefactor=[1-p(k)]k=k+1;endendsos=zp2sos(z,p,const)TheaboveprogramyieldsFactorsforthenumeratorfactor=1.0000e+001.0358e+001.0000e+00factor=1.0000e+003.7748e-011.0000e+00factor=1.0000e+001.0000e+00407
Factorsforthedenominatorfactor=1.0000e+00-2.6433e-018.6522e-01factor=1.0000e+00-5.8090e-015.0030e-01factor=1.0000e+00-4.4817e-01æ1+1.0358z-1+z-2öæ1+0.37748z-1+z-2öæ1+z-1öHenceH(z)=ç÷ç÷ç÷.çè1-0.26433z-1+0.86522z-2÷øçè1-0.5809z-1+0.5003z-2÷øçè1-0.44817z-1÷øNotethattheorderinghasnoeffectifL-scalingisused.¥111220.5330-0.5221-11-1-0.500.51Realpartsos=2.7591e-012.7591e-0101.0000e+00-4.4817e-0103.0285e-013.1370e-013.0285e-011.0000e+00-5.8090e-015.0030e-016.7335e-012.5418e-016.7335e-011.0000e+00-2.6433e-018.6522e-01M9.5[B,A]=ellip(5,0.5,45,0.45);p=roots(A);lenp=length(p);[Y,I]=sort(angle(p));fork=1:lenpif(rem(k,2)==1)p1((k+1)/2)=p(I(k));elsep2(k/2)=p(I(k));endendb1=poly(p1);b2=poly(p2);a1=fliplr(b1);a2=fliplr(b2);B1=0.5*(conv(b2,a1)-conv(b1,a2));A1=conv(b1,b2);[H,W]=freqz(B,A,512);[H1,W]=freqz(B1,A1,512);plot(W/pi,abs(H),"-",W/pi,abs(H1),"--");axis([0101.2]);xlabel("omega/pi");ylabel("Magnitude");408
pausea1=1.01*a1;a2=1.01*a2;b1=[b1(1)1.01*b1(2:length(b1))];b2=[b2(1)1.01*b2(2:length(b2))];A3=conv(b1,b2);B3=0.5*conv(a1,b2)+0.5*conv(a2,b1);B4=1.01*B;A4=[A(1)1.01*A(2:length(B))];[H2,W]=freqz(B3,A3,512);[H3,W]=freqz(B4,A4,512);plot(W/pi,abs(H2),"-",W/pi,abs(H3),"--");axis([0101.2]);xlabel("omega/pi");ylabel("Magnitude");110.80.80.60.60.40.40.20.20000.20.40.60.8100.20.40.60.81w/pw/pM9.6num1=input("Thefirstfactorofnumerator=");num2=input("Thesecondfactorofnumerator=");den1=input("Thefirstfactorofdenominator=");den2=input("Thesecondfactorofdenominator=");%Thenumeratoranddenominatorofthescaling%functionsf1andf2aref1num=1;f1den=[den1];f2num=num1;f2den=conv(den1,den2);f3num=conv(num1,num2);f3den=conv(den1,den2);x=[1zeros([1,511])];%Sufficientlengthforimpulseresponse%tohavedecayedtonearlyzerof1=filter(f1num,f1den,x);f2=filter(f2num,f2den,x);f3=filter(f3num,f3den,x);k1=sqrt(sum(f1.*f1));k2=sqrt(sum(f2.*f2));k3=sqrt(sum(f3.*f3));disp("Thefirstscalingfactor=");disp(k1);disp("Thesecondscalingfactor=");disp(k2);disp("Thethirdscalingfactor=");disp(k3);%Thenoisetransferfunctionsg1num=conv(num1,num2)/(k2*k3);g1den=conv(den1,den2)/k3;g2num=num2;g2den=den2;g1=filter(g1num,g1den,x);g2=filter(g2num,g2den,x);var=sum(f1.*f1)*3+sum(g2.*g2)*5+3;disp("Thenormalizednoisevariance");disp(var);%num1andnum2canbeinterchangedtocomeupwiththe%secondrealizationM9.7TheparallelformIstructureandtheparallelformIIstructureusedforsimulationareshownbelow:409
k4k4kk–0.3445k001k5-1-1–0.0188z1.6592kkz15–0.01881.6528k1z-1-1z–0.801–0.8010.2759kk1k–2.29k223k6-1-1–0.777z–5.162k3kz6–0.777–6.9422k-1-13–0.3434z–0.3434z0.7867k3ParallelFormIParallelFormIInum1=input("Thefirstfactorinthenumerator=");num2=input("Thesecondfactorinthenumerator=");den1=input("Thefirstfactorinthedenominator=");den2=input("Thesecondfactorinthedenominator=");num=conv(num1,num2);den=conv(den1,den2);[r1,p1,k11]=residuez(num,den);[r2,p2,k21]=residue(num,den);%SimulationofstructureforEq.9.244R1=[r1(1)r1(2)];P1=[p1(1)p1(2)];R2=[r1(3)r1(4)];P2=[p1(3)p1(4)];R3=[r2(1)r2(2)];P3=[p2(1)p2(2)];R4=[r2(3)r2(4)];P4=[p2(3)p2(4)];[num11,den11]=residuez(R1,P1,0);[num12,den12]=residuez(R2,P2,0);[num21,den21]=residue(R3,P3,0);[num22,den22]=residue(R4,P4,0);disp("ThenumeratorsforParallelFormI");disp(k11);disp(num11);disp(num12);disp("ThedenominatorsforParallelFormI");disp(den11);disp(den12);disp("ThenumeratorsforParallelFormII");disp(k21);disp(num21);disp(num22);disp("ThedenominatorsforParallelFormII");disp(den21);disp(den22);imp=[1zeros([1,2000])];y0=filter([100],den11,imp);y1=filter(num11,den11,imp);y2=filter([100],den12,imp);y3=filter(num12,den12,imp);gamma0=sum(y0.*conj(y0));gamma1=sum(y1.*conj(y1));gamma2=sum(y2.*conj(y2));gamma3=sum(y3.*conj(y3));k0=sqrt(1/gamma0);k1=sqrt(gamma0/gamma1);k2=sqrt(1/gamma2);k3=sqrt(gamma2/gamma3);y=filter(num,den,imp);gamma=sum(y.*conj(y));410
k4=sqrt(1/gamma);k5=k4/(k0*k1);k6=k4/(k2*k3);disp("ForparallelformI");disp("Thescalingconstantsare");disp(k0);disp(k1);disp(k2);disp(k3);disp(k4);disp(k5);disp(k6);disp("Theproductroundoffnoisevariance");noise=3*(k5/k0)^2+3*(k6/k2)^2+2*k5^2+2*k6^2+3;disp(noise);%%%%%%%%%%ForParallelFromII%%%%%%%%%%%%%%%%y0=filter([001],den21,imp);y1=filter(fliplr(num21),den21,imp);y2=filter([001],den22,imp);y3=filter(fliplr(num22),den22,imp);gamma0=sum(y0.*conj(y0));gamma1=sum(y1.*conj(y1));gamma2=sum(y2.*conj(y2));gamma3=sum(y3.*conj(y3));k0=sqrt(1/gamma0);k1=sqrt(gamma0/gamma1);k2=sqrt(1/gamma2);k3=sqrt(gamma2/gamma3);y=filter(num,den,imp);gamma=sum(y.*conj(y));k4=sqrt(1/gamma);k5=k4/(k0*k1);k6=k4/(k2*k3);disp("ForparallelformII");disp("Thescalingconstantsare");disp(k0);disp(k1);disp(k2);disp(k3);disp(k4);disp(k5);disp(k6);disp("Theproductroundoffnoisevariance");noise=3*(k5/k0)^2+3*(k6/k2)^2+2*k5^2+2*k6^2+3;disp(noise);M9.8ThescaledGray-Markelcascadedlatticestructureusedforsimulationisshownbelow:k4W4k3W3k2W2k1W1-d4-d3"-d2"-d1"""d4d3"d2"d1"""1/k1/k31/k21/k14–1–1–1–1zzzzaCa2Ca3Ca4Ca5C1kkkk2k3k4k1k2k3k4k1k2k3k4434%UseProgram_6_3.mpg.384togeneratethelattice%parametersandfeedforwardmultipliersd=[0.191491893489200.759534173651300.443489798462640.27506340000000];alpha=[1.00000000000000-3.502770000000004.61511974525466-1.70693992124303-0.90009664306164];imp=[1zeros([1,499])];qold1=0;fork=1:500w1=imp(k)-d(1)*qold1;y1(k)=w1;qnew1=w1;qold1=qnew1;endk1=sqrt(1/(sum(y1.*conj(y1))));imp=[1zeros([1,499])];qold1=0;qold2=0;fork=1:500w2=imp(k)-d(2)*qold2*1/k1;411
w1=k1*w2-d(1)*qold1;y1(k)=w1;y2(k)=w2;qnew1=w1;qnew2=w1*d(1)+qold1;qold1=qnew1;qold2=qnew2;endk2=sqrt(1/(sum(y2.*conj(y2))));qold1=0;qold2=0;qold3=0;fork=1:500w3=imp(k)-d(3)*qold3*1/k2;w2=k2*w3-d(2)*qold2*1/k1;w1=k1*w2-d(1)*qold1;y3(k)=w3;qnew1=w1;qnew2=w1*d(1)+qold1;qnew3=w2*d(2)+qold2*1/k1;qold1=qnew1;qold2=qnew2;qold3=qnew3;endk3=sqrt(1/sum(y3.*conj(y3)));qold1=0;qold2=0;qold3=0;qold4=0;fork=1:500w4=imp(k)-d(4)*qold4/k3;w3=k3*w4-d(3)*qold3/k2;w2=k2*w3-d(2)*qold2/k1;w1=k1*w2-d(1)*qold1;y4(k)=w4;qnew1=w1;qnew2=w1*d(1)+qold1;qnew3=w2*d(2)+qold2*1/k1;qnew4=w3*d(3)+qold3*1/k2;qold1=qnew1;qold2=qnew2;qold3=qnew3;qold4=qnew4;endk4=sqrt(1/sum(y4.*conj(y4)));const=0.135127668%Obtainedbyscalingtheo/poftheactualTFdisp("Thescalingparametersare");disp(k1);disp(k2);disp(k3);disp(k4);alpha(5)=alpha(5)/(k1*k2*k3*k4);alpha(4)=alpha(4)/(k1*k2*k3*k4);alpha(3)=alpha(3)/(k2*k3*k4);alpha(2)=alpha(2)/(k3*k4);alpha(1)=alpha(1)/k4;alpha=const*alpha;%%%%Computationofnoisevariance%%%%%%%Noisevarianceduetok4andd4=1/k4^2=1.08185285036642%Tocomputenoisevarianceduetok3,d3"=1.33858225imp=[1zeros([1,499])];fork=1:500w4=-d(4)*qold4/k3;w3=k3*w4-d(3)*qold3/k2;w2=k2*w3-d(2)*qold2/k1;w1=k1*w2-d(1)*qold1;qnew1=w1;qnew2=w1*d(1)+qold1;qnew3=w2*d(2)+qold2*1/k1;qnew4=w3*d(3)+qold3*1/k2;y11=w4*d(4)+qold4/k3;412
y0(k)=alpha(1)*y11+alpha(2)*qnew4+alpha(3)*qnew3+alpha(4)*qnew2+alpha(5)*qnew1;qold1=qnew1;qold2=qnew2;qold3=qnew3;qold4=qnew4;endnv=sum(y0.*conj(y0));%fork2,-d2""nv=3.131899935%fork1,-d1"""nv=1.00880596097028%ford1"""nv=0.95806646140013%ford2""nv=2.61615077290574%ford3"nv=0.41493478856386%ford4nv=0.01975407768839%for1/k1nv=0.75359663926391%for1/k2nv=0.58314964498424%for1/k3nv=0.09095345118133%Totalnv=23.55888782866100M9.9a=0.5a=0.50.120.70.60.10.50.080.40.060.30.040.20.020.1000510152005101520TimeindexnTimeindexna=0.5a=0.5120.5100.480.360.240.12000510152005101520TimeindexnTimeindexnInallthreecases,theconditionofEq.(9.186)issatisfiedandhence,thestructureexhibitszero-inputgranularlimitcycles.M9.10413
a=-0.875a=0.875120.40.30.20.10-0.1-0.2-0.3-0.4010203040Timeindexn414
Chapter10(2e)10.1Foraninputx1[n]andx2[n],theoutputsofthefactor-of-Lup-samplerare,respectively,givenbyìx[n/L],n=0,±L,±2L,Kìx[n/L],n=0,±L,±2L,Kx[n]=í1andx[n]=í2u1î0,otherwise,u2î0,otherwise.Letx2[n]=x1[n–no],wherenoisaninteger.Thenx2[n/L]=x1[(n/L)–no].Hence,ìx[(n/L)–n],n=0,±L,±2L,Kx[n]=í1ou2î0,otherwise.ìx[(n–n)/L],n–n=0,±L,±2L,KButx[n–n]=í1ooSincex[n]¹x[n–n],theup-u1oî0,otherwise,u2u1osamplerisatime-varyingsystem.10.2Considerfirsttheup-sampler.Letx1[n]andx2[n]betheinputswithcorrespondingoutputsìx[n/L],n=0,±L,±2L,Kgivenbyy1[n]andy2[n].Now,y[n]=í1and1î0,otherwise,ìx[n/L],n=0,±L,±2L,Ky[n]=í2Letusnowapplytheinputx[n]=ax[n]+bx[n],2î0,otherwise.312withthecorrespondingoutputgivenbyy3[n],whereìax[n/L]+bx[n/L],n=0,±L,±2L,Ky[n]=í123î0,otherwise,ìax[n/L]ìbx[n/L]n=0,±L,±2L,K=í1+í2=ay[n]+by[n].Thus,theup-samplerisaî0î0otherwise,12linearsystem.Now,considerthedown-sampler.Letx1[n]andx2[n]betheinputswithcorrespondingoutputsgivenbyy1[n]andy2[n].Now,y1[n]=x1[Mn],andy2[n]=x2[Mn].Letusnowapplytheinputx3[n]=ax1[n]+bx2[n],withthecorrespondingoutputgivenbyy3[n],wherey[n]=x[Mn]=ax[Mn]+bx[Mn].Thus,thedown-samplerisalinearsystem.331210.3–1/2–1/211/211/2z1/2z1/2Fromthefigure,V(z)=X(z)+X(–z),W(z)=X(z)–X(–z),2222–1–111zzV(z)=X(z)+X(–z),W(z)=X(z)–X(–z).Hence,u22u22–1–1Y(z)=zV(z)+W(z)=zX(z),orinotherwords,y[n]=x[n–1].uu415
M–1æ1–WnMöæö1kn1çM÷1ç1–1÷10.4c[n]=MåWM=Mçn÷.Hence,ifn¹rM,c[n]=Mçn÷=0.Ontheotherk=0è1–WMøè1–WMøM–1M–1M–11kn1krM1hand,ifn=rM,thenc[n]=MåWM=MåWM=Må1=1.Thus,k=0k=0k=01,ifn=rM,c[n]={0,otherwise.10.5M–1L1L/MkLFortheleft-handsidefigure,wehaveV1(z)=X(z),Y1(z)=MåX(zWM).k=0M–1M–111/Mk1L/MkFortheright-handsidefigure,wehaveV2(z)=MåX(zWM),Y2(z)=MåX(zWM).k=0k=0kkLSinceLandMarerelativelyprime,WandWtakethesamesetofvaluesfork=0,1,...,MMM–1.Hence,Y1(z)=Y2(z).10.6M–1M–111/Mk11/MkFortheleft-handsidefigure,wehaveV1(z)=MåX(zWM),Y1(z)=MåH(z)X(zWM),k=0k=0M–1M1kM1/MkFortheright-handsidefigure,wehaveV2(z)=H(z)X(z),Y2(z)=MåH(zWM)X(zWM)k=0M–111/Mk=MåH(z)X(zWM).Hence,Y1(z)=Y2(z).k=0LLLFortheleft-handsidefigure,wehaveV(z)=X(z),Y(z)=H(z)X(z).Fortheright-hand11LLsidefigure,wehaveV(z)=H(z)X(z),Y(z)=H(z)X(z).Hence,Y1(z)=Y2(z).2210.7x[n]5102y[n]ºx1[n]x[n]5522y[n]ºx[n]22y[n]416
ìx1[n/2],forn=2r,ìx[n],forn=2r,Hence,x1[n]=x[2n]andy[n]=í=íTherefore,î0,otherwiseî0,otherwiseìx[n],forn=2r,y[n]=íî0,otherwise.10.8H0(z)y0[n]w[n]u[n]x[n]33H1(z)y1[n]H2(z)y2[n]10.9AsoutlinedinSection6.3,thetransposeofadigitalfilterstructureisobtainedbyreversingallpaths,replacingthepick-offnodewithanadderandvice-versa,andinterchangingtheinputandtheoutputnodes.Moreover,inamultiratestructure,thetransposeofafactor-of-Mdown-samplerisafactor-of-Mup-samplerandvice-versa.Applyingtheseoperationstothefactor-of-Mdecimatorshownontheleft-handside,wearriveatafactor-of-Minterpolatorasindicatedontheright-handsideinthefigurebelow.10.10ApplyingthetransposeoperationtotheM-channelanalysisfilterbankshownbelowontheleft-handside,wearriveattheM-channelsynthesisfilterbankshownbelowontheright-handside.417
x[n]H0(z)Mv0[n]y[n]H0(z)Mv0[n]H1(z)Mv1[n]H1(z)Mv1[n]ºHM-1(z)MvM-1[n]HM-1(z)MvM-1[n]v0[n]MH0(z)y[n]ºv1[n]MH1(z)vM-1[n]MHM-1(z)10.11SpecificationsforH(z)areasfollows:Fp=180Hz,Fs=200Hz,dp=0.002,ds=0.001.H(z)3012kHz12kHz400Hz6WerealizeH(z)asH(z)=G(z)F(z).6F(z)G(z)6512kHz12kHz12kHz2kHz400HzTherefore,specificationsforG(z)areasfollows:120Fp=1080Hz,Fs=1200Hz,dp=0.001,ds=0.001.Here,Df=.Hence,from12000–20log100.001´0.001–1347´12000Eq.(7.15),orderofG(z)isgivenbyN===321.92.G14.6(120/12000)14.6´120Likewise,specificationsforF(z)are:Fp=180Hz,Fs=1800Hz,dp=0.001,ds=0.001.1620Here,Df=.Hence,orderofF(z)isgivenby12000–20log10–6–1347´1200010N===23.846.Thus,wechooseN=322andN=24.F14.6(1620/12000)14.6´1620GF2000RM,G=(322+1)´=129,200muliplications/second(mps),and512000RM,F=(24+1)´=50,000mps6418
Hence,totalno.ofmps=179,200.HencethecomputationalcomplexityofthisparticularIFIRimplementationisslightlyhigherherethanthatinExample10.8.F(z)6G(z)512kHz12kHz2kHz2kHz400Hz510.12WerealizeH(z)asH(z)=G(z)F(z).5F(z)G(z)5612kHz12kHz12kHz2.4kHz400HzSpecificationsforG(z)are:Fp=900Hz,Fs=1000Hz,dp=0.001,ds=0.001.Here,100Df=.Hence,fromEq.(7.15),orderofG(z)isgivenby12000–20log100.001´0.001–1347´12000N===386.3.Likewise,specificationsforF(z)G14.6(100/12000)14.6´1002020are:Fp=180Hz,Fs=2200Hz,dp=0.001,ds=0.001.Here,Df=.Hence,orderof12000–20log10–6–1347´1200010F(z)isgivenbyN===19.124.Thus,wechooseN=387F14.6(2020/12000)14.6´2020GandN=20.FF(z)5G(z)612kHz12kHz2.4kHz2.4kHz400Hz2400RM,G=(387+1)´=155,200muliplications/second(mps),and612000RM,F=(20+1)´=50,400mps5Hence,totalno.ofmps=205,600.HencethecomputationalcomplexityofthisparticularIFIRimplementationisslightlyhigherherethanthatinExample10.8andinProblem10.11.10.13H(z)2060kHz60kHz3kHzSpecificationsforH(z)are:F=1250kHz,F=1500kHz,d=0.02andd=0.01.Hence,pspsfromEq.(7.15),orderNofH(z)isgivenby–20log100.02´0.01–1323.989´60000N===394.34.WethuschooseN=395.14.6(250/60000)14.6´25060,000Computationalcomplexityistherefore=396´=1,188,000.20419
10.14OriginaldecimationfilterH(z)specifications:Fp=1250Hz,Fs=1500,dp=0.02,ds=0.01.5The4possibleIFIRimplementationsofH(z)areasfollows:(A)H(z)=G(z)F(z),4210(B)H(z)=G(z)F(z),(C)H(z)=G(z)F(z),and(D)H(z)=G(z)F(z).CaseA:SpecficationsforG(z):Fp=1250´5=6250Hz,Fs=1500´5=7500Hz,1250dp=0.01,ds=0.01.HereDf=.Hence,usingEq.(10.26),weobtaintheorderofG(z)60,000-20log100.01´0.01-13asNG==88.767.14.6(1250/60000)60000-5´1500SpecficationsforF(z):Fp=1250Hz,Fs==10,500Hz,59250dp=0.01,ds=0.01.HereDf=.Hence,usingEq.(10.26),weobtaintheorderofF(z)60,000-20log100.01´0.01-13asNF==11.996.WethuschooseNG=89andNF=12.14.6(9250/60000)1200060000Hence,RM,G=(89+1)´=270,000mpsandRM,F=(12+1)´=156,000mps.45Hence,totalcomputationalcomplexity=426,000mps.CaseB:SpecficationsforG(z):Fp=1250´4=5000Hz,Fs=1500´4=9000Hz,1000dp=0.01,ds=0.01.HereDf=.Hence,usingEq.(10.26),weobtaintheorderofG(z)60,000-20log100.01´0.01-13NG==110.9614.6(1000/60000)60000-5´1500SpecficationsforF(z):Fp=1250Hz,Fs==10,500Hz,512250dp=0.01,ds=0.01.HereDf=.Hence,usingEq.(10.26),weobtaintheorderofF(z)60,000-20log100.01´0.01-13NF==0.0579.WethuschooseNG=111andNF=10.14.6(12250/60000)1500060000Hence,RM,G=(111+1)´=336,000mpsandRM,F=(10+1)´=165,000mps.54Hence,totalcomputationalcomplexity=501,000mps.CaseC:SpecficationsforG(z):Fp=1250´2=2500Hz,Fs=1500´2=3000Hz,500dp=0.01,ds=0.01.HereDf=.Hence,usingEq.(10.26),weobtaintheorderofG(z)60,000-20log100.01´0.01-13NG==221.92.14.6(500/60000)420
60000-2´1500SpecficationsforF(z):Fp=1250Hz,Fs==28,500Hz,212250dp=0.01,ds=0.01.HereDf=.Hence,usingEq.(10.26),weobtaintheorderofF(z)60,000-20log100.01´0.01-13NF==4.0179.WethuschooseNG=222andNF=5.14.6(27250/60000)3000060000Hence,RM,G=(222+1)´=669,000mpsandRM,F=(5+1)´=180,000mps.102Hence,totalcomputationalcomplexity=849,000mps.CaseD:SpecficationsforG(z):Fp=1250´10=12500Hz,Fs=1500´10=15000Hz,2500dp=0.01,ds=0.01.HereDf=.Hence,usingEq.(10.26),weobtaintheorderofG(z)60,000-20log100.01´0.01-13NG==44.384.14.6(2500/60000)60000-10´1500SpecficationsforF(z):Fp=1250Hz,Fs==4,500Hz,103250dp=0.01,ds=0.01.HereDf=.Hence,usingEq.(10.26),weobtaintheorderofF(z)60,000-20log100.01´0.01-13NF==34.141.WethuschooseNG=45andNF=35.14.6(3250/60000)600060000Hence,RM,G=(45+1)´=138,000mpsandRM,F=(35+1)´=216,000mps.210Hence,totalcomputationalcomplexity=354,000mps.Thus,CaseDhasthelowestcomputationalcomplexity.10.15(a)SpecificationsforH(z)are:F=200Hz,F=300Hz,d=0.002andd=0.004.psps15H(z)600Hz9kHz9kHz100Here,Df=.Hence,fromEq.(10.26),orderNofH(z)isgivenby9000–20log100.002´0.004–1337.969´9000N===234.06.WechooseN=235.Hence,14.6(100/9000)14.6´1009000computationalcomplexity=(235+1)´=141,600mps.155(b)WerealizeH(z)=G(z)F(z).3G(z)5F(z)600Hz1.8kHz1.8kHz9kHz9kHz421
SpecificationsforG(z)are:F=200Hz,F=300Hz,d=0.002andd=0.004.Here,psps100Df=.Hence,fromEq.(7.15),orderNofH(z)isgivenby9000p+pz–1p-pz–10110110.16(a)H(z)=.H(zW)=H(-z)=.Thus,11+dz–11211-dz–11111ép+pz–1p-pz–1ùp-pdz-2E(z2)=[H(z)+H(-z)]=ê01+01ú=011,and02112êë1+dz–11-dz–1úû1-d2z-211111ép+pz–1p-pz–1ù(p-pd)z-1z–1E(z2)=[H(z)-H(-z)]=ê01-01ú=101.Hence,atwo-12112êë1+dz–11-dz–1úû1-d2z-2111bandpolyphasedecompositionofH(z)isgivenby1æp-pdz-2öæp-pdöH(z)=ç011÷+z-1ç101÷.1çè1-d2z-2÷øçè1-d2z-2÷ø11–1–2–1–22+3.1z+1.5z12-3.1z+1.5z(b)H(z)=.H(zW)=H(-z)=.Thus,21+0.9z–1+0.8z–22221-0.9z–1+0.8z–211é2+3.1z–1+1.5z–22-3.1z–1+1.5z–2ùE(z2)=[H(z)+H(-z)]=ê+ú02222êë1+0.9z–1+0.8z–21-0.9z–1+0.8z–2úû–2–44+0.62z+2.4z=.1+0.79z–2+0.64z–411é2+3.1z–1+1.5z–22-3.1z–1+1.5z–2ùz-1E(z2)=[H(z)-H(-z)]=ê-ú12222êë1+0.9z–1+0.8z–21-0.9z–1+0.8z–2úû–1–32.6z+2.26z=.Hence,atwo-bandpolyphasedecompositionofH(z)isgivenby1+0.79z–2+0.64z–42æ4+0.62z–2+2.4z–4öæ–2öH(z)=ç÷+z-1ç2.6+2.26z÷.2çè1+0.79z–2+0.64z–4÷øçè1+0.79z–2+0.64z–4÷ø–1–2–3–1–2–32+3.1z+1.5z+4z2+3.1z+1.5z+4z(c)H(z)==.3(1–0.5z–1)(1+0.9z–1+0.8z–2)1+0.4z–1+0.35z–2-0.4z–3–1–2–32-3.1z+1.5z-4zH(-z)=.31-0.4z–1+0.35z–2+0.4z–311é2+3.1z–1+1.5z–2+4z–32-3.1z–1+1.5z–2-4z–3ùE(z2)=[H(z)+H(-z)]=ê+ú02332êë1+0.4z–1+0.35z–2-0.4z–31-0.4z–1+0.35z–2+0.4z–3úû–2–4–64+1.92z+0.33z+3.2z=.1+0.54z–2+0.4425z–4-0.16z–6422
11é2+3.1z–1+1.5z–2+4z–32-3.1z–1+1.5z–2-4z–3ùz-1E(z2)=[H(z)-H(-z)]=ê-ú12332êë1+0.4z–1+0.35z–2-0.4z–31-0.4z–1+0.35z–2+0.4z–3úû–1–3–54.6z+10.57z+4z=.Hence,atwo-bandpolyphasedecompositionofH(z)is1+0.54z–2+0.4425z–4-0.16z–63æ4+1.92z–2+0.33z–4+3.2z–6öæ–2+4z–4ögivenbyH(z)=ç÷+z-1ç4.6+10.57z÷3çè1+0.54z–2+0.4425z–4-0.16z–6÷øçè1+0.54z–2+0.4425z–4-0.16z–6÷øp+pz–1201-k310.17(a)H1(z)=–1=åzEk(z).Thus,1+dz1k=0éH(z)ùéùéE(z3)ùéùéE(z3)ùê1úê111úê0úê111úê0úêH(W1z)ú=ê1W-1W-2úêz-1E(z3)ú=ê1W-1W-2úêz-1E(z3)úorê13úê33úê-213úê33úê-213úêëH(W2z)úûêë1W-2W-4úûêzE(z)úêë1W-2W-1úûêzE(z)ú1333ë2û33ë2û-1éE(z3)ùéùéH(z)ùéùéH(z)ùê0úê111úê1ú1ê111úê1úêz-1E(z3)ú=ê1W-1W-2úêH(W1z)ú=ê1W1W2úêH(W1z)ú.Theefore,ê-213úê33úê13ú3ê33úê13úêzE(z)úêë1W-2W-1úûêëH(W2z)úûêë1W2W1úûêëH(W2z)úûë2û331333133112E(z)=[H(z)+H(zW)+H(zW)]03113131ép+pz-1p+pej2p/3z-1p+pej4p/3z-1ùp+pd2z-3=ê01+01+01ú=011.3êë1+dz-11+dej2p/3z-11+dej4p/3z-1úû1+d3z-31111-1311122zE(z)=[H(z)+WH(zW)+WH(zW)]1313133131ép+pz-1p+pej2p/3z-1p+pej4p/3z-1ùp-pd=ê01+ej2p/301+ej4p/301ú=z-1101.3êë1+dz-11+dej2p/3z-11+dej4p/3z-1úû1+d3z-31111-2312112zE(z)=[H(z)+WH(zW)+WH(zW)]2313133131ép+pz-1p+pej2p/3z-1p+pej4p/3z-1ù-pd+pd2=ê01+ej4p/301+ej2p/301ú=z-21101.3êë1+dz-11+dej2p/3z-11+dej4p/3z-1úû1+d3z-31111p+pd2z-1p-pd-pd+pd20111011101Hence,E(z)=,E(z)=,andE(z)=.01+d3z-111+d3z-121+d3z-1111–1–22+3.1z+1.5z(b)H(z)=.21+0.9z–1+0.8z–2éH(z)ùéùéE(z3)ùéE(z3)ùéùéH(z)ùê2úê111úê0úê0ú1ê111úê2úêH(W1z)ú=ê1W-1W-2úêz-1E(z3)úorêz-1E(z3)ú=ê1W1W2úêH(W1z)ú.ê23úê33úê-213úê-213ú3ê33úê23úêëH(W2z)úûêë1W-2W-1úûêzE(z)úêzE(z)úêë1W2W1úûêëH(W2z)úû2333ë2ûë2û3323423
3112Thus,E(z)=[H(z)+H(zW)+H(zW)]03223231é2+3.1z–1+1.5z–22+3.1ej2p/3z–1+1.5ej4p/3z–22+3.1ej4p/3z–1+1.5ej2p/3z–2ù=ê++ú3êë1+0.9z–1+0.8z–21+0.9ej2p/3z–1+0.8ej4p/3z–21+0.9ej4p/3z–1+0.8ej2p/3z–2úû–3–66-8.277z+2.88z=.1-1.431z–3+0.512z–6-1311122zE(z)=[H(z)+WH(zW)+WH(zW)]132323323–1–2j2p/3–1j4p/3–212+3.1z+1.5zj2p/32+3.1ez+1.5ez=[+e31+0.9z–1+0.8z–21+0.9ej2p/3z–1+0.8ej4p/3z–2j4p/3–1j2p/3–2–3j4p/32+3.1ez+1.5ez-13.9-2.811z+e]=z.1+0.9ej4p/3z–1+0.8ej2p/3z–21-1.431z–3+0.512z–6-2312112zE(z)=[H(z)+WH(zW)+WH(zW)]233333333–1–2j2p/3–1j4p/3–212+3.1z+1.5zj4p/32+3.1ez+1.5ez=[+e31+0.9z–1+0.8z–21+0.9ej2p/3z–1+0.8ej4p/3z–2j4p/3–1j2p/3–2–3j2p/32+3.1ez+1.5ez-2-3.81+2.712z+e]=z.Hence,1+0.9ej4p/3z–1+0.8ej2p/3z–21-1.431z–3+0.512z–6–1–2–16-8.277z+2.88z3.9-2.811zE(z)=,E(z)=,and01-1.431z–1+0.512z–211-1.431z–1+0.512z–2–1-3.81+2.712zE(z)=.21-1.431z–1+0.512z–210.18Acomputationallyefficientrealizationofthefactor-of-4decimatorisobtainedbyapplyinga4-branchpolyphasedecompositiontoH(z):4–14–24–34H(z)=E(z)+zE(z)+zE(z)+zE(z).0123andthenmovingthedown-samplerthroughthepolyphasefiltersresultinginw1[n]4E0(z)y[n]-1zw[n]24E1(z)-1zw[n]34E2(z)-1zw4[n]4E(z)3424
FurtherreductionincomputationalcomplexityisachievedbysharingcomonmultipliersifH(z)isalinear-phaseFIRfilter.Forexample,foralength-16TypeIFIRtransferfunction–1–2–3–4–5–6–7–8H(z)=h[0]+h[1]z+h[2]z+h[3]z+h[4]z+h[5]z+h[6]z+h[7]z+h[7]z–9–10–11–12–13–14–15+h[6]z+h[5]z+h[4]z+h[3]z+h[2]z+h[1]z+h[0]z,–1–2–3–1–2–3forwhichE(z)=h[0]+h[4]z+h[7]z+h[3]z,E(z)=h[1]+h[5]z+h[6]z+h[2]z,01–1–2–3–1–2–3E(z)=h[2]+h[6]z+h[5]z+h[1]z,andE(z)=h[3]+h[7]z+h[4]z+h[0]z.23FromtheabovefigureitfollowsthatY(z)=E(z)W(z)+E(z)W(z)+E(z)W(z)+E(z)W(z)01122334-3-1-2=h[0](W(z)+zW(z))+h[4](zW(z)+zW(z))1414-2-1-3+h[7](zW(z)+zW(z))+h[3](zW(z)+W(z))1414-3-1-2+h[1](W(z)+zW(z))+h[5](zW(z)+zW(z))2323-2-1-3+h[6](zW(z)+zW(z))+h[2](zW(z)+W(z)).2323Acomputationallyefficientfactor-of-4decimatorstructurebasedontheaboveequationisthenasshownbelow:h[0]h[4]h[7]w[n]x[n]41-1-1-1zzzh[3]y[n]-1z-1-1-1zzzw[n]44h[1]h[5]-1zh[6]w2[n]-1-1-14zzzh[2]-1z-1-1-1zzzw[n]3410.19Acomputationallyefficientrealizationofthefactor-of-3interpolatorisobtainedbyapplyinga3-branchTypeIIpolyphasedecompositiontotheinterpolationfilterH(z):3–13–23H(z)=R(z)+zR(z)+zR(z),210andthenmovingtheup-samplerthroughthepolyphasefiltersresultingin425
w[n]1x[n]R30(z)-1zw2[n]R31(z)-1zw[n]3R(z)32Fromtheabovefigureitfollowsthat-1-2-3-4W(z)=h[0]X(z)+h[3]zX(z)+h[6]zX(z)+h[5]zX(z)+h[2]zX(z),3-1-2-3-4W(z)=h[2]X(z)+h[5]zX(z)+h[6]zX(z)+h[3]zX(z)+h[0]zX(z),and1-4-1-3-2W(z)=h[1](X(z)+zX(z))+h[4](zX(z)+zX(z))+h[7]zX(z).2Acomputationallyefficientfactor-of-3interpolatorstructurebasedontheaboveequationsisthenasshownbelow:w1[n]z-1z-1z-1z-13y[n]h[2]h[5]h[6]h[3]h[0]z-1x[n]-1zw3[n]z-1z-1z-1z-13-1-1zzh[7]-1-1zzh[4]3w2[n]h[1]N-1-i-1-2-3-(N-2)-(N-1)10.20H(z)=åz=(1+z)+(z+z)+L+(z+z)i=0(N/2)-1-1-2-4-(N-2)-12-2i=(1+z)(1+z+z+L+z)=(1+z)G(z)whereG(z)=åz.Usingai=0æ(N/4)-1ösimilartechniquewecanshowthatG(z)=(1+z-1)çåz-2i÷.Thereforewecanwriteç÷èi=0øæ(N/4)-1ö(N/4)-1H(z)=(1+z-1)(1+z-2)çåz-2i÷=(1+z-1)(1+z-2)F(z4)whereF(z)=åz-i.Continuingç÷èi=0øi=0426
-1-2-2K-1thisdecompositionprocessfurtherwearriveatH(z)=(1+z)(1+z)L(1+z),whereKN=2.Realizationofafactor-of-16decimatorusingabox-cardecimationfilterisasindicatedbelow:x[n]16z–1y[n]–1z16–1z16–1z1610.21Letu[n]denotetheoutputofthefactor-of-Linterpolator.Then,¥2å(u[n]-u[n-1])n=-¥E=(1)¥2åu[n]n=-¥¥åu[n]u[n-1]n=-¥andC=.(2)¥2åu[n]n=-¥SubstitutingEq.(2)inEq.(1)wegetE=2(1-C).Hence,asC®1,i.e.,asthesignalu[n]becomeshighlycorrelated,E®0.¥p1jwjwjwNow,byParseval"srelation,åu[n]v[n]=òU(e)V*(e)dw,whereU(e)and2pn=-¥-pjwV(e)aretheDTFTsofu[n]andv[n],respectively.Ifweletv[n]=u[n–1]inthenumeratorofEq.(1)andv[n]=u[n]inthedenominatorofEq.(1),thenwecanwritepp1jw2jwjw2òU(e)edwòU(e)cos(w)dw2p-p0E==,pp122jwjwòU(e)dwòU(e)dw2p-p0assumingu[n]tobearealsequence.Ifx[n]isassumedtobeabroadbandsignalwithaflatjwmagnitudespectrum,i.e.,X(e)=1for0£w£p,thenthemagnitudespectrumofu[n]is427
ì1,for0£w
ws)val=val+abs(H0(k))^2;endendDuetothenon-linearnatureofthefunctiontobeoptimized,differentvaluesofkinitshouldbeusedtooptimizetheanalysisfilter"sgainresponse.Thegainresponsesofthetwoanalysisfiltersisasshownbelow.Fromthegainresponse,theminimumstopbandattenuationoftheanalysisfiltersisobservedtobeabout24dB.Gainresponseoftheanalysisfilters100-10-20-30-40-5000.20.40.60.81w/pM10.16TheMATLABprogramusedtogeneratetheprototypelowpassfilterandtheanalysisfiltersofthe4-channeluniformDFTfilterbankisgivenbelow:L=21;f=[00.20.31];m=[1100];w=[101];N=4;WN=exp(-2*pi*j/N);plottag=["-";"--";"-.";":"];h=zeros(N,L);n=0:L-1;h(1,:)=remez(L-1,f,m,w);fori=1:N-1h(i+1,:)=h(1,:).*(WN.^(-i*n));end;clf;fori=1:N[H,w]=freqz(h(i,:),1,256,"whole");plot(w/pi,abs(H),plottag(i,:));holdon;end;gridon;holdoff;xlabel("Normalizedfrequency");ylabel("Magnitude");title("MagnituderesponsesofuniformDFTanalysisfilterbank");Theplotsgeneratedbytheaboveprogramisgivenbelow:465 M10.17Thefirst8impulseresponsecoefficientsofJohnston"s16AlowpassfilterHL(z)aregivenby0.001050167,–0.005054526,–0.002589756,0.0276414,–0.009666376,–0.09039223,0.09779817,0.4810284Theremaining8coefficientsaregivenbyflippingthecoefficientslefttoright,FromEq.(10.157),thehighpassfilterinthetree-structured3-channelfilterbankisgivenbyH2(z)=z–15HL(z–1).ThetworemainingfiltersaregivenbyH0(z)=HL(z)HL(z2)andH1(z)=HL(z)HH(z2).TheMATLABprogramusedtogeneratethegainplotsofthe3analysisfiltersisgivenby:G1=[0.10501670e-2-0.50545260e-2-0.25897560e-20.27641400e-1-0.96663760e-2-0.90392230e-10.97798170e-10.48102840];G=[G1fliplr(G1)];n=0:15;H0=(-1).^n.*G;Hsqar=zeros(1,31);Gsqar=zeros(1,31);Hsqar(1:2:31)=H0;Gsqar(1:2:31)=G;H1=conv(Hsqar,G);H2=conv(Gsqar,G);[h0,w0]=freqz(H0,[1]);[h1,w1]=freqz(H1,[1]);[h2,w2]=freqz(H2,[1]);plot(w0/pi,20*log10(abs(h0)),"b-",w1/pi,20*log10(abs(h1)),"r-",w2/pi,20*log10(abs(h2)),"g-.");axis([01-12020]);gridon;xlabel("NormalizedFrequency");ylabel("GainindB");Theplotsgeneratedaregivenbelow:466 20H(z)H(z)H(z)0210-20-40-60-8000.20.40.60.81w/p467 Chapter11(2e)750011.1IfR=1250,thenthefrequencyresolution==6Hz.12507500Iffrequencyresolution=4.5Hz,thenR==1667points.4.5800011.2(a)resolution==31.25Hz.2568000(b)Weneedtotakea=500-pointDFT.1680008000(c)resolution=.HencedesiredlengthNoftheDFTisgivenbyN==62.5.N128inceNmustbeanintegerwechooseN=63astheDFTsize.kF200´104kF350´104TT11.3(a)F===2000Hz,F===3500Hz,200R1000350R1000kF824´104TF===8240.824R1000kF195´14´103kF339´14´103TT(b)F===2702.97Hz,F===4699Hz,195R1010339R1010kF917´14´103TF===12710.89.917R1010kF97´104kF187´104TT(c)F===1876.2,F===3617.02,97R517187R517kF301´104TF===5822.05.301R51711.4Letthesamplessequenceberepresentedasg[n]=cos(wn+f).Then,itsDTFTisgivenbyo¥jwjf-jfG(e)=på(ed(w-wo+2pl)+ed(w+wo+2pl)).l=-¥1,0£n£N-1,Thewindowedsequenceisgivenbyg[n]=g[n]×w[n]wherew[n]=The{0,otherwise.DTFTofthewindowedsequenceisthengivenbyjw1jfj(w-w)1-jfj(w+w)jwG(e)=eY(eo)+eY(eo)whereY(e)istheDTFTofw[n]andis2R2RRjw-jw(N-1)/2sin(wN/2)givenbyY(e)=e.Rsin(w/2)jw2pkTheDFTG[k]isasampledversionofG(e)sampledatw=.TopreventleakagekN2plWphenomenonwerequirethatw=forsomeintegerl.Noww=whereWistheoNoFT 2plFTangularfrequencyoftheanalogsignal.IfW=,thentheCTFTG(jW)oftheanalogNasignalcanbedeterminedfromG[k].o11.5g(t)=cos(200pt).IfwerequireG[k]=0forallvaluesofkexceptk=64andk=448,wea2plFTrequireW=200ptobeoftheformW=forl=64.ThereforeooN2p´64´FT200p=orF=800Hz.512T1200011.6(a)F=6kHz.LetF=12.Theresolution=F/R.Hence,R==4616.mTT2.612000(b)R==4000.Theclosestpower-of-2to4000isR=4096.Hence,a4096-pointFFT3shouldbeused.11.7x[n]=Acos(2pfn/64)+Bcos(2pfn/64).SinceX[k]=0forallvaluesofkexceptk=15,122pf12p´152pf12p´2727,37and49,itfollowsthenthateither=or=.Therefore,64646464f=15or27.1Case(i):f=15,A=64,andf=27,B=32.Therefore,12x[n]=64cos(30pn/64)+32cos(54pn/64).Case(ii):f=27,A=32,andf=15,B=64.Therefore,12x[n]=32cos(54pn/64)+64cos(30pn/64).Thus,therearetwopossiblesolutionsasindicatedabove.211.8(a)AdirectDFTevaluation(noFFT)requiresN=250,000complexmultiplicationsandN(N-1)=249,500complexadditions.1(b)Digitalresolution=R==0.002.500100(c)Analogfrequencyresolution===0.2Hz.500FT(d)Thestopbandedgeofthefiltershouldbeat=50Hz.231(e)DigitalfrequencycorrespondingtotheDFTsampleX[31]==0.062andthat500390correspondingtotheDFTsampleX[390]==0.78.50031AnalogfrequencycorrespondingtotheDFTsampleX[31]=2p´´100=12.4prad/sand500390thatcorrespondingtotheDFTsampleX[390]=2p´´100=156prad/s.500 (f)lengthofFFT=512,andtherefore12zer-valuedsamplesshouldbeappended.512(g)TheFFTsampleindexthatisclosesttotheoldDFTsampleX[31]isk=31´=32and500512theFFTsampleindexthatisclosesttotheoldDFTsampleX[390]isk=390´@399.36500andhencek=399istheclosest.11.9Inodertodistinguishtwocloselyspacedsinusoidswerequirethattheseparationbetweenthetwofrequenciesbeatleasthalfofthemain-lobewidthofthewindowbeingused.FromTable7.3,themain-lobewidthsofthe4windowsaregivenby:4p4p2(a)Rectangularwindow:Dw==.Therefore,f>f+=0.283.N6021608p8p4(b)Hammingwindow:Dw==.Therefore,f>f+=0.316.N6021608p8p4(c)Hannwindow:Dw==.Therefore,f>f+=0.316.N60216012p12p6(d)Blackmanwindow:Dw==.Therefore,f>f+=0.35.N60216024411.10(a)f>f+=0.268.(b)f>f+=0.286.(c)f>f+=0.286.2111021110211106(d)f>f+=0.304.2111011.11(a)F³2F.Hence,theminimumsamplingfrequencyisF=2F.TmT,minmFT(b)R=£DF.ThereforeF£N(DF).HencethemaximumsamplingfrequencyisNTF=N(DF).T,max(c)IfF=4kHz,thenF=8kHz.mT,minllIfDF=10HzandN=2,thenF=2(10).T,max¥jw-jwm11.12(a)XSTFT(e,n)=åx[n–m]w[m]e.m=–¥¥¥jw-jwm-jwmGSTFT(e,n)=åg[n–m]w[m]e=å(ax[n–m]+by[n–m])w[m]em=–¥m=–¥¥¥-jwm-jwmjwjw=aåx[n–m]w[m]e+båy[n–m]w[m]e=aXSTFT(e,n)+bYSTFT(e,n).m=–¥m=–¥ ¥jw-jwm(b)y[n]=x[n–no].Hence,YSTFT(e,n)=åy[n–m]w[m]em=–¥¥-jwmjw=åx[n–no-m]w[m]e=XSTFT(e,n–no).m=–¥¥jwjw-jwm(c)y[n]=eox[n].Hence,Y(e,n)=åy[n–m]w[m]eSTFTm=–¥¥-j(w-w)mj(w-w)=åx[n-m]w[m]eo=X(eo,n).STFTm=–¥¥jw-jwm11.13XSTFT(e,n)=åx[n–m]w[m]e.Replacingminthisexpressionwithn–mwem=–¥¥¥jw-jwn-jwm-jwn-jwmarriveatXSTFT(e,n)=åx[m]w[n–m]ee=eåx[m]w[n–m]em=–¥m=–¥-jwn-jwjw-jwn-jweX(e,n).Hence,X(e,n)=eX(e,n).Thus,incomputingSTFTSTFTSTFTjwX(e,n)theinputx[n]isshiftedthroughthewindoww[n],whereas,incomputingSTFTjwX(e,n)thewindoww[n]isshiftedthroughtheinputx[n].STFT¥jw-jwm11.14XSTFT(e,n)=åx[m]w[n–m]e.Hence,byinverseDTFTweobtainm=–¥2p1jwjwmx[m]w[n-m]=X(e,n)edw.Therefore,2pòSTFT0¥2p¥1jwjwmåx[m]w[n-m]=2pòåXSTFT(e,n)edw,whichisequivalentton=–¥0n=–¥¥2p¥1jwjwmx[m]åw[n-m]=x[m]W[0]=2pòåXSTFT(e,n)edw,wheren=–¥0n=–¥¥¥2p¥1jwjwmW[0]=åw[n–m]=åw[n]orx[m]=2pW[0]òåXSTFT(e,n)edw.n=–¥n=–¥0n=–¥11.15FromthealternatedefinitionoftheSTFTgiveniProblem11.13wehave¥jw-jwmXSTFT(e,l)=åx[m]w[l-m]e.Therefore,m=-¥ ¥¥¥jwjwn-jw(m-n)åXSTFT(e,l)e=ååx[m]w[l-m]e.Hence,l=-¥l=-¥m=-¥2p¥¥¥2pjwjwn-jw(m-n)òåXSTFT(e,l)edw=ååx[m]w[l-m]òedw.0l=-¥l=-¥m=-¥02pjwk2p,ifk=0,Now,òedw={0,ifk¹0.Thus,,0¥¥2p¥jwjwnååx[m]w[l-m]×2pd[m-n]=òåXSTFT(e,l)edw,orl=-¥m=-¥0l=-¥¥2p¥jwjwn2påw[l-n]×x[n]=òåXSTFT(e,l)edw.l=-¥0l=-¥2p¥¥1jwjwnLetW[0]=ån=-¥w[n].Thenx[n]=2pW[0]òåXSTFT(e,l)edw.0l=-¥¥jw-jwm11.16XSTFT(e,n)=åx[n–m]w[m]e.Hence,m=–¥¥-j2pkm/N-j2pkn/NXSTFT[k,n]=åx[n–m]w[m]e=x[n]*w[n]e.Orinotherwords,m=–¥X[k,n]canbeobtainedbyfilteringx[n]byanLTIdystemwithanimpulseresponseSTFT-j2pkn/Nhk[n]=w[n]easindicatedinFigureP11.1.¥jw-jwm11.17XSTFT(e,n)=åx[n–m]w[m]e.Hence,m=–¥¥¥2jw-jwm-jwsXSTFT(e,n)=ååx[n–m]x[n–s]w[m]w[s]ee.Thus,s=–¥m=–¥2p¥¥1jw2jwkr[k,n]=2pòXSTFT(e,n)edw=ååx[n–m]x[n–s]w[m]w[s]d[s+k-m]0s=–¥m=–¥¥=åx[n–m]x[n–m+k]w[m]w[m-k].m=–¥11.18(a)LengthofthewindowN=FtT NFTt(b)ThenumberofcomplexmultiplicationsisC=logN=log(Ft).AnFFTis2222TperformedaftereveryKamples,i.eaftereveryK/Fseconds.Thus,thenumberofcomplexTCF2tTmultiplicationspersecondis=log(Ft).K/F2K2TT¥11.19jST[k,n]=åx[m]w[n-m]x[m+k]w[n-k-m].m=–¥¥(a)jST[-k,n]=åx[m]w[n-m]x[m-k]w[n+k-m].m=–¥Substituteintheaboveexpressionm–k=s,i.e.m=k+s.Thisyields¥jST[-k,n]=åx[s+k]w[n-k-s]x[s]w[n-s]=jST[k,n].s=–¥¥(b)Letm+k=s.Then,jST[k,n]=åx[s-k]x[s]w[n-s+k]w[n-s].Itfollowsfroms=–¥thisexpressionthatj[k,n]canbecomputedbyaconvolutionofhk[n]=w[n]w[n+k]withSTx[n]x[n–s]asindicatedinFigureP11.2.11.20–aX(z)Y(z)21–aS(z)–1za–12-1AnalysisyieldsS(z)=azS(z)+X(z),andY(z)=–aX(z)+(1-a)zS(z).SolvingtheX(z)firstequationwegetS(z)=,whichwhensubstitutedinthesecondequationyields-11-az-1Y(z)-a+zaftersomealgebra=.ThetransferfunctionisthusseentobeaType1X(z)1-az-1allpassoftheformofEq.(6.58)andcanthusberealizedusinganyoneofthesingle-multiplierstructuresofFigure6.36.-1Y(z)-a+zA(z)11.21AnalysisofthestructureofFigureP11.4yields=,whereA(z)denotestheX(z)1-az-1A(z)transferfunctionofthe"allpassreverberator".NotethatthisexpressionissimilarinformtothatofEq.(6.58)with"d"replacedby"-a"and"z–1"replacedby"z–1A(z)".HenceanefficientrealizationofthestructureofFigureP11.4alsoisobtainedreadilyfromanyoneofthestructuresofFigure6.36.Onesuchrealizationisindicatedbelow: –1X(z)z–1A(z)-aY(z)K1K2é1K11ù11.22G(z)={1-A(z)}+{1+A(z)}=Kê{1-A(z)}+{1+A(z)}ú.222222êë2K222úû2Henceinthiscase,theratioK1/K2determinestheamountofboostorcutatlowfrequencies,K2determinestheamountofdcgainorattenuationatallfrequencies,adeterminesthe-1æ2aö3-dBbandwidthDw3-dB=cosç2÷,andthecenterfrequencywoisrelatedtobè1+aøthroughb=cosw.oalpha=0.8;beta=0.4;K1=[0.9];K2=[0.52];nbp=((1-alpha)/2)*[10-1];dbp=[1-beta*(1+alpha)alpha];nbs=((1+alpha)/2)*[1-2*beta1];dbs=dbp;[Hlp,w]=freqz(nbp,dbp,512);[Hhp,w]=freqz(nbs,dbs,512);holdonfork=1:length(K1)form=1:length(K2)H=K1(k)*Hlp+K2(m)*Hhp;semilogx(w/pi,20*log10(abs(H)));xlabel("Gain,dB");ylabel("omega/pi");clearH;holdon;endendgridonaxis([.011-88]);11.23ThetransposeofthedecimatorstructureofFigure11.63yields x[n]2E00(z)3z–1E01(z)3z–1–1z–1zE02(z)32E10(z)3y[n]z–1E11(z)3z–1E12(z)311.243H(z)4R0(z)34R0(z)34-1-4zzR1(z)3R1(z)3z3-1-4zzR2(z)3R2(z)3z6(a)(b)R34R0(z)430(z)-1z-1z-1zzR1(z)34R1(z)43z-1z-1z-1zR2(z)34R2(z)43(c)(d) 4E20(z)3-1z4E21(z)-1zz-14Ez-122(z)-1z4E23(z)4E10(z)3-1z4E11(z)-1-1z-1zz4E12(z)-1z4E13(z)4E00(z)3-1z4E01(z)-1z4E02(z)-1z4E03(z)(e)M11.1FiguresbelowillustratetheapplicationofProgram11_1indetectingthetouch-tonedigitsAand3:Touch-ToneSymbol=ATouch-ToneSymbol=3M11.2ForR=16,thetwostrongpeaksoccuratk=3and5.Theassociatedfrequenciesare2p´335w=,orf==0.1875,andf==0.3125.ForR=32,thetwostrongpeaksoccurat116116216510k=5and10.Theassociatedfrequenciesaref==0.15625,andf==0.3125.132232 ForR=64,thetwostrongpeaksoccuratk=11and22.Theassociatedfrequenciesaref=11/64=0.1718,andf=20/64=0.3125.ForR=128,thetwostrongpeaksoccuratk=1221and39.Theassociatedfrequenciesaref=21/128=0.1641,andf=39/128=0.3047.12Moreover,thelasttwoplotsshowanumberofminorpeaksanditisnotclearbyexaminingtheseplotswhetherornotthereareothersinusoidsoflesserstrengthspresentinthesequencebeinganalyzed.AnincreaseinthesizeoftheDFTincreasestheresolutionofthespectralanalysisbyreducingtheseparationbetweenadjacentDFTsamples.Alsotheestimatedvaluesofthefrequenciesofthesinusoidgetclosertotheactualvaluesof0.167and0.3076asthesizeoftheDFTincreases.M11.3Astheseparationbetweenthetwofrequenciesdecreases,thedistancebetweenthetwomaximasintheDFTofthesequencedecreases,andwhenf2=0.21,thesecondsinusoidcannotbedeterminedfromtheDFTplot.Thisisduetotheuseofalength-16rectangularwindowtotruncatetheoriginalinfinite-lengthsequence.Foralength-16rectangularwindow,twoadjacentsinusoidscanbedistinguishediftheirangularfrequenciesareapartby4phalfthemainlobewidthofradiansorequivalently,iftheirfrequenciesareapartbyN2=0.0625.NotethattheDFTlengthR=128isallplots.N M11.4f2=0.21,f1=0.18.Hence,Df=0.03.ForaHammingwindowthemainlobewidth8pDML=.TheDFTlengthR=128inallplots.N(i)N=16.Hereitisnotpossibletodistinguishthetwosinusoids.Thisalsocanbeseen8fromthevalueofDML==0.5,andhence,halfofthemainlobewidthisgreaterthanDf.16(ii)IncreasingNto32,makestheseparationbetweenthetwopeaksvisible.However,itisdifficulttoidentifythepeaksaccurately. (iii)IncreasingNto64,makestheseparationbetweenthetwopeaksmorevisible.However,itisstilldifficulttoidentifythepeaksaccurately.(iv)ForN=128,theseparationbetweenthetwopeaksclearlyvisible.Notealsothesuppressionoftheminorpeaksduetotheuseofataperedwindow.M11.5ResultsaresimilartothatinProblemM11.4.M11.6f2=0.21,f1=0.18.Hence,Df=0.03.TheDFTlengthR=128inallplots. (i)ForN=16,itisdifficulttoidentifythetwosinusoids.(ii)ForN=32,therearetwopeaksclearlyvisibleatk=36and43,respectively.(iii)ForN=64,itisdifficulttoidentifythetwosinusoids.(iv)ForN=128,therearetwopeaksclearlyvisibleatk=37and41,respectively.M11.7 TheSNRcomputedbytheprogramis–7.4147dB.Thereisapeakatthefrequencyindex29whosenormalizedfrequencyequivalentisequalto29/256=0.1133.HencetheDFTapproachhascorrectlyidentifiedthefrequencyofthesinusoidcorruptedbythenoise.M11.8TheSNRcomputedbytheprogramis–7.7938dB.Thereisapeakatthefrequencyindex42whosenormalizedfrequencyequivalentisequalto42/256=0.1641.HencetheDFTapproachhascorrectlyidentifiedthefrequencyofthesinusoidcorruptedbythenoise.M11.9Thefollowingprogramcanbeusedtoplotthepowerspectrumestimatesofthenoisecorruptedsignalwindowedbyarectangularwindow.%PowerSpectrumEstimation%nfft=input("Typeinthefftsize=");n=0:1000;g=sin(0.1*pi*n)+sin(0.2*pi*n)+randn(size(n));window=boxcar(nfft);[Pxx,f]=psd(g,nfft,2,window);plot(f/2,10*log10(Pxx));gridxlabel("omega/pi");ylabel("PowerSpectrum,dB");titletext=sprintf("PowerSpectrumWithWindowSize=%d",nfft);title(titletext); Thepowerspectrumestimatewithwindowsize=64and256areshownbelowPowerSpectrumWithWindowSize=64PowerSpectrumWithWindowSize=25615201510105500-5-5-1000.10.20.30.40.500.10.20.30.40.5w/pw/pM11.10Program_11_4canbeusedtoevaluatetheBartlettandWelchestimatebychangingg=2*sin(0.12*pi*n)+sin(0.28*pi*n)+randn(size(n));tog=sin(0.1*pi*n)+sin(0.2*pi*n)+randn(size(n));andthelinewindow=hamming(256)withwindow=boxcar(1024)fortheBartlettestimate,andwithwindow=hanning(1024)fortheWelchestimate.Alsosetnfft=1024.TheplotoftheBartlettestimateofthepowerspectrumestimateforthenoisecorruptedsignalisshownbelowBartlettEstimate(Overalp=0samples)3020100-10-20-3000.10.20.30.40.5w/pTheWelchestimatesofthepowerspectrumwithanoverlappingHannwindowforoverlapsof64and128samplesareshownbelow WelchEstimate(Overalp=64samples)WelchEstimate(Overalp=128samples)30302020101000-10-10-20-20-30-3000.10.20.30.40.500.10.20.30.40.5w/pw/pM11.11Usethestatementb=remez(11,[00.30.51],[1100],[11]);todesigntheFIRfilter.Thenusethestatement[d,p0]=lpc(b,order);andrunitfororder=4,5and6todetermineanequivalentall-polemodel.ThemagnituderesponseoftheorignialFIRfilter(solidline)andtheall-poleequivalent(dottedline)areshownbelowfordifferentvaluesoforder.Orderofall-poleequivalent=4Orderofall-poleequivalent=51.51.5OriginalOriginalEstimateEstimate110.50.50000.20.40.60.8100.20.40.60.81w/pw/pOrderofall-poleequivalent=61.5OriginalEstimate10.5000.20.40.60.81w/p'
您可能关注的文档
- 国际市场营销学自学考试指导与题解 (甘碧群 著) 东北财经大学出版社 课后答案
- 操作系统 (陈向群 著) 北京大学出版社 课后答案
- 微型计算机原理及应用技术 第二版 (朱金钧 麻新旗 著) 机械工业出版社 课后答案
- 操作系统 (谭耀铭 著) 武汉大学出版社 课后答案
- 国家税收 第二版 (蒙丽珍 安仲文 著) 东北财经大学出版社 课后答案
- 操作系统概念 第六版 (Abraham Silberschatz Peter Baer Galvin Greg Gagne 著) 高等教育出版社 课后答案
- 微型计算机原理与接口技术 (王向慧 著) 中国水利水电出版社 课后答案
- 寒假生活指导 山东教育出版社 答案
- 分析化学_第六版_(华东理工_四川大学_著)_高等教育出版社_课后答案
- 微型计算机原理与接口技术 (张荣标 著) 机械工业出版社 课后答案
- 寒假作业 八年级 数学 (不详 著) 人民教育出版社 课后答案
- 微型计算机原理与接口技术 第三版 (杨立 邓振杰 荆淑霞 著) 中国铁道出版社 课后答案
- 《算法设计与分析》(王红梅)课后习题答案 清华大学出版社
- 操作系统概念 第七版 (Abraham Silberschatz Peter Baer Galvin Greg Gagne 著) 高等教育出版社 课后答案
- 宏观经济学 (多恩布什 著) 人民大学出版社 课后答案 第二篇 课后答案
- 操作系统设计与实现 上册 (Andrew S.Tanenbaum 著) 电子工业出版社 课后答案
- 微型计算机原理与接口技术 第三版 (周荷琴 著) 中国科学技术大学出版社 课后答案
- 《通信电子线路》(侯丽敏 著)课后习题答案 清华大学出版社
相关文档
- 施工规范CECS140-2002给水排水工程埋地管芯缠丝预应力混凝土管和预应力钢筒混凝土管管道结构设计规程
- 施工规范CECS141-2002给水排水工程埋地钢管管道结构设计规程
- 施工规范CECS142-2002给水排水工程埋地铸铁管管道结构设计规程
- 施工规范CECS143-2002给水排水工程埋地预制混凝土圆形管管道结构设计规程
- 施工规范CECS145-2002给水排水工程埋地矩形管管道结构设计规程
- 施工规范CECS190-2005给水排水工程埋地玻璃纤维增强塑料夹砂管管道结构设计规程
- cecs 140：2002 给水排水工程埋地管芯缠丝预应力混凝土管和预应力钢筒混凝土管管道结构设计规程（含条文说明）
- cecs 141：2002 给水排水工程埋地钢管管道结构设计规程 条文说明
- cecs 140：2002 给水排水工程埋地管芯缠丝预应力混凝土管和预应力钢筒混凝土管管道结构设计规程 条文说明
- cecs 142：2002 给水排水工程埋地铸铁管管道结构设计规程 条文说明
微信/支付宝扫码支付下载