某些线性微分方程的解析解和相应非线性方程的正解.pdf 16页

'˖ڍመ੾᝶஠ڙጲhttp://www.paper.edu.cn某些线性微分方程的解析解和相应非线性方程的正解赵增勤曲阜师范大学数学科学学院，曲阜273165摘要：对于某些具有泛函边界条件的线性微分方程，通过格林函数给出了解的解析表达式.利用得到的格林函数，研究了具有可数多个点的非局部边界条件和积分边界条件下的相应超线性方程正解的存在性.关键词：应用数学；解析解；线性泛函边界条件；格林函数；正解中图分类号：O175.14ExactsolutionsofsomelineardierentialequationsandpositivesolutionsofthecorrespondingnonlinearequationsZHAOZengqinSchoolofMathematicalSciences,QufuNormalUniversity,Qufu273165Abstract:ExactexpressionsofthesolutionsforsomelineardierentialequationswithfunctionalboundaryconditionsaregivenbytheGreen"sfunctions.UsingGreen"sfunctionsobtainedweinvestigateexistenceofpositivesolutionsforthecorrespondingsuperlinearequationswithcountablymanypointsandintegralboundaryconditions.Keywords:Appliedmathematics;Exactsolution;linearfunctionalboundarycondition;Green"sfunction;positivesolution.0IntroductionandthemainresultsThetheoryofnon-localboundaryvalueproblemshasbeenemergingasanimportantareaofinvestigationsinrecentyears.Theinvestigationofequationu00+f(t;u)=0withnon-localboundaryconditions(multi-pointorintegralboundaryconditions)canbeseenin[1,2,3,4,5,6,7,8]anditsreferences.Theinvestigationofone-dimensionalp-Laplaciannon-localboundaryproblemscanbeseenin[9,10,11,12]anditsreferences.Butconclusionsof基金项目：ResearchsupportedbytheNationalNaturalScienceFoundationofChina(11571197)，andtheDoctoralProgramFoundationofEducationMinistryofChina(20133705110003)作者简介：ZhaoZengqin(1955-),male，professor，majorresearchdirection：Nonlinearfunctionalanalysisanditsapplication.-1- ˖ڍመ੾᝶஠ڙጲhttp://www.paper.edu.cntheequation002u+ku=f(t;u);atb;(0.1)withnon-localboundaryconditionsareless,seenin[13,14].Thispaperrstinvestigatestheexactsolutionsofthelinearequation002u+ku=h(t);atb;(0.2)withtheboundaryconditions00u(a)u(a)=F1(u)+c1; u(b)+u(b)=F2(u)+c2:(0.3)WedenotetheuniquesolutionbyitsGreen"sfunction.Afterthat,weapplytheresultsobtainedtostudythenonlinearboundaryvalueproblem(0.1)(0.3).Werelatethefollowingsymbolsandterminologies.xxThehyperbolicsineandthehyperboliccosinearedenotedbysinh(x)=eeand2xxcosh(x)=e+e,respectively.LetEbearealBanachspace,Edenotethesetofboundedlin-2earfunctionalsonE.AssumethatF;F2(C[a;b]),x(t)2C[a;b],y(t;s)2C([a;b][a;b]).12WedenotetheimageofF1atx(t)byF1(x())orF1(x).F1()denotetheimageofF1atx(t)=t.F1(I)denotetheimageofF1atx(t)12C[a;b].Clearly,F1(y(t;))isafunctionoft,F2F1(y(t;))isarealnumber.AssumeDC[a;b].AfunctionalB:D!RissaidtobeincreasingifB(x1)B(x2)forx(t)x(t);x;x2D.AboundedlinearfunctionalF2(C[a;b])iscalledpositive1212ifF(x)0foranynonnegativefunctionx(t)2C[a;b].Everylinearpositivefunctionalisevidentlyincreasing.
Lemma1.[15]SupposethatEisaBanachspace,F2E,x(t)2C[a;b]!E.ThenZ!ZbbFx(s)ds=F(x(s))ds:aaLemma2.AssumethatF;F2(C[a;b]).Forthefunctionsinh(k(ts))2C([a;b][a;b]),12wehavethat2F2F1(sinh(k(t)))=2F1F2(sinh(k(t)))



(0.4)kkkk=F2e
F1eF2e
F1e:Inparticular,FiFi(sinh(k(t)))=0;i=1;2:(0.5)Proof.Weknowthatktksktks2sinh(k(ts))=e
ee
e:Therefore

ktkktk2F1(sinh(k(ts)))=e
F1ee
F1e;-2- ˖ڍመ੾᝶஠ڙጲhttp://www.paper.edu.cnhence2F2F1(sinh(k(t)))



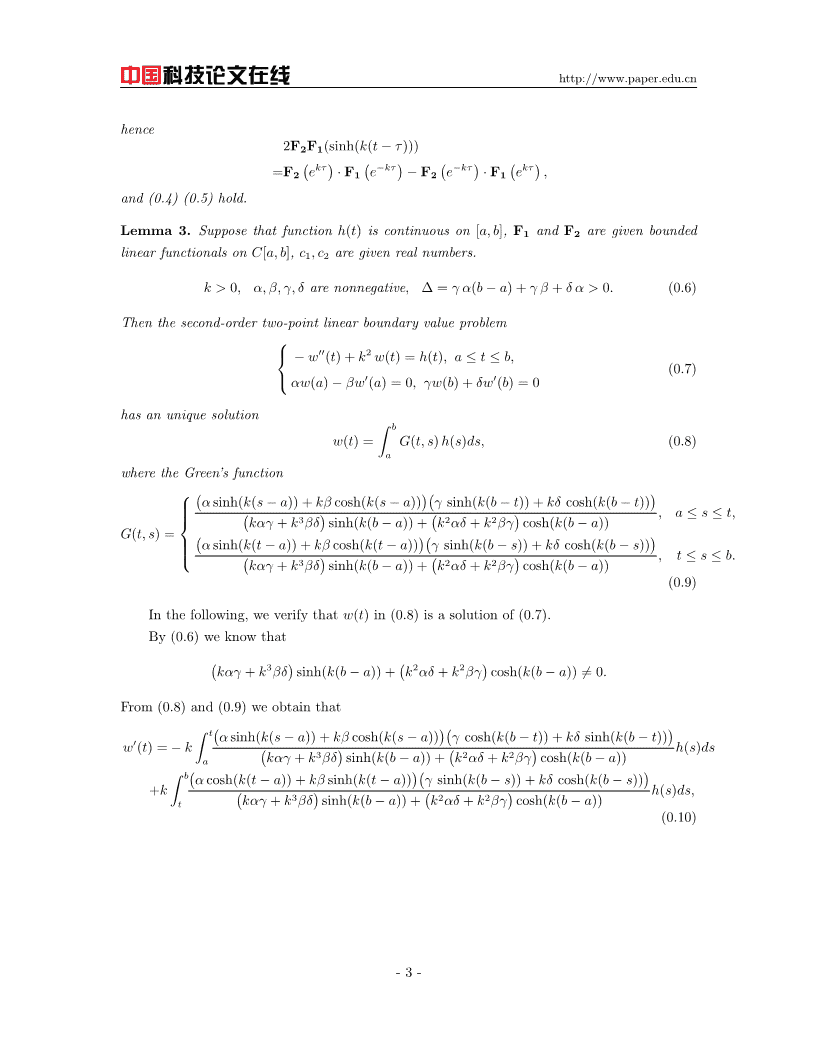
kkkk=F2e
F1eF2e
F1e;and(0.4)(0.5)hold.Lemma3.Supposethatfunctionh(t)iscontinuouson[a;b],F1andF2aregivenboundedlinearfunctionalsonC[a;b],c1;c2aregivenrealnumbers.k>0;;; ;arenonnegative;
=(ba)++>0:(0.6)Thenthesecond-ordertwo-pointlinearboundaryvalueproblem8<w00(t)+k2w(t)=h(t);atb;(0.7):w(a)w0(a)=0; w(b)+w0(b)=0hasanuniquesolutionZbw(t)=G(t;s)h(s)ds;(0.8)awheretheGreen"sfunction8

>>sinh(k(sa))+kcosh(k(sa))sinh(k(bt))+kcosh(k(bt))><

;ast;k +k3sinh(k(ba))+k2+k2cosh(k(ba))G(t;s)=

>>sinh(k(ta))+kcosh(k(ta))sinh(k(bs))+kcosh(k(bs))>:

;tsb:k +k3sinh(k(ba))+k2+k2cosh(k(ba))(0.9)Inthefollowing,weverifythatw(t)in(0.8)isasolutionof(0.7).By(0.6)weknowthat

322k +ksinh(k(ba))+k+kcosh(k(ba))6=0:From(0.8)and(0.9)weobtainthatZ

tsinh(k(sa))+kcosh(k(sa))cosh(k(bt))+ksinh(k(bt))0

w(t)=kh(s)dsak +k3sinh(k(ba))+k2+k2cosh(k(ba))Z

bcosh(k(ta))+ksinh(k(ta))sinh(k(bs))+kcosh(k(bs))+k

h(s)ds;tk +k3sinh(k(ba))+k2+k2cosh(k(ba))(0.10)-3- ˖ڍመ੾᝶஠ڙጲhttp://www.paper.edu.cnZ

tsinh(k(sa))+kcosh(k(sa))cosh(k(bt))+ksinh(k(bt))002

w(t)=kh(s)dsak +k3sinh(k(ba))+k2+k2cosh(k(ba))

sinh(k(ta))+kcosh(k(ta))cosh(k(bt))+ksinh(k(bt))k

h(t)k +k3sinh(k(ba))+k2+k2cosh(k(ba))Z

bcosh(k(ta))+ksinh(k(ta))sinh(k(bs))+kcosh(k(bs))2

+kh(s)dstk +k3sinh(k(ba))+k2+k2cosh(k(ba))

cosh(k(ta))+ksinh(k(ta))sinh(k(bt))+kcosh(k(bt))k

h(t)k +k3sinh(k(ba))+k2+k2cosh(k(ba))+(sinh(k(ta))cosh(k(bt))+cosh(k(ta))sinh(k(bt)))+k2(cosh(k(ta))sinh(k(bt))+sinh(k(ta))cosh(k(bt)))+k(sinh(k(ta))sinh(k(bt))+cosh(k(ta))cosh(k(bt)))+k (cosh(k(ta))cosh(k(bt))+sinh(k(ta))sinh(k(bt)))2

=kw(t)kh(t)k +k3sinh(k(ba))+k2+k2cosh(k(ba))2=kw(t)h(t):(0.11)By(0.8)(0.10)weobtainthatZ
bksinh(k(bs))+kcosh(k(bs))w(a)=

h(s)ds;(0.12)ak +k3sinh(k(ba))+k2+k2cosh(k(ba))Z
bksinh(k(bs))+kcosh(k(bs))0

w(a)=h(s)ds;(0.13)ak +k3sinh(k(ba))+k2+k2cosh(k(ba))Z
bsinh(k(sa))+kcosh(k(sa))kw(b)=

h(s)ds;(0.14)ak +k3sinh(k(ba))+k2+k2cosh(k(ba))Z
bsinh(k(sa))+kcosh(k(sa))k 0

w(b)=h(s)ds:(0.15)ak +k3sinh(k(ba))+k2+k2cosh(k(ba))Therefore,(0.11)|(0.15)implyw(t)isasolutionof(0.7).Assumethatw0(t)isasolutionofthehomogeneousproblemofcorrespondingtheproblem(0.7),thatis,8<w00(t)+k2w(t)=0;atb;00(0.16):w(a)w0(a)=0; w(b)+w0(b)=0:0000By(0.16)weobtainthatktkt0ktktw0(t)=c1e+c2e;w0(t)=c1kec2ke;t2[a;b];(0.17)wherec1;c2areconstantsthatwillbedetermined.From(0.17)wehavethat

0kakakakaw0(a)w0(a)=c1e+c2ec1kec2ke;

0kbkbkbkb w0(b)+w0(b)=c1e+c2e+c1kec2ke:-4- ˖ڍመ੾᝶஠ڙጲhttp://www.paper.edu.cnThesetogetherwith(0.16)andtheconditions(0.6)implythatc1=c2=0.Thereforew0(t)0by(0.17).Theuniquenessofsolutionsfor(0.7)followsfromthefactthatthecorrespondinghomogeneousproblem(0.16)hasonlythetrivialsolution.1ExactsolutionsoflineardierentialequationsWestateourrstresultsinthefollowingtheorem.Theorem1.Supposethatthefunctionh(t)iscontinuouson[a;b],F1andF2areboundedlinearfunctionalsinC[a;b].Inadditionassumethatthecondition(0.6)issatisedand(+k2)sinh(k(ba))+(k+k )cosh(k(ba))F1(sinh(k(b)))kF1(cosh(k(b)))(1.1)F2(sinh(k(a)))kF2(cosh(k(a)))+F2F1(sinh(k(t)))6=0:Thenthesecond-orderlinearboundaryvalueproblem8<u00(t)+k2u(t)=h(t);t2[a;b];(1.2):u(a)u0(a)=F(u)+c; u(b)+u0(b)=F(u)+c1122hasanuniquesolution(sinh(k(bt))+kcosh(k(bt))+F2(sinh(k(t))))(c1+F1(w))+(sinh(k(ta))+kcosh(k(ta))F1(sinh(k(t))))(c2+F2(w))u(t)=w(t)+;(+k2)sinh(k(ba))+(k+k )cosh(k(ba))F1(sinh(k(b)))kF1(cosh(k(b)))F2(sinh(k(a)))kF2(cosh(k(a)))+F2F1(sinh(k(t)))(1.3)wherew(t)isasin(0.8).Remark1.UsingLemma1,weknownthatZbFi(w)=Fi(G(;s))h(s)ds;i=1;2:aTherefore,(1.3)canbewrittenasc1(sinh(k(bt))+kcosh(k(bt))+F2(sinh(k(t))))Zb+c2(sinh(k(ta))+kcosh(k(ta))F1(sinh(k(t))))u(t)=+K(t;s)h(s)ds;2(+k)sinh(k(ba))+(k+k )cosh(k(ba))aF1(sinh(k(b)))kF1(cosh(k(b)))F2(sinh(k(a)))kF2(cosh(k(a)))+F2F1(sinh(k(t)))-5- ˖ڍመ੾᝶஠ڙጲhttp://www.paper.edu.cnwheretheGreen"sfunction(sinh(k(bt))+kcosh(k(bt))+F2(sinh(k(t))))F1(G(;s))+(sinh(k(ta))+kcosh(k(ta))F1(sinh(k(t))))F2(G(;s))K(t;s)=G(t;s)+:(+k2)sinh(k(ba))+(k+k )cosh(k(ba))F1(sinh(k(b)))kF1(cosh(k(b)))F2(sinh(k(a)))kF2(cosh(k(a)))+F2F1(sinh(k(t)))(1.4)Remark2.Thelinearfunctionalboundaryconditionspopularizesomenonlocalmulti-pointboundaryconditionsandintegralboundaryconditions.Forexample,X1X1Zb0A(v)=iv(i)+jv(j)+b(s)v(s)ds;i=1j=1aP1wherea<;1).IfthereexistsapositivenumbercsuchthatinffkAukju2P;kuk=cg>0,thenAhasaxedpointinPnfg.Now,weapplyCorollary1totheboundaryvalueproblem8>>u00+k2u=g(t;u);a>u(a)u0(a)=u(); u(b)+u0(b)=c(s)u(s)ds::iii=1awherea<i0;aai=1thereexist21>1suchthatforany00suchthatu(t)dG(t;t);t2[a;b]:(2.7)ProofofTheorem2.Weassumec1=c2=0,X1ZbF1(u)=iu(i);F2(u)=c(t)u(t)dt:(2.8)i=1ainCorollary1.Then81>>X>>F1(sinh(k(b)))=i(sinh(k(bi)));>>>>i=1>>1>>X>>>>F1(cosh(k(b)))=i(cosh(k(bi)));>>i=1>>F2(sinh(k(a)))=c(s)(sinh(k(sa)))ds;(2.9)>>a>>Zb>>>>F2(cosh(k(a)))=c(s)(cosh(k(sa)))ds;>>>>a!>>ZbX1>>>:F2F1(sinh(k(t)))=c(s)i(sinh(k(si)))ds:ai=1From(2.4)and(2.9)weknowthatthecondition(1.1)inCorollary1issatised.Therefore,theproblem(2.1)isequivalenttotheintegralequationZbu(t)=K(t;s)g(s;u(s))ds;(2.10)awhereK(t;s)isasin(1.4).-12- ˖ڍመ੾᝶஠ڙጲhttp://www.paper.edu.cnSinceF1andF2arepositivefunctionals,by(2.2)(2.3)and(2.8)wehavethat1sinh(k(t))XF1F1(I)=i+k;t2[a;b];(2.11)sinh(k(ta))i=1Zbsinh(k(t))F2F2(I)=c(s)ds+k;t2[a;b]:(2.12)sinh(k(bt))aFrom(2.11)weobtainthatF1(sinh(k(t)))(+k)sinh(k(ta));t2[a;b];hence0(+k)sinh(k(ta))F1(sinh(k(t)))sinh(k(ta))+kcosh(k(ta))F1(sinh(k(t)))(2.13)sinh(k(ba))+kcosh(k(ba))+F1(sinh(k(a)));t2[a;b]:From(2.12)weobtainthatF2(sinh(k(t)))(+k)sinh(k(bt));t2[a;b];hence0(+k)sinh(k(bt))+F2(sinh(k(t)))sinh(k(bt))+kcosh(k(bt))+F2(sinh(k(t)))(2.14)sinh(k(ba))+kcosh(k(ba))+F2(sinh(k(b)));t2[a;b]:Clearly,theG(t;s)in(0.9)satisfythatm1G(s;s)G(t;t)G(t;s)G(t;t);s;t2[a;b]:(2.15)where

k +k3sinh(k(ba))+k2+k2cosh(k(ba))m1=

:(2.16)sinh(k(ba))+kcosh(k(ba))sinh(k(ba))+kcosh(k(ba))From(2.8)weknowthatF1;F2areincreasingfunctionalonC[a;b].Thusby(1.4)(2.13),-13- ˖ڍመ੾᝶஠ڙጲhttp://www.paper.edu.cn(2.14)(2.15)and(2.3)weobtainthat(sinh(k(ba))+kcosh(k(ba))+F2(sinh(k(b))))F1(G(s;s))+(sinh(k(ba))+kcosh(k(ba))+F1(sinh(k(a))))F2(G(s;s))K(t;s)G(s;s)+(+k2)sinh(k(ba))+(k+k )cosh(k(ba))F1(sinh(k(b)))kF1(cosh(k(b)))F2(sinh(k(a)))kF2(cosh(k(a)))+F2F1(sinh(k(t)))(sinh(k(ba))+kcosh(k(ba))+F2(sinh(k(b))))F1(I)+(sinh(k(ba))+kcosh(k(ba))+F1(sinh(k(a))))F2(I)=1+G(s;s);(+k2)sinh(k(ba))+(k+k )cosh(k(ba))F1(sinh(k(b)))kF1(cosh(k(b)))F2(sinh(k(a)))kF2(cosh(k(a)))+F2F1(sinh(k(t)))(2.17)K(t;s)G(t;s)m1G(t;t)G(s;s)(2.18)From(2.17)and(2.18)weknowthatthereexistsapositivenumberM1suchthatm1G(t;t)G(s;s)K(t;s)M1G(s;s);t;s2[a;b]:(2.19)LetE=C[a;b],k
kdenotethesupnormofE,m1P=u(t)2Eu(t)kukG(t;t);(2.20)M1ZbTu(t)=K(t;s)g(s;u(s))ds;8u2P:(2.21)aWeonlywanttoprovefrom(2.10)thattheoperatorThasaxedpointinP+.Clearly,PEisanormalcone.Itiseasytoseeform(2.5)thatg(t;u)isnondecreasinginu.By(2.5)(2.15)(2.19)and(2.21)weknowthatZbTu(t)K(t;s)g(s;kuk)dsaZb2M1(1+kuk)G(s;s)g(s;1)ds;8u(t)2P:aThereforeTumakessensebythecondition(2.6).From(2.19)and(2.21)weobtainthatZbkTu(t)kM1G(s;s)g(s;u(s))ds;8u2P;aZbm1Tu(t)m1G(t;t)G(s;s)g(s;u(s))dskTukG(t;t);8u2P:aM1ThereforeT:P!P.-14- ˖ڍመ੾᝶஠ڙጲhttp://www.paper.edu.cnItiseasytoprovethatTisacompletelycontinuous,nondecreasingand1convexoperator.From(2.6)weknowthatthereexistsaninterval[a1;b1][a;b]suchthatG(t;t)
g(t;1)>0;t2[a1;b1]:(2.22)By(2.5)(2.19)and(2.21)weknowthatZb1T(G(t;t))m1G(t;t)G(s;s)g(s;G(s;s))dsa181;1a1thereG=maxfG(s;s)s2[a;b]g.Thistogetherwith(2.6)and(2.22)weknowthatkT(G(t;t))k>0:(2.23)Clearly,By(2.20)wehavethatm1m1u(t)kukG(t;t)=G(t;t);8u2P;kuk=1;M1M1hence2m1TuT(G(;));8u2P;kuk=1:M1Thisand(2.23)implyno2m1infkTukju2P;kuk=1kT(G(;))k>0:M1UsingLemma4weobtainthatThasaxedpointu2Pnfg.Therefore(2.1)hasanonneg-ativecontinuoussolutionu(t),whichisasolutionof(2.10),andu(t)satises(2.7).参考文献（References）[1]ZengqinZhao,SolutionsandGreen"sfunctionsforsomelinearsecond-orderthree-pointboundaryvalueproblems,Comput.Math.Appl.56(2008)104{113.[2]LingjuKong,QingkaiKong,JamesS.W.Wong,Nodalsolutionsofmulti-pointboundaryvalueproblems,NonlinearAnal.72(2010)382{389.[3]LiuYang,ChunfangShen,Ontheexistenceofpositivesolutionforakindofmulti-pointboundaryvalueproblematresonance,NonlinearAnal.72(2010)4211{4220.[4]XuemeiZhang,MeiqiangFeng,WeigaoGe,Multiplepositivesolutionsforaclassofm-pointboundaryvalueproblems,Appl.Math.Lett.22(2009)12{18.-15- ˖ڍመ੾᝶஠ڙጲhttp://www.paper.edu.cn[5]XuemeiZhang,MeiqiangFeng,WeigaoGe,Existenceresultofsecond-orderdierentiale-quationswithintegralboundaryconditionsatresonance,J.Math.Anal.Appl.353(2009)311{319.[6]LingjuKong,Secondordersingularboundaryvalueproblemswithintegralboundarycon-ditions,NonlinearAnal.72(2010)2628-2638.[7]RuyunMa,YulianAn,Globalstructureofpositivesolutionsfornonlocalboundaryvalueproblemsinvolvingintegralconditions,NonlinearAnal.71(2009)4364-4376.[8]AbdelkaderBoucherif,Second-orderboundaryvalueproblemswithintegralboundarycon-ditions,NonlinearAnal.70(2009)364{371.[9]YouweiZhang,Existenceandmultiplicityresultsforaclassofgeneralizedone-dimensionalp-Laplacianproblem,NonlinearAnal.72(2010)748{756.[10]Chan-GyunKim,Existenceofpositivesolutionsforsingularboundaryvalueproblemsinvolvingtheone-dimensionalp-Laplacian,NonlinearAnal.70(2009)4259{4267.[11]DehongJi,ZhanbingBai,WeigaoGe,Theexistenceofcountablymanypositivesolutionsforsingularmultipointboundaryvalueproblems,NonlinearAnal.72(2010)955{964.[12]JunfangZhao,WeigaoGe,Existenceresultsof2m-pointboundaryvalueproblemofSturm-Liouvilletypewithsignchangingnonlinearity,Math.Comput.Modelling49(2009)946{954.[13]ZengqinZhao,Exactsolutionsofaclassofsecond-ordernonlocalboundaryvalueproblemsandapplications,Appl.Math.Comput.215(2009)1926{1936.[14]ZengqinZhao,Existenceofxedpointsforsomeconvexoperatorsandapplicationstomulti-pointboundaryvalueproblems,Appl.Math.Comput.215(2009),2971{2977.[15]DajunGuo,NonlinearFunctionalAnalysis,Jinan:ShandongScienceandTechnologyPress,2001(inChinese).[16]ZengqinZhao,Fixedpointsof"convexoperatorsandapplications,Appl.Math.Lett.23(5)(2010)561{566.-16-'